Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 62227 by behi83417@gmail.com last updated on 17/Jun/19

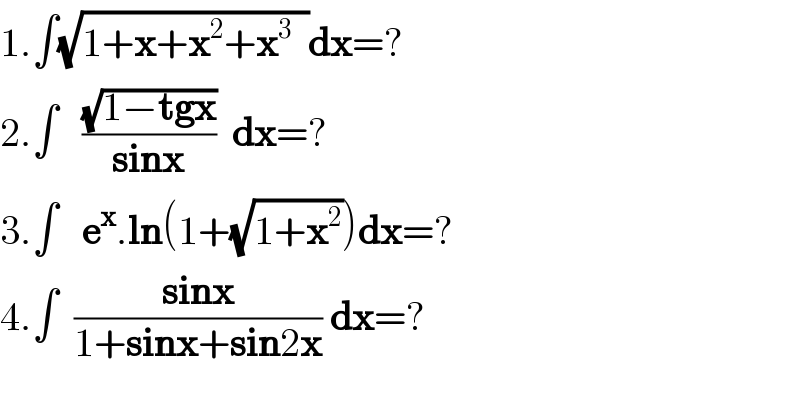
1.∫(√(1+x+x^2 +x^3 ))dx=? 2.∫ ((√(1−tgx))/(sinx)) dx=? 3.∫ e^x .ln(1+(√(1+x^2 )))dx=? 4.∫ ((sinx)/(1+sinx+sin2x)) dx=?

$$\mathrm{1}.\int\sqrt{\mathrm{1}+\boldsymbol{\mathrm{x}}+\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\boldsymbol{\mathrm{x}}^{\mathrm{3}} \:\:}\boldsymbol{\mathrm{dx}}=? \\ $$$$\mathrm{2}.\int\:\:\:\frac{\sqrt{\mathrm{1}−\boldsymbol{\mathrm{tgx}}}}{\boldsymbol{\mathrm{sinx}}}\:\:\boldsymbol{\mathrm{dx}}=? \\ $$$$\mathrm{3}.\int\:\:\:\boldsymbol{\mathrm{e}}^{\boldsymbol{\mathrm{x}}} .\boldsymbol{\mathrm{ln}}\left(\mathrm{1}+\sqrt{\mathrm{1}+\boldsymbol{\mathrm{x}}^{\mathrm{2}} }\right)\boldsymbol{\mathrm{dx}}=? \\ $$$$\mathrm{4}.\int\:\:\frac{\boldsymbol{\mathrm{sinx}}}{\mathrm{1}+\boldsymbol{\mathrm{sinx}}+\boldsymbol{\mathrm{sin}}\mathrm{2}\boldsymbol{\mathrm{x}}}\:\boldsymbol{\mathrm{dx}}=? \\ $$

Commented by maxmathsup by imad last updated on 19/Jun/19

4) let I =∫ ((sinx)/(1+sinx +sin(2x)))dx ⇒I = ∫ ((sinx)/(1+sinx +2sinx cosx))dx = ∫ (dx/((1/(sinx))+1 +2cosx)) dx =_(tan((x/2))=t) ∫ (1/(((1+t^2 )/(2t)) +1+2((1−t^2 )/(1+t^2 )))) ((2dt)/(1+t^2 )) = ∫ ((2dt)/((((1+t^2 )^2 )/(2t)) +1+t^2 +2−2t^2 )) dt =∫ ((2dt)/((((1+t^2 )^2 )/(2t)) +3−t^2 )) = ∫ ((4t dt)/((1+t^2 )^2 +6t−2t^3 )) = ∫ ((4tdt)/(t^4 +2t^2 +1+6t−2t^3 )) =∫ ((4tdt)/(t^4 −2t^3 +2t^2 +6t+1)) let decompose F(t) =((4t)/(t^4 −2t^3 +2t^2 +6t +1)) the roots of P(x) =t^4 −2t^3 +2t^2 +6t +1 are t_1 =−1 ,t_2 ∼−0,1795 t_3 ∼1,5898 +1,7445i (complex) t_4 ∼ 1,5898+1,7445i (complex) ⇒ F(t) =((4t)/((t+1)(t−t_2 )(t−t_3 )(t−t_3 ^− ))) =((4t)/((t+1)(t−t_2 )(t^2 −(t_3 +t_3 ^− )t +∣t_3 ∣^2 ))) ⇒F(t) =(a/(t+1)) +(b/(t−t_2 )) +((ct +d)/(t^2 −αt +β)) (α =t_3 +t_3 ^− and β =∣t_3 ∣^2 ) ⇒ ∫ F(t)dt =aln∣t+1∣ +bln∣t−t_2 ∣ +(c/2) ∫ ((2t−α +α)/(t^2 −αt +β)) + ∫ (d/(t^2 −αt +β)) dt =aln∣t+1∣ +bln∣t−t_2 ∣ +(c/2)ln∣t^2 −αt +β∣ +(((αc)/2) +d) ∫ (dt/(t^2 −αt +β)) ∫ (dt/(t^2 −αt +β)) =∫ (dt/(t^2 −2t(α/2) +β −(α^2 /4))) =∫ (dt/((t−(α/2))^2 +((4β−α^2 )/4))) =_(t−(α/2)=((√(4β−α^2 ))/2) u) (1/2)∫ (1/(((4β−α^2 )/4)(1+u^2 ))) (√(4β−α^2 ))du =(2/(√(4β−α^2 ))) arctan(u) +C =(2/(√(4β−α^2 ))) arctan(((2t−α)/(√(4β^2 −α^2 )))) ⇒ I =a ln∣1+tan((x/2))∣+bln∣tan((x/2))−t_2 ∣ +(c/2)ln∣tan^2 ((x/2))−αtan((x/2))+β∣ +(((αc)/2) +d) (2/(√(4β−α^2 ))) arctan(((2tan((x/2)))/(√(4β−α^2 )))) +C rest to calculate the value of a ,b,c and d ....

$$\left.\mathrm{4}\right)\:{let}\:{I}\:=\int\:\:\:\frac{{sinx}}{\mathrm{1}+{sinx}\:+{sin}\left(\mathrm{2}{x}\right)}{dx}\:\Rightarrow{I}\:=\:\int\:\:\:\:\frac{{sinx}}{\mathrm{1}+{sinx}\:+\mathrm{2}{sinx}\:{cosx}}{dx} \\ $$$$=\:\int\:\:\:\:\:\:\frac{{dx}}{\frac{\mathrm{1}}{{sinx}}+\mathrm{1}\:+\mathrm{2}{cosx}}\:{dx}\:=_{{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}} \:\:\:\:\:\:\int\:\:\:\:\:\frac{\mathrm{1}}{\frac{\mathrm{1}+{t}^{\mathrm{2}} }{\mathrm{2}{t}}\:+\mathrm{1}+\mathrm{2}\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$=\:\int\:\:\:\:\:\frac{\mathrm{2}{dt}}{\frac{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }{\mathrm{2}{t}}\:+\mathrm{1}+{t}^{\mathrm{2}} \:+\mathrm{2}−\mathrm{2}{t}^{\mathrm{2}} }\:{dt}\:=\int\:\:\:\frac{\mathrm{2}{dt}}{\frac{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }{\mathrm{2}{t}}\:+\mathrm{3}−{t}^{\mathrm{2}} } \\ $$$$=\:\int\:\:\:\:\frac{\mathrm{4}{t}\:{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} \:+\mathrm{6}{t}−\mathrm{2}{t}^{\mathrm{3}} }\:=\:\int\:\:\:\frac{\mathrm{4}{tdt}}{{t}^{\mathrm{4}} \:+\mathrm{2}{t}^{\mathrm{2}} \:+\mathrm{1}+\mathrm{6}{t}−\mathrm{2}{t}^{\mathrm{3}} } \\ $$$$=\int\:\:\:\frac{\mathrm{4}{tdt}}{{t}^{\mathrm{4}} −\mathrm{2}{t}^{\mathrm{3}} \:+\mathrm{2}{t}^{\mathrm{2}} \:+\mathrm{6}{t}+\mathrm{1}}\:\:{let}\:{decompose}\:{F}\left({t}\right)\:=\frac{\mathrm{4}{t}}{{t}^{\mathrm{4}} −\mathrm{2}{t}^{\mathrm{3}} \:+\mathrm{2}{t}^{\mathrm{2}} \:+\mathrm{6}{t}\:+\mathrm{1}} \\ $$$${the}\:{roots}\:{of}\:{P}\left({x}\right)\:={t}^{\mathrm{4}} −\mathrm{2}{t}^{\mathrm{3}} \:+\mathrm{2}{t}^{\mathrm{2}} \:+\mathrm{6}{t}\:+\mathrm{1}\:{are}\:{t}_{\mathrm{1}} =−\mathrm{1}\:\:,{t}_{\mathrm{2}} \sim−\mathrm{0},\mathrm{1795} \\ $$$${t}_{\mathrm{3}} \sim\mathrm{1},\mathrm{5898}\:+\mathrm{1},\mathrm{7445}{i}\:\:\left({complex}\right) \\ $$$${t}_{\mathrm{4}} \sim\:\mathrm{1},\mathrm{5898}+\mathrm{1},\mathrm{7445}{i}\:\left({complex}\right)\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{\mathrm{4}{t}}{\left({t}+\mathrm{1}\right)\left({t}−{t}_{\mathrm{2}} \right)\left({t}−{t}_{\mathrm{3}} \right)\left({t}−\overset{−} {{t}}_{\mathrm{3}} \right)}\:=\frac{\mathrm{4}{t}}{\left({t}+\mathrm{1}\right)\left({t}−{t}_{\mathrm{2}} \right)\left({t}^{\mathrm{2}} −\left({t}_{\mathrm{3}} +\overset{−} {{t}}_{\mathrm{3}} \right){t}\:+\mid{t}_{\mathrm{3}} \mid^{\mathrm{2}} \right)} \\ $$$$\Rightarrow{F}\left({t}\right)\:=\frac{{a}}{{t}+\mathrm{1}}\:+\frac{{b}}{{t}−{t}_{\mathrm{2}} }\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} −\alpha{t}\:+\beta}\:\:\:\:\:\:\:\:\left(\alpha\:={t}_{\mathrm{3}} +\overset{−} {{t}}_{\mathrm{3}} \:\:{and}\:\beta\:=\mid{t}_{\mathrm{3}} \mid^{\mathrm{2}} \right)\:\Rightarrow \\ $$$$\int\:{F}\left({t}\right){dt}\:={aln}\mid{t}+\mathrm{1}\mid\:+{bln}\mid{t}−{t}_{\mathrm{2}} \mid\:\:+\frac{{c}}{\mathrm{2}}\:\int\:\:\frac{\mathrm{2}{t}−\alpha\:+\alpha}{{t}^{\mathrm{2}} −\alpha{t}\:+\beta}\:+\:\int\:\:\frac{{d}}{{t}^{\mathrm{2}} −\alpha{t}\:+\beta}\:{dt} \\ $$$$={aln}\mid{t}+\mathrm{1}\mid\:+{bln}\mid{t}−{t}_{\mathrm{2}} \mid\:+\frac{{c}}{\mathrm{2}}{ln}\mid{t}^{\mathrm{2}} −\alpha{t}\:+\beta\mid\:\:+\left(\frac{\alpha{c}}{\mathrm{2}}\:+{d}\right)\:\int\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\alpha{t}\:+\beta} \\ $$$$\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\alpha{t}\:+\beta}\:=\int\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\mathrm{2}{t}\frac{\alpha}{\mathrm{2}}\:+\beta\:−\frac{\alpha^{\mathrm{2}} }{\mathrm{4}}}\:=\int\:\:\:\:\:\frac{{dt}}{\left({t}−\frac{\alpha}{\mathrm{2}}\right)^{\mathrm{2}} \:+\frac{\mathrm{4}\beta−\alpha^{\mathrm{2}} }{\mathrm{4}}} \\ $$$$=_{{t}−\frac{\alpha}{\mathrm{2}}=\frac{\sqrt{\mathrm{4}\beta−\alpha^{\mathrm{2}} }}{\mathrm{2}}\:{u}} \:\:\:\frac{\mathrm{1}}{\mathrm{2}}\int\:\:\:\:\:\:\frac{\mathrm{1}}{\frac{\mathrm{4}\beta−\alpha^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:\sqrt{\mathrm{4}\beta−\alpha^{\mathrm{2}} }{du}\:=\frac{\mathrm{2}}{\sqrt{\mathrm{4}\beta−\alpha^{\mathrm{2}} }}\:{arctan}\left({u}\right)\:\:+{C} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{4}\beta−\alpha^{\mathrm{2}} }}\:{arctan}\left(\frac{\mathrm{2}{t}−\alpha}{\sqrt{\mathrm{4}\beta^{\mathrm{2}} −\alpha^{\mathrm{2}} }}\right)\:\Rightarrow \\ $$$${I}\:={a}\:{ln}\mid\mathrm{1}+{tan}\left(\frac{{x}}{\mathrm{2}}\right)\mid+{bln}\mid{tan}\left(\frac{{x}}{\mathrm{2}}\right)−{t}_{\mathrm{2}} \mid\:+\frac{{c}}{\mathrm{2}}{ln}\mid{tan}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)−\alpha{tan}\left(\frac{{x}}{\mathrm{2}}\right)+\beta\mid \\ $$$$+\left(\frac{\alpha{c}}{\mathrm{2}}\:+{d}\right)\:\frac{\mathrm{2}}{\sqrt{\mathrm{4}\beta−\alpha^{\mathrm{2}} }}\:{arctan}\left(\frac{\mathrm{2}{tan}\left(\frac{{x}}{\mathrm{2}}\right)}{\sqrt{\mathrm{4}\beta−\alpha^{\mathrm{2}} }}\right)\:+{C}\:\:{rest}\:{to}\:{calculate}\:{the}\:{value}\:{of} \\ $$$${a}\:,{b},{c}\:{and}\:{d}\:.... \\ $$

Commented by behi83417@gmail.com last updated on 20/Jun/19

thanks in advance master proph. abdo! nice and hard work.

$$\mathrm{thanks}\:\mathrm{in}\:\mathrm{advance}\:\mathrm{master}\:\mathrm{proph}.\:\mathrm{abdo}! \\ $$$$\mathrm{nice}\:\mathrm{and}\:\mathrm{hard}\:\mathrm{work}. \\ $$

Commented by prof Abdo imad last updated on 20/Jun/19

you are welcome sir.

$${you}\:{are}\:{welcome}\:{sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com