
Question Number 62380 by ajfour last updated on 20/Jun/19
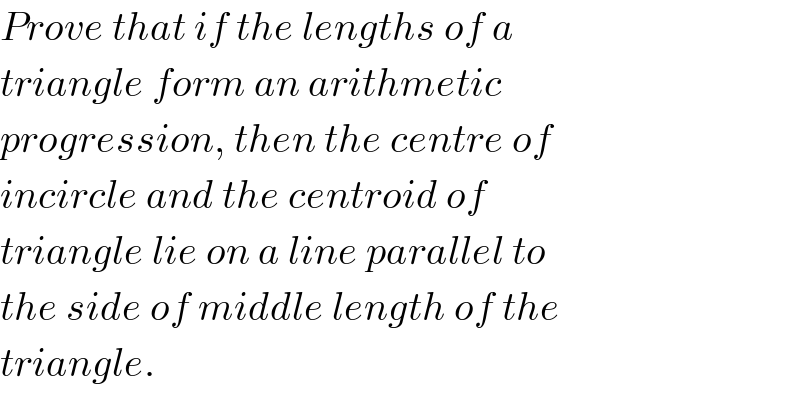
$${Prove}\:{that}\:{if}\:{the}\:{lengths}\:{of}\:{a}\: \\ $$$${triangle}\:{form}\:{an}\:{arithmetic} \\ $$$${progression},\:{then}\:{the}\:{centre}\:{of} \\ $$$${incircle}\:{and}\:{the}\:{centroid}\:{of} \\ $$$${triangle}\:{lie}\:{on}\:{a}\:{line}\:{parallel}\:{to} \\ $$$${the}\:{side}\:{of}\:{middle}\:{length}\:{of}\:{the} \\ $$$${triangle}. \\ $$
Answered by mr W last updated on 20/Jun/19

$${let}\:{the}\:{middle}\:{side}\:{length}\:{be}\:{a}, \\ $$$${then}\:{the}\:{other}\:{two}\:{sides}\:{are} \\ $$$${a}−{d}\:{and}\:{a}+{d}. \\ $$$${the}\:{perimeter}\:{of}\:{the}\:{triangle}\:{is} \\ $$$${p}={a}+\left({a}−{d}\right)+\left({a}+{d}\right)=\mathrm{3}{a}. \\ $$$${let}\:\Delta\:{be}\:{the}\:{area}\:{of}\:{the}\:{triangle}, \\ $$$${the}\:{radius}\:{of}\:{the}\:{incircle}\:{is}\:{r}, \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}{pr}=\Delta \\ $$$$\Rightarrow{r}=\frac{\mathrm{2}\Delta}{{p}}=\frac{\mathrm{2}\Delta}{\mathrm{3}{a}} \\ $$$${let}\:{h}={altitude}\:{over}\:{middle}\:{side} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}{ah}=\Delta \\ $$$$\Rightarrow{h}=\frac{\mathrm{2}\Delta}{{a}} \\ $$$${the}\:{distance}\:{of}\:{the}\:{centroid}\:{to}\:{the} \\ $$$${middle}\:{side}\:{is}\:\frac{{h}}{\mathrm{3}}=\frac{\mathrm{2}\Delta}{\mathrm{3}{a}},\:{which}\:{is} \\ $$$${equal}\:{to}\:{the}\:{radius}\:{of}\:{incircle}.\:{that} \\ $$$${means}\:{the}\:{center}\:{of}\:{incircle}\:{and}\:{the} \\ $$$${centroid}\:{have}\:{the}\:{same}\:{distance}\:{to} \\ $$$${the}\:{middle}\:{side},\:{i}.{e}.\:{they}\:{lie}\:{on}\:{a} \\ $$$${line}\:{parallel}\:{to}\:{the}\:{middle}\:{side}. \\ $$
Commented by ajfour last updated on 20/Jun/19

$${Thank}\:{you}\:{Sir},\:{very}\:{good}\:{approach}! \\ $$
