
Question Number 62428 by Tawa1 last updated on 21/Jun/19

Commented by mathsolverby Abdo last updated on 21/Jun/19

$${this}\:{question}\:{is}\:{done}\:{take}\:{a}\:{look}\:{at}\:{the} \\ $$$${platform} \\ $$
Commented by Tawa1 last updated on 21/Jun/19
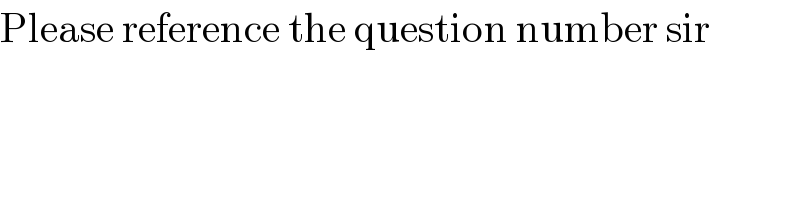
$$\mathrm{Please}\:\mathrm{reference}\:\mathrm{the}\:\mathrm{question}\:\mathrm{number}\:\mathrm{sir} \\ $$
Commented by mathmax by abdo last updated on 21/Jun/19
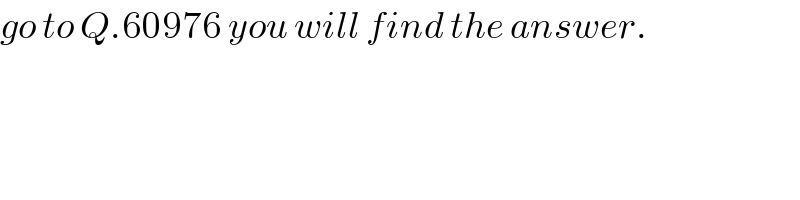
$${go}\:{to}\:{Q}.\mathrm{60976}\:{you}\:{will}\:{find}\:{the}\:{answer}. \\ $$
Commented by Tawa1 last updated on 21/Jun/19

$$\mathrm{I}\:\mathrm{searched}\:\mathrm{but}\:\mathrm{is}\:\mathrm{skip}\:\mathrm{the}\:\mathrm{question}\:\mathrm{number}\: \\ $$
Commented by mathmax by abdo last updated on 25/Jun/19
![let consider f(x)=∣x∣ ,2π periodic even we have f(x) =(a_0 /2)+Σ_(n=1) ^∞ a_n cos(nx) with a_n =(2/T) ∫_([T]) f(x)cos(nx)dx =(2/(2π)) ∫_(−π) ^π ∣x∣ cos(nx)dx =(2/π) ∫_0 ^π x cos(nx)dx by parts ∫_0 ^π x cos(nx)dx =[(x/n)sin(nx)]_0 ^π −∫_0 ^π (1/n)sin(nx)dx =−(1/n)[−(1/n)cos(nx)]_0 ^π =(1/n^2 ){(−1)^n −1} ⇒a_(2n) =0 and a_(2n+1) =−(2/n^2 )(2/π) =((−4)/(π(2n+1)^2 )) a_0 =(2/π)∫_0 ^π xdx =(2/π)[(π^2 /2)] =π ⇒ ∣x∣ =(π/2) −(4/π)Σ_(n=0) ^∞ ((cos(2n+1)x)/((2n+1)^2 )) x=0 ⇒(π/2) −(4/π) Σ_(n=0) ^∞ (1/((2n+1)^2 )) =0 ⇒(4/π)Σ_(n=0) ^∞ (1/((2n+1)^2 )) =(π/2) ⇒ Σ_(n=0) ^∞ (1/((2n+1)^2 )) =(π^2 /8) we have Σ_(n=1) ^∞ (1/n^2 ) =(1/4)Σ_(n=1) ^∞ (1/n^2 ) +Σ_(n=0) ^∞ (1/((2n+1)^2 )) ⇒ (1−(1/4))Σ_(n=1) ^∞ (1/n^2 ) =(π^2 /8) ⇒(3/4) Σ_(n=1) ^∞ (1/n^2 ) =(π^2 /8) ⇒Σ_(n=1) ^∞ (1/n^2 ) =((4π^2 )/(24)) =(π^2 /6) .](Q62755.png)
$${let}\:{consider}\:\:{f}\left({x}\right)=\mid{x}\mid\:,\mathrm{2}\pi\:{periodic}\:\:{even}\:\:{we}\:{have}\: \\ $$$${f}\left({x}\right)\:=\frac{{a}_{\mathrm{0}} }{\mathrm{2}}+\sum_{{n}=\mathrm{1}} ^{\infty} \:{a}_{{n}} {cos}\left({nx}\right)\:\:{with}\:{a}_{{n}} =\frac{\mathrm{2}}{{T}}\:\int_{\left[{T}\right]} \:\:{f}\left({x}\right){cos}\left({nx}\right){dx} \\ $$$$=\frac{\mathrm{2}}{\mathrm{2}\pi}\:\int_{−\pi} ^{\pi} \:\mid{x}\mid\:{cos}\left({nx}\right){dx}\:=\frac{\mathrm{2}}{\pi}\:\int_{\mathrm{0}} ^{\pi} \:{x}\:{cos}\left({nx}\right){dx}\:{by}\:{parts} \\ $$$$\int_{\mathrm{0}} ^{\pi} \:{x}\:{cos}\left({nx}\right){dx}\:=\left[\frac{{x}}{{n}}{sin}\left({nx}\right)\right]_{\mathrm{0}} ^{\pi} \:−\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{1}}{{n}}{sin}\left({nx}\right){dx} \\ $$$$=−\frac{\mathrm{1}}{{n}}\left[−\frac{\mathrm{1}}{{n}}{cos}\left({nx}\right)\right]_{\mathrm{0}} ^{\pi} \:=\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\left\{\left(−\mathrm{1}\right)^{{n}} −\mathrm{1}\right\}\:\Rightarrow{a}_{\mathrm{2}{n}} =\mathrm{0}\:{and}\:{a}_{\mathrm{2}{n}+\mathrm{1}} =−\frac{\mathrm{2}}{{n}^{\mathrm{2}} }\frac{\mathrm{2}}{\pi}\:=\frac{−\mathrm{4}}{\pi\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${a}_{\mathrm{0}} =\frac{\mathrm{2}}{\pi}\int_{\mathrm{0}} ^{\pi} \:{xdx}\:=\frac{\mathrm{2}}{\pi}\left[\frac{\pi^{\mathrm{2}} }{\mathrm{2}}\right]\:=\pi\:\Rightarrow \\ $$$$\mid{x}\mid\:=\frac{\pi}{\mathrm{2}}\:−\frac{\mathrm{4}}{\pi}\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left(\mathrm{2}{n}+\mathrm{1}\right){x}}{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${x}=\mathrm{0}\:\Rightarrow\frac{\pi}{\mathrm{2}}\:−\frac{\mathrm{4}}{\pi}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }\:=\mathrm{0}\:\Rightarrow\frac{\mathrm{4}}{\pi}\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }\:=\frac{\pi}{\mathrm{2}}\:\Rightarrow \\ $$$$\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }\:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}} \\ $$$${we}\:{have}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{4}}\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\:+\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }\:\Rightarrow \\ $$$$\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}}\right)\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:\Rightarrow\frac{\mathrm{3}}{\mathrm{4}}\:\sum_{{n}=\mathrm{1}} ^{\infty} \frac{\mathrm{1}}{{n}^{\mathrm{2}} }\:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:\Rightarrow\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\:=\frac{\mathrm{4}\pi^{\mathrm{2}} }{\mathrm{24}}\:=\frac{\pi^{\mathrm{2}} }{\mathrm{6}}\:. \\ $$$$ \\ $$$$ \\ $$
Commented by Tawa1 last updated on 25/Jun/19

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
