Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 62828 by mathmax by abdo last updated on 25/Jun/19

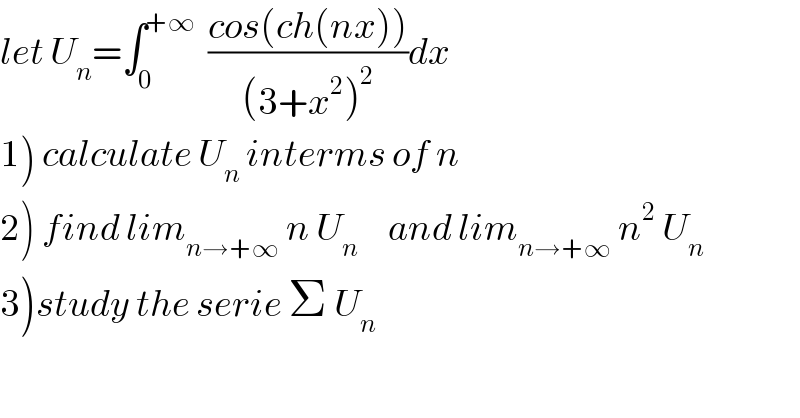
let U_n =∫_0 ^(+∞) ((cos(ch(nx)))/((3+x^2 )^2 ))dx 1) calculate U_n interms of n 2) find lim_(n→+∞) n U_n and lim_(n→+∞) n^2 U_n 3)study the serie Σ U_n

$${let}\:{U}_{{n}} =\int_{\mathrm{0}} ^{+\infty} \:\:\frac{{cos}\left({ch}\left({nx}\right)\right)}{\left(\mathrm{3}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{U}_{{n}} \:{interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{lim}_{{n}\rightarrow+\infty} \:{n}\:{U}_{{n}} \:\:\:\:\:{and}\:{lim}_{{n}\rightarrow+\infty} \:{n}^{\mathrm{2}} \:{U}_{{n}} \\ $$$$\left.\mathrm{3}\right){study}\:{the}\:{serie}\:\Sigma\:{U}_{{n}} \\ $$

Commented by mathmax by abdo last updated on 26/Jun/19

1) we have 2U_n =∫_(−∞) ^(+∞) ((cos(ch(nx)))/((x^2 +3)^2 ))dx =_(x=(√3)t) ∫_(−∞) ^(+∞) ((cos(ch(n(√3)t))/(9(t^2 +1)^2 )) (√3)dt =((√3)/9) Re(∫_(−∞) ^(+∞) (e^(ich(n(√3)t)) /((t^2 +1)^2 ))dt) let consider the complex function ϕ(z) =(e^(ich(n(√3)z)) /((z^2 +1)^2 )) ⇒ϕ(z) =(e^(ich(n(√3)z)) /((z−i)^2 (z+i)^2 )) sl the poles of ϕ are +^− i (doules) residus theorem give ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ Res(ϕ,i) Res(ϕ,i) =lim_(z→i) (1/((2−1)!)){ (z−i)^2 ϕ(z)}^((1)) =lim_(z→i) { (e^(ich(n(√3)z)) /((z+i)^2 ))}^((1)) =lim_(z→i) ((in(√3)sh(n(√3)z)e^(ich(n(√3)z)) (z+i)^2 −2(z+i)e^(ich(n(√3)z)) )/((z+i)^4 )) =lim_(z→i) ((in(√3)sh(n(√3)z)e^(ich(n(√3)z)) (z+i)−2 e^(ich(n(√3)z)) )/((z+i)^3 )) =((−2n(√3)sh(in(√3))e^(ich(in(√3))) −2 e^(ich(in(√3))) )/((2i)^3 )) =−((2n(√3)sh(in(√3))+2)/(−8i)) e^(ich(in(√3))) =(1/(4i))(n(√3)sin(n(√3))+1)cos(n(√3)) ⇒∫_(−∞) ^(+∞) ϕ(z)dz =2iπ (1/(4i))(1+n(√3)sin(n(√3))cos(n(√3)) =(π/2)(1+n(√3)sin(n(√3)))cos(n(√3)) ⇒2U_n =((√3)/(18))(1+n(√3)sin(n(√3))cos(n(√3)) ⇒ U_n =((√3)/(36))(1+n(√3)sin(n(√3)))cos(n(√3)) ⇒ U_n =((√3)/(36)){ cos(n(√3))+((n(√3))/2)sin(2n(√3))} 2) the sequences (cos(n(√3))) and (sin(2n(√3)) diverges so lim_(n→∞) nU_n and lim_(n→+∞) n^2 U_n dont exist. 3) its clear that Σ U_n is dkvergent.

$$\left.\mathrm{1}\right)\:{we}\:{have}\:\mathrm{2}{U}_{{n}} \:=\int_{−\infty} ^{+\infty} \:\:\frac{{cos}\left({ch}\left({nx}\right)\right)}{\left({x}^{\mathrm{2}} +\mathrm{3}\right)^{\mathrm{2}} }{dx}\:=_{{x}=\sqrt{\mathrm{3}}{t}} \:\:\:\:\int_{−\infty} ^{+\infty} \:\:\frac{{cos}\left({ch}\left({n}\sqrt{\mathrm{3}}{t}\right)\right.}{\mathrm{9}\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }\:\sqrt{\mathrm{3}}{dt} \\ $$$$=\frac{\sqrt{\mathrm{3}}}{\mathrm{9}}\:{Re}\left(\int_{−\infty} ^{+\infty} \:\:\frac{{e}^{{ich}\left({n}\sqrt{\mathrm{3}}{t}\right)} }{\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }{dt}\right)\:\:{let}\:{consider}\:{the}\:{complex}\:{function} \\ $$$$\varphi\left({z}\right)\:=\frac{{e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} }{\left({z}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:\Rightarrow\varphi\left({z}\right)\:=\frac{{e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} }{\left({z}−{i}\right)^{\mathrm{2}} \left({z}+{i}\right)^{\mathrm{2}} }\:\:{sl}\:{the}\:{poles}\:{of}\:\varphi\:{are}\:\overset{−} {+}{i}\:\:\left({doules}\right) \\ $$$${residus}\:{theorem}\:{give}\:\int_{−\infty} ^{+\infty} \:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:{Res}\left(\varphi,{i}\right) \\ $$$${Res}\left(\varphi,{i}\right)\:={lim}_{{z}\rightarrow{i}} \:\frac{\mathrm{1}}{\left(\mathrm{2}−\mathrm{1}\right)!}\left\{\:\left({z}−{i}\right)^{\mathrm{2}} \varphi\left({z}\right)\right\}^{\left(\mathrm{1}\right)} \\ $$$$={lim}_{{z}\rightarrow{i}} \:\:\:\left\{\:\frac{{e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} }{\left({z}+{i}\right)^{\mathrm{2}} }\right\}^{\left(\mathrm{1}\right)} \:={lim}_{{z}\rightarrow{i}} \:\:\:\frac{{in}\sqrt{\mathrm{3}}{sh}\left({n}\sqrt{\mathrm{3}}{z}\right){e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} \:\left({z}+{i}\right)^{\mathrm{2}} −\mathrm{2}\left({z}+{i}\right){e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} }{\left({z}+{i}\right)^{\mathrm{4}} } \\ $$$$={lim}_{{z}\rightarrow{i}} \:\:\:\:\:\:\frac{{in}\sqrt{\mathrm{3}}{sh}\left({n}\sqrt{\mathrm{3}}{z}\right){e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} \left({z}+{i}\right)−\mathrm{2}\:{e}^{{ich}\left({n}\sqrt{\mathrm{3}}{z}\right)} }{\left({z}+{i}\right)^{\mathrm{3}} } \\ $$$$=\frac{−\mathrm{2}{n}\sqrt{\mathrm{3}}{sh}\left({in}\sqrt{\mathrm{3}}\right){e}^{{ich}\left({in}\sqrt{\mathrm{3}}\right)} −\mathrm{2}\:{e}^{{ich}\left({in}\sqrt{\mathrm{3}}\right)} }{\left(\mathrm{2}{i}\right)^{\mathrm{3}} }\:=−\frac{\mathrm{2}{n}\sqrt{\mathrm{3}}{sh}\left({in}\sqrt{\mathrm{3}}\right)+\mathrm{2}}{−\mathrm{8}{i}}\:{e}^{{ich}\left({in}\sqrt{\mathrm{3}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}{i}}\left({n}\sqrt{\mathrm{3}}{sin}\left({n}\sqrt{\mathrm{3}}\right)+\mathrm{1}\right){cos}\left({n}\sqrt{\mathrm{3}}\right)\:\Rightarrow\int_{−\infty} ^{+\infty} \:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\frac{\mathrm{1}}{\mathrm{4}{i}}\left(\mathrm{1}+{n}\sqrt{\mathrm{3}}{sin}\left({n}\sqrt{\mathrm{3}}\right){cos}\left({n}\sqrt{\mathrm{3}}\right)\right. \\ $$$$=\frac{\pi}{\mathrm{2}}\left(\mathrm{1}+{n}\sqrt{\mathrm{3}}{sin}\left({n}\sqrt{\mathrm{3}}\right)\right){cos}\left({n}\sqrt{\mathrm{3}}\right)\:\Rightarrow\mathrm{2}{U}_{{n}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{18}}\left(\mathrm{1}+{n}\sqrt{\mathrm{3}}{sin}\left({n}\sqrt{\mathrm{3}}\right){cos}\left({n}\sqrt{\mathrm{3}}\right)\:\Rightarrow\right. \\ $$$${U}_{{n}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{36}}\left(\mathrm{1}+{n}\sqrt{\mathrm{3}}{sin}\left({n}\sqrt{\mathrm{3}}\right)\right){cos}\left({n}\sqrt{\mathrm{3}}\right)\:\Rightarrow \\ $$$${U}_{{n}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{36}}\left\{\:{cos}\left({n}\sqrt{\mathrm{3}}\right)+\frac{{n}\sqrt{\mathrm{3}}}{\mathrm{2}}{sin}\left(\mathrm{2}{n}\sqrt{\mathrm{3}}\right)\right\} \\ $$$$\left.\mathrm{2}\right)\:{the}\:{sequences}\:\left({cos}\left({n}\sqrt{\mathrm{3}}\right)\right)\:{and}\:\left({sin}\left(\mathrm{2}{n}\sqrt{\mathrm{3}}\right)\:{diverges}\:{so}\:{lim}_{{n}\rightarrow\infty} {nU}_{{n}} \:{and}\right. \\ $$$${lim}_{{n}\rightarrow+\infty} {n}^{\mathrm{2}} \:{U}_{{n}} \:{dont}\:{exist}. \\ $$$$\left.\mathrm{3}\right)\:{its}\:{clear}\:{that}\:\Sigma\:{U}_{{n}} {is}\:{dkvergent}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com