Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 62856 by mathmax by abdo last updated on 26/Jun/19

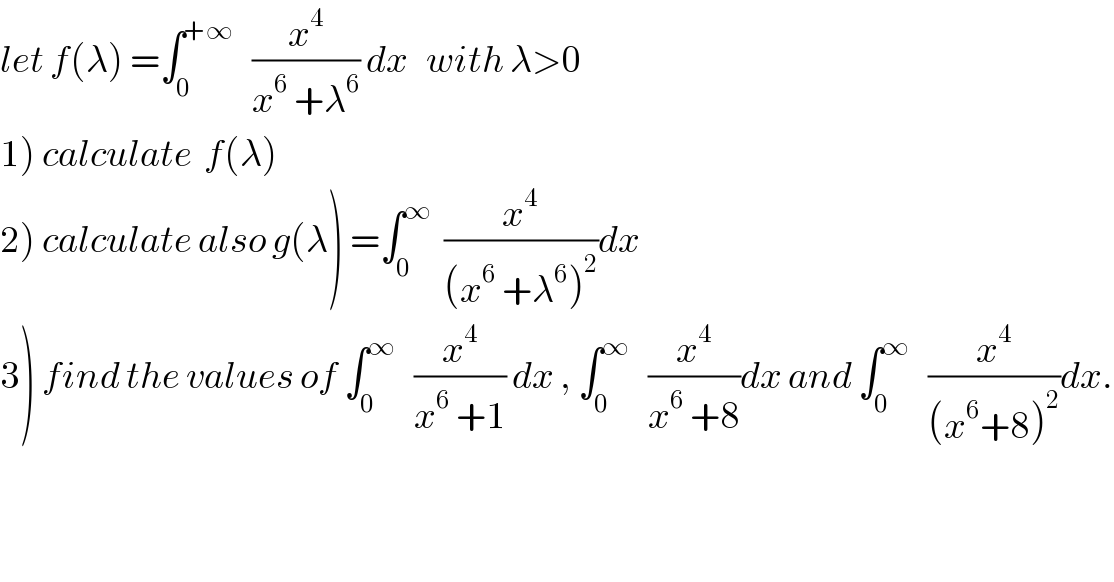
let f(λ) =∫_0 ^(+∞) (x^4 /(x^6 +λ^6 )) dx with λ>0 1) calculate f(λ) 2) calculate also g(λ) =∫_0 ^∞ (x^4 /((x^6 +λ^6 )^2 ))dx 3) find the values of ∫_0 ^∞ (x^4 /(x^6 +1)) dx , ∫_0 ^∞ (x^4 /(x^6 +8))dx and ∫_0 ^∞ (x^4 /((x^6 +8)^2 ))dx.

$${let}\:{f}\left(\lambda\right)\:=\int_{\mathrm{0}} ^{+\infty} \:\:\:\frac{{x}^{\mathrm{4}} }{{x}^{\mathrm{6}} \:+\lambda^{\mathrm{6}} }\:{dx}\:\:\:{with}\:\lambda>\mathrm{0} \\ $$ $$\left.\mathrm{1}\right)\:{calculate}\:\:{f}\left(\lambda\right) \\ $$ $$\left.\mathrm{2}\right)\:{calculate}\:{also}\:{g}\left(\lambda\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{x}^{\mathrm{4}} }{\left({x}^{\mathrm{6}} \:+\lambda^{\mathrm{6}} \right)^{\mathrm{2}} }{dx} \\ $$ $$\left.\mathrm{3}\right)\:{find}\:{the}\:{values}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{x}^{\mathrm{4}} }{{x}^{\mathrm{6}} \:+\mathrm{1}}\:{dx}\:,\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{x}^{\mathrm{4}} }{{x}^{\mathrm{6}} \:+\mathrm{8}}{dx}\:{and}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{x}^{\mathrm{4}} }{\left({x}^{\mathrm{6}} +\mathrm{8}\right)^{\mathrm{2}} }{dx}. \\ $$

Commented bymathmax by abdo last updated on 26/Jun/19

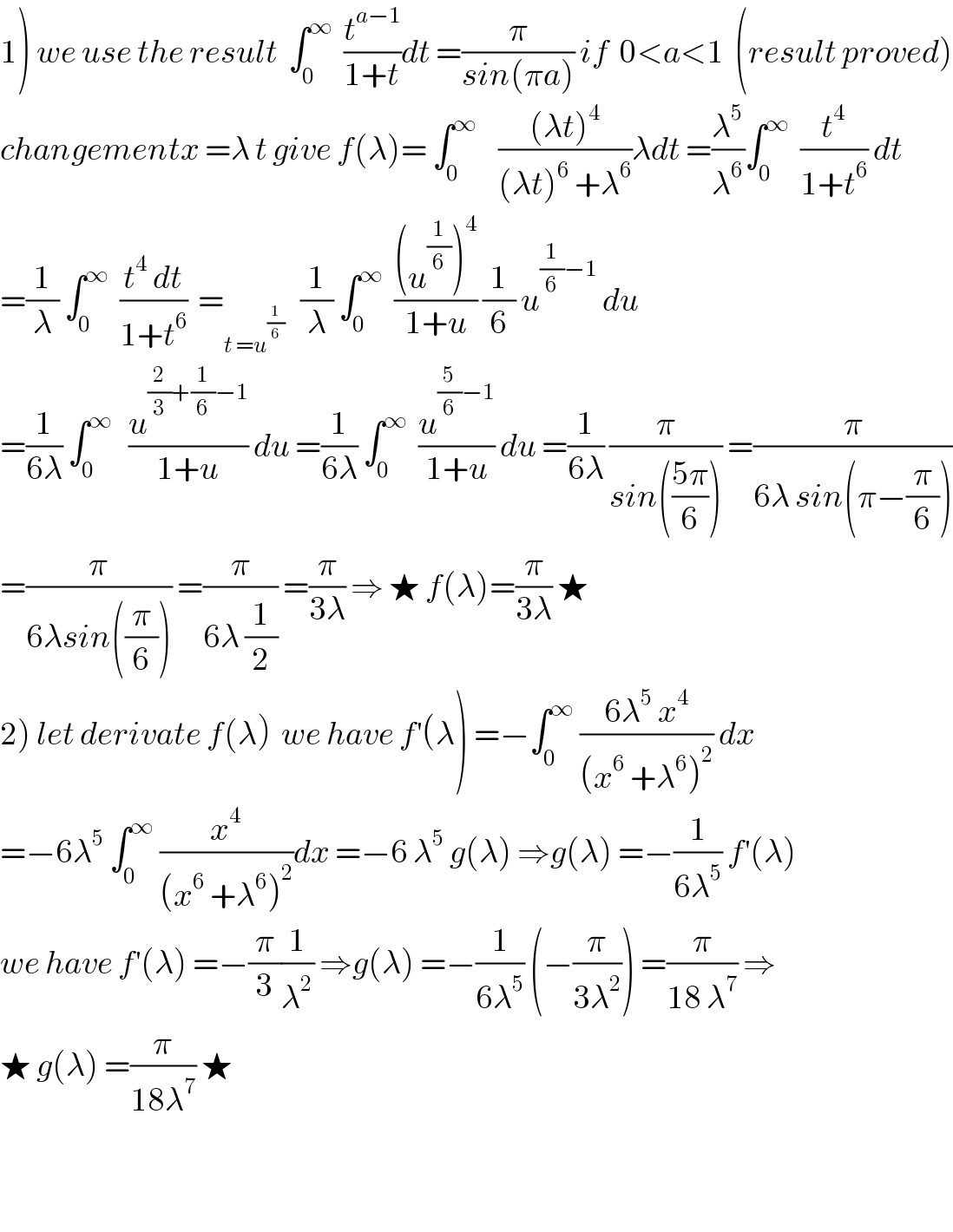
1) we use the result ∫_0 ^∞ (t^(a−1) /(1+t))dt =(π/(sin(πa))) if 0<a<1 (result proved) changementx =λ t give f(λ)= ∫_0 ^∞ (((λt)^4 )/((λt)^6 +λ^6 ))λdt =(λ^5 /λ^6 )∫_0 ^∞ (t^4 /(1+t^6 )) dt =(1/λ) ∫_0 ^∞ ((t^4 dt)/(1+t^6 )) =_(t =u^(1/6) ) (1/λ) ∫_0 ^∞ (((u^(1/6) )^4 )/(1+u)) (1/6) u^((1/6)−1) du =(1/(6λ)) ∫_0 ^∞ (u^((2/3)+(1/6)−1) /(1+u)) du =(1/(6λ)) ∫_0 ^∞ (u^((5/6)−1) /(1+u)) du =(1/(6λ)) (π/(sin(((5π)/6)))) =(π/(6λ sin(π−(π/6)))) =(π/(6λsin((π/6)))) =(π/(6λ (1/2))) =(π/(3λ)) ⇒ ★ f(λ)=(π/(3λ)) ★ 2) let derivate f(λ) we have f^′ (λ) =−∫_0 ^∞ ((6λ^5 x^4 )/((x^6 +λ^6 )^2 )) dx =−6λ^5 ∫_0 ^∞ (x^4 /((x^6 +λ^6 )^2 ))dx =−6 λ^5 g(λ) ⇒g(λ) =−(1/(6λ^5 )) f^′ (λ) we have f^′ (λ) =−(π/3)(1/λ^2 ) ⇒g(λ) =−(1/(6λ^5 )) (−(π/(3λ^2 ))) =(π/(18 λ^7 )) ⇒ ★ g(λ) =(π/(18λ^7 )) ★

$$\left.\mathrm{1}\right)\:{we}\:{use}\:{the}\:{result}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}^{{a}−\mathrm{1}} }{\mathrm{1}+{t}}{dt}\:=\frac{\pi}{{sin}\left(\pi{a}\right)}\:{if}\:\:\mathrm{0}<{a}<\mathrm{1}\:\:\left({result}\:{proved}\right) \\ $$ $${changementx}\:=\lambda\:{t}\:{give}\:{f}\left(\lambda\right)=\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{\left(\lambda{t}\right)^{\mathrm{4}} }{\left(\lambda{t}\right)^{\mathrm{6}} \:+\lambda^{\mathrm{6}} }\lambda{dt}\:=\frac{\lambda^{\mathrm{5}} }{\lambda^{\mathrm{6}} }\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}^{\mathrm{4}} }{\mathrm{1}+{t}^{\mathrm{6}} }\:{dt} \\ $$ $$=\frac{\mathrm{1}}{\lambda}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}^{\mathrm{4}} \:{dt}}{\mathrm{1}+{t}^{\mathrm{6}} }\:\:=_{{t}\:={u}^{\frac{\mathrm{1}}{\mathrm{6}}} } \:\:\:\frac{\mathrm{1}}{\lambda}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\left({u}^{\frac{\mathrm{1}}{\mathrm{6}}} \right)^{\mathrm{4}} }{\mathrm{1}+{u}}\:\frac{\mathrm{1}}{\mathrm{6}}\:{u}^{\frac{\mathrm{1}}{\mathrm{6}}−\mathrm{1}} \:{du} \\ $$ $$=\frac{\mathrm{1}}{\mathrm{6}\lambda}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{u}^{\frac{\mathrm{2}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{6}}−\mathrm{1}} }{\mathrm{1}+{u}}\:{du}\:=\frac{\mathrm{1}}{\mathrm{6}\lambda}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{u}^{\frac{\mathrm{5}}{\mathrm{6}}−\mathrm{1}} }{\mathrm{1}+{u}}\:{du}\:=\frac{\mathrm{1}}{\mathrm{6}\lambda}\:\frac{\pi}{{sin}\left(\frac{\mathrm{5}\pi}{\mathrm{6}}\right)}\:=\frac{\pi}{\mathrm{6}\lambda\:{sin}\left(\pi−\frac{\pi}{\mathrm{6}}\right)} \\ $$ $$=\frac{\pi}{\mathrm{6}\lambda{sin}\left(\frac{\pi}{\mathrm{6}}\right)}\:=\frac{\pi}{\mathrm{6}\lambda\:\frac{\mathrm{1}}{\mathrm{2}}}\:=\frac{\pi}{\mathrm{3}\lambda}\:\Rightarrow\:\bigstar\:{f}\left(\lambda\right)=\frac{\pi}{\mathrm{3}\lambda}\:\bigstar \\ $$ $$\left.\mathrm{2}\right)\:{let}\:{derivate}\:{f}\left(\lambda\right)\:\:{we}\:{have}\:{f}^{'} \left(\lambda\right)\:=−\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{6}\lambda^{\mathrm{5}} \:{x}^{\mathrm{4}} }{\left({x}^{\mathrm{6}} \:+\lambda^{\mathrm{6}} \right)^{\mathrm{2}} }\:{dx} \\ $$ $$=−\mathrm{6}\lambda^{\mathrm{5}} \:\int_{\mathrm{0}} ^{\infty} \:\frac{{x}^{\mathrm{4}} }{\left({x}^{\mathrm{6}} \:+\lambda^{\mathrm{6}} \right)^{\mathrm{2}} }{dx}\:=−\mathrm{6}\:\lambda^{\mathrm{5}} \:{g}\left(\lambda\right)\:\Rightarrow{g}\left(\lambda\right)\:=−\frac{\mathrm{1}}{\mathrm{6}\lambda^{\mathrm{5}} }\:{f}^{'} \left(\lambda\right) \\ $$ $${we}\:{have}\:{f}^{'} \left(\lambda\right)\:=−\frac{\pi}{\mathrm{3}}\frac{\mathrm{1}}{\lambda^{\mathrm{2}} }\:\Rightarrow{g}\left(\lambda\right)\:=−\frac{\mathrm{1}}{\mathrm{6}\lambda^{\mathrm{5}} }\:\left(−\frac{\pi}{\mathrm{3}\lambda^{\mathrm{2}} }\right)\:=\frac{\pi}{\mathrm{18}\:\lambda^{\mathrm{7}} }\:\Rightarrow \\ $$ $$\bigstar\:{g}\left(\lambda\right)\:=\frac{\pi}{\mathrm{18}\lambda^{\mathrm{7}} }\:\bigstar\: \\ $$ $$ \\ $$ $$ \\ $$

Commented bymathmax by abdo last updated on 26/Jun/19

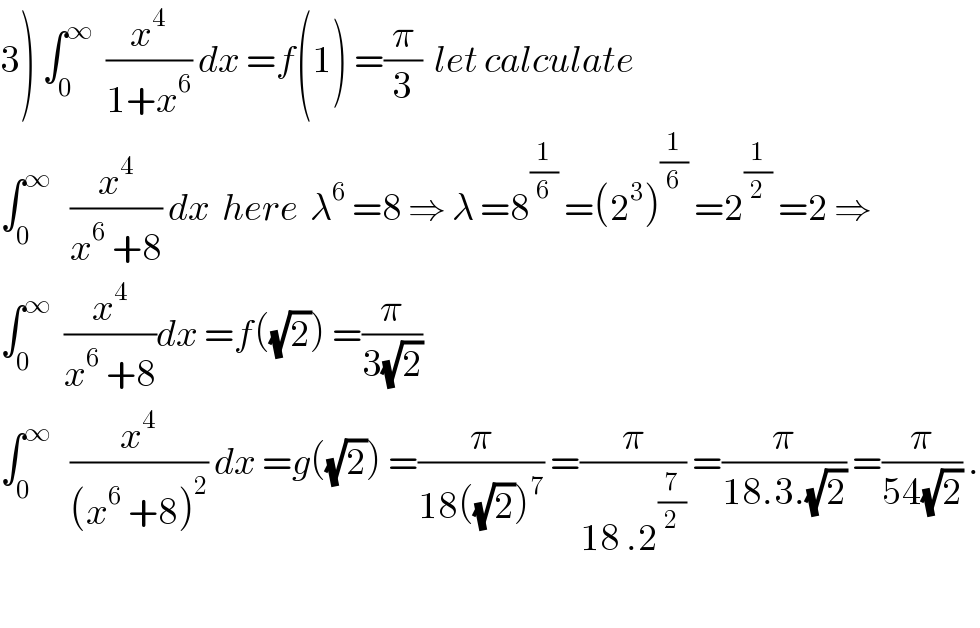
3) ∫_0 ^∞ (x^4 /(1+x^6 )) dx =f(1) =(π/3) let calculate ∫_0 ^∞ (x^4 /(x^6 +8)) dx here λ^6 =8 ⇒ λ =8^(1/6) =(2^3 )^(1/6) =2^(1/2) =2 ⇒ ∫_0 ^∞ (x^4 /(x^6 +8))dx =f((√2)) =(π/(3(√2))) ∫_0 ^∞ (x^4 /((x^6 +8)^2 )) dx =g((√2)) =(π/(18((√2))^7 )) =(π/(18 .2^(7/2) )) =(π/(18.3.(√2))) =(π/(54(√2))) .

$$\left.\mathrm{3}\right)\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{x}^{\mathrm{4}} }{\mathrm{1}+{x}^{\mathrm{6}} }\:{dx}\:={f}\left(\mathrm{1}\right)\:=\frac{\pi}{\mathrm{3}}\:\:{let}\:{calculate}\: \\ $$ $$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{x}^{\mathrm{4}} }{{x}^{\mathrm{6}} \:+\mathrm{8}}\:{dx}\:\:{here}\:\:\lambda^{\mathrm{6}} \:=\mathrm{8}\:\Rightarrow\:\lambda\:=\mathrm{8}^{\frac{\mathrm{1}}{\mathrm{6}}} \:=\left(\mathrm{2}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{6}}} \:=\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}}} \:=\mathrm{2}\:\Rightarrow \\ $$ $$\int_{\mathrm{0}} ^{\infty} \:\:\frac{{x}^{\mathrm{4}} }{{x}^{\mathrm{6}} \:+\mathrm{8}}{dx}\:={f}\left(\sqrt{\mathrm{2}}\right)\:=\frac{\pi}{\mathrm{3}\sqrt{\mathrm{2}}} \\ $$ $$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{x}^{\mathrm{4}} }{\left({x}^{\mathrm{6}} \:+\mathrm{8}\right)^{\mathrm{2}} }\:{dx}\:={g}\left(\sqrt{\mathrm{2}}\right)\:=\frac{\pi}{\mathrm{18}\left(\sqrt{\mathrm{2}}\right)^{\mathrm{7}} }\:=\frac{\pi}{\mathrm{18}\:.\mathrm{2}^{\frac{\mathrm{7}}{\mathrm{2}}} }\:=\frac{\pi}{\mathrm{18}.\mathrm{3}.\sqrt{\mathrm{2}}}\:=\frac{\pi}{\mathrm{54}\sqrt{\mathrm{2}}}\:. \\ $$ $$ \\ $$

Commented bymathmax by abdo last updated on 26/Jun/19

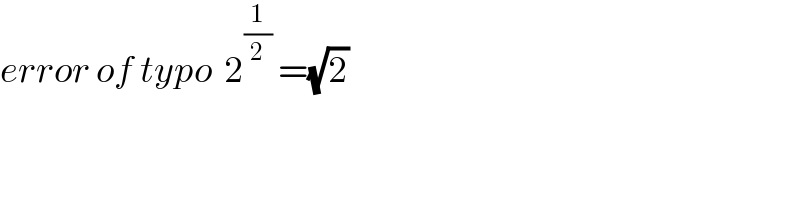
error of typo 2^(1/2) =(√2)

$${error}\:{of}\:{typo}\:\:\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}}} \:=\sqrt{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com