
Question and Answers Forum
Question Number 63481 by naka3546 last updated on 04/Jul/19
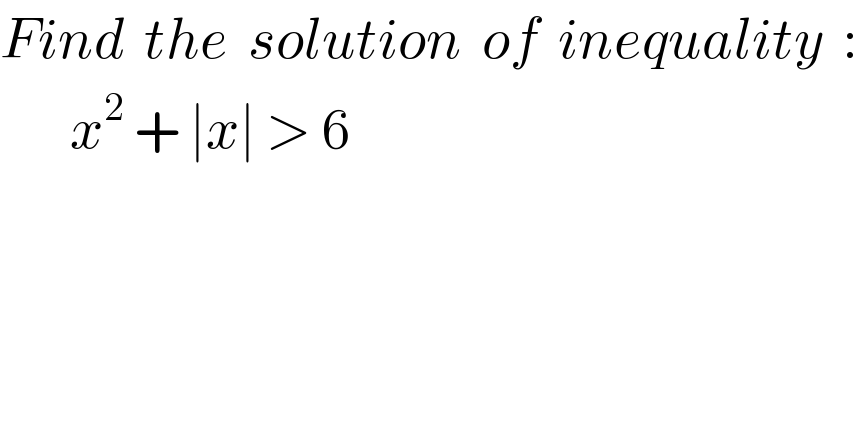
Commented bymathmax by abdo last updated on 04/Jul/19
![(ine) ⇒∣x∣^2 +∣x∣−6>0 ⇒t^2 +t−6>0 Δ=1−4(−6)=25 ⇒ t_1 =((−1+5)/2) =2 and t_2 =((−1−5)/2) =−3 ⇒ ∣x∣^2 +∣x∣−6 =(∣x∣−2)(∣x∣+3) so (ine) ⇒{∣x∣−2}{∣x∣+3}>0 ⇒ ∣x∣−2 >0 (because ∣x∣ +3>0 ⇒ x>2 or x<−2 ⇒ x ∈]−∞,−2[∪]2,+∞[](Q63496.png)
Answered by MJS last updated on 04/Jul/19
![x>0 x^2 +x>6 x^2 +x−6=0 ⇒ x=−3∨x=2 ⇒ x<−3∨x>2 but x>0 ⇒ x>2 x<0 x^2 −x>6 x^2 −x−6=0 ⇒ x=−2∨x=3 ⇒ x<−2∨x>3 but x<0 ⇒ x<−2 x^2 +∣x∣>6 ⇒ x<−2∨2<x ⇔ x∈]−∞; −2[∪]2; +∞[](Q63501.png)
| ||
Question and Answers Forum | ||
Question Number 63481 by naka3546 last updated on 04/Jul/19 | ||
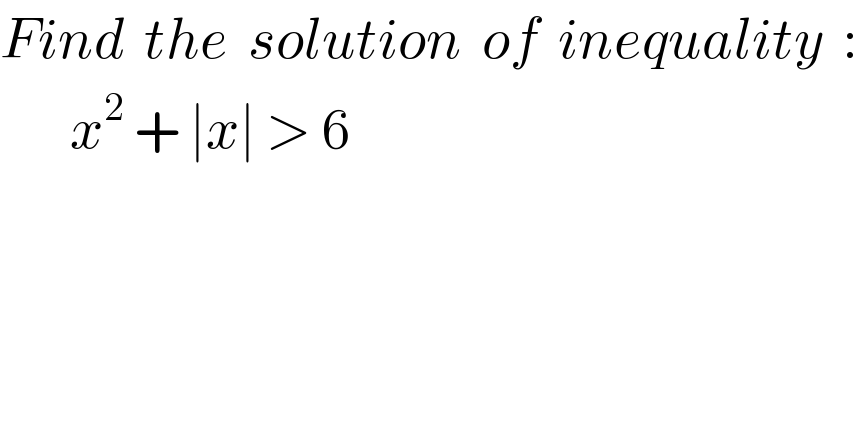 | ||
Commented bymathmax by abdo last updated on 04/Jul/19 | ||
![(ine) ⇒∣x∣^2 +∣x∣−6>0 ⇒t^2 +t−6>0 Δ=1−4(−6)=25 ⇒ t_1 =((−1+5)/2) =2 and t_2 =((−1−5)/2) =−3 ⇒ ∣x∣^2 +∣x∣−6 =(∣x∣−2)(∣x∣+3) so (ine) ⇒{∣x∣−2}{∣x∣+3}>0 ⇒ ∣x∣−2 >0 (because ∣x∣ +3>0 ⇒ x>2 or x<−2 ⇒ x ∈]−∞,−2[∪]2,+∞[](Q63496.png) | ||
Answered by MJS last updated on 04/Jul/19 | ||
![x>0 x^2 +x>6 x^2 +x−6=0 ⇒ x=−3∨x=2 ⇒ x<−3∨x>2 but x>0 ⇒ x>2 x<0 x^2 −x>6 x^2 −x−6=0 ⇒ x=−2∨x=3 ⇒ x<−2∨x>3 but x<0 ⇒ x<−2 x^2 +∣x∣>6 ⇒ x<−2∨2<x ⇔ x∈]−∞; −2[∪]2; +∞[](Q63501.png) | ||
| ||