Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 63508 by mathmax by abdo last updated on 05/Jul/19

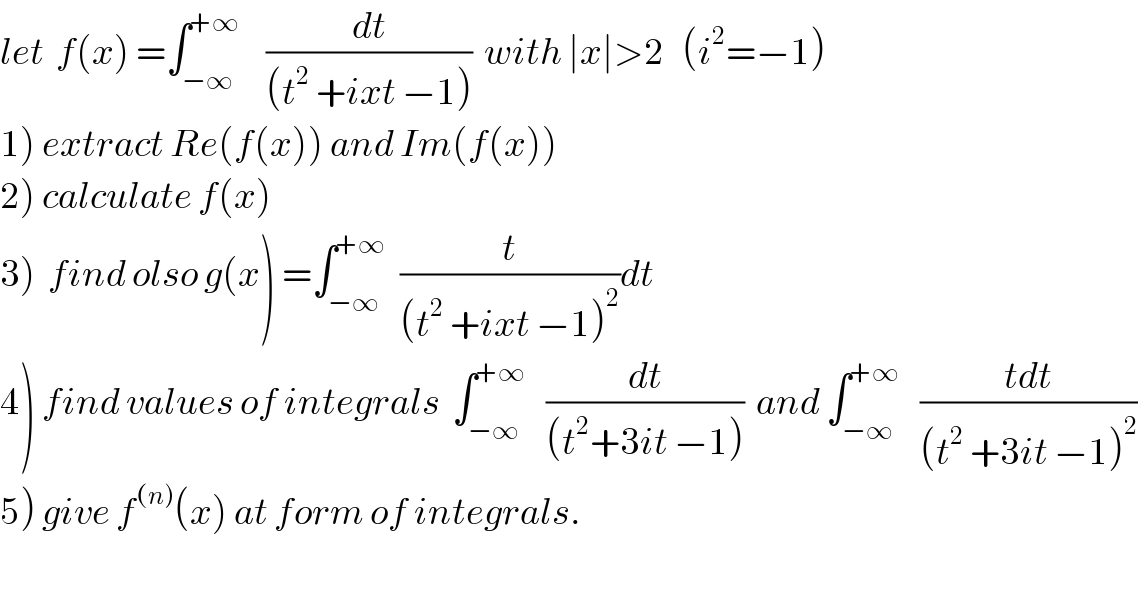
let f(x) =∫_(−∞) ^(+∞) (dt/((t^2 +ixt −1))) with ∣x∣>2 (i^2 =−1) 1) extract Re(f(x)) and Im(f(x)) 2) calculate f(x) 3) find olso g(x) =∫_(−∞) ^(+∞) (t/((t^2 +ixt −1)^2 ))dt 4) find values of integrals ∫_(−∞) ^(+∞) (dt/((t^2 +3it −1))) and ∫_(−∞) ^(+∞) ((tdt)/((t^2 +3it −1)^2 )) 5) give f^((n)) (x) at form of integrals.

$${let}\:\:{f}\left({x}\right)\:=\int_{−\infty} ^{+\infty} \:\:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+{ixt}\:−\mathrm{1}\right)}\:\:{with}\:\mid{x}\mid>\mathrm{2}\:\:\:\left({i}^{\mathrm{2}} =−\mathrm{1}\right) \\ $$ $$\left.\mathrm{1}\right)\:{extract}\:{Re}\left({f}\left({x}\right)\right)\:{and}\:{Im}\left({f}\left({x}\right)\right) \\ $$ $$\left.\mathrm{2}\right)\:{calculate}\:{f}\left({x}\right) \\ $$ $$\left.\mathrm{3}\right)\:\:{find}\:{olso}\:{g}\left({x}\right)\:=\int_{−\infty} ^{+\infty} \:\:\frac{{t}}{\left({t}^{\mathrm{2}} \:+{ixt}\:−\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$ $$\left.\mathrm{4}\right)\:{find}\:{values}\:{of}\:{integrals}\:\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} +\mathrm{3}{it}\:−\mathrm{1}\right)}\:\:{and}\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{tdt}}{\left({t}^{\mathrm{2}} \:+\mathrm{3}{it}\:−\mathrm{1}\right)^{\mathrm{2}} } \\ $$ $$\left.\mathrm{5}\right)\:{give}\:{f}^{\left({n}\right)} \left({x}\right)\:{at}\:{form}\:{of}\:{integrals}. \\ $$

Commented bymathmax by abdo last updated on 06/Jul/19

1) we have f(x) =∫_(−∞) ^(+∞) (dt/(t^2 −1 +ixt)) =∫_(−∞) ^(+∞) ((t^2 −1−ixt)/((t^2 −1)^2 +x^2 t^2 ))dt =∫_(−∞) ^(+∞) ((t^2 −1)/(t^4 −2t^2 +1 +x^2 t^2 )) dt −i ∫_(−∞) ^(+∞) ((xt)/(t^4 −2t^2 +1 +x^2 t^2 )) dt =∫_(−∞) ^(+∞) ((t^2 −1)/(t^4 +(x^2 −2)t^2 +1)) −i×0 (the function t→(t/(t^4 +(x^2 −2)t^2 +1)) is odd)⇒ Re(f(x)) =∫_(−∞) ^(+∞) ((t^2 −1)/(t^4 +(x^2 −2)t^2 +1))dt and Im(f(x))=0

$$\left.\mathrm{1}\right)\:{we}\:{have}\:{f}\left({x}\right)\:=\int_{−\infty} ^{+\infty} \:\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\mathrm{1}\:+{ixt}}\:=\int_{−\infty} ^{+\infty} \:\:\frac{{t}^{\mathrm{2}} −\mathrm{1}−{ixt}}{\left({t}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +{x}^{\mathrm{2}} {t}^{\mathrm{2}} }{dt} \\ $$ $$=\int_{−\infty} ^{+\infty} \:\:\:\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}^{\mathrm{4}} −\mathrm{2}{t}^{\mathrm{2}} \:+\mathrm{1}\:+{x}^{\mathrm{2}} {t}^{\mathrm{2}} }\:{dt}\:−{i}\:\int_{−\infty} ^{+\infty} \:\frac{{xt}}{{t}^{\mathrm{4}} −\mathrm{2}{t}^{\mathrm{2}} \:+\mathrm{1}\:+{x}^{\mathrm{2}} {t}^{\mathrm{2}} }\:{dt} \\ $$ $$=\int_{−\infty} ^{+\infty} \:\:\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}^{\mathrm{4}} +\left({x}^{\mathrm{2}} −\mathrm{2}\right){t}^{\mathrm{2}} \:+\mathrm{1}}\:−{i}×\mathrm{0}\:\:\:\:\left({the}\:{function}\:{t}\rightarrow\frac{{t}}{{t}^{\mathrm{4}} \:+\left({x}^{\mathrm{2}} −\mathrm{2}\right){t}^{\mathrm{2}} \:+\mathrm{1}}\:{is}\:{odd}\right)\Rightarrow \\ $$ $${Re}\left({f}\left({x}\right)\right)\:=\int_{−\infty} ^{+\infty} \:\:\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}^{\mathrm{4}} \:+\left({x}^{\mathrm{2}} −\mathrm{2}\right){t}^{\mathrm{2}} +\mathrm{1}}{dt}\:{and}\:{Im}\left({f}\left({x}\right)\right)=\mathrm{0} \\ $$

Commented bymathmax by abdo last updated on 06/Jul/19

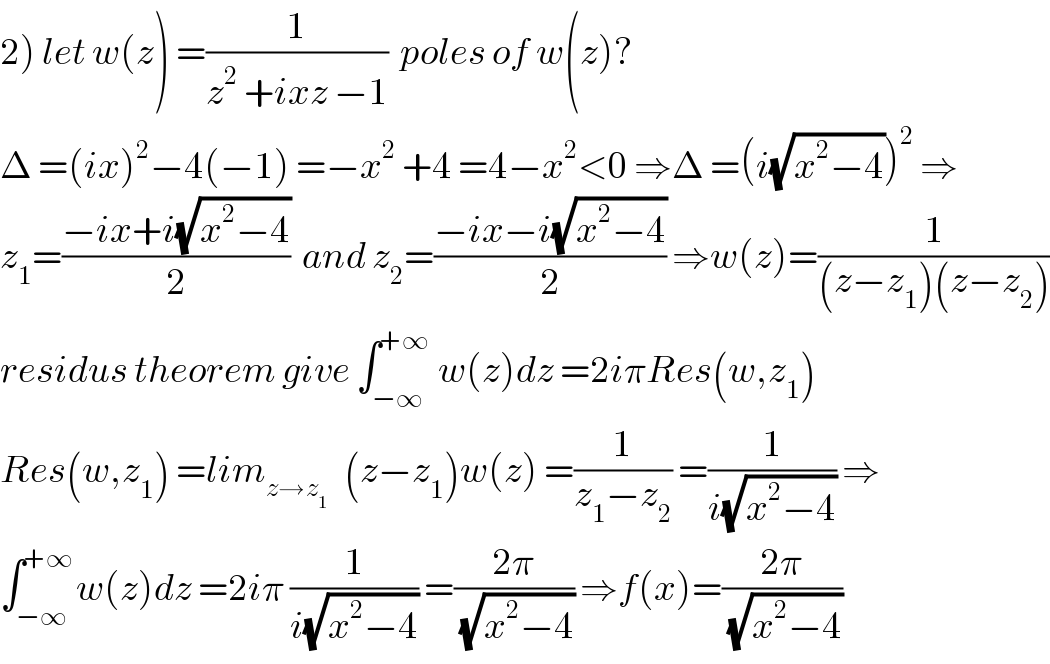
2) let w(z) =(1/(z^2 +ixz −1)) poles of w(z)? Δ =(ix)^2 −4(−1) =−x^2 +4 =4−x^2 <0 ⇒Δ =(i(√(x^2 −4)))^2 ⇒ z_1 =((−ix+i(√(x^2 −4)))/2) and z_2 =((−ix−i(√(x^2 −4)))/2) ⇒w(z)=(1/((z−z_1 )(z−z_2 ))) residus theorem give ∫_(−∞) ^(+∞) w(z)dz =2iπRes(w,z_1 ) Res(w,z_1 ) =lim_(z→z_1 ) (z−z_1 )w(z) =(1/(z_1 −z_2 )) =(1/(i(√(x^2 −4)))) ⇒ ∫_(−∞) ^(+∞) w(z)dz =2iπ (1/(i(√(x^2 −4)))) =((2π)/(√(x^2 −4))) ⇒f(x)=((2π)/(√(x^2 −4)))

$$\left.\mathrm{2}\right)\:{let}\:{w}\left({z}\right)\:=\frac{\mathrm{1}}{{z}^{\mathrm{2}} \:+{ixz}\:−\mathrm{1}}\:\:{poles}\:{of}\:{w}\left({z}\right)? \\ $$ $$\Delta\:=\left({ix}\right)^{\mathrm{2}} −\mathrm{4}\left(−\mathrm{1}\right)\:=−{x}^{\mathrm{2}} \:+\mathrm{4}\:=\mathrm{4}−{x}^{\mathrm{2}} <\mathrm{0}\:\Rightarrow\Delta\:=\left({i}\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}\right)^{\mathrm{2}} \:\Rightarrow \\ $$ $${z}_{\mathrm{1}} =\frac{−{ix}+{i}\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}}\:\:{and}\:{z}_{\mathrm{2}} =\frac{−{ix}−{i}\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}}\:\Rightarrow{w}\left({z}\right)=\frac{\mathrm{1}}{\left({z}−{z}_{\mathrm{1}} \right)\left({z}−{z}_{\mathrm{2}} \right)} \\ $$ $${residus}\:{theorem}\:{give}\:\int_{−\infty} ^{+\infty} \:{w}\left({z}\right){dz}\:=\mathrm{2}{i}\pi{Res}\left({w},{z}_{\mathrm{1}} \right) \\ $$ $${Res}\left({w},{z}_{\mathrm{1}} \right)\:={lim}_{{z}\rightarrow{z}_{\mathrm{1}} \:} \:\:\left({z}−{z}_{\mathrm{1}} \right){w}\left({z}\right)\:=\frac{\mathrm{1}}{{z}_{\mathrm{1}} −{z}_{\mathrm{2}} }\:=\frac{\mathrm{1}}{{i}\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:\Rightarrow \\ $$ $$\int_{−\infty} ^{+\infty} {w}\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\frac{\mathrm{1}}{{i}\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:=\frac{\mathrm{2}\pi}{\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:\Rightarrow{f}\left({x}\right)=\frac{\mathrm{2}\pi}{\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}} \\ $$

Commented bymathmax by abdo last updated on 06/Jul/19

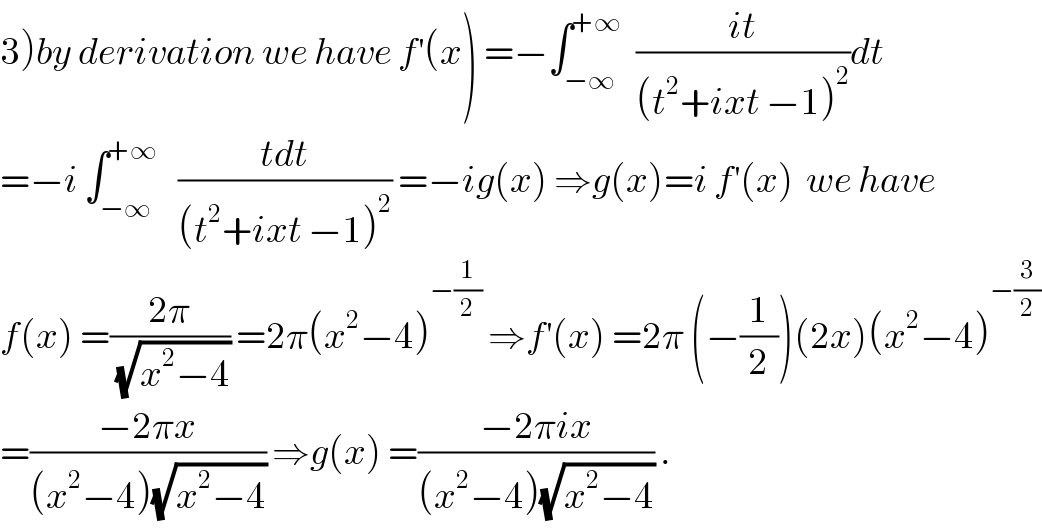
3)by derivation we have f^′ (x) =−∫_(−∞) ^(+∞) ((it)/((t^2 +ixt −1)^2 ))dt =−i ∫_(−∞) ^(+∞) ((tdt)/((t^2 +ixt −1)^2 )) =−ig(x) ⇒g(x)=i f^′ (x) we have f(x) =((2π)/(√(x^2 −4))) =2π(x^2 −4)^(−(1/2)) ⇒f^′ (x) =2π (−(1/2))(2x)(x^2 −4)^(−(3/2)) =((−2πx)/((x^2 −4)(√(x^2 −4)))) ⇒g(x) =((−2πix)/((x^2 −4)(√(x^2 −4)))) .

$$\left.\mathrm{3}\right){by}\:{derivation}\:{we}\:{have}\:{f}^{'} \left({x}\right)\:=−\int_{−\infty} ^{+\infty} \:\:\frac{{it}}{\left({t}^{\mathrm{2}} +{ixt}\:−\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$ $$=−{i}\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{tdt}}{\left({t}^{\mathrm{2}} +{ixt}\:−\mathrm{1}\right)^{\mathrm{2}} }\:=−{ig}\left({x}\right)\:\Rightarrow{g}\left({x}\right)={i}\:{f}^{'} \left({x}\right)\:\:{we}\:{have}\: \\ $$ $${f}\left({x}\right)\:=\frac{\mathrm{2}\pi}{\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:=\mathrm{2}\pi\left({x}^{\mathrm{2}} −\mathrm{4}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\Rightarrow{f}^{'} \left({x}\right)\:=\mathrm{2}\pi\:\left(−\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\mathrm{2}{x}\right)\left({x}^{\mathrm{2}} −\mathrm{4}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} \\ $$ $$=\frac{−\mathrm{2}\pi{x}}{\left({x}^{\mathrm{2}} −\mathrm{4}\right)\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:\Rightarrow{g}\left({x}\right)\:=\frac{−\mathrm{2}\pi{ix}}{\left({x}^{\mathrm{2}} −\mathrm{4}\right)\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:. \\ $$

Commented bymathmax by abdo last updated on 06/Jul/19

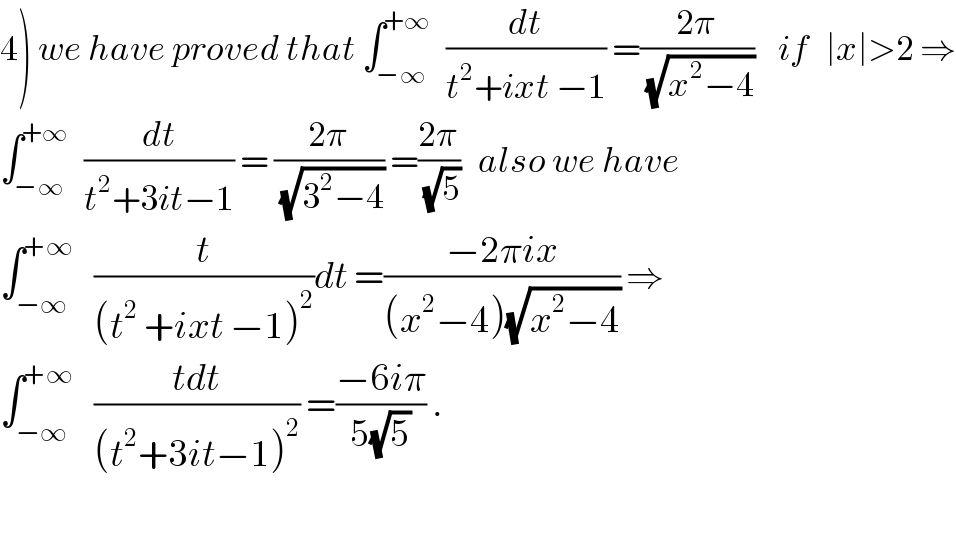
4) we have proved that ∫_(−∞) ^(+∞) (dt/(t^2 +ixt −1)) =((2π)/(√(x^2 −4))) if ∣x∣>2 ⇒ ∫_(−∞) ^(+∞) (dt/(t^2 +3it−1)) = ((2π)/(√(3^2 −4))) =((2π)/(√5)) also we have ∫_(−∞) ^(+∞) (t/((t^2 +ixt −1)^2 ))dt =((−2πix)/((x^2 −4)(√(x^2 −4)))) ⇒ ∫_(−∞) ^(+∞) ((tdt)/((t^2 +3it−1)^2 )) =((−6iπ)/(5(√5))) .

$$\left.\mathrm{4}\right)\:{we}\:{have}\:{proved}\:{that}\:\int_{−\infty} ^{+\infty} \:\:\frac{{dt}}{{t}^{\mathrm{2}} +{ixt}\:−\mathrm{1}}\:=\frac{\mathrm{2}\pi}{\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:\:\:\:{if}\:\:\:\mid{x}\mid>\mathrm{2}\:\Rightarrow \\ $$ $$\int_{−\infty} ^{+\infty} \:\:\frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{3}{it}−\mathrm{1}}\:=\:\frac{\mathrm{2}\pi}{\sqrt{\mathrm{3}^{\mathrm{2}} −\mathrm{4}}}\:=\frac{\mathrm{2}\pi}{\sqrt{\mathrm{5}}}\:\:\:{also}\:{we}\:{have} \\ $$ $$\int_{−\infty} ^{+\infty} \:\:\:\frac{{t}}{\left({t}^{\mathrm{2}} \:+{ixt}\:−\mathrm{1}\right)^{\mathrm{2}} }{dt}\:=\frac{−\mathrm{2}\pi{ix}}{\left({x}^{\mathrm{2}} −\mathrm{4}\right)\sqrt{{x}^{\mathrm{2}} −\mathrm{4}}}\:\Rightarrow \\ $$ $$\int_{−\infty} ^{+\infty} \:\:\:\frac{{tdt}}{\left({t}^{\mathrm{2}} +\mathrm{3}{it}−\mathrm{1}\right)^{\mathrm{2}} }\:=\frac{−\mathrm{6}{i}\pi}{\mathrm{5}\sqrt{\mathrm{5}}}\:. \\ $$ $$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com