Question and Answers Forum
Question Number 63722 by mathmax by abdo last updated on 08/Jul/19
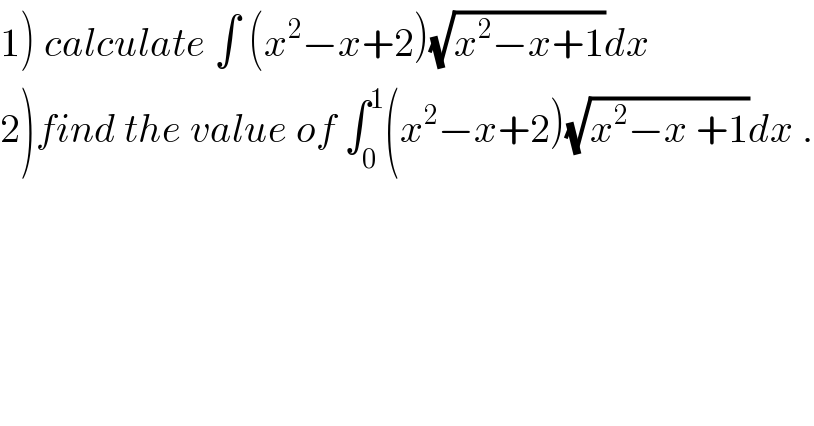
Commented by Prithwish sen last updated on 08/Jul/19
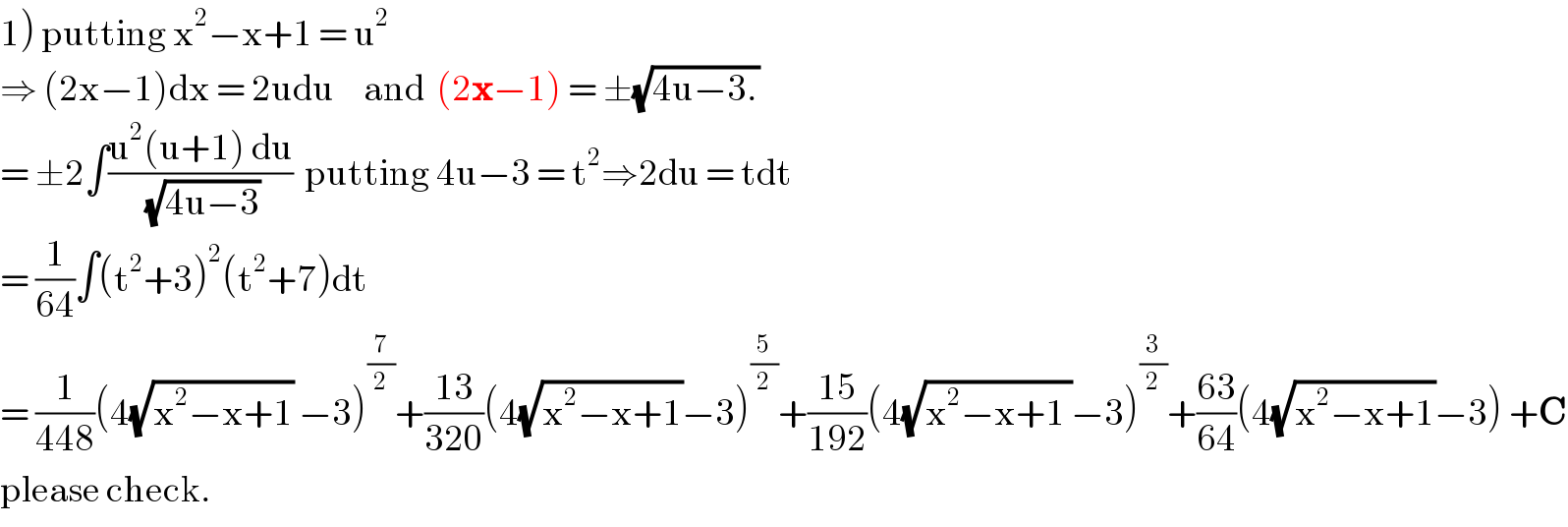
Commented by MJS last updated on 08/Jul/19

Commented by Prithwish sen last updated on 08/Jul/19

Commented by mathmax by abdo last updated on 08/Jul/19

Commented by mathmax by abdo last updated on 08/Jul/19
![2) ∫_0 ^1 (x^2 −x+2)(√(x^2 −x+1))dx =[F(x)]_0 ^1 =F(1)−F(0) F(1)=((3(√3))/(32)){(1/2)((1/(√3)) +(√(1+(1/3))))^2 +(1/(((1/(√3))+(√(1+(1/3))))^2 ))}−1} +((7(√3))/(16)){ (1/(√3)) +(√(1+(1/3))) −(1/((1/(√3))+(√(1+(1/3)))))} F(0) =((3(√3))/(32)){(−(1/3) +(√(1+(1/3))))^2 +(1/((−(1/3) +(√(1+(1/3))))^2 ))} +((7(√3))/(16)){−(1/(√3)) +(√(1+(1/3)))−(1/(−(1/(√3))+(√(1+(1/3)))))}](Q63773.png)
Commented by mathmax by abdo last updated on 08/Jul/19
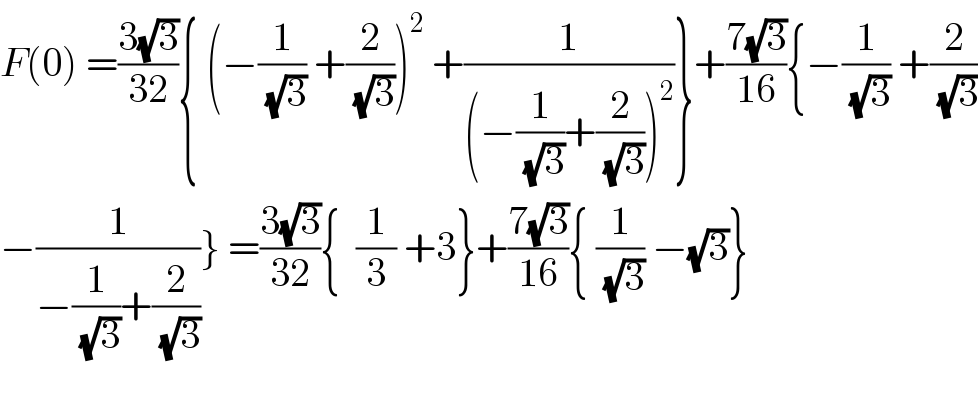
Answered by MJS last updated on 08/Jul/19
![∫(x^2 −x+2)(√(x^2 −x+1))dx= [t=sinh^(−1) (((√3)(2x−1))/3) → dx=(√(x^2 −x+1))dt] =((75)/(128))∫dt+(9/(128))∫cosh 4t dt+((21)/(32))∫cosh 2t dt= =((75)/(128))t+(9/(512))sinh 4t +((21)/(64))sinh 2t= =((75)/(128))sinh^(−1) (((√3)(2x−1))/3) +(1/(64))(2x−1)(8x^2 −8x+5)(√(x^2 −x+1)) +(7/(16))(2x−1)(√(x^2 −x+1))= =((75)/(128))sinh^(−1) (((√3)(2x−1))/3) +(1/(64))(2x−1)(8x^2 −8x+33)(√(x^2 −x+1)) +C ∫_0 ^1 (x^2 −x+2)(√(x^2 −x+1))dx=((33)/(32))+((75)/(64))sinh^(−1) ((√3)/3) =((33)/(32))+((75)/(128))ln 3](Q63737.png)
Commented by Prithwish sen last updated on 08/Jul/19
Commented by mathmax by abdo last updated on 08/Jul/19