Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 64065 by mathmax by abdo last updated on 12/Jul/19

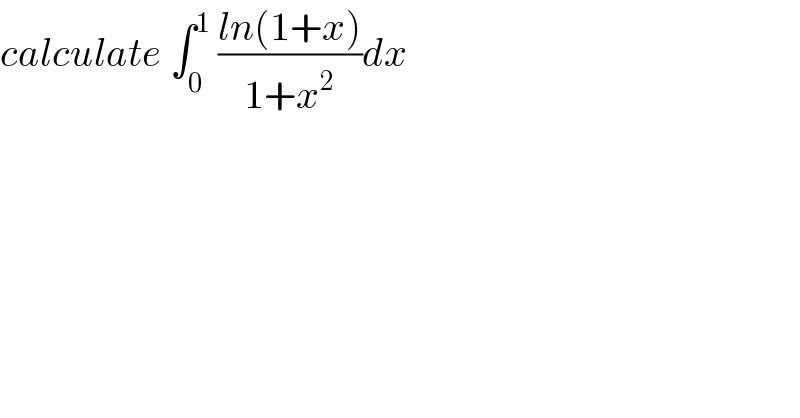
calculate ∫_0 ^1 ((ln(1+x))/(1+x^2 ))dx

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$

Commented by mathmax by abdo last updated on 13/Jul/19

let f(t) =∫_0 ^1 ((ln(1+tx))/(1+x^2 ))dx with t>0 we have f^′ (t) =∫_0 ^1 (x/((1+tx)(1+x^2 ))) dx let decompose F(x) = (x/((tx+1)(x^2 +1))) ⇒F(x)=(a/(tx+1)) +((bx +c)/(x^2 +1)) a= lim_(x→−(1/t)) (tx+1)F(x)=((−1)/(t((1/t^2 )+1))) =((−t^2 )/(t(1+t^2 ))) =−(t/(t^2 +1)) lim_(x→+∞) xF(x) =0 =(a/t) +b ⇒b =−(a/t) =(1/(t^2 +1)) ⇒ F(x)=−(t/((t^2 +1)(tx+1))) +(((x/(t^2 +1)) +c)/(x^2 +1)) F(0) =0 =(t/(t^2 +1)) +c ⇒c=−(t/(t^2 +1)) ⇒ F(x)=(t/((t^2 +1)(tx+1))) +(1/(t^2 +1)) ((x−t)/(x^2 +1)) ⇒ f^′ (t)=∫_0 ^1 F(x)dx =(t/(t^2 +1)) ∫_0 ^1 (dx/(tx+1)) +(1/(t^2 +1)) ∫_0 ^1 ((x−t)/(x^2 +1)) dx =(t/(t^2 +1))(1/t)[ln(tx+1)]_0 ^1 +(1/(2(t^2 +1)))∫_0 ^1 ((2x)/(x^2 +1)) dx−(t/(t^2 +1)) ∫_0 ^1 (dx/(x^2 +1)) =((ln(t+1))/(t^2 +1)) +(1/(2(t^2 +1)))ln(2) −(t/(t^2 +1))(π/4) ⇒ f(t) =∫_0 ^t ((ln(1+u))/(u^2 +1))du+((ln(2))/2) ∫_0 ^t (du/(1+u^2 )) −(π/8) ∫_0 ^t ((2u)/(1+u^2 ))du +c =∫_0 ^t ((ln(1+u))/(1+u^2 )) +((ln(2))/2) arctan(t)−(π/8)ln(1+t^2 ) +c =∫_0 ^1 ((ln(1+tx))/(1+x^2 ))dx ...be continued...

$${let}\:{f}\left({t}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:\:{with}\:{t}>\mathrm{0}\:{we}\:{have} \\ $$$${f}^{'} \left({t}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{x}}{\left(\mathrm{1}+{tx}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:{dx}\:\:\:{let}\:{decompose}\: \\ $$$${F}\left({x}\right)\:=\:\:\frac{{x}}{\left({tx}+\mathrm{1}\right)\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\Rightarrow{F}\left({x}\right)=\frac{{a}}{{tx}+\mathrm{1}}\:+\frac{{bx}\:+{c}}{{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${a}=\:{lim}_{{x}\rightarrow−\frac{\mathrm{1}}{{t}}} \:\:\left({tx}+\mathrm{1}\right){F}\left({x}\right)=\frac{−\mathrm{1}}{{t}\left(\frac{\mathrm{1}}{{t}^{\mathrm{2}} }+\mathrm{1}\right)}\:=\frac{−{t}^{\mathrm{2}} }{{t}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:=−\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${lim}_{{x}\rightarrow+\infty} \:{xF}\left({x}\right)\:=\mathrm{0}\:=\frac{{a}}{{t}}\:+{b}\:\Rightarrow{b}\:=−\frac{{a}}{{t}}\:=\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${F}\left({x}\right)=−\frac{{t}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\left({tx}+\mathrm{1}\right)}\:+\frac{\frac{{x}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+{c}}{{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{0}\:=\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+{c}\:\Rightarrow{c}=−\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${F}\left({x}\right)=\frac{{t}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\left({tx}+\mathrm{1}\right)}\:+\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\frac{{x}−{t}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${f}^{'} \left({t}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:{F}\left({x}\right){dx}\:=\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{dx}}{{tx}+\mathrm{1}}\:+\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}−{t}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:{dx} \\ $$$$=\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\frac{\mathrm{1}}{{t}}\left[{ln}\left({tx}+\mathrm{1}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \:\:+\frac{\mathrm{1}}{\mathrm{2}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:{dx}−\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{dx}}{{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$$=\frac{{ln}\left({t}+\mathrm{1}\right)}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{\mathrm{1}}{\mathrm{2}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}{ln}\left(\mathrm{2}\right)\:−\frac{{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\frac{\pi}{\mathrm{4}}\:\Rightarrow \\ $$$${f}\left({t}\right)\:=\int_{\mathrm{0}} ^{{t}} \:\:\:\frac{{ln}\left(\mathrm{1}+{u}\right)}{{u}^{\mathrm{2}} \:+\mathrm{1}}{du}+\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{2}}\:\int_{\mathrm{0}} ^{{t}} \:\:\frac{{du}}{\mathrm{1}+{u}^{\mathrm{2}} }\:−\frac{\pi}{\mathrm{8}}\:\int_{\mathrm{0}} ^{{t}} \:\:\frac{\mathrm{2}{u}}{\mathrm{1}+{u}^{\mathrm{2}} }{du}\:+{c} \\ $$$$=\int_{\mathrm{0}} ^{{t}} \:\frac{{ln}\left(\mathrm{1}+{u}\right)}{\mathrm{1}+{u}^{\mathrm{2}} }\:+\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{2}}\:{arctan}\left({t}\right)−\frac{\pi}{\mathrm{8}}{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\:\:+{c}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}+{tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$$...{be}\:{continued}... \\ $$

Commented by mathmax by abdo last updated on 13/Jul/19

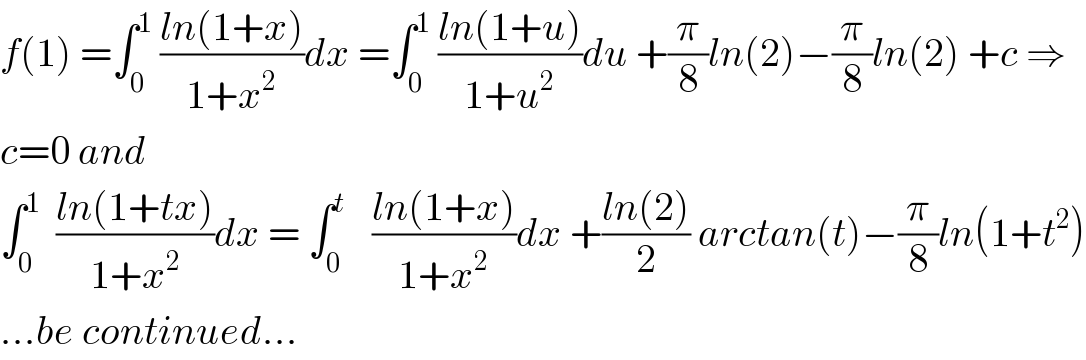
f(1) =∫_0 ^1 ((ln(1+x))/(1+x^2 ))dx =∫_0 ^1 ((ln(1+u))/(1+u^2 ))du +(π/8)ln(2)−(π/8)ln(2) +c ⇒ c=0 and ∫_0 ^1 ((ln(1+tx))/(1+x^2 ))dx = ∫_0 ^t ((ln(1+x))/(1+x^2 ))dx +((ln(2))/2) arctan(t)−(π/8)ln(1+t^2 ) ...be continued...

$${f}\left(\mathrm{1}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{u}\right)}{\mathrm{1}+{u}^{\mathrm{2}} }{du}\:+\frac{\pi}{\mathrm{8}}{ln}\left(\mathrm{2}\right)−\frac{\pi}{\mathrm{8}}{ln}\left(\mathrm{2}\right)\:+{c}\:\Rightarrow \\ $$$${c}=\mathrm{0}\:{and}\: \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:=\:\int_{\mathrm{0}} ^{{t}} \:\:\:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:+\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{2}}\:{arctan}\left({t}\right)−\frac{\pi}{\mathrm{8}}{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right) \\ $$$$...{be}\:{continued}... \\ $$

Commented by mathmax by abdo last updated on 14/Jul/19

let try another way changement x=tanθ give I =∫_0 ^1 ((ln(1+x))/(1+x^2 ))dx = ∫_0 ^(π/4) ((ln(1+tanθ))/(1+tan^2 θ))(1+tan^2 θ)dθ =∫_0 ^(π/4) ln(1+tanθ)dθ chang θ =(π/4)−u ⇒ ∫_0 ^(π/4) ln(1+tanθ)dθ =∫_(π/4) ^0 ln(1+((1−tanu)/(1+tanu)))(−du) =∫_0 ^(π/4) ln((2/(1+tanu)))du =(π/4)ln(2)−∫_0 ^(π/4) ln(1+tanu)du ⇒ I =(π/4)ln(2)−I ⇒2I=(π/4)ln(2) ⇒ I=(π/8)ln(2)

$${let}\:{try}\:{another}\:{way}\:\:{changement}\:\:{x}={tan}\theta\:{give} \\ $$$${I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{{ln}\left(\mathrm{1}+{tan}\theta\right)}{\mathrm{1}+{tan}^{\mathrm{2}} \theta}\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right){d}\theta \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} {ln}\left(\mathrm{1}+{tan}\theta\right){d}\theta\:\:\:{chang}\:\theta\:=\frac{\pi}{\mathrm{4}}−{u}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{ln}\left(\mathrm{1}+{tan}\theta\right){d}\theta\:=\int_{\frac{\pi}{\mathrm{4}}} ^{\mathrm{0}} \:\:{ln}\left(\mathrm{1}+\frac{\mathrm{1}−{tanu}}{\mathrm{1}+{tanu}}\right)\left(−{du}\right) \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{ln}\left(\frac{\mathrm{2}}{\mathrm{1}+{tanu}}\right){du}\:=\frac{\pi}{\mathrm{4}}{ln}\left(\mathrm{2}\right)−\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{ln}\left(\mathrm{1}+{tanu}\right){du}\: \\ $$$$\Rightarrow\:{I}\:=\frac{\pi}{\mathrm{4}}{ln}\left(\mathrm{2}\right)−{I}\:\Rightarrow\mathrm{2}{I}=\frac{\pi}{\mathrm{4}}{ln}\left(\mathrm{2}\right)\:\Rightarrow\:\:{I}=\frac{\pi}{\mathrm{8}}{ln}\left(\mathrm{2}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com