
Question Number 64561 by aliesam last updated on 19/Jul/19

Commented by mathmax by abdo last updated on 20/Jul/19
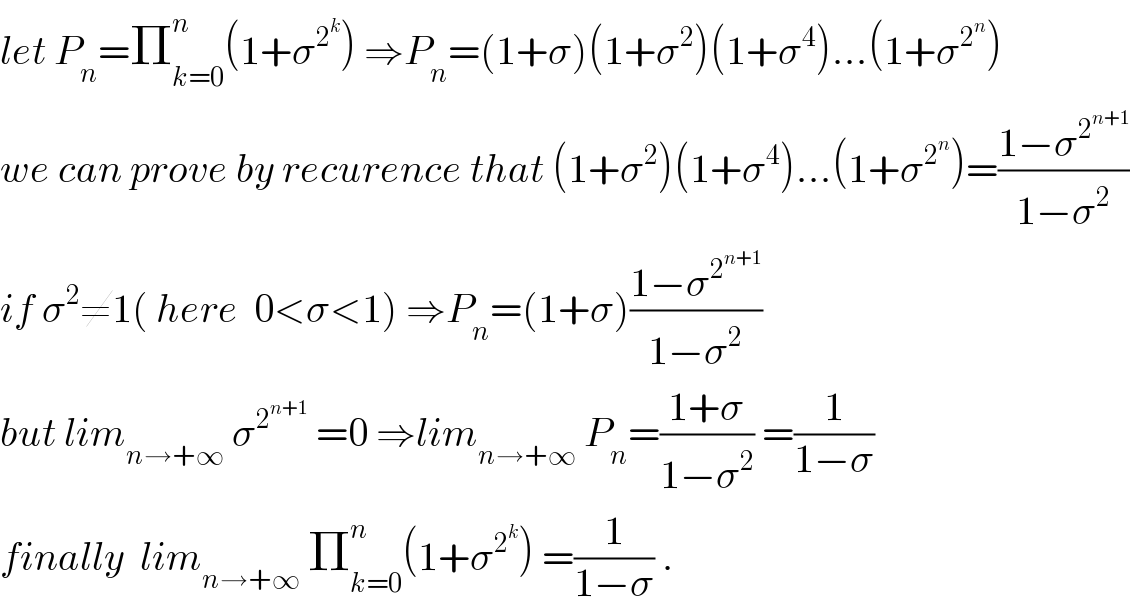
$${let}\:{P}_{{n}} =\prod_{{k}=\mathrm{0}} ^{{n}} \left(\mathrm{1}+\sigma^{\mathrm{2}^{{k}} } \right)\:\Rightarrow{P}_{{n}} =\left(\mathrm{1}+\sigma\right)\left(\mathrm{1}+\sigma^{\mathrm{2}} \right)\left(\mathrm{1}+\sigma^{\mathrm{4}} \right)...\left(\mathrm{1}+\sigma^{\mathrm{2}^{{n}} } \right) \\ $$$${we}\:{can}\:{prove}\:{by}\:{recurence}\:{that}\:\left(\mathrm{1}+\sigma^{\mathrm{2}} \right)\left(\mathrm{1}+\sigma^{\mathrm{4}} \right)...\left(\mathrm{1}+\sigma^{\mathrm{2}^{{n}} } \right)=\frac{\mathrm{1}−\sigma^{\mathrm{2}^{{n}+\mathrm{1}} } }{\mathrm{1}−\sigma^{\mathrm{2}} } \\ $$$${if}\:\sigma^{\mathrm{2}} \neq\mathrm{1}\left(\:{here}\:\:\mathrm{0}<\sigma<\mathrm{1}\right)\:\Rightarrow{P}_{{n}} =\left(\mathrm{1}+\sigma\right)\frac{\mathrm{1}−\sigma^{\mathrm{2}^{{n}+\mathrm{1}} } }{\mathrm{1}−\sigma^{\mathrm{2}} } \\ $$$${but}\:{lim}_{{n}\rightarrow+\infty} \:\sigma^{\mathrm{2}^{{n}+\mathrm{1}} } \:=\mathrm{0}\:\Rightarrow{lim}_{{n}\rightarrow+\infty} \:{P}_{{n}} =\frac{\mathrm{1}+\sigma}{\mathrm{1}−\sigma^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{1}−\sigma} \\ $$$${finally}\:\:{lim}_{{n}\rightarrow+\infty} \:\prod_{{k}=\mathrm{0}} ^{{n}} \left(\mathrm{1}+\sigma^{\mathrm{2}^{{k}} } \right)\:=\frac{\mathrm{1}}{\mathrm{1}−\sigma}\:. \\ $$
Answered by JDamian last updated on 20/Jul/19

$${The}\:{answer}\:{is}\:\frac{\mathrm{1}}{\mathrm{1}−\delta} \\ $$$$ \\ $$$${let}\:{P}_{{n}} =\underset{{k}=\mathrm{0}} {\overset{{n}} {\Pi}}\left(\mathrm{1}+\delta^{\mathrm{2}^{{k}} } \right).\:{It}\:{is}\:{easy}\:{to}\:{show}\:{that} \\ $$$${P}_{{n}} \:{is}\:{actually}\:{the}\:\boldsymbol{{sum}}\:\boldsymbol{{of}}\:\boldsymbol{{the}}\:\boldsymbol{{following}} \\ $$$$\boldsymbol{{geometric}}\:\boldsymbol{{progession}},\:{which}: \\ $$$$−\:{first}\:{value}\:{is}\:\mathrm{1}. \\ $$$$−\:{common}\:{ratio}\:{is}\:\delta. \\ $$$$−\:{length}\:{is}\:\mathrm{2}^{{n}} . \\ $$$$ \\ $$$${P}_{{n}} =\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}^{{n}} −\mathrm{1}} {\sum}}\delta^{{k}} \:\:\forall\delta\:\:\:\mathrm{0}<\delta<\mathrm{1} \\ $$$$\underset{{n}\rightarrow\infty} {{lim}}\:{P}_{{n}} =\frac{\mathrm{1}}{\mathrm{1}−\delta} \\ $$
