
Question and Answers Forum
Question Number 65200 by rajesh4661kumar@gmail.com last updated on 26/Jul/19
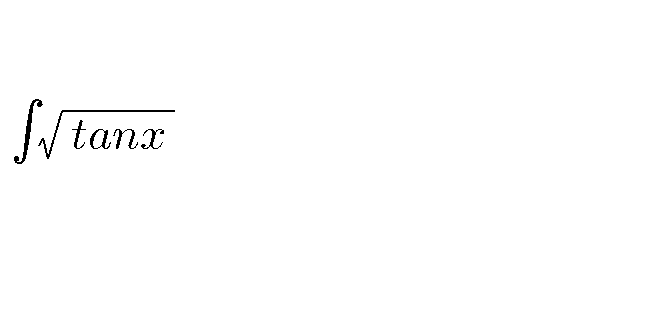
Answered by ajfour last updated on 26/Jul/19
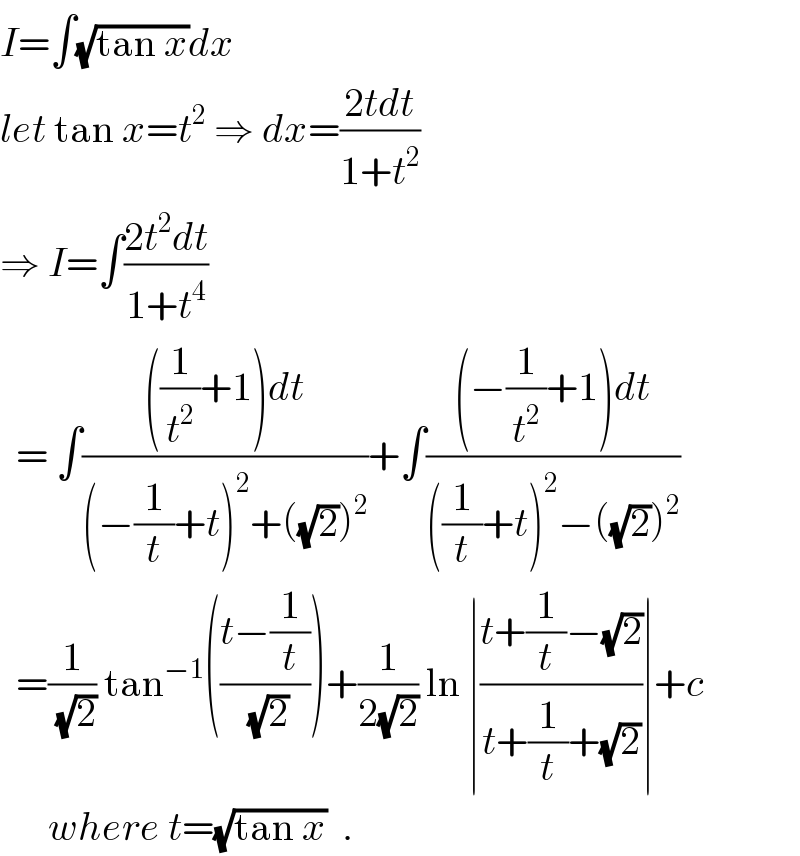
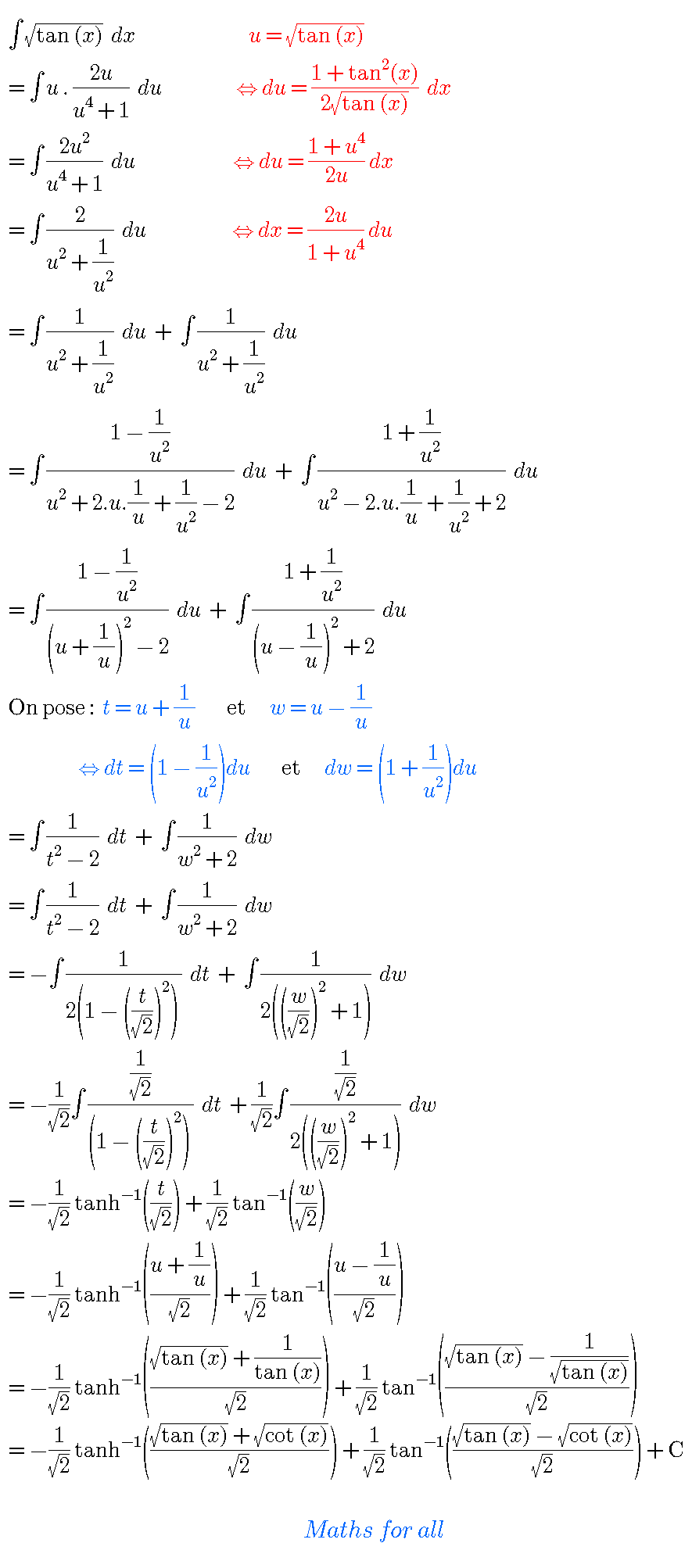
Answered by zakaria elghaouti last updated on 27/Jul/19
| ||
Question and Answers Forum | ||
Question Number 65200 by rajesh4661kumar@gmail.com last updated on 26/Jul/19 | ||
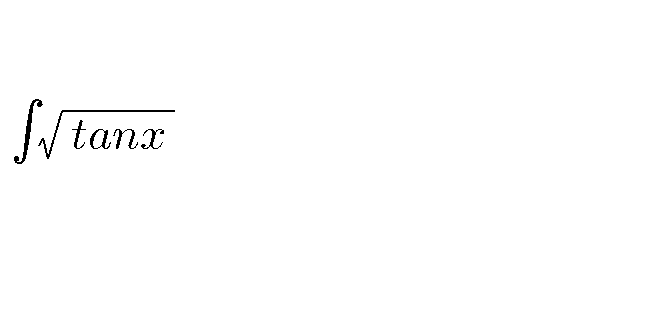 | ||
Answered by ajfour last updated on 26/Jul/19 | ||
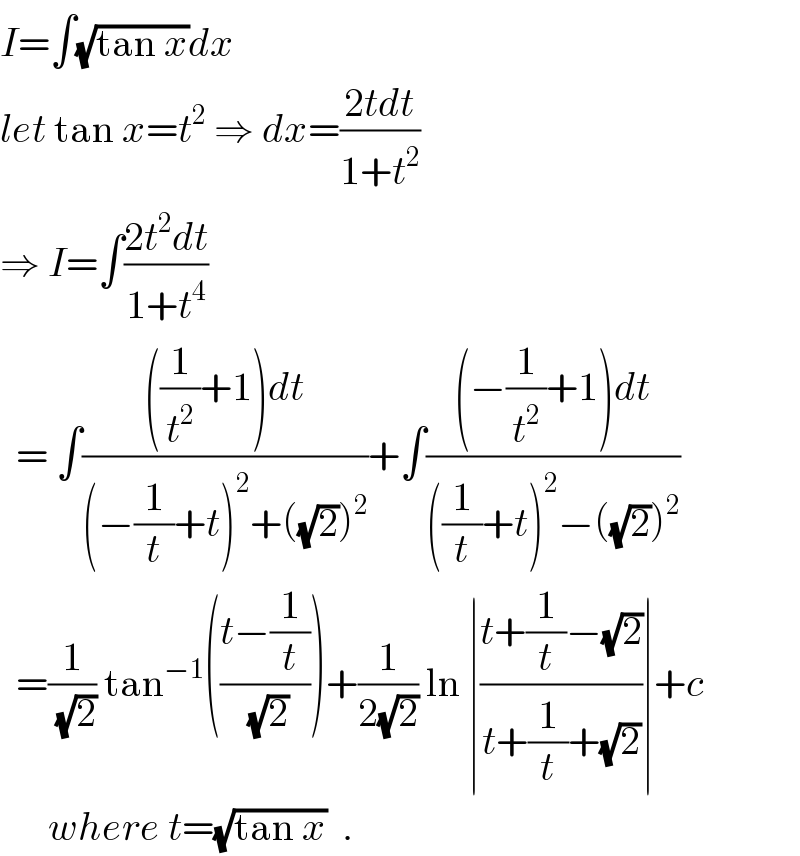 | ||
| ||
Answered by zakaria elghaouti last updated on 27/Jul/19 | ||
 | ||