
Question Number 65491 by mr W last updated on 30/Jul/19

Commented by mr W last updated on 30/Jul/19
![[Q65312 reposted] find x=?](Q65493.png)
$$\left[{Q}\mathrm{65312}\:{reposted}\right] \\ $$$${find}\:{x}=? \\ $$
Answered by mr W last updated on 30/Jul/19

Commented by mr W last updated on 30/Jul/19
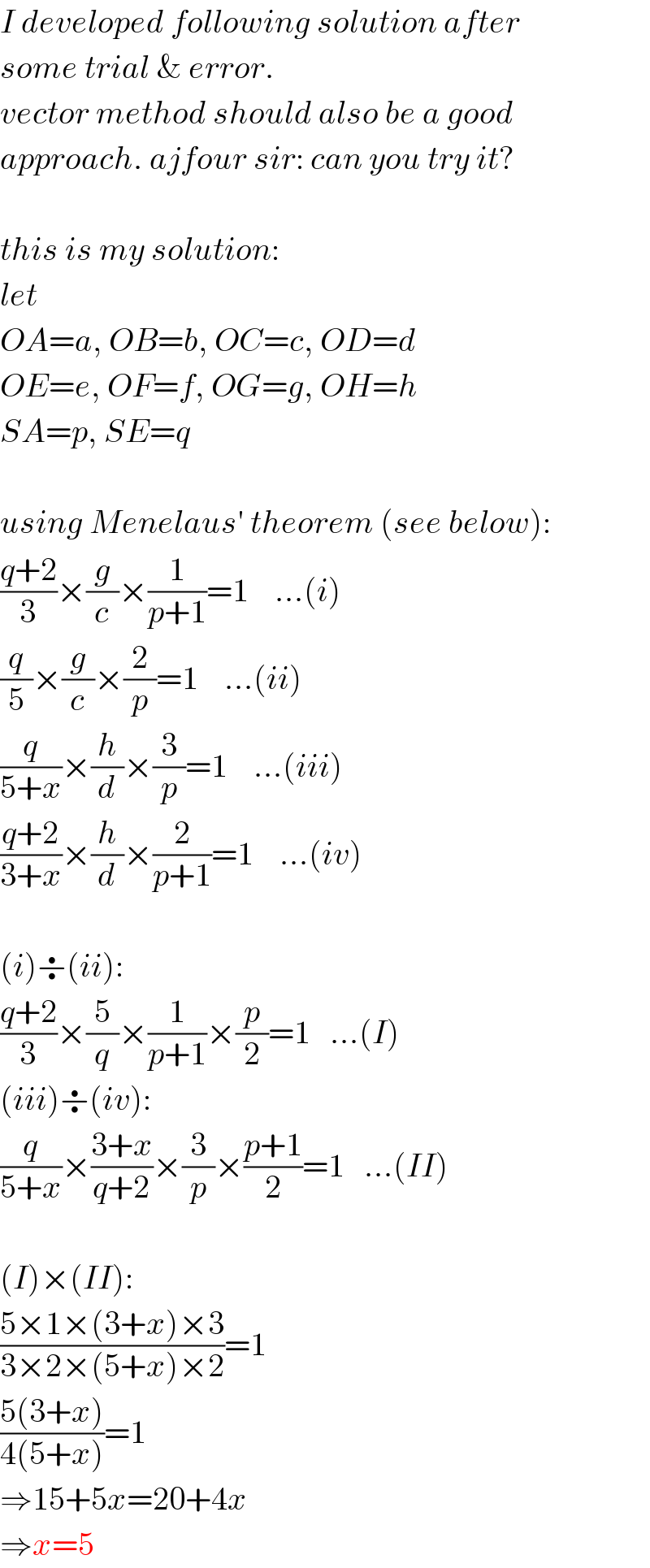
$${I}\:{developed}\:{following}\:{solution}\:{after} \\ $$$${some}\:{trial}\:\&\:{error}. \\ $$$${vector}\:{method}\:{should}\:{also}\:{be}\:{a}\:{good} \\ $$$${approach}.\:{ajfour}\:{sir}:\:{can}\:{you}\:{try}\:{it}? \\ $$$$ \\ $$$${this}\:{is}\:{my}\:{solution}: \\ $$$${let} \\ $$$${OA}={a},\:{OB}={b},\:{OC}={c},\:{OD}={d} \\ $$$${OE}={e},\:{OF}={f},\:{OG}={g},\:{OH}={h} \\ $$$${SA}={p},\:{SE}={q} \\ $$$$ \\ $$$${using}\:{Menelaus}'\:{theorem}\:\left({see}\:{below}\right): \\ $$$$\frac{{q}+\mathrm{2}}{\mathrm{3}}×\frac{{g}}{{c}}×\frac{\mathrm{1}}{{p}+\mathrm{1}}=\mathrm{1}\:\:\:\:...\left({i}\right) \\ $$$$\frac{{q}}{\mathrm{5}}×\frac{{g}}{{c}}×\frac{\mathrm{2}}{{p}}=\mathrm{1}\:\:\:\:...\left({ii}\right) \\ $$$$\frac{{q}}{\mathrm{5}+{x}}×\frac{{h}}{{d}}×\frac{\mathrm{3}}{{p}}=\mathrm{1}\:\:\:\:...\left({iii}\right) \\ $$$$\frac{{q}+\mathrm{2}}{\mathrm{3}+{x}}×\frac{{h}}{{d}}×\frac{\mathrm{2}}{{p}+\mathrm{1}}=\mathrm{1}\:\:\:\:...\left({iv}\right) \\ $$$$ \\ $$$$\left({i}\right)\boldsymbol{\div}\left({ii}\right): \\ $$$$\frac{{q}+\mathrm{2}}{\mathrm{3}}×\frac{\mathrm{5}}{{q}}×\frac{\mathrm{1}}{{p}+\mathrm{1}}×\frac{{p}}{\mathrm{2}}=\mathrm{1}\:\:\:...\left({I}\right) \\ $$$$\left({iii}\right)\boldsymbol{\div}\left({iv}\right): \\ $$$$\frac{{q}}{\mathrm{5}+{x}}×\frac{\mathrm{3}+{x}}{{q}+\mathrm{2}}×\frac{\mathrm{3}}{{p}}×\frac{{p}+\mathrm{1}}{\mathrm{2}}=\mathrm{1}\:\:\:...\left({II}\right) \\ $$$$ \\ $$$$\left({I}\right)×\left({II}\right): \\ $$$$\frac{\mathrm{5}×\mathrm{1}×\left(\mathrm{3}+{x}\right)×\mathrm{3}}{\mathrm{3}×\mathrm{2}×\left(\mathrm{5}+{x}\right)×\mathrm{2}}=\mathrm{1} \\ $$$$\frac{\mathrm{5}\left(\mathrm{3}+{x}\right)}{\mathrm{4}\left(\mathrm{5}+{x}\right)}=\mathrm{1} \\ $$$$\Rightarrow\mathrm{15}+\mathrm{5}{x}=\mathrm{20}+\mathrm{4}{x} \\ $$$$\Rightarrow{x}=\mathrm{5} \\ $$
Commented by mr W last updated on 30/Jul/19

Commented by MJS last updated on 31/Jul/19

$$\mathrm{what}\:\mathrm{I}\:\mathrm{did}\:\mathrm{to}\:\mathrm{find}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{was}\:\mathrm{the} \\ $$$$\mathrm{coordinates}−\mathrm{method}\:\mathrm{once}\:\mathrm{more}\:\mathrm{but}\:\mathrm{I}\:\mathrm{had} \\ $$$$\mathrm{no}\:\mathrm{time}\:\mathrm{to}\:\mathrm{type}\:\mathrm{it}.\:\mathrm{anyway}\:\mathrm{I}\:\mathrm{didn}'\mathrm{t}\:\mathrm{expect} \\ $$$$\mathrm{an}\:\mathrm{answer}\:\mathrm{from}\:\mathrm{the}\:\mathrm{original}\:\mathrm{poster}... \\ $$
Commented by Tony Lin last updated on 31/Jul/19

$${actually}\:{there}\:{is}\:{a}\:{special}\:{way}\:{to}\:{solve} \\ $$$${this}\:{question}\:{called}\:``\:{cross}\:{ratio}'' \\ $$$${R}\left({A},{B};{C},{D}\right)={R}\left({E},{F};{G},{H}\right) \\ $$$$\frac{\frac{{AC}}{{AD}}}{\frac{{BC}}{{BD}}}=\frac{\frac{{EG}}{{EH}}}{\frac{{FG}}{{FH}}} \\ $$$$\frac{\frac{\mathrm{2}}{\mathrm{3}}}{\frac{\mathrm{1}}{\mathrm{2}}}=\frac{\frac{\mathrm{5}}{\mathrm{5}+{x}}}{\frac{\mathrm{3}}{\mathrm{3}+{x}}} \\ $$$$\mathrm{20}+\mathrm{4}{x}=\mathrm{15}+\mathrm{5}{x} \\ $$$${x}=\mathrm{5} \\ $$$${but}\:{i}\:{hope}\:{that}\:{there}\:{are}\:{some}\:{simple} \\ $$$${ways}\:{to}\:{solve}\:{without}\:{a}\:{theorem}\:{that} \\ $$$${few}\:{people}\:{heard} \\ $$$${thanks}\:{for}\:{your}\:{help} \\ $$
Commented by Tony Lin last updated on 31/Jul/19

Commented by Tony Lin last updated on 31/Jul/19

Commented by Tony Lin last updated on 31/Jul/19
![without loss of generality ⇒using coordinate-system hypothesis let O(0,0),A(0,−s),B(1,−s),C(2,−s) D(3,−s),E(0,−t),F((6/5),−t−(8/5)) G(3,−t−4),H(x,y) O,B,F are collinear ⇒t+(8/5)=(6/5)s O,C,G are collinear ⇒t+4=(3/2)s ⇒ { ((t+(8/5)=(6/5)s)),((t+4=(3/2)s)) :} ⇒(t,s)=(8,8) ∵H is the point of intersection of L_(OD) &L_(EG) ∴ { ((y=((−8)/3)x)),((y=((−4)/3)x−8)) :} ⇒(x,y)=(6,−16) GH=(√((6−3)^2 +[−16−(−12)]^2 ))=5 i only think of this idea now](Q65540.png)
$${without}\:{loss}\:{of}\:{generality} \\ $$$$\Rightarrow{using}\:{coordinate}-{system}\:{hypothesis} \\ $$$${let}\:{O}\left(\mathrm{0},\mathrm{0}\right),{A}\left(\mathrm{0},−{s}\right),{B}\left(\mathrm{1},−{s}\right),{C}\left(\mathrm{2},−{s}\right) \\ $$$${D}\left(\mathrm{3},−{s}\right),{E}\left(\mathrm{0},−{t}\right),{F}\left(\frac{\mathrm{6}}{\mathrm{5}},−{t}−\frac{\mathrm{8}}{\mathrm{5}}\right) \\ $$$${G}\left(\mathrm{3},−{t}−\mathrm{4}\right),{H}\left({x},{y}\right) \\ $$$${O},{B},{F}\:{are}\:{collinear} \\ $$$$\Rightarrow{t}+\frac{\mathrm{8}}{\mathrm{5}}=\frac{\mathrm{6}}{\mathrm{5}}{s} \\ $$$${O},{C},{G}\:{are}\:{collinear} \\ $$$$\Rightarrow{t}+\mathrm{4}=\frac{\mathrm{3}}{\mathrm{2}}{s} \\ $$$$\Rightarrow\begin{cases}{{t}+\frac{\mathrm{8}}{\mathrm{5}}=\frac{\mathrm{6}}{\mathrm{5}}{s}}\\{{t}+\mathrm{4}=\frac{\mathrm{3}}{\mathrm{2}}{s}}\end{cases} \\ $$$$\Rightarrow\left({t},{s}\right)=\left(\mathrm{8},\mathrm{8}\right) \\ $$$$\because{H}\:{is}\:{the}\:{point}\:{of}\:{intersection}\:{of}\:{L}_{{OD}} \&{L}_{{EG}} \\ $$$$\therefore\begin{cases}{{y}=\frac{−\mathrm{8}}{\mathrm{3}}{x}}\\{{y}=\frac{−\mathrm{4}}{\mathrm{3}}{x}−\mathrm{8}}\end{cases} \\ $$$$\Rightarrow\left({x},{y}\right)=\left(\mathrm{6},−\mathrm{16}\right) \\ $$$${GH}=\sqrt{\left(\mathrm{6}−\mathrm{3}\right)^{\mathrm{2}} +\left[−\mathrm{16}−\left(−\mathrm{12}\right)\right]^{\mathrm{2}} }=\mathrm{5} \\ $$$${i}\:{only}\:{think}\:{of}\:{this}\:{idea}\:{now} \\ $$
Commented by MJS last updated on 31/Jul/19

$$...\mathrm{this}\:\mathrm{is}\:\mathrm{exactly}\:\mathrm{what}\:\mathrm{I}\:\mathrm{did}. \\ $$$$\mathrm{thank}\:\mathrm{you}\:\mathrm{both}\:\mathrm{for}\:\mathrm{above}\:\mathrm{theorems} \\ $$
Commented by mr W last updated on 31/Jul/19

$${Lin}\:{sir},\:{thanks}\:{for}\:{all}\:{the}\:{information}! \\ $$
