
Question Number 65589 by mr W last updated on 31/Jul/19

Commented by mr W last updated on 31/Jul/19

$${Answer}\:{to}\:{Q}\mathrm{65277} \\ $$
Commented by Tanmay chaudhury last updated on 31/Jul/19
$${sir}\:{pls}\:{explain}\:{if}\:{possible}.. \\ $$
Commented by mr W last updated on 12/Feb/21
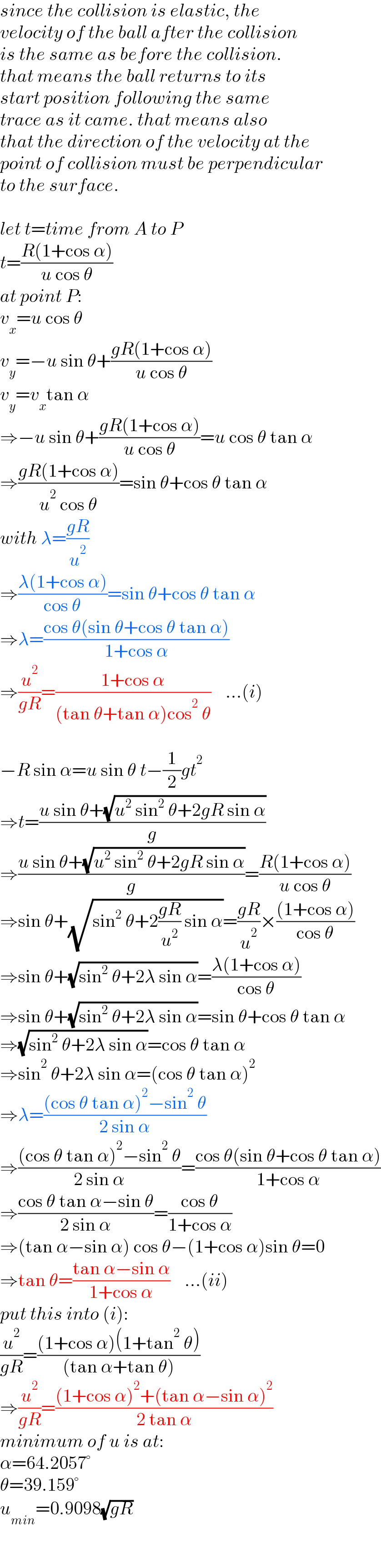
$${since}\:{the}\:{collision}\:{is}\:{elastic},\:{the} \\ $$$${velocity}\:{of}\:{the}\:{ball}\:{after}\:{the}\:{collision} \\ $$$${is}\:{the}\:{same}\:{as}\:{before}\:{the}\:{collision}. \\ $$$${that}\:{means}\:{the}\:{ball}\:{returns}\:{to}\:{its} \\ $$$${start}\:{position}\:{following}\:{the}\:{same} \\ $$$${trace}\:{as}\:{it}\:{came}.\:{that}\:{means}\:{also} \\ $$$${that}\:{the}\:{direction}\:{of}\:{the}\:{velocity}\:{at}\:{the} \\ $$$${point}\:{of}\:{collision}\:{must}\:{be}\:{perpendicular} \\ $$$${to}\:{the}\:{surface}. \\ $$$$ \\ $$$${let}\:{t}={time}\:{from}\:{A}\:{to}\:{P} \\ $$$${t}=\frac{{R}\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{{u}\:\mathrm{cos}\:\theta} \\ $$$${at}\:{point}\:{P}: \\ $$$${v}_{{x}} ={u}\:\mathrm{cos}\:\theta \\ $$$${v}_{{y}} =−{u}\:\mathrm{sin}\:\theta+\frac{{gR}\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{{u}\:\mathrm{cos}\:\theta} \\ $$$${v}_{{y}} ={v}_{{x}} \mathrm{tan}\:\alpha \\ $$$$\Rightarrow−{u}\:\mathrm{sin}\:\theta+\frac{{gR}\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{{u}\:\mathrm{cos}\:\theta}={u}\:\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha \\ $$$$\Rightarrow\frac{{gR}\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{{u}^{\mathrm{2}} \:\mathrm{cos}\:\theta}=\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha \\ $$$${with}\:\lambda=\frac{{gR}}{{u}^{\mathrm{2}} } \\ $$$$\Rightarrow\frac{\lambda\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{\mathrm{cos}\:\theta}=\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha \\ $$$$\Rightarrow\lambda=\frac{\mathrm{cos}\:\theta\left(\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha\right)}{\mathrm{1}+\mathrm{cos}\:\alpha} \\ $$$$\Rightarrow\frac{{u}^{\mathrm{2}} }{{gR}}=\frac{\mathrm{1}+\mathrm{cos}\:\alpha}{\left(\mathrm{tan}\:\theta+\mathrm{tan}\:\alpha\right)\mathrm{cos}^{\mathrm{2}} \:\theta}\:\:\:\:...\left({i}\right) \\ $$$$ \\ $$$$−{R}\:\mathrm{sin}\:\alpha={u}\:\mathrm{sin}\:\theta\:{t}−\frac{\mathrm{1}}{\mathrm{2}}{gt}^{\mathrm{2}} \\ $$$$\Rightarrow{t}=\frac{{u}\:\mathrm{sin}\:\theta+\sqrt{{u}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}{gR}\:\mathrm{sin}\:\alpha}}{{g}} \\ $$$$\Rightarrow\frac{{u}\:\mathrm{sin}\:\theta+\sqrt{{u}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}{gR}\:\mathrm{sin}\:\alpha}}{{g}}=\frac{{R}\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{{u}\:\mathrm{cos}\:\theta} \\ $$$$\Rightarrow\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}\frac{{gR}}{{u}^{\mathrm{2}} }\:\mathrm{sin}\:\alpha}=\frac{{gR}}{{u}^{\mathrm{2}} }×\frac{\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{\mathrm{cos}\:\theta} \\ $$$$\Rightarrow\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}\lambda\:\mathrm{sin}\:\alpha}=\frac{\lambda\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)}{\mathrm{cos}\:\theta} \\ $$$$\Rightarrow\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}\lambda\:\mathrm{sin}\:\alpha}=\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha \\ $$$$\Rightarrow\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}\lambda\:\mathrm{sin}\:\alpha}=\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha \\ $$$$\Rightarrow\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}\lambda\:\mathrm{sin}\:\alpha=\left(\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha\right)^{\mathrm{2}} \\ $$$$\Rightarrow\lambda=\frac{\left(\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha\right)^{\mathrm{2}} −\mathrm{sin}^{\mathrm{2}} \:\theta}{\mathrm{2}\:\mathrm{sin}\:\alpha} \\ $$$$\Rightarrow\frac{\left(\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha\right)^{\mathrm{2}} −\mathrm{sin}^{\mathrm{2}} \:\theta}{\mathrm{2}\:\mathrm{sin}\:\alpha}=\frac{\mathrm{cos}\:\theta\left(\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha\right)}{\mathrm{1}+\mathrm{cos}\:\alpha} \\ $$$$\Rightarrow\frac{\mathrm{cos}\:\theta\:\mathrm{tan}\:\alpha−\mathrm{sin}\:\theta}{\mathrm{2}\:\mathrm{sin}\:\alpha}=\frac{\mathrm{cos}\:\theta}{\mathrm{1}+\mathrm{cos}\:\alpha} \\ $$$$\Rightarrow\left(\mathrm{tan}\:\alpha−\mathrm{sin}\:\alpha\right)\:\mathrm{cos}\:\theta−\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)\mathrm{sin}\:\theta=\mathrm{0} \\ $$$$\Rightarrow\mathrm{tan}\:\theta=\frac{\mathrm{tan}\:\alpha−\mathrm{sin}\:\alpha}{\mathrm{1}+\mathrm{cos}\:\alpha}\:\:\:\:...\left({ii}\right) \\ $$$${put}\:{this}\:{into}\:\left({i}\right): \\ $$$$\frac{{u}^{\mathrm{2}} }{{gR}}=\frac{\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta\right)}{\left(\mathrm{tan}\:\alpha+\mathrm{tan}\:\theta\right)} \\ $$$$\Rightarrow\frac{{u}^{\mathrm{2}} }{{gR}}=\frac{\left(\mathrm{1}+\mathrm{cos}\:\alpha\right)^{\mathrm{2}} +\left(\mathrm{tan}\:\alpha−\mathrm{sin}\:\alpha\right)^{\mathrm{2}} }{\mathrm{2}\:\mathrm{tan}\:\alpha} \\ $$$${minimum}\:{of}\:{u}\:{is}\:{at}: \\ $$$$\alpha=\mathrm{64}.\mathrm{2057}° \\ $$$$\theta=\mathrm{39}.\mathrm{159}° \\ $$$${u}_{{min}} =\mathrm{0}.\mathrm{9098}\sqrt{{gR}} \\ $$
Commented by Tanmay chaudhury last updated on 31/Jul/19

$${thank}\:{you}\:{sir}...{this}\:{problem}\:{remain}\:{unsolved} \\ $$$${till}\:{date}...{you}\:{did}\:{it}\:{excellent}... \\ $$
Commented by mr W last updated on 01/Aug/19

Commented by mr W last updated on 01/Aug/19

Commented by mr W last updated on 01/Aug/19

Commented by mr W last updated on 01/Aug/19

