Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 65775 by mathmax by abdo last updated on 03/Aug/19

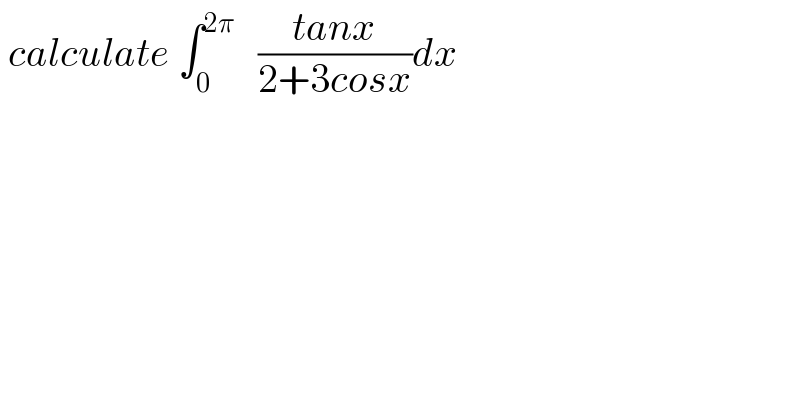
calculate ∫_0 ^(2π) ((tanx)/(2+3cosx))dx

$$\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\:\frac{{tanx}}{\mathrm{2}+\mathrm{3}{cosx}}{dx} \\ $$

Commented by mathmax by abdo last updated on 04/Aug/19

let I =∫_0 ^(2π) ((tanx)/(2+3cosx))dx ⇒ I =∫_0 ^(2π) ((sinx)/(2cosx +3cos^2 x))dx =∫_0 ^(2π) ((sinx)/(2cosx +3((1+cos(2x))/2)))dx =∫_0 ^(2π) ((2sinx)/(4cosx +3+3cos(2x)))dx changement e^(ix) =z give I =∫_(∣z∣=1) ((2((z−z^(−1) )/(2i)))/(4((z+z^(−1) )/2)+3((z^2 +z^(−2) )/2)+3)) (dz/(iz)) =− ∫_(∣z∣=1) ((z−z^(−1) )/(z(2z+2z^(−1) +(3/2)z^2 +(3/2)z^(−2) )))dz =−2∫_(∣z∣=1) ((z−z^(−1) )/(z{4z+4z^(−1) +3z^2 +3z^(−2) }))dz =−2 ∫_(∣z∣=2) ((z^2 −1)/(z^2 {4z +4z^(−1) +3z^2 +3z^(−2) }))dz =−2 ∫_(∣z∣=2) ((z^2 −1)/(4z^3 +4z +3z^4 +3)) dz let ϕ(z) =((z^2 −1)/(3z^4 +4z^3 +4z +3)) poles of ϕ? let solve 3z^4 +4z^3 +4z +3 =0 the roots are z_1 ∼−1,6084(real) z_2 ∼−0,6217(real) z_3 ∼0,4484+0,8938i (complex) z_4 =−0,4484 −0,8938i )complex) ⇒ ϕ(z) =((z^2 −1)/(3(z−z_1 )(z−z_2 )(z−z_3 )(z−z_4 ))) ∫_(∣z∣=1) ϕ(z)dz =2iπ {Res(ϕ,z_2 )+Res(ϕ,z_3 )} if ∣z_3 ∣<1 but ∣z_3 ∣ =(√((0,4484)^(2 ) +(0,8938)^2 )))=....be continued...

$${let}\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\frac{{tanx}}{\mathrm{2}+\mathrm{3}{cosx}}{dx}\:\Rightarrow\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\frac{{sinx}}{\mathrm{2}{cosx}\:+\mathrm{3}{cos}^{\mathrm{2}} {x}}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\:\frac{{sinx}}{\mathrm{2}{cosx}\:+\mathrm{3}\frac{\mathrm{1}+{cos}\left(\mathrm{2}{x}\right)}{\mathrm{2}}}{dx}\:=\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\frac{\mathrm{2}{sinx}}{\mathrm{4}{cosx}\:+\mathrm{3}+\mathrm{3}{cos}\left(\mathrm{2}{x}\right)}{dx} \\ $$$${changement}\:{e}^{{ix}} \:={z}\:{give}\: \\ $$$${I}\:=\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\:\frac{\mathrm{2}\frac{{z}−{z}^{−\mathrm{1}} }{\mathrm{2}{i}}}{\mathrm{4}\frac{{z}+{z}^{−\mathrm{1}} }{\mathrm{2}}+\mathrm{3}\frac{{z}^{\mathrm{2}} \:+{z}^{−\mathrm{2}} }{\mathrm{2}}+\mathrm{3}}\:\frac{{dz}}{{iz}} \\ $$$$=−\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\:\frac{{z}−{z}^{−\mathrm{1}} }{{z}\left(\mathrm{2}{z}+\mathrm{2}{z}^{−\mathrm{1}} \:+\frac{\mathrm{3}}{\mathrm{2}}{z}^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{2}}{z}^{−\mathrm{2}} \right)}{dz} \\ $$$$=−\mathrm{2}\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\frac{{z}−{z}^{−\mathrm{1}} }{{z}\left\{\mathrm{4}{z}+\mathrm{4}{z}^{−\mathrm{1}} \:+\mathrm{3}{z}^{\mathrm{2}} \:+\mathrm{3}{z}^{−\mathrm{2}} \right\}}{dz} \\ $$$$=−\mathrm{2}\:\int_{\mid{z}\mid=\mathrm{2}} \:\:\:\:\frac{{z}^{\mathrm{2}} −\mathrm{1}}{{z}^{\mathrm{2}} \left\{\mathrm{4}{z}\:+\mathrm{4}{z}^{−\mathrm{1}} \:+\mathrm{3}{z}^{\mathrm{2}} \:+\mathrm{3}{z}^{−\mathrm{2}} \right\}}{dz} \\ $$$$=−\mathrm{2}\:\int_{\mid{z}\mid=\mathrm{2}} \:\:\:\:\frac{{z}^{\mathrm{2}} −\mathrm{1}}{\mathrm{4}{z}^{\mathrm{3}} +\mathrm{4}{z}\:+\mathrm{3}{z}^{\mathrm{4}} \:+\mathrm{3}}\:{dz}\:\:{let}\:\varphi\left({z}\right)\:=\frac{{z}^{\mathrm{2}} −\mathrm{1}}{\mathrm{3}{z}^{\mathrm{4}} \:+\mathrm{4}{z}^{\mathrm{3}} \:+\mathrm{4}{z}\:+\mathrm{3}} \\ $$$${poles}\:{of}\:\varphi?\:\:\:{let}\:{solve}\:\mathrm{3}{z}^{\mathrm{4}} \:+\mathrm{4}{z}^{\mathrm{3}} \:+\mathrm{4}{z}\:+\mathrm{3}\:=\mathrm{0}\:{the}\:{roots}\:{are}\: \\ $$$${z}_{\mathrm{1}} \sim−\mathrm{1},\mathrm{6084}\left({real}\right) \\ $$$${z}_{\mathrm{2}} \sim−\mathrm{0},\mathrm{6217}\left({real}\right) \\ $$$${z}_{\mathrm{3}} \sim\mathrm{0},\mathrm{4484}+\mathrm{0},\mathrm{8938}{i}\:\:\left({complex}\right) \\ $$$$\left.{z}_{\mathrm{4}} \left.=−\mathrm{0},\mathrm{4484}\:−\mathrm{0},\mathrm{8938}{i}\:\right){complex}\right)\:\Rightarrow \\ $$$$\varphi\left({z}\right)\:=\frac{{z}^{\mathrm{2}} −\mathrm{1}}{\mathrm{3}\left({z}−{z}_{\mathrm{1}} \right)\left({z}−{z}_{\mathrm{2}} \right)\left({z}−{z}_{\mathrm{3}} \right)\left({z}−{z}_{\mathrm{4}} \right)} \\ $$$$\int_{\mid{z}\mid=\mathrm{1}} \varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\left\{{Res}\left(\varphi,{z}_{\mathrm{2}} \right)+{Res}\left(\varphi,{z}_{\mathrm{3}} \right)\right\}\:{if}\:\mid{z}_{\mathrm{3}} \mid<\mathrm{1}\:{but} \\ $$$$\left.\mid{z}_{\mathrm{3}} \mid\:=\sqrt{\left(\mathrm{0},\mathrm{4484}\right)^{\mathrm{2}\:} +\left(\mathrm{0},\mathrm{8938}\right)^{\mathrm{2}} }\right)=....{be}\:{continued}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com