Question and Answers Forum
Question Number 66055 by ajfour last updated on 08/Aug/19

Commented by ajfour last updated on 08/Aug/19
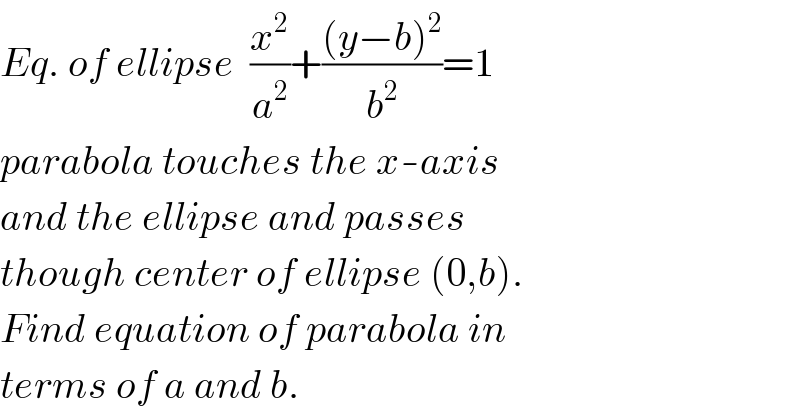
Answered by mr W last updated on 09/Aug/19
![eqn. of parabola: y=b(1−(x/p))^2 eqn. of ellipse: ((x/a))^2 +((y/b)−1)^2 =1 touch point P(h,k) let m=tan θ for tangent at touch point y′=−((2b)/p)(1−(h/p))=m ⇒h=p(1+((pm)/(2b))) (x/a^2 )+(1/b)((y/b)−1)y′=0 (h/a^2 )+(1/b)((k/b)−1)m=0 ⇒k=b[1−((pb)/(ma^2 ))(1+((pm)/(2b)))] ((h/a))^2 +((k/b)−1)^2 =1 ⇒(p^2 /a^2 )(1+(b^2 /(m^2 a^2 )))(1+((pm)/(2b)))^2 =1 ...(i) (k/b)=(1−(h/p))^2 ⇒1−((pb)/(ma^2 ))(1+((pm)/(2b)))=(((pm)/(2b)))^2 ...(ii) let λ=(p/a), μ=(b/a) from (i): ⇒λ^2 (1+(μ^2 /m^2 ))(1+((mλ)/(2μ)))^2 =1 ...(I) from (ii): ⇒1−((μλ)/m)(1+((mλ)/(2μ)))=(((mλ)/(2μ)))^2 ⇒(2+(m^2 /μ^2 ))λ^2 +((4μ)/m)λ−4 =0 ...(II) ⇒λ=(((2m)/μ)/(2+(m^2 /μ^2 )))=((2μm)/(2μ^2 +m^2 )) or m^2 −((2μ)/λ)m+2μ^2 =0 ⇒(m/μ)=(1/λ)(1+(√(1−2λ^2 ))) put this into (I): ⇒((λ^2 (3+(√(1−2λ^2 )))^2 )/8)(2+(λ^2 /(1−λ^2 +(√(1−2λ^2 )))))=1 ⇒λ=0.5194 (constant, independent from μ!) ⇒p=λa=0.5194a eqn. of parabola: ⇒y=b(1−(x/(0.5194a)))^2](Q66076.png)
Commented by MJS last updated on 09/Aug/19
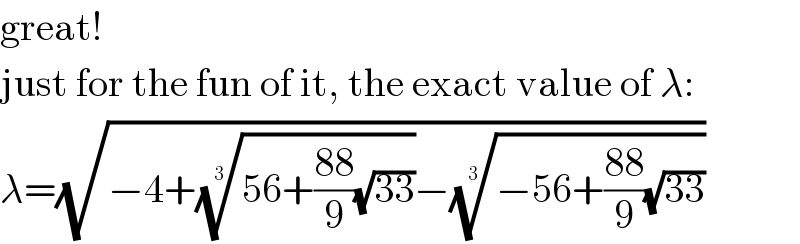
Commented by mr W last updated on 08/Aug/19

Commented by mr W last updated on 08/Aug/19

Commented by mr W last updated on 09/Aug/19
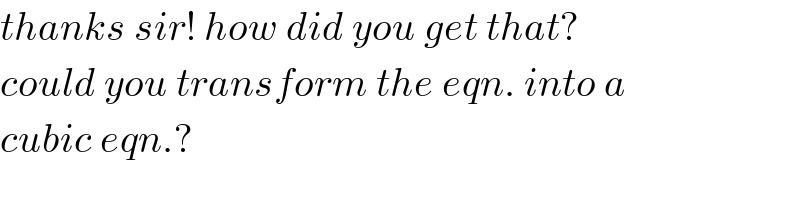
Commented by MJS last updated on 12/Aug/19

Commented by mr W last updated on 14/Aug/19