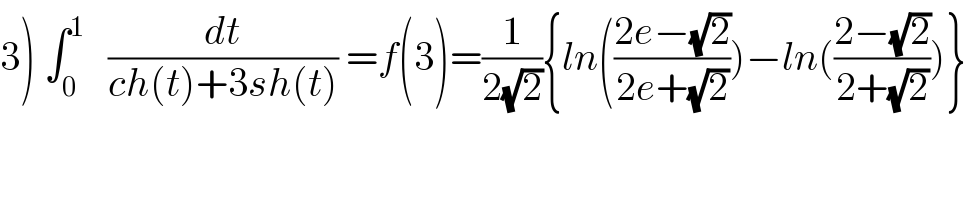Question and Answers Forum
Question Number 66062 by mathmax by abdo last updated on 08/Aug/19
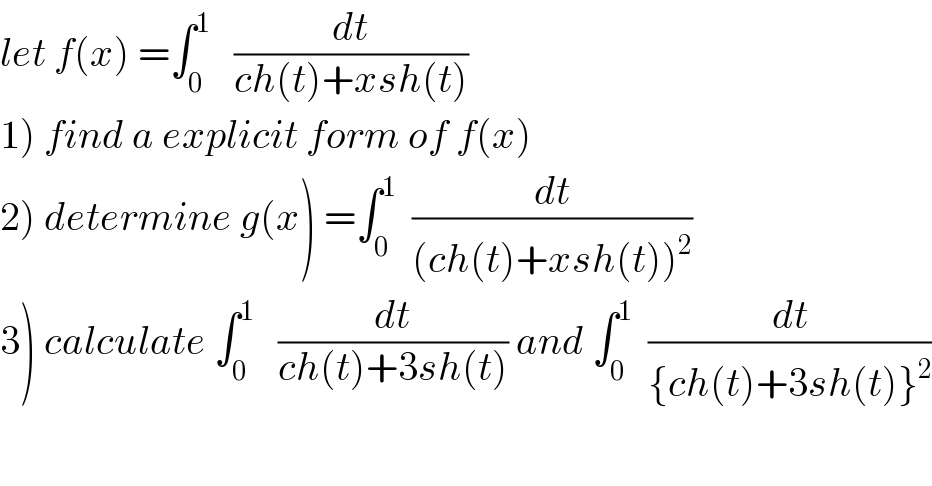
Commented by mathmax by abdo last updated on 11/Aug/19
![1) we have f(x)=∫_0 ^1 (dt/(ch(t)+xsh(t))) ⇒ f(x)=∫_0 ^1 ((2dt)/(e^t +e^(−t) +x(e^t −e^(−t) ))) =∫_0 ^1 ((2dt)/((1+x)e^t +(1−x)e^(−t) )) changement e^t =u give f(x)=∫_1 ^e (2/((1+x)u +(1−x)u^(−1) ))(du/u) =∫_1 ^e ((2du)/((1+x)u^2 +(1−x))) =(2/(1+x)) ∫_1 ^e (du/(u^2 +((1−x)/(1+x)))) case 1 ((1−x)/(1+x))>0 ⇒∣x∣<1 we do the changement u=(√((1−x)/(1+x)))z ⇒ f(x) =(2/(1+x)) ∫_(√((1+x)/(1−x))) ^(e(√((1+x)/(1−x)))) ((1+x)/(1−x)) (1/(1+z^2 )) (√((1−x)/(1+x)))dz =(2/(1−x))((√(1−x))/(√(1+x))) [arctan(z)]_(√((1+x)/(1−x))) ^(e(√((1+x)/(1−x)))) =(2/(√(1−x^2 ))){ arctan(e(√((1+x)/(1−x))))−arctan((√((1+x)/(1−x))))} case 2 ((1−x)/(1−x))<0 ⇒∣x∣>1 ⇒f(x)=(2/(x+1)) ∫_1 ^e (du/(u^2 −((√((x−1)/(x+1))))^2 )) =_(u=(√((x−1)/(x+1)))z) (2/(x+1)) ∫_(√((x+1)/(x−1))) ^(e(√((x+1)/(x−1)))) ((x+1)/(x−1)) (1/(z^2 −1))(√((x−1)/(x+1)))dz =(1/(x−1))((√(x−1))/(√(x+1))) ∫_(√((x+1)/(x−1))) ^(e(√((x+1)/(x−1)))) {(1/(z−1))−(1/(z+1))}dz =(1/(√(x^2 −1)))[ln∣((z−1)/(z+1))∣]_(√((x+1)/(x−1))) ^(e(√((x+1)/(x−1)))) =(1/(√(x^2 −1))){ln∣((((e(√(x+1)))/(√(x−1)))−1)/(((e(√(x+1)))/(√(x−1)))+1))∣ −ln∣((((√(x+1))/(√(x−1)))−1)/(((√(x+1))/(√(x−1)))+1))∣} ⇒ f(x)=(1/(√(x^2 −1))){ln∣((e(√(x+1))−(√(x−1)))/(e(√(x+1))+(√(x−1))))∣−ln∣(((√(x+1))−(√(x−1)))/((√(x+1))+(√(x−1))))∣}](Q66246.png)
Commented by mathmax by abdo last updated on 11/Aug/19
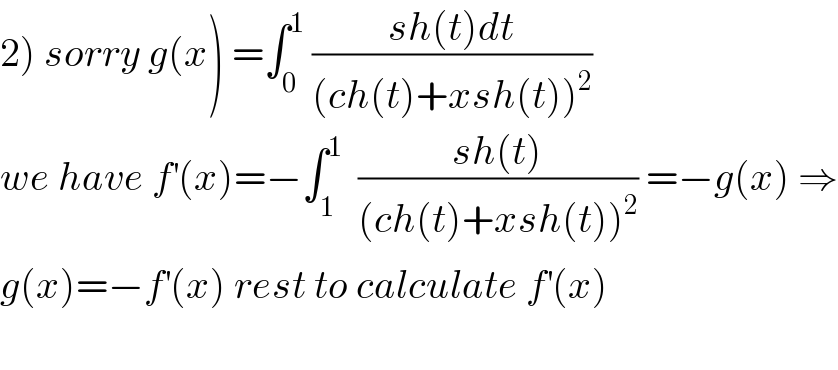
Commented by mathmax by abdo last updated on 11/Aug/19