
Question and Answers Forum
Question Number 66096 by sitangshu17 last updated on 09/Aug/19
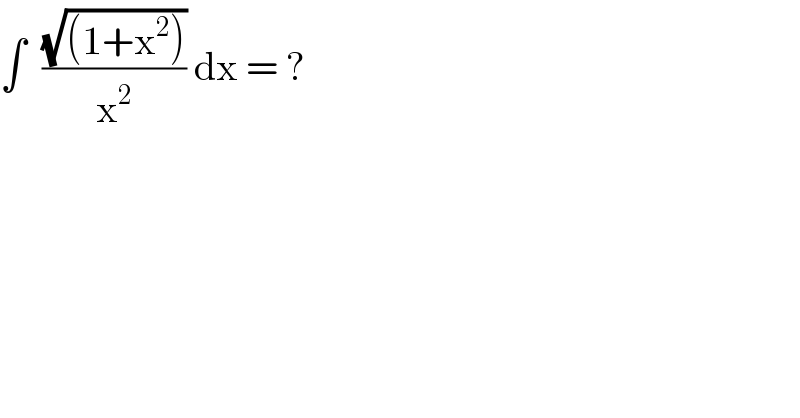
Commented by mathmax by abdo last updated on 09/Aug/19
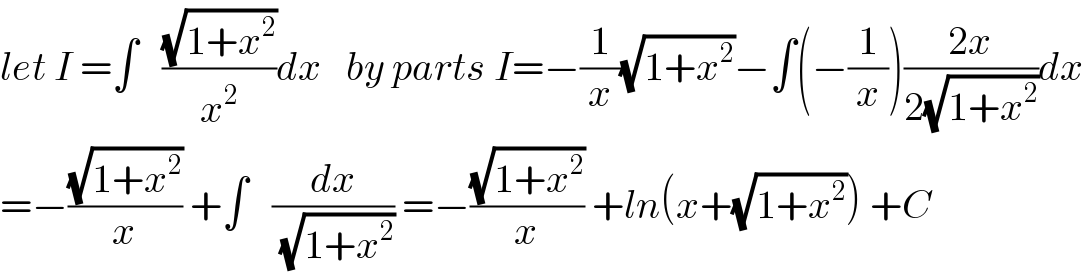
Commented by Prithwish sen last updated on 09/Aug/19
![put 1+x^2 =x^2 z^2 (dx/x) = ((zdz)/(1−z^2 )) ∫((√(1+x^2 ))/x^2 ) dx = ∫((xz)/x^2 )dx =∫((zdx)/x) = ∫(z^2 /(1−z^2 ))dz =∫[(1/(1−z^2 )) −1]dz = (1/2)ln∣((x+(√(1+x^2 )))/(x−(√(1+x^2 ))))∣−((√(1+x^2 ))/x) +C by putting z=((√(1+x^2 ))/x) please check](Q66133.png)
| ||
Question and Answers Forum | ||
Question Number 66096 by sitangshu17 last updated on 09/Aug/19 | ||
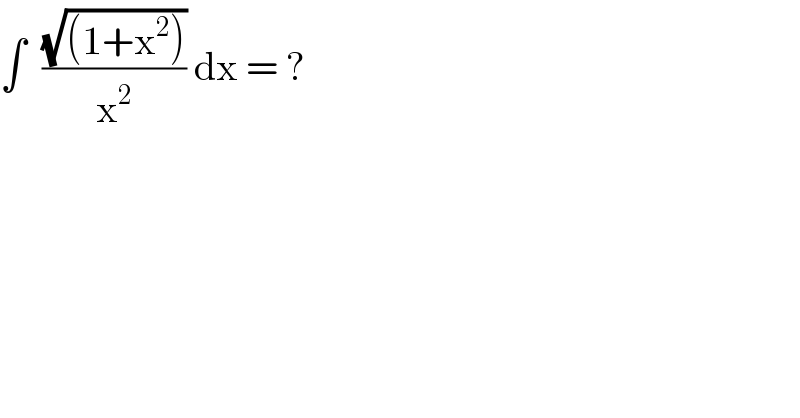 | ||
Commented by mathmax by abdo last updated on 09/Aug/19 | ||
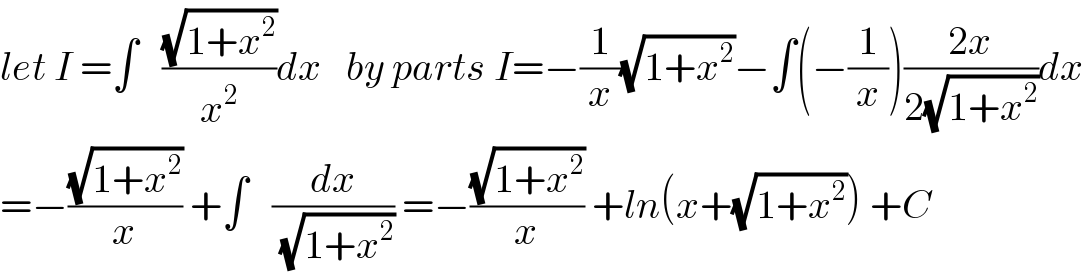 | ||
Commented by Prithwish sen last updated on 09/Aug/19 | ||
![put 1+x^2 =x^2 z^2 (dx/x) = ((zdz)/(1−z^2 )) ∫((√(1+x^2 ))/x^2 ) dx = ∫((xz)/x^2 )dx =∫((zdx)/x) = ∫(z^2 /(1−z^2 ))dz =∫[(1/(1−z^2 )) −1]dz = (1/2)ln∣((x+(√(1+x^2 )))/(x−(√(1+x^2 ))))∣−((√(1+x^2 ))/x) +C by putting z=((√(1+x^2 ))/x) please check](Q66133.png) | ||