
Question Number 66104 by Rio Michael last updated on 09/Aug/19
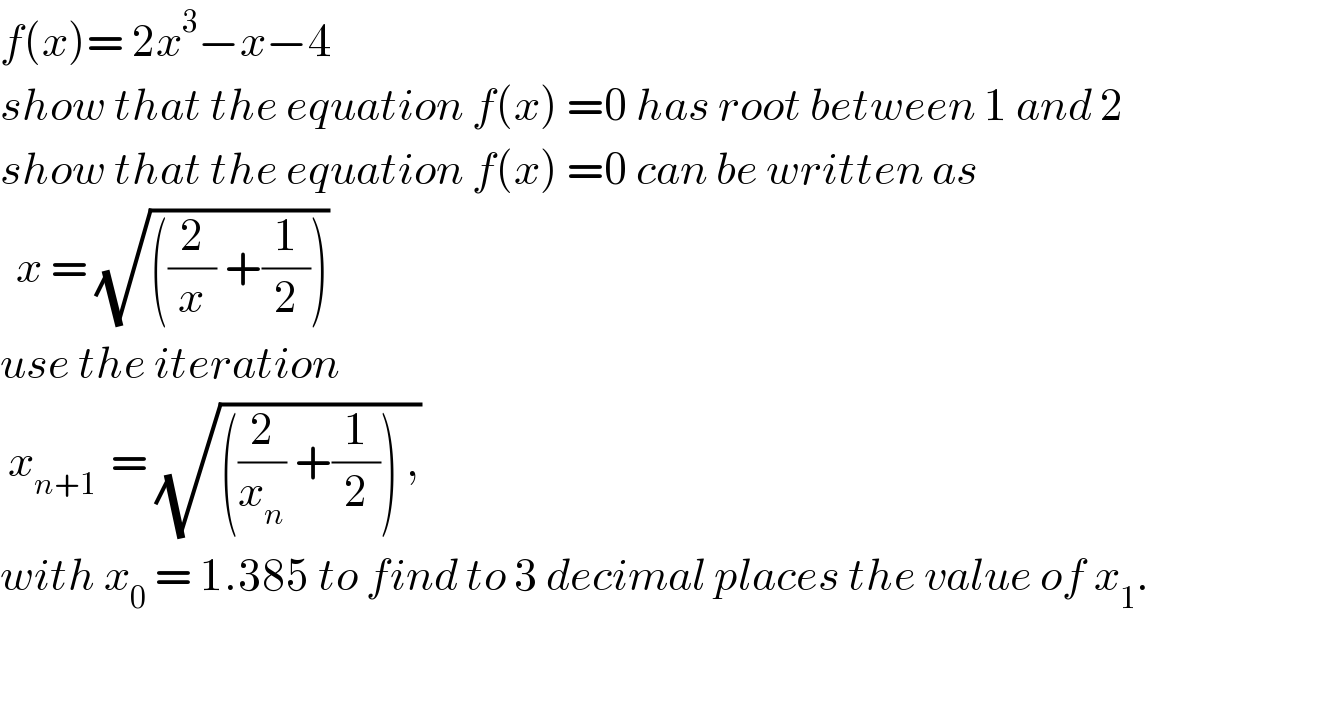
$${f}\left({x}\right)=\:\mathrm{2}{x}^{\mathrm{3}} −{x}−\mathrm{4} \\ $$$${show}\:{that}\:{the}\:{equation}\:{f}\left({x}\right)\:=\mathrm{0}\:{has}\:{root}\:{between}\:\mathrm{1}\:{and}\:\mathrm{2} \\ $$$${show}\:{that}\:{the}\:{equation}\:{f}\left({x}\right)\:=\mathrm{0}\:{can}\:{be}\:{written}\:{as}\: \\ $$$$\:\:{x}\:=\:\sqrt{\left(\frac{\mathrm{2}}{{x}}\:+\frac{\mathrm{1}}{\mathrm{2}}\right)} \\ $$$${use}\:{the}\:{iteration} \\ $$$$\:{x}_{{n}+\mathrm{1}\:} \:=\:\sqrt{\left(\frac{\mathrm{2}}{{x}_{{n}} }\:+\frac{\mathrm{1}}{\mathrm{2}}\right)\:,} \\ $$$${with}\:{x}_{\mathrm{0}} \:=\:\mathrm{1}.\mathrm{385}\:{to}\:{find}\:{to}\:\mathrm{3}\:{decimal}\:{places}\:{the}\:{value}\:{of}\:{x}_{\mathrm{1}} . \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 09/Aug/19
![we have f(x) =2x^3 −x−4 ⇒f^′ (x)=6x^2 −1 =((√6)x−1)((√6)x+1) f^′ (x)=0 ⇔x =+^− (1/(√6)) variation of f(x) x −∞ −(1/(√6)) (1/(√6)) +∞ f^′ (x) + − + f(x) −∞ incr. f(−(1/(√6))) decr f((1/(√6))) incr +∞ f is increasing on [(1/(√6)) ,+∞[ f(1) =2−1−4 =−5 f(2)=16−2−4 =10 ⇒f(1)f(2)<0 ⇒∃ α ∈]1,2[ /f(α)=0 x=(√((2/x)+(1/2)))and x>0 ⇒x^2 =((4+x)/(2x)) ⇒2x^3 =4+x ⇒2x^3 −x−4=0 ⇒ f(x)=0 so f(x)=0 and x>0 ⇔x=(√((2/x)+(1/2))) if we consider the iteration x_(n+1) =(√((2/x_n )+(1/2))) we get x_1 =(√((2/x_0 )+(1/2)))=(√((4+x_0 )/(2x_0 )))=(√((4+1,385)/(2×1,385))) rest to finish the calculus....](Q66167.png)
$${we}\:{have}\:{f}\left({x}\right)\:=\mathrm{2}{x}^{\mathrm{3}} −{x}−\mathrm{4}\:\Rightarrow{f}^{'} \left({x}\right)=\mathrm{6}{x}^{\mathrm{2}} −\mathrm{1}\:=\left(\sqrt{\mathrm{6}}{x}−\mathrm{1}\right)\left(\sqrt{\mathrm{6}}{x}+\mathrm{1}\right) \\ $$$${f}^{'} \left({x}\right)=\mathrm{0}\:\Leftrightarrow{x}\:=\overset{−} {+}\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\:\:\:{variation}\:{of}\:{f}\left({x}\right) \\ $$$${x}\:\:\:\:\:\:\:\:\:−\infty\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\infty \\ $$$${f}^{'} \left({x}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+ \\ $$$${f}\left({x}\right)\:\:\:\:\:\:\:\:\:−\infty\:\:\:\:\:{incr}.\:\:{f}\left(−\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\right)\:{decr}\:{f}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\right)\:\:\:\:{incr}\:\:+\infty \\ $$$${f}\:{is}\:{increasing}\:{on}\:\left[\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\:,+\infty\left[\right.\right. \\ $$$${f}\left(\mathrm{1}\right)\:=\mathrm{2}−\mathrm{1}−\mathrm{4}\:=−\mathrm{5} \\ $$$$\left.{f}\left(\mathrm{2}\right)=\mathrm{16}−\mathrm{2}−\mathrm{4}\:=\mathrm{10}\:\Rightarrow{f}\left(\mathrm{1}\right){f}\left(\mathrm{2}\right)<\mathrm{0}\:\Rightarrow\exists\:\alpha\:\in\right]\mathrm{1},\mathrm{2}\left[\:/{f}\left(\alpha\right)=\mathrm{0}\right. \\ $$$${x}=\sqrt{\frac{\mathrm{2}}{{x}}+\frac{\mathrm{1}}{\mathrm{2}}}{and}\:{x}>\mathrm{0}\:\Rightarrow{x}^{\mathrm{2}} =\frac{\mathrm{4}+{x}}{\mathrm{2}{x}}\:\Rightarrow\mathrm{2}{x}^{\mathrm{3}} =\mathrm{4}+{x}\:\Rightarrow\mathrm{2}{x}^{\mathrm{3}} −{x}−\mathrm{4}=\mathrm{0}\:\Rightarrow \\ $$$${f}\left({x}\right)=\mathrm{0}\:\:{so}\:\:\:{f}\left({x}\right)=\mathrm{0}\:{and}\:{x}>\mathrm{0}\:\Leftrightarrow{x}=\sqrt{\frac{\mathrm{2}}{{x}}+\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$${if}\:{we}\:{consider}\:{the}\:{iteration}\:{x}_{{n}+\mathrm{1}} =\sqrt{\frac{\mathrm{2}}{{x}_{{n}} }+\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$${we}\:{get}\:{x}_{\mathrm{1}} =\sqrt{\frac{\mathrm{2}}{{x}_{\mathrm{0}} }+\frac{\mathrm{1}}{\mathrm{2}}}=\sqrt{\frac{\mathrm{4}+{x}_{\mathrm{0}} }{\mathrm{2}{x}_{\mathrm{0}} }}=\sqrt{\frac{\mathrm{4}+\mathrm{1},\mathrm{385}}{\mathrm{2}×\mathrm{1},\mathrm{385}}} \\ $$$${rest}\:{to}\:{finish}\:{the}\:{calculus}.... \\ $$
