
Question and Answers Forum
Question Number 66866 by rajesh4661kumar@gmail.com last updated on 20/Aug/19
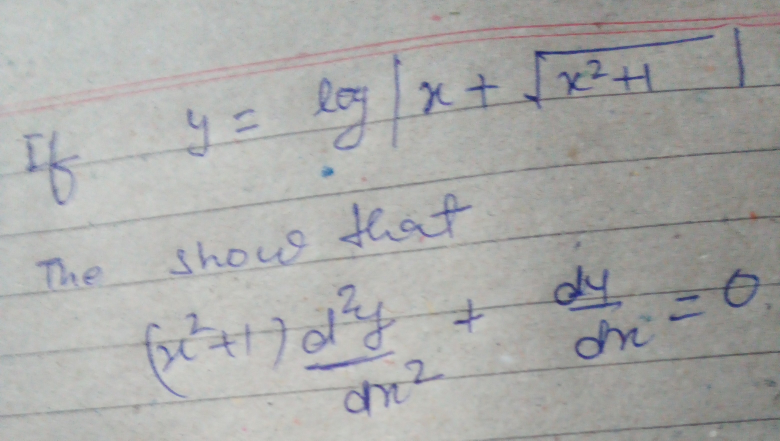
Commented by mathmax by abdo last updated on 20/Aug/19
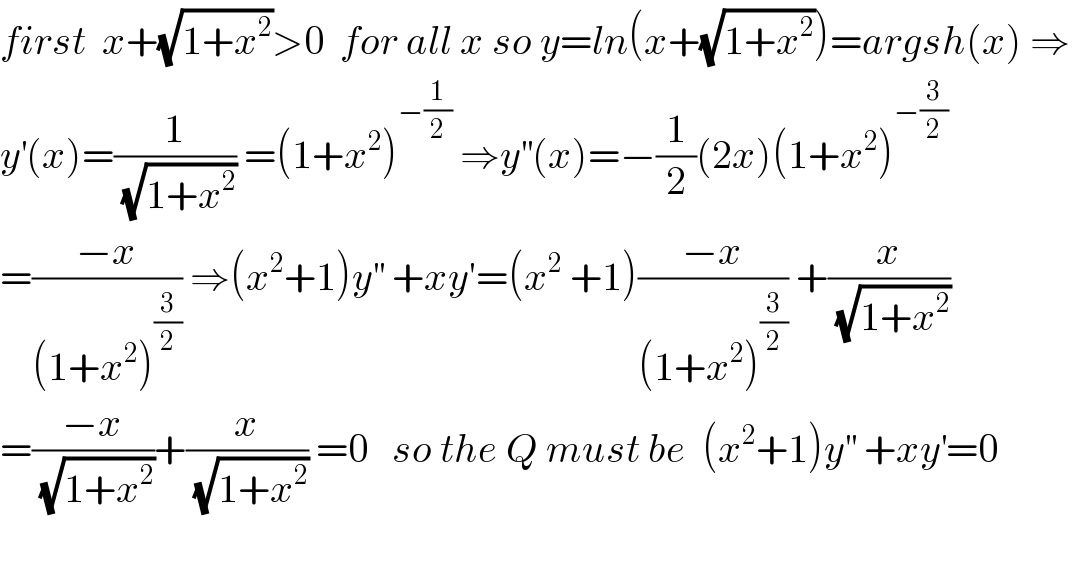
Answered by Kunal12588 last updated on 20/Aug/19
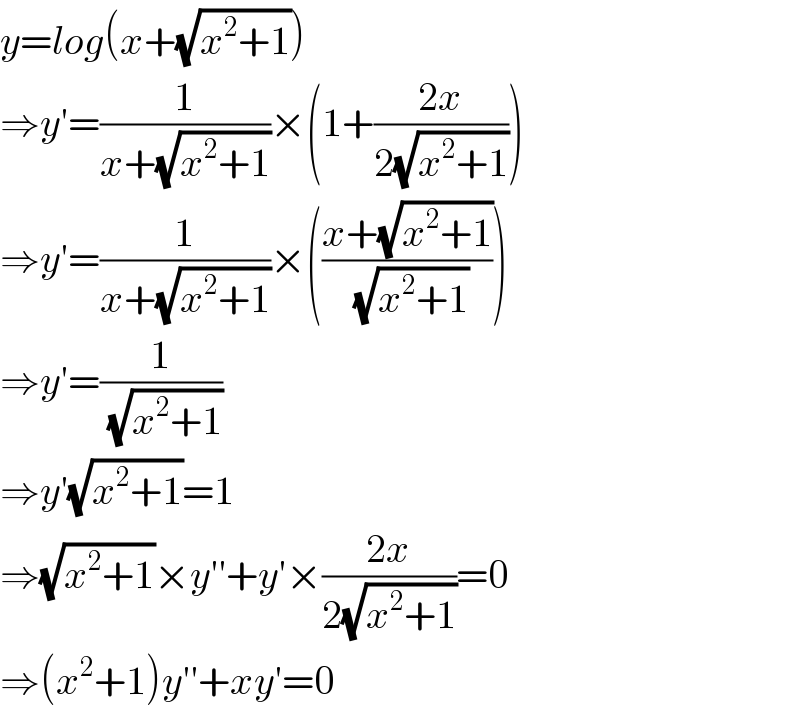
| ||
Question and Answers Forum | ||
Question Number 66866 by rajesh4661kumar@gmail.com last updated on 20/Aug/19 | ||
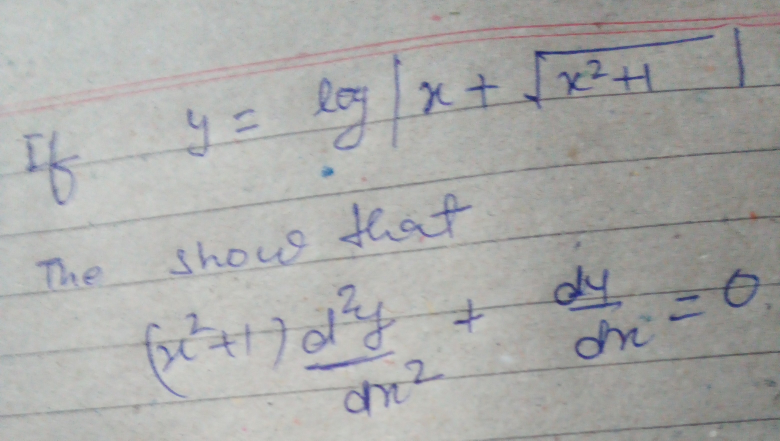 | ||
Commented by mathmax by abdo last updated on 20/Aug/19 | ||
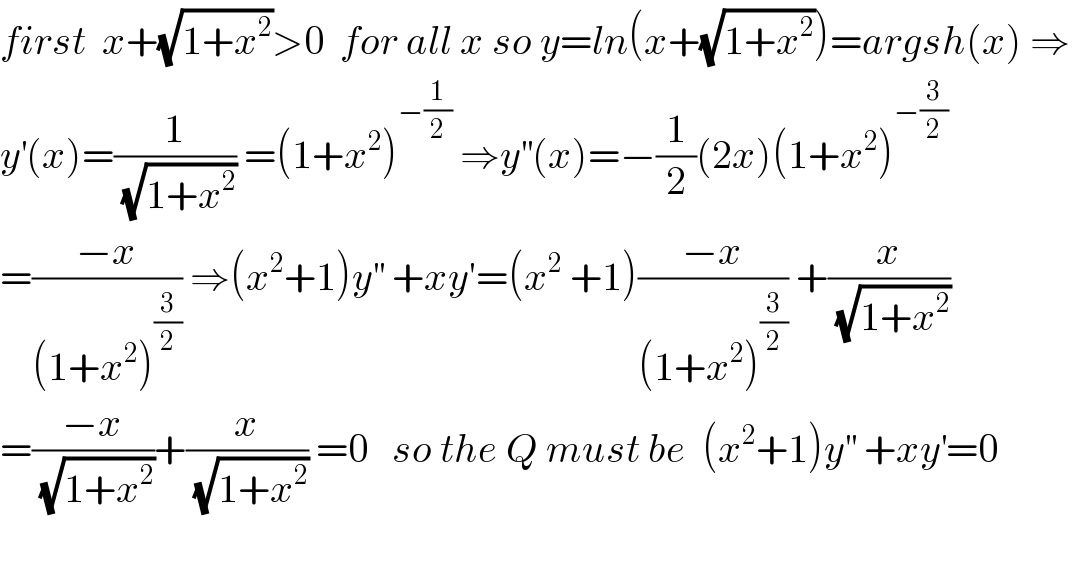 | ||
Answered by Kunal12588 last updated on 20/Aug/19 | ||
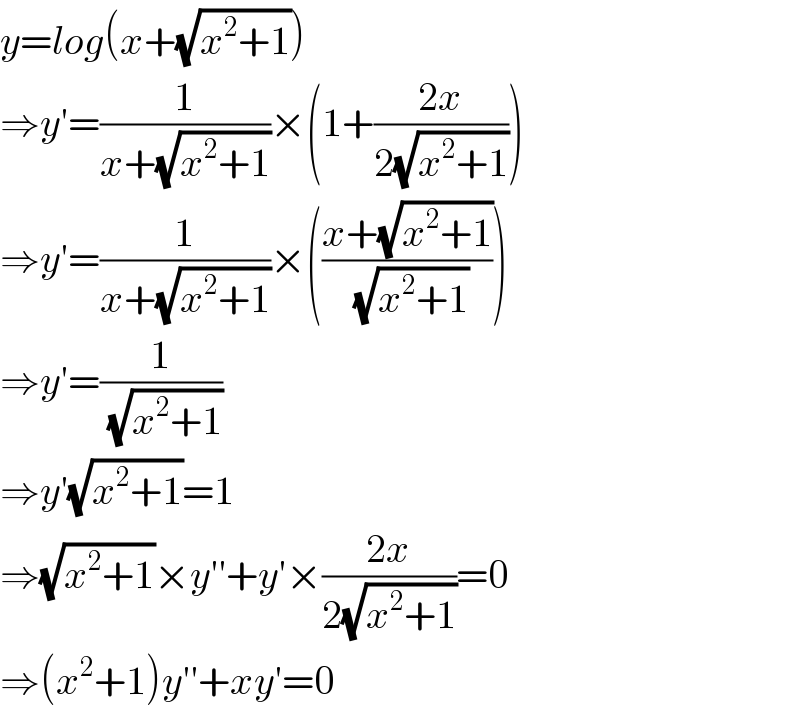 | ||
| ||