
Question Number 67310 by mathmax by abdo last updated on 25/Aug/19
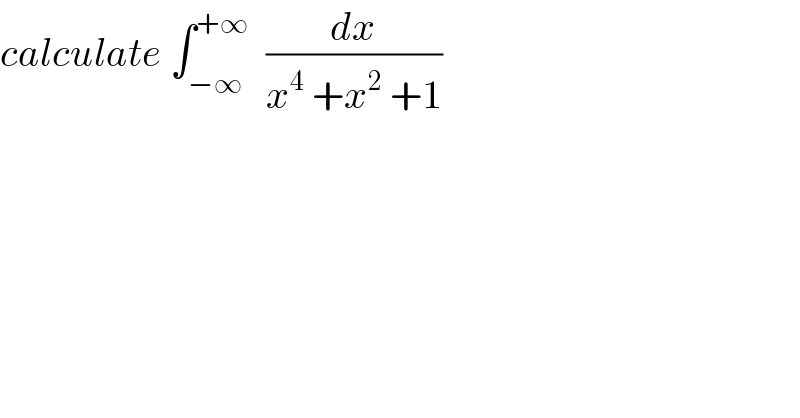
$${calculate}\:\int_{−\infty} ^{+\infty} \:\:\frac{{dx}}{{x}^{\mathrm{4}} \:+{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$
Commented by mathmax by abdo last updated on 25/Aug/19
![let I =∫_(−∞) ^(+∞) (dx/(x^4 +x^2 +1)) ⇒I =2 ∫_0 ^(+∞) (dx/(x^4 +x^2 +1)) we have x^4 +x^2 +1 =(x^2 +1)^2 −2x^2 +x^2 =(x^2 +1)^2 −x^2 =(x^2 −x+1)(x^2 +x+1) ⇒ I =2 ∫_0 ^(+∞) (dx/((x^2 +x+1)(x^2 −x+1))) let decompose F(x) =(1/((x^2 +x+1)(x^2 −x+1))) F(x) =((ax+b)/(x^2 +x +1)) +((cx+d)/(x^2 −x+1)) F(−x)=F(x) ⇒((−ax+b)/(x^2 −x+1)) +((−cx+d)/(x^2 +x+1)) =F(x) ⇒ c=−a and d=b ⇒F(x) =((ax+b)/(x^2 +x+1)) +((−ax+b)/(x^2 −x +1)) F(0) =1 =2b ⇒b =(1/2) F(1) =(1/3) =((a+b)/3) +b−a ⇒3 =a+b+3b−3a =−2a+2 ⇒1 =−2a ⇒ a=−(1/2) ⇒ F(x) =((−(1/2)x+(1/2))/(x^2 +x+1)) +(((1/2)x+(1/2))/(x^2 −x +1)) =(1/2){((−x+1)/(x^2 +x+1))+((x+1)/(x^2 −x +1))} ⇒∫_0 ^(+∞) F(x)dx =−(1/2) ∫_0 ^∞ ((x−1)/(x^2 +x+1))dx +(1/2)∫_0 ^∞ ((x+1)/(x^2 −x+1))dx =−(1/4)∫_0 ^∞ ((2x+1−3)/(x^2 +x+1))dx +(1/4)∫_0 ^∞ ((2x−1+3)/(x^2 −x +1))dx =(1/4)[ln∣((x^2 −x+1)/(x^2 +x+1))∣]_0 ^(+∞) +(3/4)∫_0 ^∞ (dx/(x^2 −x +1)) +(3/4)∫_0 ^∞ (dx/(x^2 +x+1)) =0 +(3/4) ∫_0 ^∞ (dx/(x^2 −x+1)) +(3/4)∫_0 ^∞ (dx/(x^2 +x +1)) ∫_0 ^∞ (dx/(x^2 −x +1)) =∫_0 ^∞ (dx/((x−(1/2))^2 +(3/4))) =_(x−(1/2)=((√3)/2)u) (4/3) ∫_(−(1/(√3))) ^(+∞) (1/(u^2 +1))((√3)/2)du =(2/(√3))[arctanu]_(−(1/(√3))) ^(+∞) =(2/(√3)){ (π/2) +arctan((1/(√3)))}=(2/(√3)){(π/2)+(π/6)} =(2/(√3)){((2π)/3)} =((4π)/(3(√3))) ∫_0 ^∞ (dx/(x^2 +x +1)) =∫_0 ^∞ (dx/((x+(1/2))^(2 ) +(3/4))) =_(x+(1/2)=((√3)/2)u) (4/3) ∫_(1/(√3)) ^∞ (1/(u^2 +1))((√3)/2)du =((2(√3))/3)[arctan(u)]_(1/(√3)) ^(+∞) =(2/(√3)){(π/2)−(π/6)}=(2/(√3)){(π/3)} =((2π)/(3(√3))) ⇒ ∫_0 ^∞ F(x)dx =(3/4)×((4π)/(3(√3))) +(3/4)×((2π)/(3(√3))) =(π/(√3)) +(π/(2(√3))) =(3/2)(π/(√3)) we have I =2 ∫_0 ^∞ F(x)dx =((3π)/(√3)) =π(√3)](Q67339.png)
$${let}\:{I}\:=\int_{−\infty} ^{+\infty} \:\frac{{dx}}{{x}^{\mathrm{4}} \:+{x}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow{I}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{+\infty} \:\frac{{dx}}{{x}^{\mathrm{4}} \:+{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${we}\:{have}\:{x}^{\mathrm{4}} \:\:+{x}^{\mathrm{2}} \:+\mathrm{1}\:=\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}{x}^{\mathrm{2}} \:+{x}^{\mathrm{2}} \:=\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} −{x}^{\mathrm{2}} \\ $$$$=\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)\:\Rightarrow\:{I}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{+\infty} \:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)} \\ $$$${let}\:{decompose}\:{F}\left({x}\right)\:=\frac{\mathrm{1}}{\left({x}^{\mathrm{2}} \:+{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)}\: \\ $$$${F}\left({x}\right)\:=\frac{{ax}+{b}}{{x}^{\mathrm{2}} \:+{x}\:+\mathrm{1}}\:+\frac{{cx}+{d}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}} \\ $$$${F}\left(−{x}\right)={F}\left({x}\right)\:\Rightarrow\frac{−{ax}+{b}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}\:+\frac{−{cx}+{d}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}}\:={F}\left({x}\right)\:\Rightarrow \\ $$$${c}=−{a}\:{and}\:{d}={b}\:\Rightarrow{F}\left({x}\right)\:=\frac{{ax}+{b}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}\:+\frac{−{ax}+{b}}{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{1}\:=\mathrm{2}{b}\:\Rightarrow{b}\:=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${F}\left(\mathrm{1}\right)\:=\frac{\mathrm{1}}{\mathrm{3}}\:=\frac{{a}+{b}}{\mathrm{3}}\:+{b}−{a}\:\Rightarrow\mathrm{3}\:={a}+{b}+\mathrm{3}{b}−\mathrm{3}{a}\:=−\mathrm{2}{a}+\mathrm{2}\:\Rightarrow\mathrm{1}\:=−\mathrm{2}{a}\:\Rightarrow \\ $$$${a}=−\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\:{F}\left({x}\right)\:=\frac{−\frac{\mathrm{1}}{\mathrm{2}}{x}+\frac{\mathrm{1}}{\mathrm{2}}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}\:+\frac{\frac{\mathrm{1}}{\mathrm{2}}{x}+\frac{\mathrm{1}}{\mathrm{2}}}{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\frac{−{x}+\mathrm{1}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}+\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}}\right\}\:\Rightarrow\int_{\mathrm{0}} ^{+\infty} {F}\left({x}\right){dx} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{x}−\mathrm{1}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}{dx}\:+\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}{dx} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{2}{x}+\mathrm{1}−\mathrm{3}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}{dx}\:+\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{2}{x}−\mathrm{1}+\mathrm{3}}{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\left[{ln}\mid\frac{{x}^{\mathrm{2}} −{x}+\mathrm{1}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}\mid\right]_{\mathrm{0}} ^{+\infty} \:+\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}}\:+\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}} \\ $$$$=\mathrm{0}\:+\frac{\mathrm{3}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}\:+\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\infty} \:\frac{{dx}}{{x}^{\mathrm{2}} \:+{x}\:+\mathrm{1}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}}\:=_{{x}−\frac{\mathrm{1}}{\mathrm{2}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{u}} \:\frac{\mathrm{4}}{\mathrm{3}}\:\:\int_{−\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{+\infty} \:\:\frac{\mathrm{1}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{du} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\left[{arctanu}\right]_{−\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{+\infty} \:=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\left\{\:\frac{\pi}{\mathrm{2}}\:+{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\right)\right\}=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\left\{\frac{\pi}{\mathrm{2}}+\frac{\pi}{\mathrm{6}}\right\} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\left\{\frac{\mathrm{2}\pi}{\mathrm{3}}\right\}\:=\frac{\mathrm{4}\pi}{\mathrm{3}\sqrt{\mathrm{3}}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+{x}\:+\mathrm{1}}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dx}}{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}\:} \:+\frac{\mathrm{3}}{\mathrm{4}}}\:=_{{x}+\frac{\mathrm{1}}{\mathrm{2}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{u}} \frac{\mathrm{4}}{\mathrm{3}}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\infty} \:\:\:\frac{\mathrm{1}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{du} \\ $$$$=\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}\left[{arctan}\left({u}\right)\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{+\infty} \:=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\left\{\frac{\pi}{\mathrm{2}}−\frac{\pi}{\mathrm{6}}\right\}=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\left\{\frac{\pi}{\mathrm{3}}\right\}\:=\frac{\mathrm{2}\pi}{\mathrm{3}\sqrt{\mathrm{3}}}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\infty} \:{F}\left({x}\right){dx}\:=\frac{\mathrm{3}}{\mathrm{4}}×\frac{\mathrm{4}\pi}{\mathrm{3}\sqrt{\mathrm{3}}}\:+\frac{\mathrm{3}}{\mathrm{4}}×\frac{\mathrm{2}\pi}{\mathrm{3}\sqrt{\mathrm{3}}}\:=\frac{\pi}{\sqrt{\mathrm{3}}}\:+\frac{\pi}{\mathrm{2}\sqrt{\mathrm{3}}}\:=\frac{\mathrm{3}}{\mathrm{2}}\frac{\pi}{\sqrt{\mathrm{3}}} \\ $$$${we}\:{have}\:{I}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:{F}\left({x}\right){dx}\:=\frac{\mathrm{3}\pi}{\sqrt{\mathrm{3}}}\:=\pi\sqrt{\mathrm{3}} \\ $$
Commented by MJS last updated on 26/Aug/19

$$\int\frac{{dx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}= \\ $$$$=\int\left(−\frac{\mathrm{2}{x}−\mathrm{1}}{\mathrm{4}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)}+\frac{\mathrm{1}}{\mathrm{4}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)}+\frac{\mathrm{2}{x}+\mathrm{1}}{\mathrm{4}\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}+\frac{\mathrm{1}}{\mathrm{4}\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}\right){dx}= \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\:\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{6}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{x}−\mathrm{1}\right)}{\mathrm{3}}\:+\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{6}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{x}+\mathrm{1}\right)}{\mathrm{3}}\:= \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\:\frac{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{6}}\left(\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{x}−\mathrm{1}\right)}{\mathrm{3}}\:+\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{x}+\mathrm{1}\right)}{\mathrm{3}}\right)\:+{C} \\ $$$$\underset{−\infty} {\overset{+\infty} {\int}}\frac{{dx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}=\frac{\pi\sqrt{\mathrm{3}}}{\mathrm{3}} \\ $$
Answered by Kunal12588 last updated on 25/Aug/19
![(1/2)∫((2/x^2 )/(x^2 +1+(1/x^2 )))dx=(1/2)∫((1+(1/x^2 ))/(x^2 +(1/x^2 )+1))dx−(1/2)∫((1−(1/x^2 ))/(x^2 +(1/x^2 )+1))dx (x+(1/x))=t⇒(1−(1/x^2 ))dx=dt (x−(1/x))=u⇒(1+(1/x^2 ))dx=du x^2 +(1/x^2 )+1=t^2 −1 and x^2 +(1/x^2 )+1=u^2 +3 I=(1/2)∫(du/(u^2 +3))−(1/2)∫(dt/(t^2 −1)) =(1/2)×(1/(√3))×tan^(−1) ((u/(√3)))−(1/2)×(1/2)ln∣((t−1)/(t+1))∣+C =(1/(2(√3))) tan^(−1) (((x^2 −1)/(x(√3))))−(1/4) ln∣((x^2 −x+1)/(x^2 +x+1))∣+C I_1 =∫_(−∞) ^(+∞) (dx/(x^4 +x^2 +1))=[I ]_(−∞) ^(+∞) lim_(x→+∞) I = (1/(2(√3))) lim_(x→+∞) arctan((x/(√3))−(1/(x(√3))))−(1/4) lim_(x→+∞) ln∣((1−(1/x)+(1/x^2 ))/(1+(1/x)+(1/x^2 )))∣ = (1/(2(√3))) lim_(x→+∞) arctan((x/(√3))−(1/(x(√3))))−(1/4) lim_(x→+∞) ln∣1∣ = (1/(2(√3)))×(π/2)−0=(π/(4(√3))) lim_(x→−∞) I= (1/(2(√3))) lim_(x→−∞) arctan((x/(√3))−(1/(x(√3))))−0 =(1/(2(√3)))×((−π)/2)=−(π/(4(√3))) I_1 =(π/(4(√3)))+(π/(4(√3)))=(π/(2(√3)))](Q67329.png)
$$\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\frac{\mathrm{2}}{{x}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{dx}=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}^{\mathrm{2}} }+\mathrm{1}}{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}−\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}^{\mathrm{2}} }+\mathrm{1}}{dx} \\ $$$$\left({x}+\frac{\mathrm{1}}{{x}}\right)={t}\Rightarrow\left(\mathrm{1}−\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\right){dx}={dt} \\ $$$$\left({x}−\frac{\mathrm{1}}{{x}}\right)={u}\Rightarrow\left(\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\right){dx}={du} \\ $$$${x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}^{\mathrm{2}} }+\mathrm{1}={t}^{\mathrm{2}} −\mathrm{1}\:{and}\:{x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}^{\mathrm{2}} }+\mathrm{1}={u}^{\mathrm{2}} +\mathrm{3} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{du}}{{u}^{\mathrm{2}} +\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dt}}{{t}^{\mathrm{2}} −\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}×{tan}^{−\mathrm{1}} \left(\frac{{u}}{\sqrt{\mathrm{3}}}\right)−\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{2}}{ln}\mid\frac{{t}−\mathrm{1}}{{t}+\mathrm{1}}\mid+{C} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:{tan}^{−\mathrm{1}} \left(\frac{{x}^{\mathrm{2}} −\mathrm{1}}{{x}\sqrt{\mathrm{3}}}\right)−\frac{\mathrm{1}}{\mathrm{4}}\:{ln}\mid\frac{{x}^{\mathrm{2}} −{x}+\mathrm{1}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}}\mid+{C} \\ $$$${I}_{\mathrm{1}} =\int_{−\infty} ^{+\infty} \:\:\frac{{dx}}{{x}^{\mathrm{4}} \:+{x}^{\mathrm{2}} \:+\mathrm{1}}=\left[{I}\:\right]_{−\infty} ^{+\infty} \\ $$$$\underset{{x}\rightarrow+\infty} {{lim}}\:{I}\:=\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\underset{{x}\rightarrow+\infty} {{lim}arctan}\left(\frac{{x}}{\sqrt{\mathrm{3}}}−\frac{\mathrm{1}}{{x}\sqrt{\mathrm{3}}}\right)−\frac{\mathrm{1}}{\mathrm{4}}\:\underset{{x}\rightarrow+\infty} {{lim}}\:{ln}\mid\frac{\mathrm{1}−\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{\mathrm{1}+\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}\mid \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\underset{{x}\rightarrow+\infty} {{lim}arctan}\left(\frac{{x}}{\sqrt{\mathrm{3}}}−\frac{\mathrm{1}}{{x}\sqrt{\mathrm{3}}}\right)−\frac{\mathrm{1}}{\mathrm{4}}\:\underset{{x}\rightarrow+\infty} {{lim}}\:{ln}\mid\mathrm{1}\mid \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}×\frac{\pi}{\mathrm{2}}−\mathrm{0}=\frac{\pi}{\mathrm{4}\sqrt{\mathrm{3}}} \\ $$$$\underset{{x}\rightarrow−\infty} {{lim}I}=\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\underset{{x}\rightarrow−\infty} {{lim}}\:{arctan}\left(\frac{{x}}{\sqrt{\mathrm{3}}}−\frac{\mathrm{1}}{{x}\sqrt{\mathrm{3}}}\right)−\mathrm{0} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}×\frac{−\pi}{\mathrm{2}}=−\frac{\pi}{\mathrm{4}\sqrt{\mathrm{3}}} \\ $$$${I}_{\mathrm{1}} =\frac{\pi}{\mathrm{4}\sqrt{\mathrm{3}}}+\frac{\pi}{\mathrm{4}\sqrt{\mathrm{3}}}=\frac{\pi}{\mathrm{2}\sqrt{\mathrm{3}}} \\ $$
Commented by mathmax by abdo last updated on 25/Aug/19

$${thanks}\:{a}\:{lots}\:{for}\:{this}\:{hard}\:{work}. \\ $$
Commented by Kunal12588 last updated on 26/Aug/19
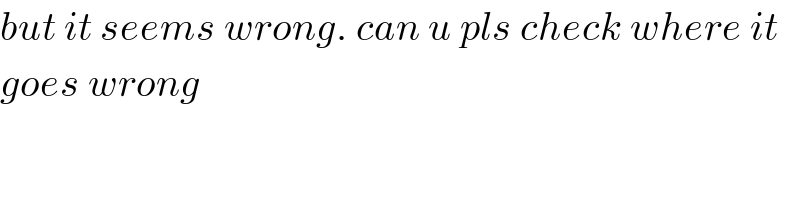
$${but}\:{it}\:{seems}\:{wrong}.\:{can}\:{u}\:{pls}\:{check}\:{where}\:{it} \\ $$$${goes}\:{wrong} \\ $$
Commented by mathmax by abdo last updated on 26/Aug/19
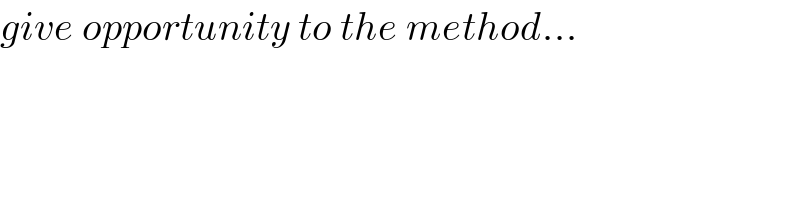
$${give}\:{opportunity}\:{to}\:{the}\:{method}... \\ $$
