
Question Number 68241 by mathmax by abdo last updated on 07/Sep/19
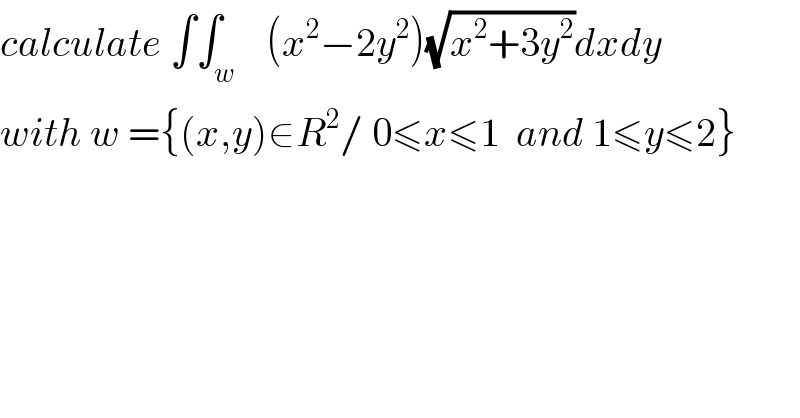
$${calculate}\:\int\int_{{w}} \:\:\:\left({x}^{\mathrm{2}} −\mathrm{2}{y}^{\mathrm{2}} \right)\sqrt{{x}^{\mathrm{2}} +\mathrm{3}{y}^{\mathrm{2}} }{dxdy} \\ $$$${with}\:{w}\:=\left\{\left({x},{y}\right)\in{R}^{\mathrm{2}} /\:\mathrm{0}\leqslant{x}\leqslant\mathrm{1}\:\:{and}\:\mathrm{1}\leqslant{y}\leqslant\mathrm{2}\right\} \\ $$
Commented by mathmax by abdo last updated on 10/Sep/19
![we use the diffeomorphism (r,θ)→ϕ(r,θ)=(x,y)=(rcosθ,(r/(√3))sinθ) =(ϕ_1 ,ϕ_2 ) we have 0≤x^2 ≤1 and 1≤y^2 ≤4 ⇒3≤3y^2 ≤12 ⇒ 3≤x^2 +3y^2 ≤13 ⇒(√3)≤(√(x^2 +3y^2 ))≤(√(13)) ⇒(√3)≤r≤(√(13)) M_j (ϕ) = ((((∂ϕ_1 /∂r) (∂ϕ_1 /∂θ))),(((∂ϕ_2 /∂r) (∂ϕ_2 /∂θ) )) ) = (((cosθ −rsinθ)),(((1/(√3))sinθ (r/(√3))cosθ)) ) ⇒det(M_j (ϕ))=(r/(√3))cos^2 θ+(r/(√3))sin^2 θ =(r/(√3)) ⇒∫∫_w (x^2 −2y^2 )(√(x^2 +3y^2 ))dxdy =∫∫_((√3)≤r≤(√(13))and 0≤θ≤(π/2)) (r^2 cos^2 θ−(2/3)r^2 sin^2 θ)r(r/(√3))drdθ =(1/(√3))∫_(√3) ^(√(13)) r^4 dr ∫_0 ^(π/2) ( cos^2 θ−(2/3)sin^2 θ)dθ we have ∫_(√3) ^(√(13)) r^4 dr =[(r^5 /5)]_(√3) ^(√(13)) =(1/5){((√(13)))^5 −((√3))^5 ∫_0 ^(π/2) (cos^2 θ−(2/3)sin^2 θ)dθ =(1/3)∫_0 ^(π/2) (3cos^2 θ−2sin^2 θ)dθ =(1/3) ∫_0 ^(π/2) (3((1+cos(2θ))/2)−2((1−cos(2θ))/2))dθ =(1/2)∫_0 ^(π/2) (1+cos(2θ))dθ−(1/3)∫_0 ^(π/2) (1−cos(2θ))dθ =(π/4) +(1/4)[sin(2θ)]_0 ^(π/2) −(π/6) +(1/6)[sin(2θ)]_0 ^(π/2) =(π/(12)) ⇒ ∫∫_w (x^2 −2y^2 )(√(x^2 +3y^2 ))dxdy =(1/(5(√3))){((√(13)))^5 −((√3))^5 }(π/(12)) =(π/(60(√3))){ ((√(13)))^5 −((√3))^5 }.](Q68408.png)
$${we}\:{use}\:{the}\:{diffeomorphism}\:\:\left({r},\theta\right)\rightarrow\varphi\left({r},\theta\right)=\left({x},{y}\right)=\left({rcos}\theta,\frac{{r}}{\sqrt{\mathrm{3}}}{sin}\theta\right) \\ $$$$=\left(\varphi_{\mathrm{1}} ,\varphi_{\mathrm{2}} \right)\:\:{we}\:{have}\:\:\mathrm{0}\leqslant{x}^{\mathrm{2}} \:\leqslant\mathrm{1}\:\:{and}\:\mathrm{1}\leqslant{y}^{\mathrm{2}} \leqslant\mathrm{4}\:\Rightarrow\mathrm{3}\leqslant\mathrm{3}{y}^{\mathrm{2}} \:\leqslant\mathrm{12}\:\Rightarrow \\ $$$$\mathrm{3}\leqslant{x}^{\mathrm{2}} \:+\mathrm{3}{y}^{\mathrm{2}} \leqslant\mathrm{13}\:\Rightarrow\sqrt{\mathrm{3}}\leqslant\sqrt{{x}^{\mathrm{2}} \:+\mathrm{3}{y}^{\mathrm{2}} }\leqslant\sqrt{\mathrm{13}}\:\Rightarrow\sqrt{\mathrm{3}}\leqslant{r}\leqslant\sqrt{\mathrm{13}} \\ $$$${M}_{{j}} \left(\varphi\right)\:=\begin{pmatrix}{\frac{\partial\varphi_{\mathrm{1}} }{\partial{r}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\partial\varphi_{\mathrm{1}} }{\partial\theta}}\\{\frac{\partial\varphi_{\mathrm{2}} }{\partial{r}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\partial\varphi_{\mathrm{2}} }{\partial\theta}\:\:\:\:\:\:}\end{pmatrix} \\ $$$$=\begin{pmatrix}{{cos}\theta\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−{rsin}\theta}\\{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{sin}\theta\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{{r}}{\sqrt{\mathrm{3}}}{cos}\theta}\end{pmatrix}\:\:\:\:\:\:\Rightarrow{det}\left({M}_{{j}} \left(\varphi\right)\right)=\frac{{r}}{\sqrt{\mathrm{3}}}{cos}^{\mathrm{2}} \theta+\frac{{r}}{\sqrt{\mathrm{3}}}{sin}^{\mathrm{2}} \theta \\ $$$$=\frac{{r}}{\sqrt{\mathrm{3}}}\:\Rightarrow\int\int_{{w}} \left({x}^{\mathrm{2}} −\mathrm{2}{y}^{\mathrm{2}} \right)\sqrt{{x}^{\mathrm{2}} \:+\mathrm{3}{y}^{\mathrm{2}} }{dxdy} \\ $$$$=\int\int_{\sqrt{\mathrm{3}}\leqslant{r}\leqslant\sqrt{\mathrm{13}}{and}\:\:\mathrm{0}\leqslant\theta\leqslant\frac{\pi}{\mathrm{2}}} \left({r}^{\mathrm{2}} {cos}^{\mathrm{2}} \theta−\frac{\mathrm{2}}{\mathrm{3}}{r}^{\mathrm{2}} {sin}^{\mathrm{2}} \theta\right){r}\frac{{r}}{\sqrt{\mathrm{3}}}{drd}\theta \\ $$$$=\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\int_{\sqrt{\mathrm{3}}} ^{\sqrt{\mathrm{13}}} {r}^{\mathrm{4}} {dr}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\:{cos}^{\mathrm{2}} \theta−\frac{\mathrm{2}}{\mathrm{3}}{sin}^{\mathrm{2}} \theta\right){d}\theta\:\:\:\:\:{we}\:{have} \\ $$$$\int_{\sqrt{\mathrm{3}}} ^{\sqrt{\mathrm{13}}} \:{r}^{\mathrm{4}} \:{dr}\:\:=\left[\frac{{r}^{\mathrm{5}} }{\mathrm{5}}\right]_{\sqrt{\mathrm{3}}} ^{\sqrt{\mathrm{13}}} =\frac{\mathrm{1}}{\mathrm{5}}\left\{\left(\sqrt{\mathrm{13}}\right)^{\mathrm{5}} −\left(\sqrt{\mathrm{3}}\right)^{\mathrm{5}} \right. \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({cos}^{\mathrm{2}} \theta−\frac{\mathrm{2}}{\mathrm{3}}{sin}^{\mathrm{2}} \theta\right){d}\theta\:=\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{3}{cos}^{\mathrm{2}} \theta−\mathrm{2}{sin}^{\mathrm{2}} \theta\right){d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{3}\frac{\mathrm{1}+{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}−\mathrm{2}\frac{\mathrm{1}−{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}\right){d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{1}+{cos}\left(\mathrm{2}\theta\right)\right){d}\theta−\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{1}−{cos}\left(\mathrm{2}\theta\right)\right){d}\theta \\ $$$$=\frac{\pi}{\mathrm{4}}\:+\frac{\mathrm{1}}{\mathrm{4}}\left[{sin}\left(\mathrm{2}\theta\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} −\frac{\pi}{\mathrm{6}}\:+\frac{\mathrm{1}}{\mathrm{6}}\left[{sin}\left(\mathrm{2}\theta\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \\ $$$$=\frac{\pi}{\mathrm{12}}\:\Rightarrow \\ $$$$\int\int_{{w}} \left({x}^{\mathrm{2}} −\mathrm{2}{y}^{\mathrm{2}} \right)\sqrt{{x}^{\mathrm{2}} +\mathrm{3}{y}^{\mathrm{2}} }{dxdy}\:=\frac{\mathrm{1}}{\mathrm{5}\sqrt{\mathrm{3}}}\left\{\left(\sqrt{\mathrm{13}}\right)^{\mathrm{5}} −\left(\sqrt{\mathrm{3}}\right)^{\mathrm{5}} \right\}\frac{\pi}{\mathrm{12}} \\ $$$$=\frac{\pi}{\mathrm{60}\sqrt{\mathrm{3}}}\left\{\:\left(\sqrt{\mathrm{13}}\right)^{\mathrm{5}} −\left(\sqrt{\mathrm{3}}\right)^{\mathrm{5}} \right\}. \\ $$$$ \\ $$
