Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 68350 by mhmd last updated on 09/Sep/19

Commented by MJS last updated on 09/Sep/19

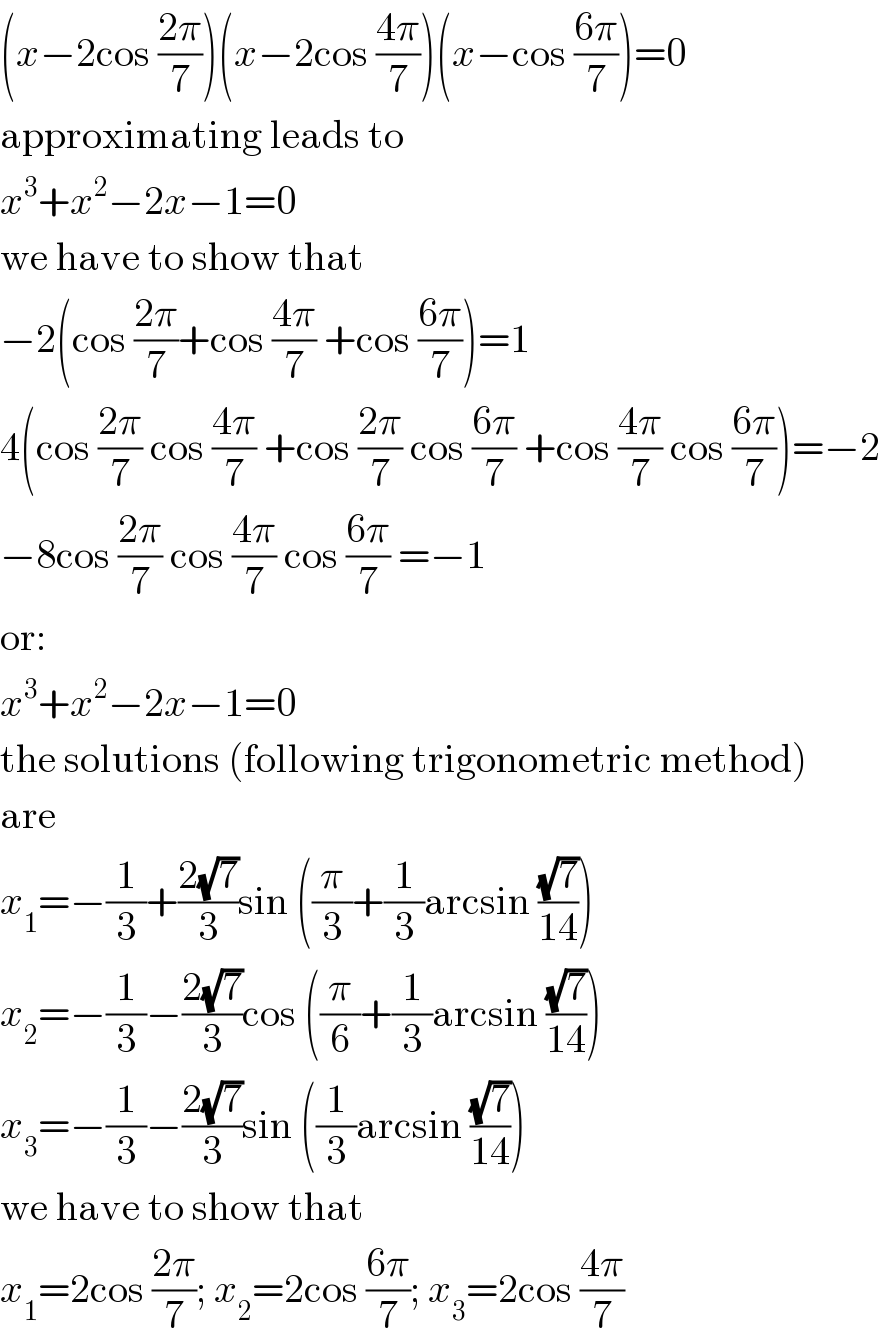
(x−2cos ((2π)/7))(x−2cos ((4π)/7))(x−cos ((6π)/7))=0 approximating leads to x^3 +x^2 −2x−1=0 we have to show that −2(cos ((2π)/7)+cos ((4π)/7) +cos ((6π)/7))=1 4(cos ((2π)/7) cos ((4π)/7) +cos ((2π)/7) cos ((6π)/7) +cos ((4π)/7) cos ((6π)/7))=−2 −8cos ((2π)/7) cos ((4π)/7) cos ((6π)/7) =−1 or: x^3 +x^2 −2x−1=0 the solutions (following trigonometric method) are x_1 =−(1/3)+((2(√7))/3)sin ((π/3)+(1/3)arcsin ((√7)/(14))) x_2 =−(1/3)−((2(√7))/3)cos ((π/6)+(1/3)arcsin ((√7)/(14))) x_3 =−(1/3)−((2(√7))/3)sin ((1/3)arcsin ((√7)/(14))) we have to show that x_1 =2cos ((2π)/7); x_2 =2cos ((6π)/7); x_3 =2cos ((4π)/7)

$$\left({x}−\mathrm{2cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}\right)\left({x}−\mathrm{2cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}\right)\left({x}−\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}}\right)=\mathrm{0} \\ $$$$\mathrm{approximating}\:\mathrm{leads}\:\mathrm{to} \\ $$$${x}^{\mathrm{3}} +{x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{to}\:\mathrm{show}\:\mathrm{that} \\ $$$$−\mathrm{2}\left(\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}\:+\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}}\right)=\mathrm{1} \\ $$$$\mathrm{4}\left(\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}\:\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}\:+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}\:\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}}\:+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}\:\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}}\right)=−\mathrm{2} \\ $$$$−\mathrm{8cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}}\:\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}}\:\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}}\:=−\mathrm{1} \\ $$$$\mathrm{or}: \\ $$$${x}^{\mathrm{3}} +{x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{the}\:\mathrm{solutions}\:\left(\mathrm{following}\:\mathrm{trigonometric}\:\mathrm{method}\right) \\ $$$$\mathrm{are} \\ $$$${x}_{\mathrm{1}} =−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right) \\ $$$${x}_{\mathrm{2}} =−\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{cos}\:\left(\frac{\pi}{\mathrm{6}}+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right) \\ $$$${x}_{\mathrm{3}} =−\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right) \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{to}\:\mathrm{show}\:\mathrm{that} \\ $$$${x}_{\mathrm{1}} =\mathrm{2cos}\:\frac{\mathrm{2}\pi}{\mathrm{7}};\:{x}_{\mathrm{2}} =\mathrm{2cos}\:\frac{\mathrm{6}\pi}{\mathrm{7}};\:{x}_{\mathrm{3}} =\mathrm{2cos}\:\frac{\mathrm{4}\pi}{\mathrm{7}} \\ $$

Commented by peter frank last updated on 09/Sep/19

thank you

$${thank}\:{you} \\ $$

Answered by mind is power last updated on 09/Sep/19

Z^7 −1=0 Z=e^((2ikπ)/7) .0≤k≤6 ⇒(z−1)(1+z+z^2 +z^3 +z^4 +z^5 +z^6 )=0 for k≥1 e^((2ikπ)/7) root of 1+z+z^2 +z^3 +z^4 +z^5 +z^6 =0 z≠0 ⇔(1/z^3 )(1+z+z^2 +z^3 +z^4 +z^5 +z^6 )=0 ⇒z^3 +(1/z^3 )+z^2 +(1/z^2 )+z+(1/z)+1=0..E z=e^((2ikπ)/7) solution of ..E z^3 +(1/z^3 )=(z+(1/z))^3 −3(z+(1/z)) z^2 +(1/z^2 )=(z+(1/z))^2 −2 ⇒(z+(1/z))^3 +((1/z)+z)^2 −2(z+(1/z))−1=0 z+(1/z)=2cos(((2kπ)/7)) ⇒2cos(((2kπ)/7)) root of X^3 +X^2 −2X−1=0 cos(((8π)/7))=cos(((6π)/7)) cos(((10π)/7))=cos(((4π)/7)) cos(((12π)/7))=cos(((2π)/7))..this shiw se can tak k≤3 k=1′2′3

$${Z}^{\mathrm{7}} −\mathrm{1}=\mathrm{0} \\ $$$${Z}={e}^{\frac{\mathrm{2}{ik}\pi}{\mathrm{7}}} .\mathrm{0}\leqslant{k}\leqslant\mathrm{6} \\ $$$$\Rightarrow\left({z}−\mathrm{1}\right)\left(\mathrm{1}+{z}+{z}^{\mathrm{2}} +{z}^{\mathrm{3}} +{z}^{\mathrm{4}} +{z}^{\mathrm{5}} +{z}^{\mathrm{6}} \right)=\mathrm{0} \\ $$$${for}\:{k}\geqslant\mathrm{1}\:{e}^{\frac{\mathrm{2}{ik}\pi}{\mathrm{7}}} {root}\:{of}\: \\ $$$$\mathrm{1}+{z}+{z}^{\mathrm{2}} +{z}^{\mathrm{3}} +{z}^{\mathrm{4}} +{z}^{\mathrm{5}} +{z}^{\mathrm{6}} =\mathrm{0} \\ $$$${z}\neq\mathrm{0} \\ $$$$\Leftrightarrow\frac{\mathrm{1}}{{z}^{\mathrm{3}} }\left(\mathrm{1}+{z}+{z}^{\mathrm{2}} +{z}^{\mathrm{3}} +{z}^{\mathrm{4}} +{z}^{\mathrm{5}} +{z}^{\mathrm{6}} \right)=\mathrm{0} \\ $$$$\Rightarrow{z}^{\mathrm{3}} +\frac{\mathrm{1}}{{z}^{\mathrm{3}} }+{z}^{\mathrm{2}} +\frac{\mathrm{1}}{{z}^{\mathrm{2}} }+{z}+\frac{\mathrm{1}}{{z}}+\mathrm{1}=\mathrm{0}..{E} \\ $$$${z}={e}^{\frac{\mathrm{2}{ik}\pi}{\mathrm{7}}} {solution}\:{of}\:..{E} \\ $$$${z}^{\mathrm{3}} +\frac{\mathrm{1}}{{z}^{\mathrm{3}} }=\left({z}+\frac{\mathrm{1}}{{z}}\right)^{\mathrm{3}} −\mathrm{3}\left({z}+\frac{\mathrm{1}}{{z}}\right) \\ $$$${z}^{\mathrm{2}} +\frac{\mathrm{1}}{{z}^{\mathrm{2}} }=\left({z}+\frac{\mathrm{1}}{{z}}\right)^{\mathrm{2}} −\mathrm{2} \\ $$$$\Rightarrow\left({z}+\frac{\mathrm{1}}{{z}}\right)^{\mathrm{3}} +\left(\frac{\mathrm{1}}{{z}}+{z}\right)^{\mathrm{2}} −\mathrm{2}\left({z}+\frac{\mathrm{1}}{{z}}\right)−\mathrm{1}=\mathrm{0} \\ $$$${z}+\frac{\mathrm{1}}{{z}}=\mathrm{2}{cos}\left(\frac{\mathrm{2}{k}\pi}{\mathrm{7}}\right) \\ $$$$\Rightarrow\mathrm{2}{cos}\left(\frac{\mathrm{2}{k}\pi}{\mathrm{7}}\right)\:{root}\:{of}\:{X}^{\mathrm{3}} +{X}^{\mathrm{2}} −\mathrm{2}{X}−\mathrm{1}=\mathrm{0} \\ $$$${cos}\left(\frac{\mathrm{8}\pi}{\mathrm{7}}\right)={cos}\left(\frac{\mathrm{6}\pi}{\mathrm{7}}\right) \\ $$$${cos}\left(\frac{\mathrm{10}\pi}{\mathrm{7}}\right)={cos}\left(\frac{\mathrm{4}\pi}{\mathrm{7}}\right) \\ $$$${cos}\left(\frac{\mathrm{12}\pi}{\mathrm{7}}\right)={cos}\left(\frac{\mathrm{2}\pi}{\mathrm{7}}\right)..{this}\:{shiw}\:{se}\:{can}\:{tak}\:{k}\leqslant\mathrm{3} \\ $$$${k}=\mathrm{1}'\mathrm{2}'\mathrm{3} \\ $$$$ \\ $$$$ \\ $$

Commented by MJS last updated on 09/Sep/19

great, thank you!

$$\mathrm{great},\:\mathrm{thank}\:\mathrm{you}! \\ $$

Commented by mind is power last updated on 09/Sep/19

y′re welcom

$${y}'{re}\:{welcom} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com