
Question and Answers Forum
Question Number 68487 by mr W last updated on 11/Sep/19
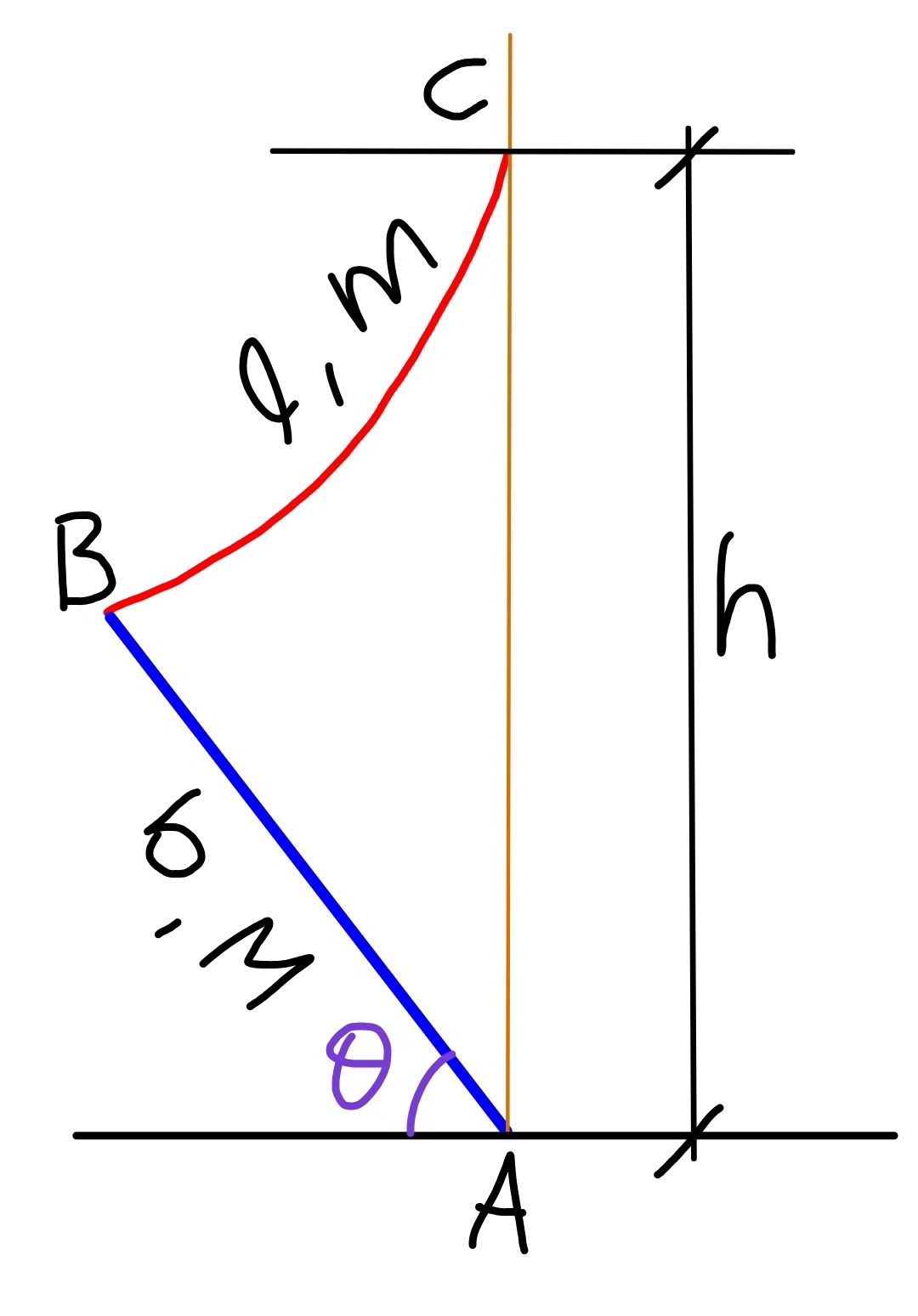
Commented by mr W last updated on 11/Sep/19
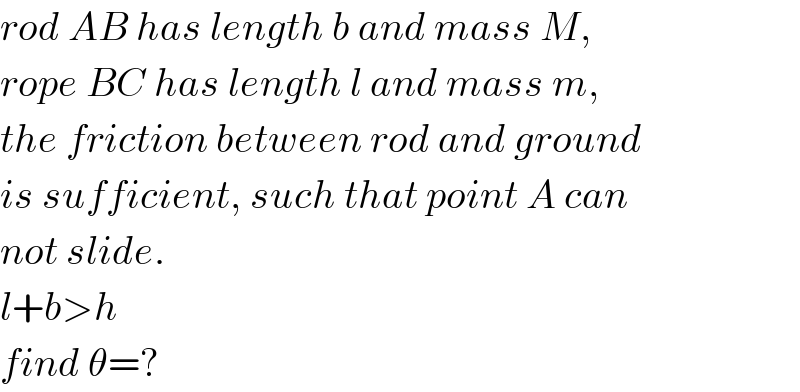
Commented by mr W last updated on 13/Sep/19
Answered by mr W last updated on 12/Sep/19
Commented by Prithwish sen last updated on 13/Sep/19

Commented by mr W last updated on 13/Sep/19
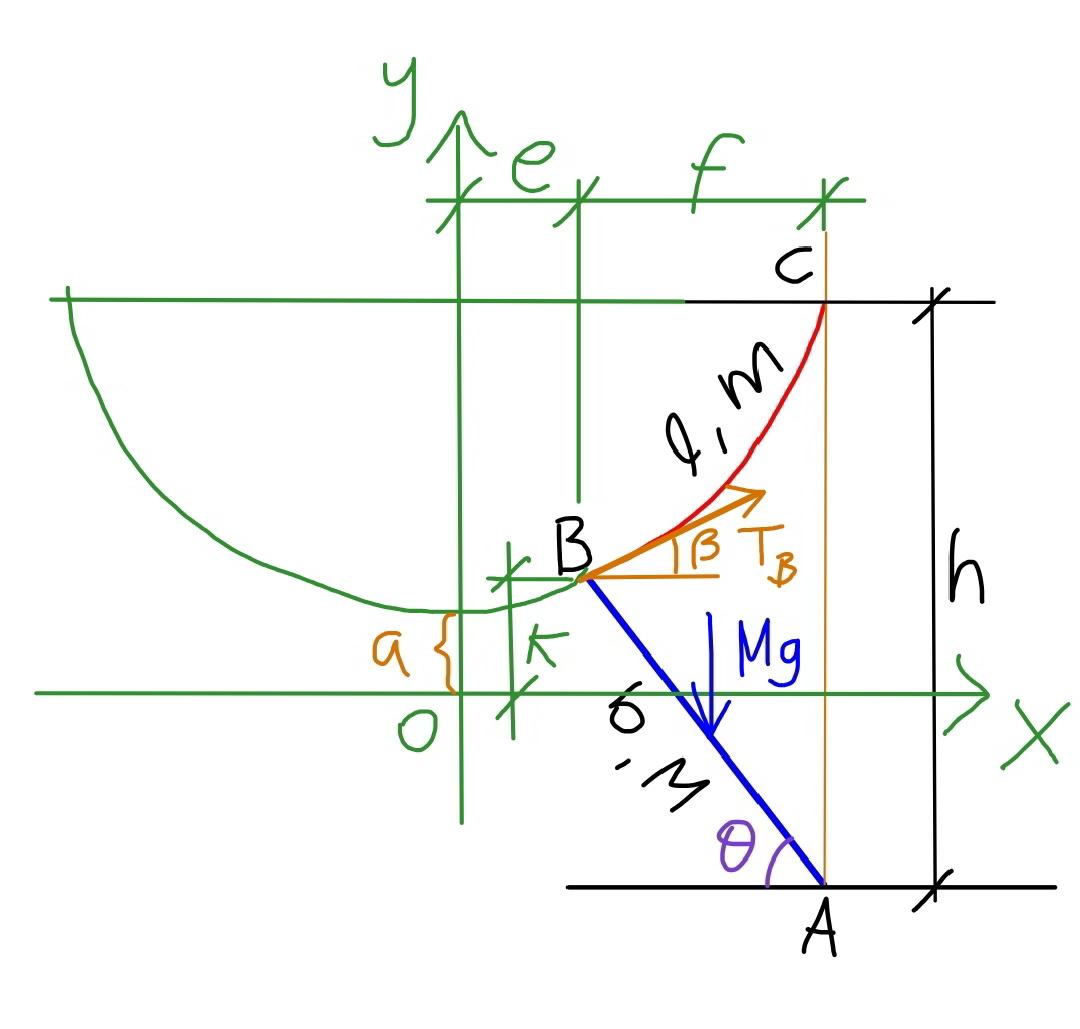
![mass of rope of unit length ρ=(m/l) T_0 =horizontal component of tension in rope let a=(T_0 /(ρg)) the rope BC is a part of a catenary with equation y=a cosh (x/a) in coordinate system as shown in the diagram above. T_B cos β=T_0 =aρg T_B b sin (θ+β)=((Mgb cos θ)/2) ((aρg)/(cos β))sin (θ+β)=((Mg cos θ)/2) ((am)/(l cos β))sin (θ+β)=((M cos θ)/2) ((a(sin θ cos β+cos θ sin β))/(cos β cos θ))=((Ml)/(2m)) ⇒tan θ+tan β=((μl)/a) with μ=(M/(2m)) at point B: k=a cosh (e/a) y′=tan β=sinh (e/a) at point C: f=b cos θ k+h−b sin θ=a cosh ((e+f)/a) a cosh (e/a)+h−b sin θ=a cosh ((e+f)/a) ⇒h−b sin θ=a(cosh ((e+f)/a)−cosh (e/a)) l=a(sinh ((e+f)/a)−sinh (e/a)) ⇒sinh ((e+f)/a)−sinh (e/a)=(l/a) ⇒tan θ+sinh (e/a)=((μl)/a) ⇒(e/a)=sinh^(−1) (((μl)/a)−tan θ) ⇒sinh ((e+f)/a)=((l(1+μ))/a)−tan θ ⇒((e+f)/a)=sinh^(−1) [((l(1+μ))/a)−tan θ] ⇒(e/a)+(f/a)=sinh^(−1) [((l(1+μ))/a)−tan θ] ⇒sinh^(−1) (((μl)/a)−tan θ)+((b cos θ)/a)=sinh^(−1) [((l(1+μ))/a)−tan θ] ⇒sinh^(−1) [((l(1+μ))/a)−tan θ]−sinh^(−1) (((μl)/a)−tan θ)=(l/a)×((b cos θ)/l) ⇒cosh {sinh^(−1) [((l(1+μ))/a)−tan θ]}−cosh {sinh^(−1) (((μl)/a)−tan θ)}=(l/a)×((h−b sin θ)/l) let λ=(l/a) ⇒sinh^(−1) [(1+μ)λ−tan θ]−sinh^(−1) (μλ−tan θ)=((λb cos θ)/l) ...(i) ⇒cosh {sinh^(−1) [(1+μ)λ−tan θ]}−cosh {sinh^(−1) (μλ−tan θ)}=((λ(h−b sin θ))/l) ...(ii) unknowns in (i) and (ii): λ and θ example: μ=(M/(2m))=2 l=2 b=3 h=4 ⇒θ=61.617° (θ=61.045° if rope were massless)](Q68520.png)
Commented by Prithwish sen last updated on 13/Sep/19
Commented by mr W last updated on 13/Sep/19
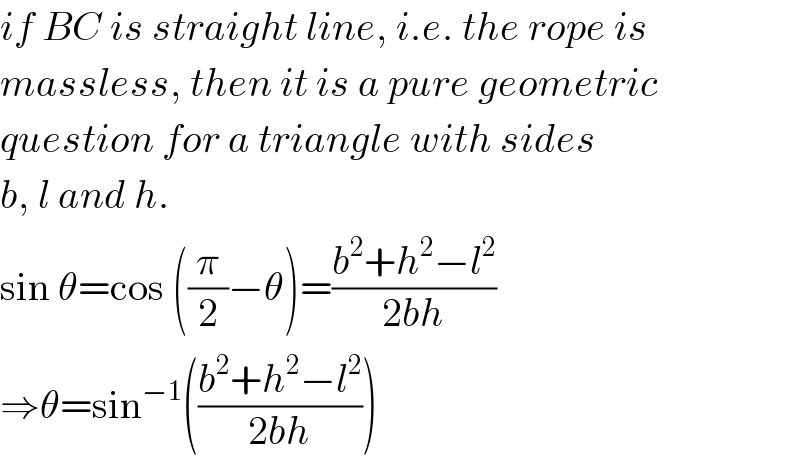
Commented by Prithwish sen last updated on 13/Sep/19

