Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 68666 by ahmadshah last updated on 14/Sep/19

Commented by mr W last updated on 14/Sep/19

Commented by mr W last updated on 14/Sep/19

α=55+25=80° x=α+40=80+40=120°

$$\alpha=\mathrm{55}+\mathrm{25}=\mathrm{80}° \\ $$$${x}=\alpha+\mathrm{40}=\mathrm{80}+\mathrm{40}=\mathrm{120}° \\ $$

Answered by ahmadshah last updated on 14/Sep/19

Commented by ahmadshah last updated on 14/Sep/19

$$ \\ $$

Commented by Prithwish sen last updated on 15/Sep/19

You have a creative mind.Thanks rasheed Sir.

$$\mathrm{You}\:\mathrm{have}\:\mathrm{a}\:\mathrm{creative}\:\mathrm{mind}.\mathrm{Thanks}\:\mathrm{rasheed}\:\mathrm{Sir}. \\ $$

Commented by Prithwish sen last updated on 16/Sep/19

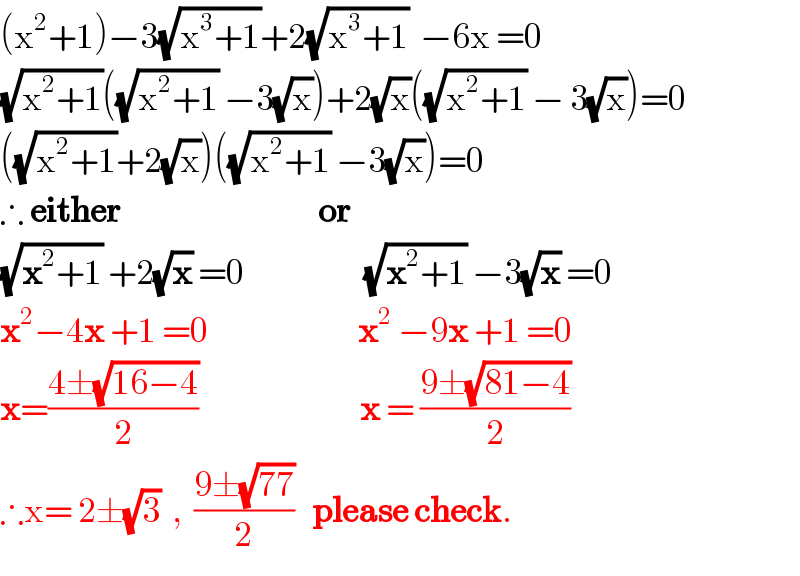
(x^2 +1)−3(√(x^3 +1))+2(√(x^3 +1)) −6x =0 (√(x^2 +1))((√(x^2 +1)) −3(√x))+2(√x)((√(x^2 +1)) − 3(√x))=0 ((√(x^2 +1))+2(√x))((√(x^2 +1)) −3(√x))=0 ∴ either or (√(x^2 +1)) +2(√x) =0 (√(x^2 +1)) −3(√x) =0 x^2 −4x +1 =0 x^2 −9x +1 =0 x=((4±(√(16−4)))/2) x = ((9±(√(81−4)))/2) ∴x= 2±(√3) , ((9±(√(77)))/2) please check.

$$\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)−\mathrm{3}\sqrt{\mathrm{x}^{\mathrm{3}} +\mathrm{1}}+\mathrm{2}\sqrt{\mathrm{x}^{\mathrm{3}} +\mathrm{1}}\:\:−\mathrm{6x}\:=\mathrm{0} \\ $$$$\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\:−\mathrm{3}\sqrt{\mathrm{x}}\right)+\mathrm{2}\sqrt{\mathrm{x}}\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\:−\:\mathrm{3}\sqrt{\mathrm{x}}\right)=\mathrm{0} \\ $$$$\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}+\mathrm{2}\sqrt{\mathrm{x}}\right)\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\:−\mathrm{3}\sqrt{\mathrm{x}}\right)=\mathrm{0} \\ $$$$\therefore\:\boldsymbol{\mathrm{either}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{or}} \\ $$$$\sqrt{\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\mathrm{1}}\:+\mathrm{2}\sqrt{\boldsymbol{\mathrm{x}}}\:=\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\sqrt{\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\mathrm{1}}\:−\mathrm{3}\sqrt{\boldsymbol{\mathrm{x}}}\:=\mathrm{0} \\ $$$$\boldsymbol{\mathrm{x}}^{\mathrm{2}} −\mathrm{4}\boldsymbol{\mathrm{x}}\:+\mathrm{1}\:=\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{x}}^{\mathrm{2}} \:−\mathrm{9}\boldsymbol{\mathrm{x}}\:+\mathrm{1}\:=\mathrm{0} \\ $$$$\boldsymbol{\mathrm{x}}=\frac{\mathrm{4}\pm\sqrt{\mathrm{16}−\mathrm{4}}}{\mathrm{2}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{x}}\:=\:\frac{\mathrm{9}\pm\sqrt{\mathrm{81}−\mathrm{4}}}{\mathrm{2}}\:\:\:\: \\ $$$$\therefore\mathrm{x}=\:\mathrm{2}\pm\sqrt{\mathrm{3}}\:\:,\:\:\frac{\mathrm{9}\pm\sqrt{\mathrm{77}}}{\mathrm{2}}\:\:\:\boldsymbol{\mathrm{please}}\:\boldsymbol{\mathrm{check}}. \\ $$

Commented by Rasheed.Sindhi last updated on 15/Sep/19

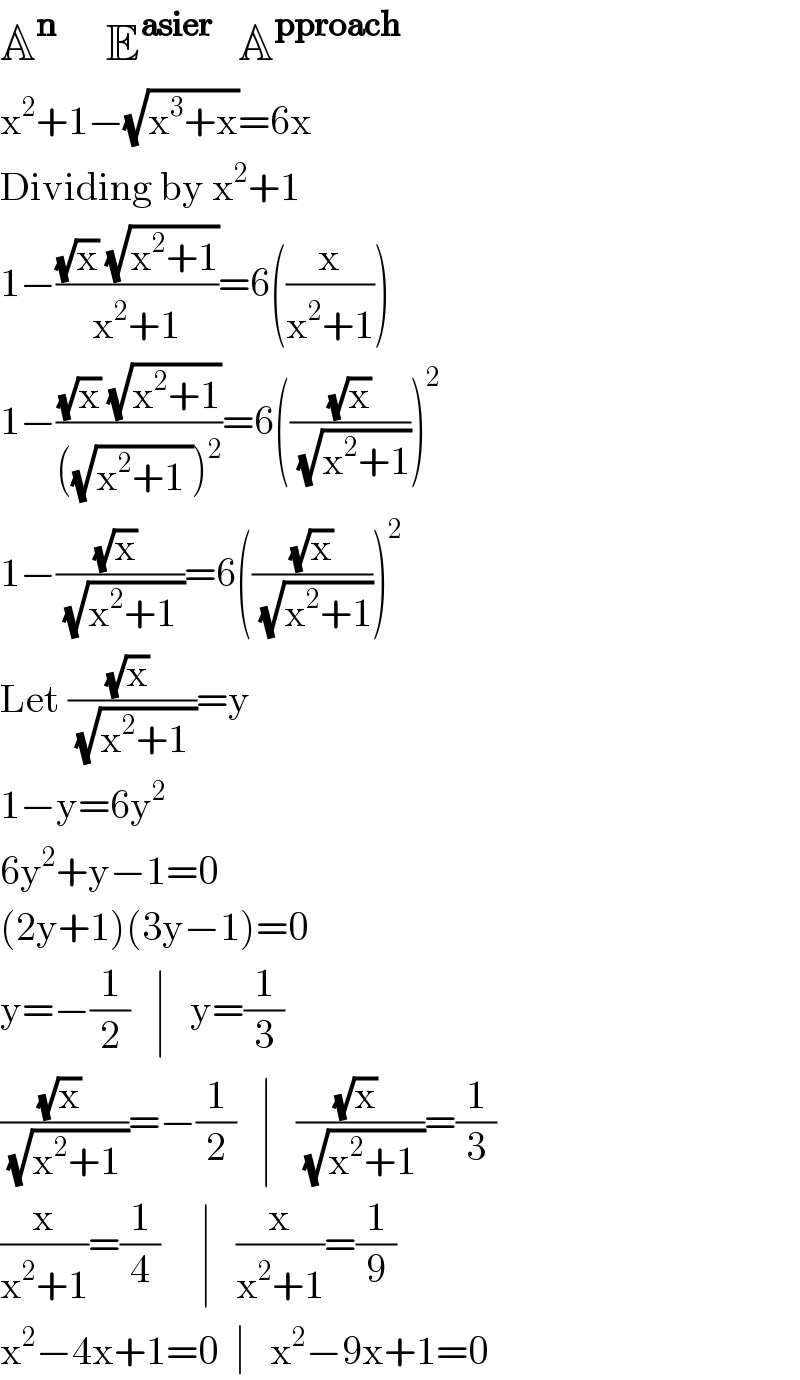
A^n E^(asier ) A^(pproach) x^2 +1−(√(x^3 +x))=6x Dividing by x^2 +1 1−(((√x) (√(x^2 +1)))/(x^2 +1))=6((x/(x^2 +1))) 1−(((√x) (√(x^2 +1)))/(((√(x^2 +1 )))^2 ))=6(((√x)/(√(x^2 +1))))^2 1−(((√x) )/(√(x^2 +1 )))=6(((√x)/(√(x^2 +1))))^2 Let (((√x) )/(√(x^2 +1 )))=y 1−y=6y^2 6y^2 +y−1=0 (2y+1)(3y−1)=0 y=−(1/2) ∣ y=(1/3) (((√x) )/(√(x^2 +1 )))=−(1/2) ∣ (((√x) )/(√(x^2 +1 )))=(1/3) (x/(x^2 +1))=(1/4) ∣ (x/(x^2 +1))=(1/9) x^2 −4x+1=0 ∣ x^2 −9x+1=0

$$\mathbb{A}^{\boldsymbol{\mathrm{n}}} \:\:\:\:\:\mathbb{E}^{\boldsymbol{\mathrm{asier}}\:} \:\:\mathbb{A}^{\boldsymbol{\mathrm{pproach}}} \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{1}−\sqrt{\mathrm{x}^{\mathrm{3}} +\mathrm{x}}=\mathrm{6x} \\ $$$$\mathrm{Dividing}\:\mathrm{by}\:\mathrm{x}^{\mathrm{2}} +\mathrm{1} \\ $$$$\mathrm{1}−\frac{\sqrt{\mathrm{x}}\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}=\mathrm{6}\left(\frac{\mathrm{x}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$$\mathrm{1}−\frac{\sqrt{\mathrm{x}}\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}}{\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}\:}\right)^{\mathrm{2}} }=\mathrm{6}\left(\frac{\sqrt{\mathrm{x}}}{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}}\right)^{\mathrm{2}} \\ $$$$\mathrm{1}−\frac{\sqrt{\mathrm{x}}\:}{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}\:}}=\mathrm{6}\left(\frac{\sqrt{\mathrm{x}}}{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}}\right)^{\mathrm{2}} \\ $$$$\mathrm{Let}\:\frac{\sqrt{\mathrm{x}}\:}{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}\:}}=\mathrm{y} \\ $$$$\mathrm{1}−\mathrm{y}=\mathrm{6y}^{\mathrm{2}} \\ $$$$\mathrm{6y}^{\mathrm{2}} +\mathrm{y}−\mathrm{1}=\mathrm{0} \\ $$$$\left(\mathrm{2y}+\mathrm{1}\right)\left(\mathrm{3y}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\mathrm{y}=−\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\mid\:\:\:\mathrm{y}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\frac{\sqrt{\mathrm{x}}\:}{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}\:}}=−\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\mid\:\:\:\frac{\sqrt{\mathrm{x}}\:}{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}\:}}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\frac{\mathrm{x}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}=\frac{\mathrm{1}}{\mathrm{4}}\:\:\:\:\:\mid\:\:\:\frac{\mathrm{x}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}=\frac{\mathrm{1}}{\mathrm{9}} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{4x}+\mathrm{1}=\mathrm{0}\:\:\mid\:\:\:\mathrm{x}^{\mathrm{2}} −\mathrm{9x}+\mathrm{1}=\mathrm{0} \\ $$

Commented by Prithwish sen last updated on 15/Sep/19

Excellent Sir.

$$\mathrm{Excellent}\:\mathrm{Sir}. \\ $$

Commented by Rasheed.Sindhi last updated on 15/Sep/19

Sir, actually this solution raised in my mind after seeing your solution. I like your solution!

$$\boldsymbol{\mathrm{Sir}},\:\mathrm{actually}\:\mathrm{this}\:\mathrm{solution}\:\mathrm{raised}\:\mathrm{in} \\ $$$$\mathrm{my}\:\mathrm{mind}\:\mathrm{after}\:\mathrm{seeing}\:\mathrm{your}\:\mathrm{solution}. \\ $$$$\mathrm{I}\:\mathrm{like}\:\mathrm{your}\:\mathrm{solution}! \\ $$

Commented by mind is power last updated on 15/Sep/19

x=tg(t)..x≥0⇒t∈[0,(π/2)] ⇒(1/(cos^2 (t)))−(√((sin(t))/(cos^3 (t))))=6((sin(t))/(cos(t))) ⇒1−(√(sin(t)cos(t)))=6sin(t)cos(t) ⇒1−(√((sin(2t))/2))=3sin(2t) ⇒(√(sin(2t) )) solution of 1−(X/(√2))=3X^2

$${x}={tg}\left({t}\right)..{x}\geqslant\mathrm{0}\Rightarrow{t}\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right] \\ $$$$\Rightarrow\frac{\mathrm{1}}{{cos}^{\mathrm{2}} \left({t}\right)}−\sqrt{\frac{{sin}\left({t}\right)}{{cos}^{\mathrm{3}} \left({t}\right)}}=\mathrm{6}\frac{{sin}\left({t}\right)}{{cos}\left({t}\right)} \\ $$$$ \\ $$$$\Rightarrow\mathrm{1}−\sqrt{{sin}\left({t}\right){cos}\left({t}\right)}=\mathrm{6}{sin}\left({t}\right){cos}\left({t}\right) \\ $$$$\Rightarrow\mathrm{1}−\sqrt{\frac{{sin}\left(\mathrm{2}{t}\right)}{\mathrm{2}}}=\mathrm{3}{sin}\left(\mathrm{2}{t}\right) \\ $$$$\Rightarrow\sqrt{{sin}\left(\mathrm{2}{t}\right)\:}\:\:{solution}\:{of}\:\mathrm{1}−\frac{{X}}{\sqrt{\mathrm{2}}}=\mathrm{3}{X}^{\mathrm{2}} \\ $$

Commented by Rasheed.Sindhi last updated on 15/Sep/19

Sir mind-is-power,Nice idea of connecting the algebraic problem to trigonometry!

$${Sir}\:{mind}-{is}-{power},{Nice}\:{idea}\:{of} \\ $$$${connecting}\:{the}\:{algebraic}\:{problem} \\ $$$${to}\:{trigonom}\mathrm{etry}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com