Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 69593 by ahmadshahhimat775@gmail.com last updated on 25/Sep/19

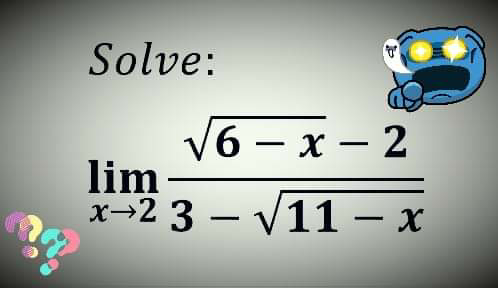
Commented by mathmax by abdo last updated on 25/Sep/19

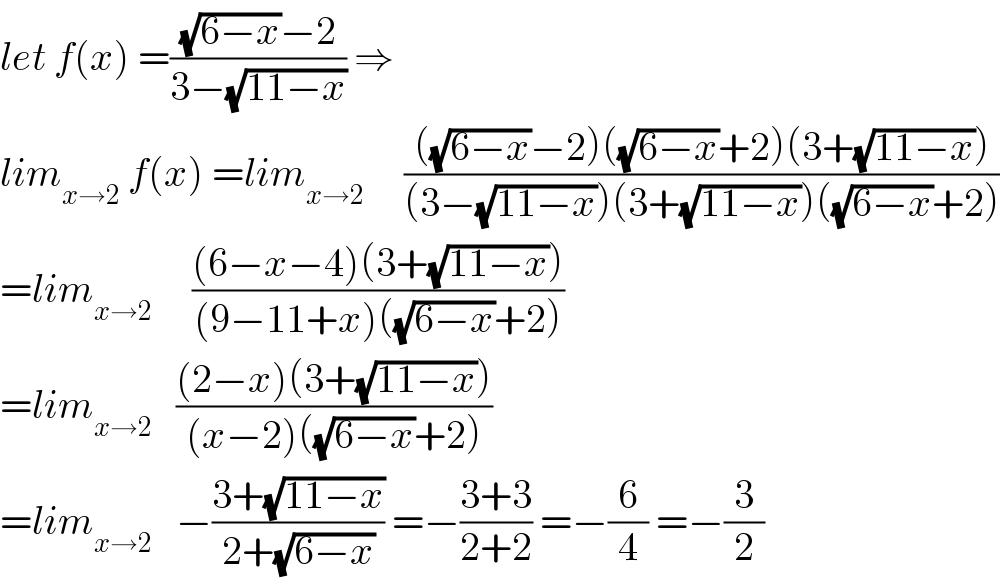
let f(x) =(((√(6−x))−2)/(3−(√(11−x)))) ⇒ lim_(x→2) f(x) =lim_(x→2) ((((√(6−x))−2)((√(6−x))+2)(3+(√(11−x))))/((3−(√(11−x)))(3+(√(11−x)))((√(6−x))+2))) =lim_(x→2) (((6−x−4)(3+(√(11−x))))/((9−11+x)((√(6−x))+2))) =lim_(x→2) (((2−x)(3+(√(11−x))))/((x−2)((√(6−x))+2))) =lim_(x→2) −((3+(√(11−x)))/(2+(√(6−x)))) =−((3+3)/(2+2)) =−(6/4) =−(3/2)

$${let}\:{f}\left({x}\right)\:=\frac{\sqrt{\mathrm{6}−{x}}−\mathrm{2}}{\mathrm{3}−\sqrt{\mathrm{11}−{x}}}\:\Rightarrow \\ $$$${lim}_{{x}\rightarrow\mathrm{2}} \:{f}\left({x}\right)\:={lim}_{{x}\rightarrow\mathrm{2}} \:\:\:\:\:\frac{\left(\sqrt{\mathrm{6}−{x}}−\mathrm{2}\right)\left(\sqrt{\mathrm{6}−{x}}+\mathrm{2}\right)\left(\mathrm{3}+\sqrt{\mathrm{11}−{x}}\right)}{\left(\mathrm{3}−\sqrt{\mathrm{11}−{x}}\right)\left(\mathrm{3}+\sqrt{\mathrm{11}−{x}}\right)\left(\sqrt{\mathrm{6}−{x}}+\mathrm{2}\right)} \\ $$$$={lim}_{{x}\rightarrow\mathrm{2}} \:\:\:\:\:\frac{\left(\mathrm{6}−{x}−\mathrm{4}\right)\left(\mathrm{3}+\sqrt{\mathrm{11}−{x}}\right)}{\left(\mathrm{9}−\mathrm{11}+{x}\right)\left(\sqrt{\mathrm{6}−{x}}+\mathrm{2}\right)} \\ $$$$={lim}_{{x}\rightarrow\mathrm{2}} \:\:\:\frac{\left(\mathrm{2}−{x}\right)\left(\mathrm{3}+\sqrt{\mathrm{11}−{x}}\right)}{\left({x}−\mathrm{2}\right)\left(\sqrt{\mathrm{6}−{x}}+\mathrm{2}\right)}\: \\ $$$$={lim}_{{x}\rightarrow\mathrm{2}} \:\:\:−\frac{\mathrm{3}+\sqrt{\mathrm{11}−{x}}}{\mathrm{2}+\sqrt{\mathrm{6}−{x}}}\:=−\frac{\mathrm{3}+\mathrm{3}}{\mathrm{2}+\mathrm{2}}\:=−\frac{\mathrm{6}}{\mathrm{4}}\:=−\frac{\mathrm{3}}{\mathrm{2}} \\ $$

Commented by Rasheed.Sindhi last updated on 26/Sep/19

V nice!

$${V}\:{nice}! \\ $$

Answered by MJS last updated on 25/Sep/19

lim_(x→2) (((√(6−x))−2)/(3−(√(11−x))))=lim_(x→2) (((d/dx)[(√(6−x))−2])/((d/dx)[3−(√(11−x))]))= =−lim_(x→2) ((√(11−x))/(√(6−x)))=−(3/2)

$$\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\frac{\sqrt{\mathrm{6}−{x}}−\mathrm{2}}{\mathrm{3}−\sqrt{\mathrm{11}−{x}}}=\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\frac{\frac{{d}}{{dx}}\left[\sqrt{\mathrm{6}−{x}}−\mathrm{2}\right]}{\frac{{d}}{{dx}}\left[\mathrm{3}−\sqrt{\mathrm{11}−{x}}\right]}= \\ $$$$=−\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\frac{\sqrt{\mathrm{11}−{x}}}{\sqrt{\mathrm{6}−{x}}}=−\frac{\mathrm{3}}{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com