
Question and Answers Forum
Question Number 70167 by Kunal12588 last updated on 01/Oct/19
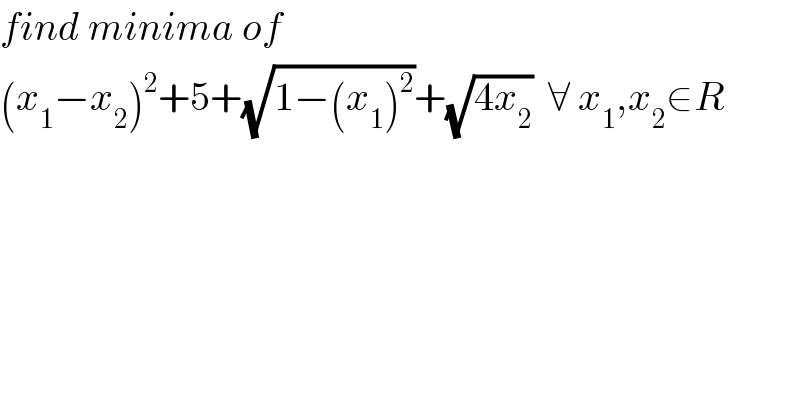
Commented by MJS last updated on 02/Oct/19

| ||
Question and Answers Forum | ||
Question Number 70167 by Kunal12588 last updated on 01/Oct/19 | ||
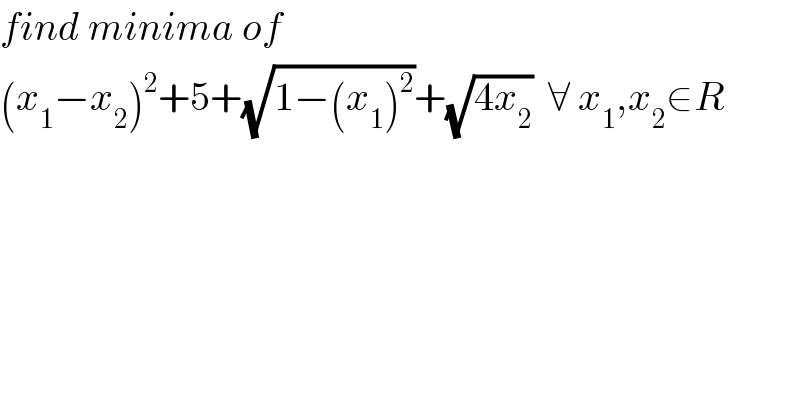 | ||
Commented by MJS last updated on 02/Oct/19 | ||
 | ||