Question Number 71421 by ajfour last updated on 15/Oct/19
Commented by ajfour last updated on 15/Oct/19
$${Find}\:{speed}\:{of}\:{sphere}\:{as}\:{it}\:{rolls} \\ $$$${down}\:{the}\:{wedge},\:{and}\:{is}\:{about}\:{to} \\ $$$${touch}\:{the}\:{ground}.\:{Also}\:{find}\:{the} \\ $$$${least}\:{coefficient}\:{of}\:{friction}, \\ $$$${so}\:{that}\:{sphere}\:{comes}\:{rolling}\:{all} \\ $$$${the}\:{way};\:{wedge}\:{isn}'{t}\:{fixed}, \\ $$$${assume}\:{that}\:{ground}\:{is}\:{smooth}. \\ $$
Answered by mr W last updated on 16/Oct/19
Commented by mr W last updated on 19/Oct/19
$${v}=\frac{{ds}}{{dt}}=\omega{r} \\ $$$${A}={r}\alpha \\ $$$$\alpha=\frac{{d}\omega}{{dt}}=\frac{{d}\omega}{{ds}}×\frac{{ds}}{{dt}}=\frac{{v}}{{r}}×\frac{{dv}}{{ds}} \\ $$$${I}=\frac{\mathrm{2}{mr}^{\mathrm{2}} }{\mathrm{5}} \\ $$$${I}\alpha={rf} \\ $$$$\alpha=\frac{{rf}}{{I}}=\frac{\mathrm{5}{f}}{\mathrm{2}{mr}} \\ $$$$ \\ $$$${Ma}=−{f}\:\mathrm{cos}\:\theta+{N}\:\mathrm{sin}\:\theta \\ $$$${a}=\frac{−{f}\:\mathrm{cos}\:\theta+{N}\:\mathrm{sin}\:\theta}{{M}} \\ $$$$ \\ $$$${f}={mg}\:\mathrm{sin}\:\theta+{ma}\:\mathrm{cos}\:\theta−{mr}\alpha \\ $$$$\frac{\mathrm{7}}{\mathrm{2}}{f}={mg}\:\mathrm{sin}\:\theta+{ma}\:\mathrm{cos}\:\theta \\ $$$${N}={mg}\:\mathrm{cos}\:\theta−{ma}\:\mathrm{sin}\:\theta \\ $$$$\frac{\mathrm{7}}{\mathrm{2}}{f}={mg}\:\mathrm{sin}\:\theta+\frac{{m}}{{M}}\left(−{f}\:\mathrm{cos}\:\theta+{N}\:\mathrm{sin}\:\theta\right)\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow\left(\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\:\mathrm{cos}^{\mathrm{2}} \:\theta\right){f}−\frac{{m}}{{M}}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\:{N}={mg}\:\mathrm{sin}\:\theta \\ $$$${N}={mg}\:\mathrm{cos}\:\theta−\frac{{m}}{{M}}\left(−{f}\:\mathrm{cos}\:\theta+{N}\:\mathrm{sin}\:\theta\right)\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow−\frac{{m}}{{M}}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\:{f}+\left(\mathrm{1}+\frac{{m}}{{M}}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right){N}={mg}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{f}=\frac{{mg}\:\mathrm{sin}\:\theta\:\left(\mathrm{1}+\frac{{m}}{{M}}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)−{mg}\:\mathrm{cos}\:\theta\:\left(−\frac{{m}}{{M}}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\right)}{\left(\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\:\mathrm{cos}^{\mathrm{2}} \:\theta\right)\left(\mathrm{1}+\frac{{m}}{{M}}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)−\left(−\frac{{m}}{{M}}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} } \\ $$$$\Rightarrow{f}=\frac{{mg}\:\mathrm{sin}\:\theta\:\left(\mathrm{1}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)} \\ $$$$ \\ $$$$\Rightarrow{N}=\frac{{mg}\:\mathrm{cos}\:\theta\:\left(\frac{\mathrm{7}}{\mathrm{2}}−\frac{{m}}{{M}}\:\mathrm{cos}^{\mathrm{2}} \:\theta\right)−{mg}\:\mathrm{sin}\:\theta\:\left(−\frac{{m}}{{M}}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\right)}{\left(\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\:\mathrm{cos}^{\mathrm{2}} \:\theta\right)\left(\mathrm{1}+\frac{{m}}{{M}}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)−\left(−\frac{{m}}{{M}}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} } \\ $$$$\Rightarrow{N}=\frac{{mg}\:\mathrm{cos}\:\theta\left(\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)} \\ $$$${f}\leqslant\mu{N} \\ $$$$\frac{{mg}\:\mathrm{sin}\:\theta\:\left(\mathrm{1}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)}\leqslant\mu\frac{{mg}\:\mathrm{cos}\:\theta\left(\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)} \\ $$$$\Rightarrow\mu\geqslant\frac{\mathrm{1}+\frac{{m}}{{M}}}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}}×\mathrm{tan}\:\theta \\ $$$$\Rightarrow\alpha=\frac{\mathrm{5}{f}}{\mathrm{2}{mr}}=\frac{\mathrm{5}{g}}{\mathrm{2}{r}}×\frac{\mathrm{sin}\:\theta\left(\mathrm{1}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)} \\ $$$$\Rightarrow\frac{{v}}{{r}}×\frac{{dv}}{{ds}}=\frac{\mathrm{5}{g}}{\mathrm{2}{r}}×\frac{\mathrm{sin}\:\theta\left(\mathrm{1}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)} \\ $$$$\Rightarrow{vdv}=\frac{\frac{\mathrm{5}}{\mathrm{2}}{g}\:\mathrm{sin}\:\theta\left(\mathrm{1}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)}{ds}=\lambda{ds} \\ $$$${with}\:\lambda=\frac{\frac{\mathrm{5}}{\mathrm{2}}{g}\:\mathrm{sin}\:\theta\left(\mathrm{1}+\frac{{m}}{{M}}\right)}{\frac{\mathrm{7}}{\mathrm{2}}+\frac{{m}}{{M}}\left(\frac{\mathrm{5}}{\mathrm{2}}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{5}{g}\:\mathrm{sin}\:\theta}{\frac{\mathrm{5}{M}+\mathrm{2}{M}+\mathrm{2}{m}}{{M}+{m}}+\frac{\mathrm{5}{m}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \:\theta\right)}{{M}+{m}}} \\ $$$$=\frac{{g}\:\mathrm{sin}\:\theta}{\frac{\mathrm{7}}{\mathrm{5}}−\frac{{m}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{{M}+{m}}} \\ $$$$\Rightarrow\frac{{v}^{\mathrm{2}} }{\mathrm{2}}=\lambda{s} \\ $$$$\Rightarrow{v}=\sqrt{\mathrm{2}\lambda{s}}=\sqrt{\frac{\mathrm{2}{gs}\:\mathrm{sin}\:\theta}{\frac{\mathrm{7}}{\mathrm{5}}−\frac{{m}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{{M}+{m}}}} \\ $$$${when}\:{the}\:{ball}\:{is}\:{about}\:{to}\:{touch}\:{the} \\ $$$${ground},\:{s}=\frac{{h}}{\mathrm{sin}\:\theta}−\frac{{r}}{\mathrm{tan}\:\frac{\theta}{\mathrm{2}}} \\ $$$$\Rightarrow{v}=\sqrt{\frac{\mathrm{2}{g}\left({h}−\mathrm{2}{r}\:\mathrm{cos}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}\right)\:}{\frac{\mathrm{7}}{\mathrm{5}}−\frac{{m}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{{M}+{m}}}} \\ $$
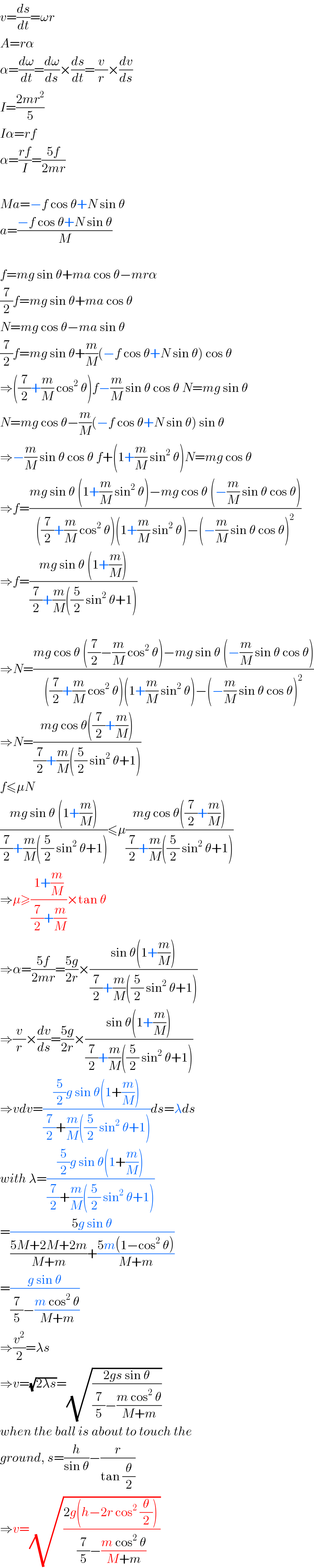
Commented by ajfour last updated on 18/Oct/19
Commented by ajfour last updated on 18/Oct/19
$$\:\:\:\:{s}=\frac{{h}}{\mathrm{sin}\:\theta}−{r}\mathrm{tan}\:\frac{\theta}{\mathrm{2}} \\ $$
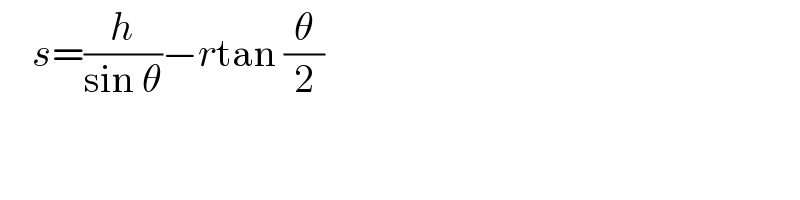
Commented by ajfour last updated on 18/Oct/19
![mgssin θ=((MV^( 2) )/2)+(1/2)(((2mr^2 )/5))(v^2 /r^2 ) +(m/2)[(vcos θ−V)^2 +v^2 sin^2 θ] & mvcos θ=(M+m)V ⇒ V=((mvcos θ)/(M+m)) & vcos θ−V = ((Mvcos θ)/(M+m)) substituting in first eq. mgssin θ=mv^2 ((1/5)+((sin^2 θ)/2)) +(M/2)(((mvcos θ)/(M+m)))^2 +(m/2)(((Mvcos θ)/(M+m)))^2 ⇒ mgssin θ=mv^2 ((1/5)+((sin^2 θ)/2)) +((Mmv^2 cos^2 θ)/(2(M+m))) ⇒ v^2 =((gssin θ)/((1/5)+((sin^2 θ)/2)+((Mcos^2 θ)/(2(M+m))))) v = (√((gssin θ)/((1/5)+((sin^2 θ)/2)+((Mcos^2 θ)/(2(M+m)))))) v=(√((2gssin θ)/((7/5)−((mcos^2 θ)/(M+m))))) And with s=(h/(sin θ))−rtan (θ/2) v=(√((g(h−rtan (θ/2)sin θ))/((1/5)+((sin^2 θ)/2)+((Mcos^2 θ)/(2(M+m)))))) . vcos θ−V = ((Mvcos θ)/(M+m)) ⇒ v_(actual) ^2 =(((Mvcos θ)/(M+m)))^2 +(vsin θ)^2 v_(actual) =v(√(((M/(M+m)))^2 cos^2 θ+sin^2 θ)) this is speed of sphere relative to wedge..](Q71566.png)
$${mgs}\mathrm{sin}\:\theta=\frac{{MV}^{\:\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{2}{mr}^{\mathrm{2}} }{\mathrm{5}}\right)\frac{{v}^{\mathrm{2}} }{{r}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:+\frac{{m}}{\mathrm{2}}\left[\left({v}\mathrm{cos}\:\theta−{V}\right)^{\mathrm{2}} +{v}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta\right] \\ $$$$\:\&\:\:\:\:\:{mv}\mathrm{cos}\:\theta=\left({M}+{m}\right){V} \\ $$$$\Rightarrow\:{V}=\frac{{mv}\mathrm{cos}\:\theta}{{M}+{m}} \\ $$$$\&\:\:\:\:{v}\mathrm{cos}\:\theta−{V}\:=\:\frac{{Mv}\mathrm{cos}\:\theta}{{M}+{m}} \\ $$$${substituting}\:{in}\:{first}\:{eq}. \\ $$$$\:\:{mgs}\mathrm{sin}\:\theta={mv}^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{sin}\:^{\mathrm{2}} \theta}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:\:+\frac{{M}}{\mathrm{2}}\left(\frac{{mv}\mathrm{cos}\:\theta}{{M}+{m}}\right)^{\mathrm{2}} +\frac{{m}}{\mathrm{2}}\left(\frac{{Mv}\mathrm{cos}\:\theta}{{M}+{m}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\:{mgs}\mathrm{sin}\:\theta={mv}^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{sin}\:^{\mathrm{2}} \theta}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\frac{{Mmv}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta}{\mathrm{2}\left({M}+{m}\right)} \\ $$$$\Rightarrow\:\:{v}^{\mathrm{2}} =\frac{{gs}\mathrm{sin}\:\theta}{\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{sin}\:^{\mathrm{2}} \theta}{\mathrm{2}}+\frac{{M}\mathrm{cos}\:^{\mathrm{2}} \theta}{\mathrm{2}\left({M}+{m}\right)}} \\ $$$$\:\:\:{v}\:=\:\sqrt{\frac{{gs}\mathrm{sin}\:\theta}{\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{sin}\:^{\mathrm{2}} \theta}{\mathrm{2}}+\frac{{M}\mathrm{cos}\:^{\mathrm{2}} \theta}{\mathrm{2}\left({M}+{m}\right)}}}\: \\ $$$$\:\:\:\:\:\:{v}=\sqrt{\frac{\mathrm{2}{gs}\mathrm{sin}\:\theta}{\frac{\mathrm{7}}{\mathrm{5}}−\frac{{m}\mathrm{cos}\:^{\mathrm{2}} \theta}{{M}+{m}}}}\: \\ $$$${And}\:\:{with}\:{s}=\frac{{h}}{\mathrm{sin}\:\theta}−{r}\mathrm{tan}\:\frac{\theta}{\mathrm{2}} \\ $$$$\:\:{v}=\sqrt{\frac{{g}\left({h}−{r}\mathrm{tan}\:\frac{\theta}{\mathrm{2}}\mathrm{sin}\:\theta\right)}{\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{sin}\:^{\mathrm{2}} \theta}{\mathrm{2}}+\frac{{M}\mathrm{cos}\:^{\mathrm{2}} \theta}{\mathrm{2}\left({M}+{m}\right)}}}\:. \\ $$$${v}\mathrm{cos}\:\theta−{V}\:=\:\frac{{Mv}\mathrm{cos}\:\theta}{{M}+{m}} \\ $$$$\:\:\Rightarrow\:{v}_{{actual}} ^{\mathrm{2}} =\left(\frac{{Mv}\mathrm{cos}\:\theta}{{M}+{m}}\right)^{\mathrm{2}} +\left({v}\mathrm{sin}\:\theta\right)^{\mathrm{2}} \\ $$$$\:\:\:\:{v}_{{actual}} ={v}\sqrt{\left(\frac{{M}}{{M}+{m}}\right)^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta+\mathrm{sin}\:^{\mathrm{2}} \theta} \\ $$$$\:\:{this}\:{is}\:{speed}\:{of}\:{sphere} \\ $$$$\:\:{relative}\:{to}\:{wedge}.. \\ $$$$ \\ $$
Commented by mr W last updated on 17/Oct/19
$${your}\:{method}\:{is}\:{more}\:{effective},\:{very} \\ $$$${nice}!\:{but}\:{i}\:{didn}'{t}\:{find}\:{where}\:{my}\:{error} \\ $$$${is}. \\ $$
Commented by ajfour last updated on 18/Oct/19
![Nsin θ−fcos θ=Ma ...(i) N+masin θ=mgcos θ ...(ii) mgsin θ+macos θ=f+mαr ...(iii) rf=(2/5)mr^2 α ....(iv) ⇒ masin^2 θ+Ma+fcos θ =mgsin θcos θ ⇒ (M+msin^2 θ)(((f−mgsin θ+mαr)/(mcos θ))) +fcos θ=mgsin θcos θ f=((mgsin θcos θ+(((M+msin^2 θ)(gsin θ−αr))/(cos θ)))/(cos θ+((M+msin^2 θ)/(mcos θ)))) =((m[(M+m)gsin θ−αr(M+msin^2 θ)])/(M+m)) = (2/5)mrα [from (iv)] ⇒ αr[M+msin^2 θ+(2/5)(M+m)] = (M+m)gsin θ ⇒ αr = ((gsin θ)/((7/5)−((mcos^2 θ)/(M+m)))) ((vdv)/ds)=αr ⇒ v=(√(2αrs)) ⇒ v=(√((2gssin θ)/((7/5)−((mcos^2 θ)/(M+m))))) .](Q71621.png)
$${N}\mathrm{sin}\:\theta−{f}\mathrm{cos}\:\theta={Ma}\:\:\:\:\:\:\:\:\:...\left({i}\right) \\ $$$${N}+{ma}\mathrm{sin}\:\theta={mg}\mathrm{cos}\:\theta\:\:\:\:\:\:...\left({ii}\right) \\ $$$${mg}\mathrm{sin}\:\theta+{ma}\mathrm{cos}\:\theta={f}+{m}\alpha{r}\:\:\:\:...\left({iii}\right) \\ $$$${rf}=\frac{\mathrm{2}}{\mathrm{5}}{mr}^{\mathrm{2}} \alpha\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:....\left({iv}\right) \\ $$$$\Rightarrow\:{ma}\mathrm{sin}\:^{\mathrm{2}} \theta+{Ma}+{f}\mathrm{cos}\:\theta \\ $$$$\:\:\:\:\:\:\:\:\:\:\:={mg}\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$$\Rightarrow\:\left({M}+{m}\mathrm{sin}\:^{\mathrm{2}} \theta\right)\left(\frac{{f}−{mg}\mathrm{sin}\:\theta+{m}\alpha{r}}{{m}\mathrm{cos}\:\theta}\right) \\ $$$$\:\:\:\:\:\:\:+{f}\mathrm{cos}\:\theta={mg}\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$${f}=\frac{{mg}\mathrm{sin}\:\theta\mathrm{cos}\:\theta+\frac{\left({M}+{m}\mathrm{sin}\:^{\mathrm{2}} \theta\right)\left({g}\mathrm{sin}\:\theta−\alpha{r}\right)}{\mathrm{cos}\:\theta}}{\mathrm{cos}\:\theta+\frac{{M}+{m}\mathrm{sin}\:^{\mathrm{2}} \theta}{{m}\mathrm{cos}\:\theta}} \\ $$$$\:\:\:=\frac{{m}\left[\left({M}+{m}\right){g}\mathrm{sin}\:\theta−\alpha{r}\left({M}+{m}\mathrm{sin}\:^{\mathrm{2}} \theta\right)\right]}{{M}+{m}} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\mathrm{5}}{mr}\alpha\:\:\:\:\:\:\:\:\left[{from}\:\left({iv}\right)\right]\:\:\:\Rightarrow \\ $$$$\alpha{r}\left[{M}+{m}\mathrm{sin}\:^{\mathrm{2}} \theta+\frac{\mathrm{2}}{\mathrm{5}}\left({M}+{m}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\left({M}+{m}\right){g}\mathrm{sin}\:\theta \\ $$$$\Rightarrow\:\:\alpha{r}\:=\:\frac{{g}\mathrm{sin}\:\theta}{\frac{\mathrm{7}}{\mathrm{5}}−\frac{{m}\mathrm{cos}\:^{\mathrm{2}} \theta}{{M}+{m}}}\:\: \\ $$$$\:\:\:\frac{{vdv}}{{ds}}=\alpha{r}\:\:\Rightarrow\:{v}=\sqrt{\mathrm{2}\alpha{rs}} \\ $$$$\Rightarrow\:\:{v}=\sqrt{\frac{\mathrm{2}{gs}\mathrm{sin}\:\theta}{\frac{\mathrm{7}}{\mathrm{5}}−\frac{{m}\mathrm{cos}\:^{\mathrm{2}} \theta}{{M}+{m}}}}\:. \\ $$