Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 71717 by peter frank last updated on 19/Oct/19

Commented by mathmax by abdo last updated on 19/Oct/19

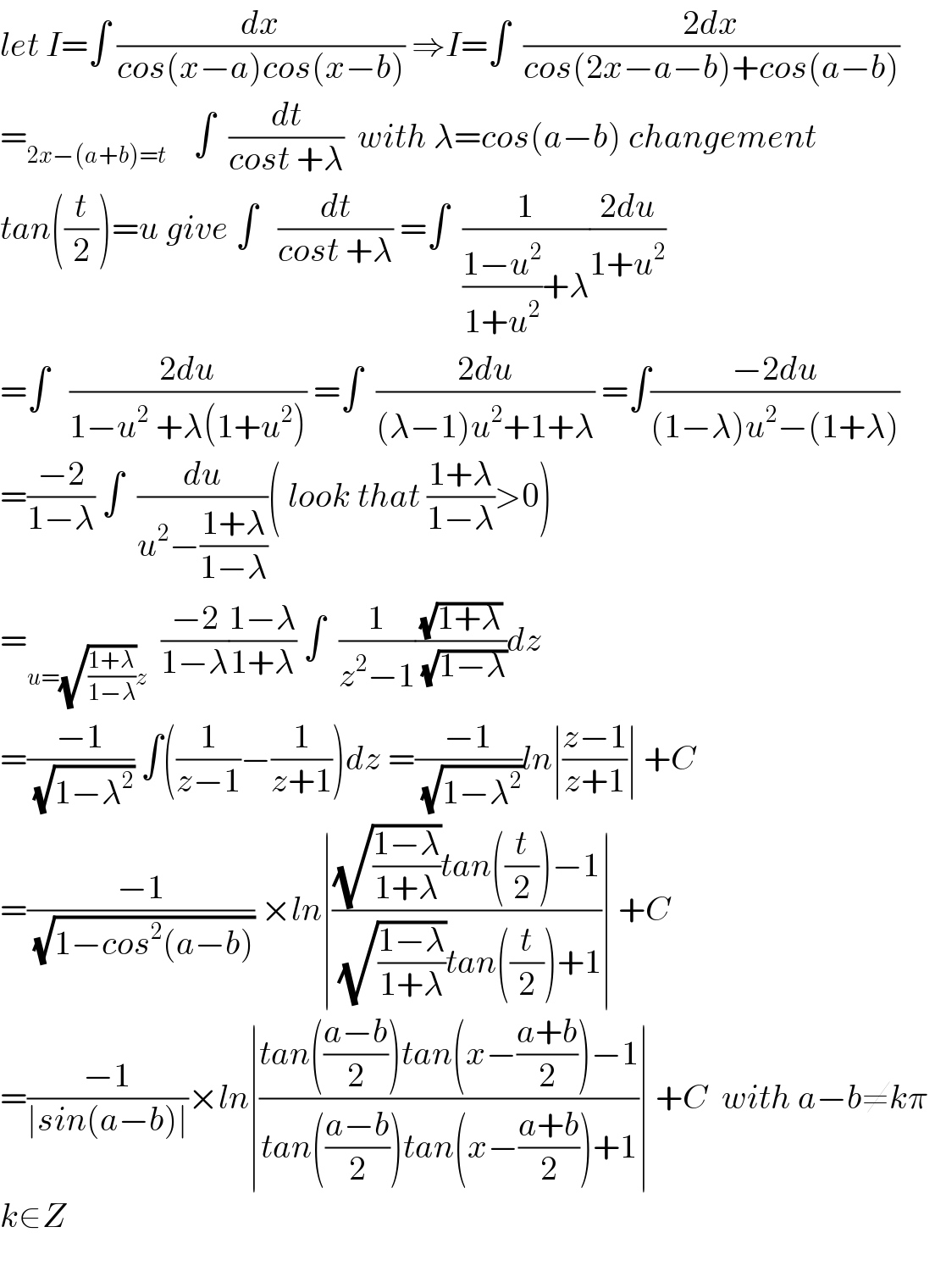
let I=∫ (dx/(cos(x−a)cos(x−b))) ⇒I=∫ ((2dx)/(cos(2x−a−b)+cos(a−b))) =_(2x−(a+b)=t) ∫ (dt/(cost +λ)) with λ=cos(a−b) changement tan((t/2))=u give ∫ (dt/(cost +λ)) =∫ (1/(((1−u^2 )/(1+u^2 ))+λ))((2du)/(1+u^2 )) =∫ ((2du)/(1−u^2 +λ(1+u^2 ))) =∫ ((2du)/((λ−1)u^2 +1+λ)) =∫((−2du)/((1−λ)u^2 −(1+λ))) =((−2)/(1−λ)) ∫ (du/(u^2 −((1+λ)/(1−λ))))( look that ((1+λ)/(1−λ))>0) =_(u=(√((1+λ)/(1−λ)))z) ((−2)/(1−λ))((1−λ)/(1+λ)) ∫ (1/(z^2 −1))((√(1+λ))/(√(1−λ)))dz =((−1)/(√(1−λ^2 ))) ∫((1/(z−1))−(1/(z+1)))dz =((−1)/(√(1−λ^2 )))ln∣((z−1)/(z+1))∣ +C =((−1)/(√(1−cos^2 (a−b)))) ×ln∣(((√((1−λ)/(1+λ)))tan((t/2))−1)/((√((1−λ)/(1+λ)))tan((t/2))+1))∣ +C =((−1)/(∣sin(a−b)∣))×ln∣((tan(((a−b)/2))tan(x−((a+b)/2))−1)/(tan(((a−b)/2))tan(x−((a+b)/2))+1))∣ +C with a−b≠kπ k∈Z

$${let}\:{I}=\int\:\frac{{dx}}{{cos}\left({x}−{a}\right){cos}\left({x}−{b}\right)}\:\Rightarrow{I}=\int\:\:\frac{\mathrm{2}{dx}}{{cos}\left(\mathrm{2}{x}−{a}−{b}\right)+{cos}\left({a}−{b}\right)} \\ $$$$=_{\mathrm{2}{x}−\left({a}+{b}\right)={t}} \:\:\:\:\int\:\:\frac{{dt}}{{cost}\:+\lambda}\:\:{with}\:\lambda={cos}\left({a}−{b}\right)\:{changement} \\ $$$${tan}\left(\frac{{t}}{\mathrm{2}}\right)={u}\:{give}\:\int\:\:\:\frac{{dt}}{{cost}\:+\lambda}\:=\int\:\:\frac{\mathrm{1}}{\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }+\lambda}\frac{\mathrm{2}{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$$$=\int\:\:\:\frac{\mathrm{2}{du}}{\mathrm{1}−{u}^{\mathrm{2}} \:+\lambda\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:=\int\:\:\frac{\mathrm{2}{du}}{\left(\lambda−\mathrm{1}\right){u}^{\mathrm{2}} +\mathrm{1}+\lambda}\:=\int\frac{−\mathrm{2}{du}}{\left(\mathrm{1}−\lambda\right){u}^{\mathrm{2}} −\left(\mathrm{1}+\lambda\right)} \\ $$$$=\frac{−\mathrm{2}}{\mathrm{1}−\lambda}\:\int\:\:\frac{{du}}{{u}^{\mathrm{2}} −\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}}\left(\:{look}\:{that}\:\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}>\mathrm{0}\right) \\ $$$$=_{{u}=\sqrt{\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}}{z}} \:\:\frac{−\mathrm{2}}{\mathrm{1}−\lambda}\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda}\:\int\:\:\frac{\mathrm{1}}{{z}^{\mathrm{2}} −\mathrm{1}}\frac{\sqrt{\mathrm{1}+\lambda}}{\sqrt{\mathrm{1}−\lambda}}{dz} \\ $$$$=\frac{−\mathrm{1}}{\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}\:\int\left(\frac{\mathrm{1}}{{z}−\mathrm{1}}−\frac{\mathrm{1}}{{z}+\mathrm{1}}\right){dz}\:=\frac{−\mathrm{1}}{\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}{ln}\mid\frac{{z}−\mathrm{1}}{{z}+\mathrm{1}}\mid\:+{C} \\ $$$$=\frac{−\mathrm{1}}{\sqrt{\mathrm{1}−{cos}^{\mathrm{2}} \left({a}−{b}\right)}}\:×{ln}\mid\frac{\sqrt{\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda}}{tan}\left(\frac{{t}}{\mathrm{2}}\right)−\mathrm{1}}{\sqrt{\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda}}{tan}\left(\frac{{t}}{\mathrm{2}}\right)+\mathrm{1}}\mid\:+{C} \\ $$$$=\frac{−\mathrm{1}}{\mid{sin}\left({a}−{b}\right)\mid}×{ln}\mid\frac{{tan}\left(\frac{{a}−{b}}{\mathrm{2}}\right){tan}\left({x}−\frac{{a}+{b}}{\mathrm{2}}\right)−\mathrm{1}}{{tan}\left(\frac{{a}−{b}}{\mathrm{2}}\right){tan}\left({x}−\frac{{a}+{b}}{\mathrm{2}}\right)+\mathrm{1}}\mid\:+{C}\:\:{with}\:{a}−{b}\neq{k}\pi\: \\ $$$${k}\in{Z}\: \\ $$$$ \\ $$

Commented by peter frank last updated on 20/Oct/19

thank you

$${thank}\:{you} \\ $$$$ \\ $$

Commented by mathmax by abdo last updated on 23/Oct/19

you are welcome.

$${you}\:{are}\:{welcome}. \\ $$

Answered by Tanmay chaudhury last updated on 19/Oct/19

(1/(sin(a−b)))∫((sin{(x−b)−(x−a)})/(cos(x−a)cos(x−b)))dx (1/(sin(a−b)))∫((sin(x−b)cos(x−a)−cos(x−b)sin(x−a))/(cos(x−a)cos(x−b)))dx (1/(sin(a−b)))∫[tan(x−b)−tan(x−a)]dx (1/(sin(a−b)))[lnsec(x−b)−lnsec(x−a)]+c (1/(sin(a−b)))ln(((sec(x−b))/(sec(x−a))))+c

$$\frac{\mathrm{1}}{{sin}\left({a}−{b}\right)}\int\frac{{sin}\left\{\left({x}−{b}\right)−\left({x}−{a}\right)\right\}}{{cos}\left({x}−{a}\right){cos}\left({x}−{b}\right)}{dx} \\ $$$$\frac{\mathrm{1}}{{sin}\left({a}−{b}\right)}\int\frac{{sin}\left({x}−{b}\right){cos}\left({x}−{a}\right)−{cos}\left({x}−{b}\right){sin}\left({x}−{a}\right)}{{cos}\left({x}−{a}\right){cos}\left({x}−{b}\right)}{dx} \\ $$$$\frac{\mathrm{1}}{{sin}\left({a}−{b}\right)}\int\left[{tan}\left({x}−{b}\right)−{tan}\left({x}−{a}\right)\right]{dx} \\ $$$$\frac{\mathrm{1}}{{sin}\left({a}−{b}\right)}\left[{lnsec}\left({x}−{b}\right)−{lnsec}\left({x}−{a}\right)\right]+{c} \\ $$$$\frac{\mathrm{1}}{{sin}\left({a}−{b}\right)}{ln}\left(\frac{{sec}\left({x}−{b}\right)}{{sec}\left({x}−{a}\right)}\right)+{c} \\ $$

Commented by $@ty@m123 last updated on 19/Oct/19

Quite easier but tricky.

$${Quite}\:{easier}\:{but}\:{tricky}. \\ $$

Commented by peter frank last updated on 19/Oct/19

thank you very much

$${thank}\:{you}\:{very}\:{much} \\ $$$$ \\ $$

Commented by Prithwish sen last updated on 19/Oct/19

tanmoy sir pujo kamon katlo. subho bijaya. kothae chilen ? bhalo achen ?

$$\mathrm{tanmoy}\:\mathrm{sir}\:\mathrm{pujo}\:\mathrm{kamon}\:\mathrm{katlo}.\:\mathrm{subho}\:\mathrm{bijaya}. \\ $$$$\mathrm{kothae}\:\mathrm{chilen}\:?\:\mathrm{bhalo}\:\mathrm{achen}\:? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com