Let x = f(x) e^(f(x)) ∫_( 0) ^( e) f(x) dx = ?


Question and Answers Forum

All Questions Topic List
None Questions
Previous in All Question Next in All Question
Previous in None Next in None
Question Number 72296 by naka3546 last updated on 27/Oct/19

$L e t x = f (x) e^{f (x)}$ $\int_{0} \overset{e}{} f (x) d x = ?$
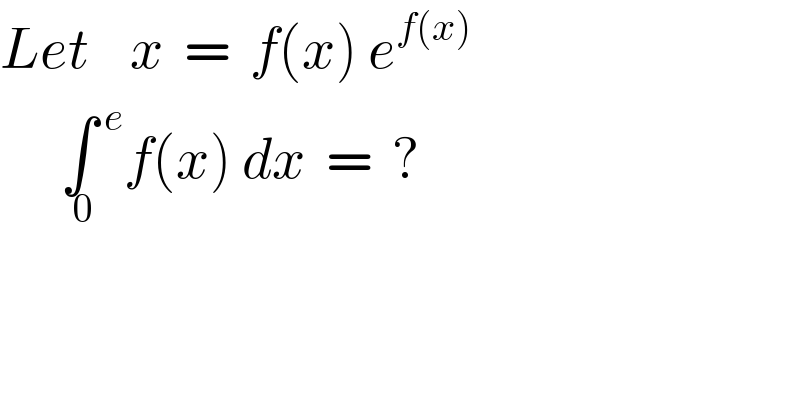
Commented by naka3546 last updated on 27/Oct/19

$e^{\ln x} = x$
Commented by mr W last updated on 27/Oct/19

Commented by mind is power last updated on 27/Oct/19

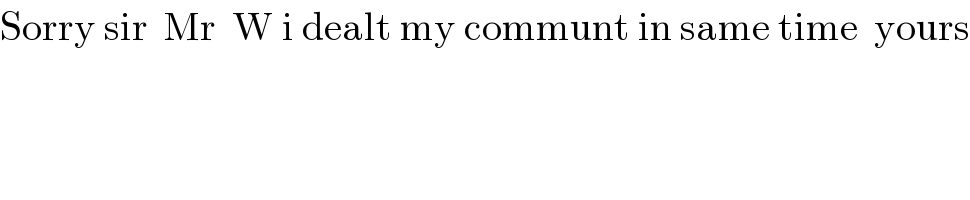
$Sorry sir Mr W i dealt my communt in same time yours$
Commented by mr W last updated on 27/Oct/19

${t h a t}^{'} s o k . i p u t s o m e m a t e r i a l i f o u n d$ $i n w e k i p e d i a .$
	Answered by mind is power last updated on 27/Oct/19
	$∫f(x)dx=[xf(x)]−∫xf′(x)dx x=f(x)e^(f(x)) ⇒1=(f′(x)+f′(x).f(x))e^(f(x)) ⇒f′(x)=(1/((1+f(x))e^(f(x)) ))=((f(x))/(x(1+f(x))))⇒xf′(x)=((f(x))/(1+f(x))) ⇒∫f(x)dx=xf(x)−∫((f(x))/(1+f(x)))dx let u=f(x) ⇒du=f′(x)dx=((f(x))/(x(1+f(x))))dx x=f(x)e^(f(x)) =ue^u ⇒du=(u/(ue^u (1+u)))dx⇒dx=((ue^u du)/u).((1+u)/) ∫((f(x))/(1+f(x)))dx=∫(u/(1+u)).((ue^u du)/u).(1+u)=∫ue^u =(u−1)e^u +c =(f(x)−1)e^(f(x)) +c=x−e^(f(x)) +c=x(1−(1/(f(x))))+c ⇒∫f(x)dx=xf(x)−x(1−(1/(f(x))))+c=x(f(x)−1+(1/(f(x))))+c ∫_0 ^e f(x)dx=e(f(e)−1+(1/(f(e)))) f(e)e^(f(e)) =e=1.e^1 ..z f(t)=te^t ⇒f′(t)=(t+1)e^t ≥0 ∀t∈IR^+ f(1)=e⇒xe^x =e⇔x∈{1} z⇒f(e)=1 ∫_0 ^e f(x)dx=e(1−1+(1/1))−lim_(x→0) x(f(x)−1+(1/(f(x)))) (1/(f(x)))=(e^(f(x)) /x) x(f(x)−1+(1/(f(x))))=x(f(x)−1+(e^(f(x)) /x))=x(f(x)−1)+e^(f(x)) lim_(x→0) x(f(x)−1)+e^(f(x)) =e^(f(0)) f(0)e^(f(0)) =0⇒f(0)=0⇒e^(f(0)) =1 ∫_0 ^e f(x)dx=e−1$
	$\int f (x) dx = [xf (x)] - \int {xf}^{'} (x) dx$ $x = f (x) e^{f (x)} \Rightarrow 1 = (f^{'} (x) + f^{'} (x) . f (x)) e^{f (x)}$ $\Rightarrow f^{'} (x) = \frac{1}{(1 + f (x)) e^{f (x)}} = \frac{f (x)}{x (1 + f (x))} \Rightarrow {xf}^{'} (x) = \frac{f (x)}{1 + f (x)}$ $\Rightarrow \int f (x) dx = xf (x) - \int \frac{f (x)}{1 + f (x)} dx$ $let u = f (x)$ $\Rightarrow du = f^{'} (x) dx = \frac{f (x)}{x (1 + f (x))} dx$ $x = f (x) e^{f (x)} = {ue}^{u} \Rightarrow du = \frac{u}{{ue}^{u} (1 + u)} dx \Rightarrow dx = \frac{{ue}^{u} du}{u} . \frac{1 + u}{}$ $\int \frac{f (x)}{1 + f (x)} dx = \int \frac{u}{1 + u} . \frac{{ue}^{u} du}{u} . (1 + u) = \int {ue}^{u} = (u - 1) e^{u} + c$ $= (f (x) - 1) e^{f (x)} + c = x - e^{f (x)} + c = x (1 - \frac{1}{f (x)}) + c$ $\Rightarrow \int f (x) dx = xf (x) - x (1 - \frac{1}{f (x)}) + c = x (f (x) - 1 + \frac{1}{f (x)}) + c$ $\int_{0}^{e} f (x) dx = e (f (e) - 1 + \frac{1}{f (e)})$ $f (e) e^{f (e)} = e = 1 . e^{1} . . z$ $f (t) = {te}^{t} \Rightarrow f^{'} (t) = (t + 1) e^{t} ⩾ 0 \forall t \in {IR}^{+}$ $f (1) = e \Rightarrow {xe}^{x} = e \Leftrightarrow x \in {1}$ $z \Rightarrow f (e) = 1$ $\int_{0}^{e} f (x) dx = e (1 - 1 + \frac{1}{1}) - \lim_{x \to 0} x (f (x) - 1 + \frac{1}{f (x)})$ $\frac{1}{f (x)} = \frac{e^{f (x)}}{x}$ $x (f (x) - 1 + \frac{1}{f (x)}) = x (f (x) - 1 + \frac{e^{f (x)}}{x}) = x (f (x) - 1) + e^{f (x)}$ $\lim_{x \to 0} x (f (x) - 1) + e^{f (x)} = e^{f (0)}$ $f (0) e^{f (0)} = 0 \Rightarrow f (0) = 0 \Rightarrow e^{f (0)} = 1$ $\int_{0}^{e} f (x) dx = e - 1$
	Commented by mr W last updated on 27/Oct/19

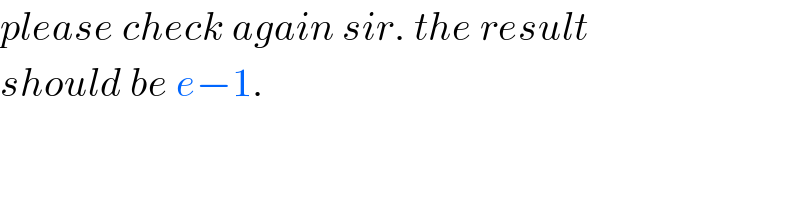
	$p l e a s e c h e c k a g a i n s i r . t h e r e s u l t$ $s h o u l d b e e - 1 .$
	Commented by mr W last updated on 27/Oct/19

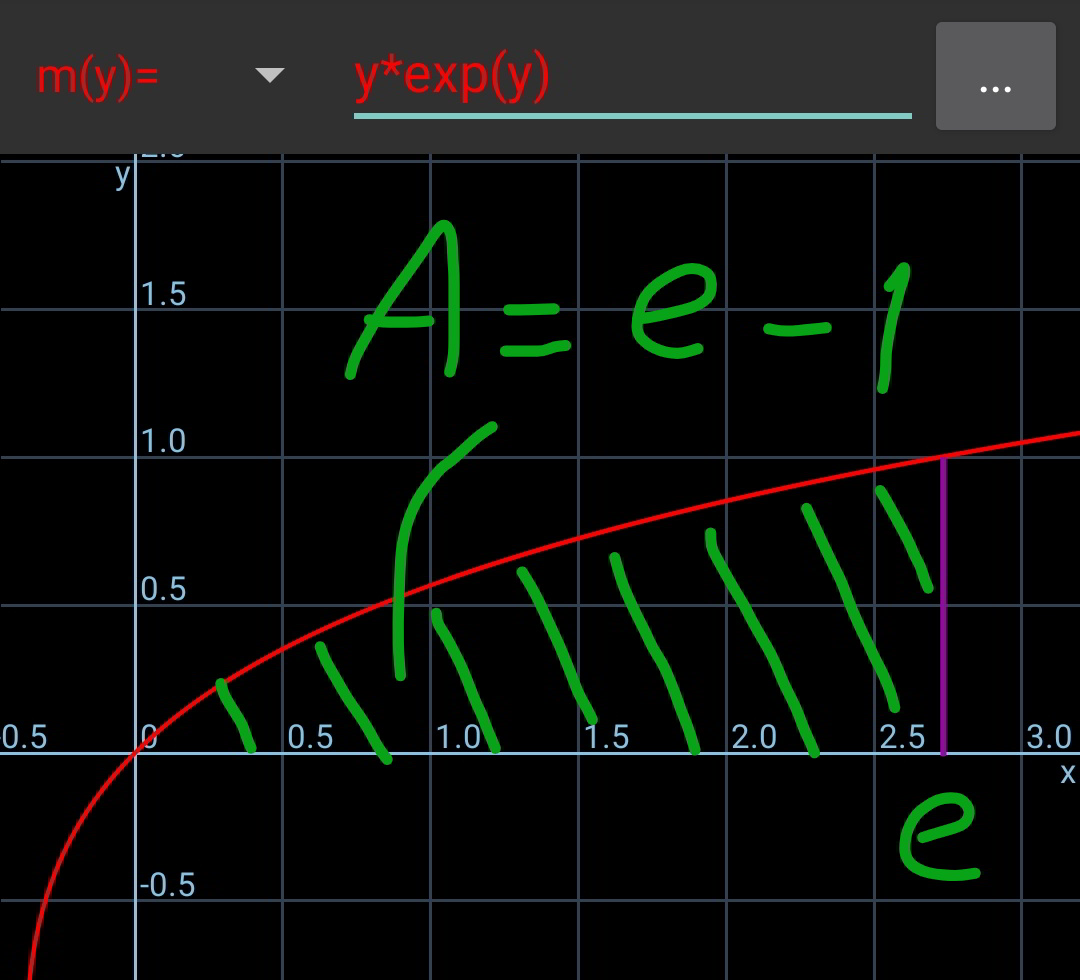
	Commented by mind is power last updated on 27/Oct/19

	$yeah i got it x (f (x) - 1 + \frac{1}{f (x)}) in 0 i did zero$ $but its not [defind cause f (0) = 0$ $we should tack \lim$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum