Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 72397 by mathmax by abdo last updated on 28/Oct/19

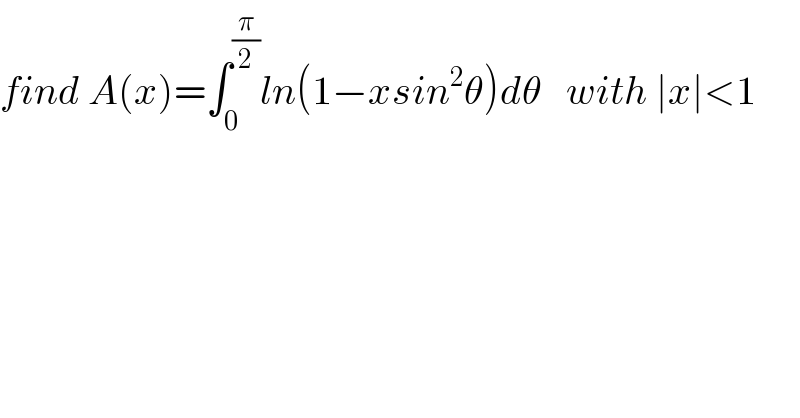
find A(x)=∫_0 ^(π/2) ln(1−xsin^2 θ)dθ with ∣x∣<1

$${find}\:{A}\left({x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{1}−{xsin}^{\mathrm{2}} \theta\right){d}\theta\:\:\:{with}\:\mid{x}\mid<\mathrm{1} \\ $$

Commented bymathmax by abdo last updated on 28/Oct/19

at form of serie we have ln^′ (1−u)=((−1)/(1−u)) =−Σ_(n=0) ^∞ u^n ⇒ ln(1−u)=−Σ_(n=0) ^∞ (u^(n+1) /(n+1)) +c (c=0) =−Σ_(n=1) ^∞ (u^n /n) ⇒ln(1−xsin^2 θ)=−Σ_(n=1) ^∞ ((x^n sin^(2n) θ)/n) ⇒ A(x)=−Σ_(n=1) ^∞ (∫_0 ^(π/2) sin^(2n) θ dθ)(x^n /n) =−Σ_(n=1) ^∞ (W_n /n) x^n with W_n =∫_0 ^(π/2) sin^(2n) dθ (wallis integral)

$${at}\:{form}\:{of}\:{serie}\:{we}\:{have}\:{ln}^{'} \left(\mathrm{1}−{u}\right)=\frac{−\mathrm{1}}{\mathrm{1}−{u}}\:=−\sum_{{n}=\mathrm{0}} ^{\infty} \:{u}^{{n}} \:\Rightarrow \\ $$ $${ln}\left(\mathrm{1}−{u}\right)=−\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{u}^{{n}+\mathrm{1}} }{{n}+\mathrm{1}}\:+{c}\:\:\:\left({c}=\mathrm{0}\right) \\ $$ $$=−\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{{u}^{{n}} }{{n}}\:\Rightarrow{ln}\left(\mathrm{1}−{xsin}^{\mathrm{2}} \theta\right)=−\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{{x}^{{n}} \:{sin}^{\mathrm{2}{n}} \theta}{{n}}\:\Rightarrow \\ $$ $${A}\left({x}\right)=−\sum_{{n}=\mathrm{1}} ^{\infty} \left(\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:{sin}^{\mathrm{2}{n}} \theta\:{d}\theta\right)\frac{{x}^{{n}} }{{n}}\:=−\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{{W}_{{n}} }{{n}}\:{x}^{{n}} \\ $$ $${with}\:{W}_{{n}} =\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:{sin}^{\mathrm{2}{n}} {d}\theta\:\:\left({wallis}\:{integral}\right) \\ $$

Commented bymathmax by abdo last updated on 28/Oct/19

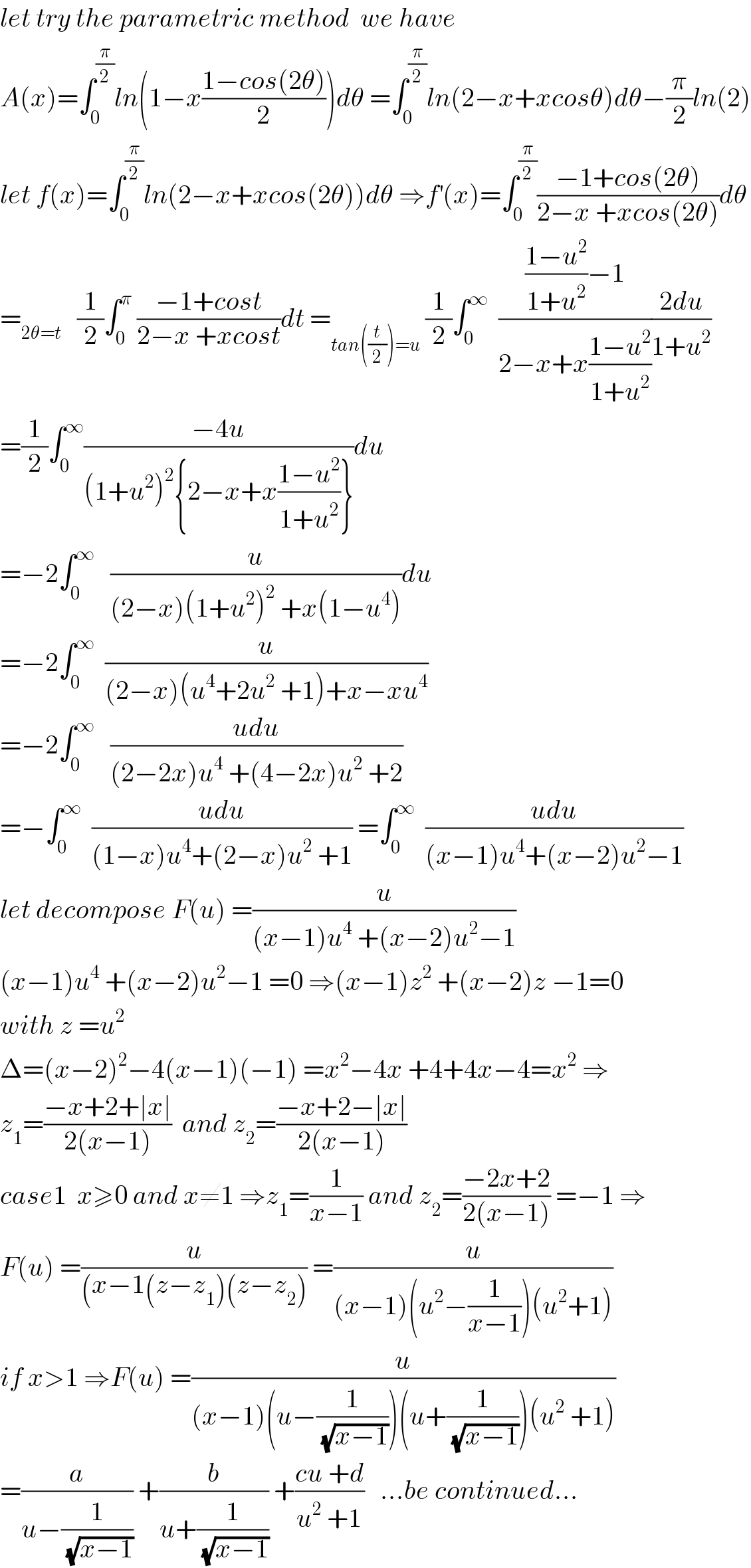
let try the parametric method we have A(x)=∫_0 ^(π/2) ln(1−x((1−cos(2θ))/2))dθ =∫_0 ^(π/2) ln(2−x+xcosθ)dθ−(π/2)ln(2) let f(x)=∫_0 ^(π/2) ln(2−x+xcos(2θ))dθ ⇒f^′ (x)=∫_0 ^(π/2) ((−1+cos(2θ))/(2−x +xcos(2θ)))dθ =_(2θ=t) (1/2)∫_0 ^π ((−1+cost)/(2−x +xcost))dt =_(tan((t/2))=u) (1/2)∫_0 ^∞ ((((1−u^2 )/(1+u^2 ))−1)/(2−x+x((1−u^2 )/(1+u^2 ))))((2du)/(1+u^2 )) =(1/2)∫_0 ^∞ ((−4u)/((1+u^2 )^2 {2−x+x((1−u^2 )/(1+u^2 ))}))du =−2∫_0 ^∞ (u/((2−x)(1+u^2 )^2 +x(1−u^4 )))du =−2∫_0 ^∞ (u/((2−x)(u^4 +2u^2 +1)+x−xu^4 )) =−2∫_0 ^∞ ((udu)/((2−2x)u^4 +(4−2x)u^2 +2)) =−∫_0 ^∞ ((udu)/((1−x)u^4 +(2−x)u^2 +1)) =∫_0 ^∞ ((udu)/((x−1)u^4 +(x−2)u^2 −1)) let decompose F(u) =(u/((x−1)u^4 +(x−2)u^2 −1)) (x−1)u^4 +(x−2)u^2 −1 =0 ⇒(x−1)z^2 +(x−2)z −1=0 with z =u^2 Δ=(x−2)^2 −4(x−1)(−1) =x^2 −4x +4+4x−4=x^2 ⇒ z_1 =((−x+2+∣x∣)/(2(x−1))) and z_2 =((−x+2−∣x∣)/(2(x−1))) case1 x≥0 and x≠1 ⇒z_1 =(1/(x−1)) and z_2 =((−2x+2)/(2(x−1))) =−1 ⇒ F(u) =(u/((x−1(z−z_1 )(z−z_2 ))) =(u/((x−1)(u^2 −(1/(x−1)))(u^2 +1))) if x>1 ⇒F(u) =(u/((x−1)(u−(1/(√(x−1))))(u+(1/(√(x−1))))(u^2 +1))) =(a/(u−(1/(√(x−1))))) +(b/(u+(1/(√(x−1))))) +((cu +d)/(u^2 +1)) ...be continued...

$${let}\:{try}\:{the}\:{parametric}\:{method}\:\:{we}\:{have} \\ $$ $${A}\left({x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{1}−{x}\frac{\mathrm{1}−{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}\right){d}\theta\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{2}−{x}+{xcos}\theta\right){d}\theta−\frac{\pi}{\mathrm{2}}{ln}\left(\mathrm{2}\right) \\ $$ $${let}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{2}−{x}+{xcos}\left(\mathrm{2}\theta\right)\right){d}\theta\:\Rightarrow{f}^{'} \left({x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{−\mathrm{1}+{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}−{x}\:+{xcos}\left(\mathrm{2}\theta\right)}{d}\theta \\ $$ $$=_{\mathrm{2}\theta={t}} \:\:\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \:\frac{−\mathrm{1}+{cost}}{\mathrm{2}−{x}\:+{xcost}}{dt}\:=_{{tan}\left(\frac{{t}}{\mathrm{2}}\right)={u}} \:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }−\mathrm{1}}{\mathrm{2}−{x}+{x}\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }}\frac{\mathrm{2}{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$ $$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{−\mathrm{4}{u}}{\left(\mathrm{1}+{u}^{\mathrm{2}} \right)^{\mathrm{2}} \left\{\mathrm{2}−{x}+{x}\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }\right\}}{du} \\ $$ $$=−\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{u}}{\left(\mathrm{2}−{x}\right)\left(\mathrm{1}+{u}^{\mathrm{2}} \right)^{\mathrm{2}} \:+{x}\left(\mathrm{1}−{u}^{\mathrm{4}} \right)}{du} \\ $$ $$=−\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\frac{{u}}{\left(\mathrm{2}−{x}\right)\left({u}^{\mathrm{4}} +\mathrm{2}{u}^{\mathrm{2}} \:+\mathrm{1}\right)+{x}−{xu}^{\mathrm{4}} } \\ $$ $$=−\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{udu}}{\left(\mathrm{2}−\mathrm{2}{x}\right){u}^{\mathrm{4}} \:+\left(\mathrm{4}−\mathrm{2}{x}\right){u}^{\mathrm{2}} \:+\mathrm{2}} \\ $$ $$=−\int_{\mathrm{0}} ^{\infty} \:\:\frac{{udu}}{\left(\mathrm{1}−{x}\right){u}^{\mathrm{4}} +\left(\mathrm{2}−{x}\right){u}^{\mathrm{2}} \:+\mathrm{1}}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{udu}}{\left({x}−\mathrm{1}\right){u}^{\mathrm{4}} +\left({x}−\mathrm{2}\right){u}^{\mathrm{2}} −\mathrm{1}} \\ $$ $${let}\:{decompose}\:{F}\left({u}\right)\:=\frac{{u}}{\left({x}−\mathrm{1}\right){u}^{\mathrm{4}} \:+\left({x}−\mathrm{2}\right){u}^{\mathrm{2}} −\mathrm{1}} \\ $$ $$\left({x}−\mathrm{1}\right){u}^{\mathrm{4}} \:+\left({x}−\mathrm{2}\right){u}^{\mathrm{2}} −\mathrm{1}\:=\mathrm{0}\:\Rightarrow\left({x}−\mathrm{1}\right){z}^{\mathrm{2}} \:+\left({x}−\mathrm{2}\right){z}\:−\mathrm{1}=\mathrm{0} \\ $$ $${with}\:{z}\:={u}^{\mathrm{2}} \\ $$ $$\Delta=\left({x}−\mathrm{2}\right)^{\mathrm{2}} −\mathrm{4}\left({x}−\mathrm{1}\right)\left(−\mathrm{1}\right)\:={x}^{\mathrm{2}} −\mathrm{4}{x}\:+\mathrm{4}+\mathrm{4}{x}−\mathrm{4}={x}^{\mathrm{2}} \:\Rightarrow \\ $$ $${z}_{\mathrm{1}} =\frac{−{x}+\mathrm{2}+\mid{x}\mid}{\mathrm{2}\left({x}−\mathrm{1}\right)}\:\:{and}\:{z}_{\mathrm{2}} =\frac{−{x}+\mathrm{2}−\mid{x}\mid}{\mathrm{2}\left({x}−\mathrm{1}\right)} \\ $$ $${case}\mathrm{1}\:\:{x}\geqslant\mathrm{0}\:{and}\:{x}\neq\mathrm{1}\:\Rightarrow{z}_{\mathrm{1}} =\frac{\mathrm{1}}{{x}−\mathrm{1}}\:{and}\:{z}_{\mathrm{2}} =\frac{−\mathrm{2}{x}+\mathrm{2}}{\mathrm{2}\left({x}−\mathrm{1}\right)}\:=−\mathrm{1}\:\Rightarrow \\ $$ $${F}\left({u}\right)\:=\frac{{u}}{\left({x}−\mathrm{1}\left({z}−{z}_{\mathrm{1}} \right)\left({z}−{z}_{\mathrm{2}} \right)\right.}\:=\frac{{u}}{\left({x}−\mathrm{1}\right)\left({u}^{\mathrm{2}} −\frac{\mathrm{1}}{{x}−\mathrm{1}}\right)\left({u}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$ $${if}\:{x}>\mathrm{1}\:\Rightarrow{F}\left({u}\right)\:=\frac{{u}}{\left({x}−\mathrm{1}\right)\left({u}−\frac{\mathrm{1}}{\sqrt{{x}−\mathrm{1}}}\right)\left({u}+\frac{\mathrm{1}}{\sqrt{{x}−\mathrm{1}}}\right)\left({u}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$ $$=\frac{{a}}{{u}−\frac{\mathrm{1}}{\sqrt{{x}−\mathrm{1}}}}\:+\frac{{b}}{{u}+\frac{\mathrm{1}}{\sqrt{{x}−\mathrm{1}}}}\:+\frac{{cu}\:+{d}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\:\:\:...{be}\:{continued}... \\ $$

Commented bymind is power last updated on 29/Oct/19

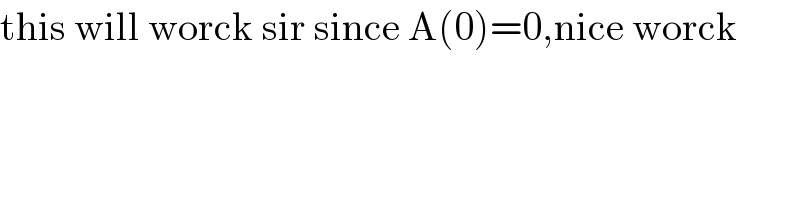
this will worck sir since A(0)=0,nice worck

$$\mathrm{this}\:\mathrm{will}\:\mathrm{worck}\:\mathrm{sir}\:\mathrm{since}\:\mathrm{A}\left(\mathrm{0}\right)=\mathrm{0},\mathrm{nice}\:\mathrm{worck} \\ $$

Commented bymathmax by abdo last updated on 29/Oct/19

we have A(0)=0

$${we}\:{have}\:{A}\left(\mathrm{0}\right)=\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com