
Question and Answers Forum
Question Number 72789 by Learner-123 last updated on 02/Nov/19
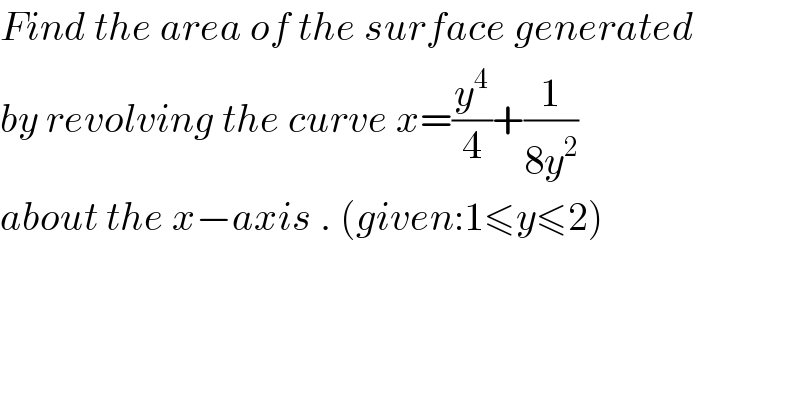
Commented by MJS last updated on 02/Nov/19
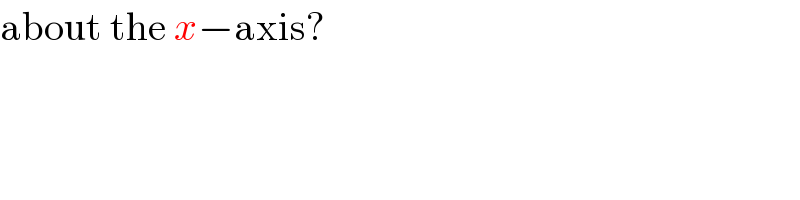
Commented by Learner-123 last updated on 03/Nov/19
Commented by MJS last updated on 03/Nov/19

Commented by Learner-123 last updated on 03/Nov/19

Answered by MJS last updated on 02/Nov/19
![about the y−axis the formula is ∫_a ^b x(√(1+(x′)^2 ))dy x=((2y^6 +1)/(8y^2 )) ⇒ x′=((4y^6 −1)/(4y^3 )) 2π∫_1 ^2 ((2y^6 +1)/(8y^2 ))(√(1+(((4y^6 −1)/(4y^3 )))^2 ))dy= =2π∫_1 ^2 ((2y^6 +1)/(8y^2 ))(√((16y^(12) +8y^6 +1)/(16y^6 )))dy= =2π∫_1 ^2 ((2y^6 +1)/(8y^2 ))(√((((4y^6 +1)/(4y^3 )))^2 ))dy= =2π∫_1 ^2 ((2y^6 +1)/(8y^2 ))×((4y^6 +1)/(4y^3 ))dy= =(π/2)∫_1 ^2 y^7 dy+((3π)/8)∫_1 ^2 ydy+(π/(16))∫_1 ^2 (dy/y^5 )= =[(π/(16))y^8 +((3π)/(16))y^2 −(π/(64y^4 ))]_1 ^2 =((16911π)/(1024))](Q72793.png)
Commented by Learner-123 last updated on 03/Nov/19

