Question Number 73673 by ajfour last updated on 14/Nov/19
Commented by ajfour last updated on 14/Nov/19
$${Q}\:\mathrm{73503}\:\:\:\left({another}\:{way}\:\:{a}\:{try}..\right) \\ $$
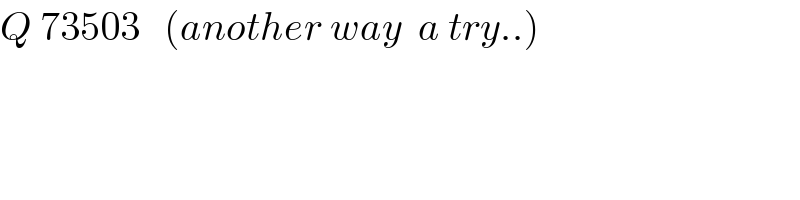
Commented by ajfour last updated on 14/Nov/19
Commented by mind is power last updated on 15/Nov/19
![we can use parametric surface if f:IR^2 →IR^3 a surface paramtric f(u,v)=(a(u,v),b(u,v),c(u,v)) parametric of surface we will ginde parametrisation of ilimunated light A(u)=∫∫_A ∣(∂f/∂u)(u,v)∧(∂f/∂v)(u,v)∣dudv i show steps 1 put repert 2,equation of semis disc ∴ D ∵wich light pass throw 3,∀a∈D→a′∈A∩C since light horizontal C is Cone D=(0,pcos(t),psin(t)),t∈[(π/2),((3π)/2)],p∈[0,(a/(√3))] ⇒aa′^→ =s.i^→ i^→ =(1,0,0) use C={(x,y,z)∈R^3 }∣x^2 +y^2 =z^2 tg^2 (θ) we find s ther s (t,p) a′(t,p) is our paramtrisation of A our f(u,v) below i will poste it this methode use somm topological object surfaces](Q73754.png)
$${we}\:{can}\:{use}\:\:\:{parametric}\:{surface} \\ $$$${if}\:\:{f}:{IR}^{\mathrm{2}} \rightarrow{IR}^{\mathrm{3}} \:\:\:{a}\:{surface}\:\:\:{paramtric} \\ $$$${f}\left({u},{v}\right)=\left({a}\left({u},{v}\right),{b}\left({u},{v}\right),{c}\left({u},{v}\right)\right)\:{parametric}\:\:{of}\:{surface} \\ $$$${we}\:{will}\:{ginde}\:{parametrisation}\:{of}\:{ilimunated}\:{light} \\ $$$$\mathcal{A}\left({u}\right)=\int\int_{\mathcal{A}} \:\mid\frac{\partial{f}}{\partial{u}}\left({u},{v}\right)\wedge\frac{\partial{f}}{\partial{v}}\left({u},{v}\right)\mid{dudv} \\ $$$${i}\:{show}\:{steps} \\ $$$$\mathrm{1}\:{put}\:{repert} \\ $$$$\mathrm{2},{equation}\:{of}\:{semis}\:{disc}\:\therefore\:\:{D}\:\:\because{wich}\:{light}\:{pass}\:{throw} \\ $$$$ \\ $$$$\mathrm{3},\forall{a}\in{D}\rightarrow{a}'\in\mathcal{A}\cap{C}\:\:\:{since}\:{light}\:{horizontal} \\ $$$${C}\:{is}\:{Cone} \\ $$$${D}=\left(\mathrm{0},{pcos}\left({t}\right),{psin}\left({t}\right)\right),{t}\in\left[\frac{\pi}{\mathrm{2}},\frac{\mathrm{3}\pi}{\mathrm{2}}\right],{p}\in\left[\mathrm{0},\frac{{a}}{\sqrt{\mathrm{3}}}\right] \\ $$$$\Rightarrow{aa}\overset{\rightarrow} {'}={s}.\overset{\rightarrow} {{i}} \\ $$$$\overset{\rightarrow} {{i}}=\left(\mathrm{1},\mathrm{0},\mathrm{0}\right) \\ $$$${use}\:{C}=\left\{\left({x},{y},{z}\right)\in\mathbb{R}^{\mathrm{3}} \right\}\mid{x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={z}^{\mathrm{2}} {tg}^{\mathrm{2}} \left(\theta\right) \\ $$$${we}\:{find}\:{s}\:{ther}\:{s}\:\left({t},{p}\right) \\ $$$${a}'\left({t},{p}\right)\:{is}\:{our}\:{paramtrisation}\:{of}\:\mathcal{A}\:{our}\:{f}\left({u},{v}\right)\:{below} \\ $$$${i}\:{will}\:{poste}\:{it}\:\:\:{this}\:{methode}\:{use}\:{somm}\:{topological}\:{object} \\ $$$${surfaces} \\ $$
Answered by ajfour last updated on 15/Nov/19
![Let P be a point on circumference of circle y^2 +(z−r)^2 =r^2 P (0, rsin θ, r−rcos θ) Its shadow on slant surface of cone S(ssin αcos φ, ssin αsin φ, a(√3)−scos α) ⇒ ssin αsin φ=rsin θ (α=30°) a(√3)−scos α=r−rcos θ s=((a(√3)−r(1−cos θ))/(cos α)) sin φ=((rsin θcos α)/((a(√3)−r+rcos θ)sin α)) sin φ=((sin θ)/((√3)−1+cos θ)) ds=((−rsin θdθ)/(cos α)) (s/(2a))=(ρ/a) ⇒ ρ=(s/2) A=∫ρ(2φ)(−ds) = ∫(s)(−ds)φ =∫_0 ^( π) (((a(√3)−r(1−cos θ))/(cos α)))(((rsin θdθ)/(cos α)))(sin^(−1) {((rsin θcos α)/((a(√3)−r+rcos θ)sin α))}) =((4a^2 )/3)∫_0 ^( π) [1−(1/3)(1−cos θ)][sin^(−1) (((sin θ)/((√3)−(1/(√3))+((cos θ)/(√3)))))]sin θdθ =((4a^2 )/9)∫_(−1) ^( 1) (2+t)sin^(−1) ((((√3)(√(1−t^2 )))/(2+t)))dt A=((4a^2 )/9)((π/(√3))+(3/2))≈1.4728a^2 . A=((2a^2 (2π(√3)+9))/(27)) . (see Q. 73689 on how to solve the integral,special thanks to MjS Sir)](Q73681.png)
$${Let}\:{P}\:\:{be}\:{a}\:{point}\:{on}\:{circumference} \\ $$$${of}\:{circle}\:\:{y}^{\mathrm{2}} +\left({z}−{r}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$${P}\:\left(\mathrm{0},\:{r}\mathrm{sin}\:\theta,\:{r}−{r}\mathrm{cos}\:\theta\right) \\ $$$${Its}\:{shadow}\:{on}\:{slant}\:{surface}\:{of}\:{cone} \\ $$$$\:{S}\left({s}\mathrm{sin}\:\alpha\mathrm{cos}\:\phi,\:{s}\mathrm{sin}\:\alpha\mathrm{sin}\:\phi,\:{a}\sqrt{\mathrm{3}}−{s}\mathrm{cos}\:\alpha\right) \\ $$$$\Rightarrow\:\:{s}\mathrm{sin}\:\alpha\mathrm{sin}\:\phi={r}\mathrm{sin}\:\theta\:\:\:\:\:\:\:\left(\alpha=\mathrm{30}°\right) \\ $$$$\:\:\:\:\:\:\:{a}\sqrt{\mathrm{3}}−{s}\mathrm{cos}\:\alpha={r}−{r}\mathrm{cos}\:\theta \\ $$$$\:\:\:\:{s}=\frac{{a}\sqrt{\mathrm{3}}−{r}\left(\mathrm{1}−\mathrm{cos}\:\theta\right)}{\mathrm{cos}\:\alpha} \\ $$$$\:\:\:\mathrm{sin}\:\phi=\frac{{r}\mathrm{sin}\:\theta\mathrm{cos}\:\alpha}{\left({a}\sqrt{\mathrm{3}}−{r}+{r}\mathrm{cos}\:\theta\right)\mathrm{sin}\:\alpha} \\ $$$$\:\:\:\mathrm{sin}\:\phi=\frac{\mathrm{sin}\:\theta}{\sqrt{\mathrm{3}}−\mathrm{1}+\mathrm{cos}\:\theta} \\ $$$$\:\:{ds}=\frac{−{r}\mathrm{sin}\:\theta{d}\theta}{\mathrm{cos}\:\alpha} \\ $$$$\:\:\frac{{s}}{\mathrm{2}{a}}=\frac{\rho}{{a}}\:\:\Rightarrow\:\:\rho=\frac{{s}}{\mathrm{2}} \\ $$$${A}=\int\rho\left(\mathrm{2}\phi\right)\left(−{ds}\right)\:=\:\int\left({s}\right)\left(−{ds}\right)\phi \\ $$$$\:\:\:=\int_{\mathrm{0}} ^{\:\:\pi} \left(\frac{{a}\sqrt{\mathrm{3}}−{r}\left(\mathrm{1}−\mathrm{cos}\:\theta\right)}{\mathrm{cos}\:\alpha}\right)\left(\frac{{r}\mathrm{sin}\:\theta{d}\theta}{\mathrm{cos}\:\alpha}\right)\left(\mathrm{sin}^{−\mathrm{1}} \left\{\frac{{r}\mathrm{sin}\:\theta\mathrm{cos}\:\alpha}{\left({a}\sqrt{\mathrm{3}}−{r}+{r}\mathrm{cos}\:\theta\right)\mathrm{sin}\:\alpha}\right\}\right) \\ $$$$=\frac{\mathrm{4}{a}^{\mathrm{2}} }{\mathrm{3}}\int_{\mathrm{0}} ^{\:\pi} \left[\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}−\mathrm{cos}\:\theta\right)\right]\left[\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{sin}\:\theta}{\sqrt{\mathrm{3}}−\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}+\frac{\mathrm{cos}\:\theta}{\sqrt{\mathrm{3}}}}\right)\right]\mathrm{sin}\:\theta{d}\theta \\ $$$$=\frac{\mathrm{4}{a}^{\mathrm{2}} }{\mathrm{9}}\int_{−\mathrm{1}} ^{\:\mathrm{1}} \left(\mathrm{2}+{t}\right)\mathrm{sin}^{−\mathrm{1}} \left(\frac{\sqrt{\mathrm{3}}\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}{\mathrm{2}+{t}}\right){dt} \\ $$$$\:\:{A}=\frac{\mathrm{4}{a}^{\mathrm{2}} }{\mathrm{9}}\left(\frac{\pi}{\sqrt{\mathrm{3}}}+\frac{\mathrm{3}}{\mathrm{2}}\right)\approx\mathrm{1}.\mathrm{4728}{a}^{\mathrm{2}} \:. \\ $$$$\:\:{A}=\frac{\mathrm{2}{a}^{\mathrm{2}} \left(\mathrm{2}\pi\sqrt{\mathrm{3}}+\mathrm{9}\right)}{\mathrm{27}}\:. \\ $$$$\left({see}\:{Q}.\:\mathrm{73689}\:{on}\:{how}\:{to}\:{solve}\right. \\ $$$${the}\:{integral},{special}\:{thanks}\:{to}\: \\ $$$$\left.\:\:{MjS}\:{Sir}\right) \\ $$
Commented by mr W last updated on 14/Nov/19
$${nice}\:{solution}\:{sir}! \\ $$
Answered by liki last updated on 14/Nov/19
Commented by ajfour last updated on 14/Nov/19
$${wrong}\:{place}\:! \\ $$