
Question and Answers Forum
Question Number 73689 by ajfour last updated on 14/Nov/19

Answered by mind is power last updated on 15/Nov/19
![(d/dx){sin^(−1) (((√(3−3x^2 ))/(2+x)))}=f(x)^′ ={((−3x)/((2+x)(√(3−3x^2 )))) −((√(3−3x^2 ))/((2+x)^2 ))}.(1/(√(1−((3−3x^2 )/((2+x)^2 ))))) ={((−3x(2+x)−(3−3x^2 ))/((2+x)^2 .(√(3−3x^2 ))))}.((2+x)/(√((2x+1)^2 )))={((−6x−3)/(∣2x+1∣))}.(1/((2+x)(√(3−3x^2 )))) =((−3sign(2x+1))/((2+x)(√(3−3x^2 )))) if xε[−(1/2),1] =((−3)/((2+x)(√(3−3x^2 )))),(3/((2+x)(√(3−3x^2 )))) ifx∈[−1,((−1)/2)] ∫_(−1) ^1 (2+x)sin^(−1) (((√(3−3x^2 ))/(2+x)))=[(2x+(x^2 /2))sin^(−1) (((√(3−3x^2 ))/(2+x)))]_(−1) ^1 −∫_(−1) ^(−(1/2)) ((4x+x^2 )/2).(3/((2+x)(√(3−3x^2 ))))−∫_(−(1/2)) ^1 ((4x+x^2 )/2).((−3dx)/((2+x)(√(3−3x^2 )))) all we need is ∫((3(x^2 +4x)dx)/((2+x)(√(3−3x^2 )))),x=sin(θ),θ∈[−(π/2),(π/2)]⇒cos(θ)≥0 ⇒∫((3(sin^2 +4sin(θ))cos(θ)dθ)/({2+sin(θ)}.cos(θ).(√3)))=∫((3(sin(θ)+2)^2 −12)/((√3)(2+sin(θ)}))dθ =(√3)∫(sin(θ)+2)−4(√3)∫(dθ/(2+sin(θ))) ,tg((θ/2))=t in2nd =−(√3)cos(θ)+2(√3)θ−4(√3)∫(2/(2+((2t)/(1+t^2 )))).(dt/(1+t^2 ))=−(√3)cos(θ)+2(√(3 θ)) −4(√3)∫(dt/(t^2 +t+1)) =−(√3)cos(θ)+2(√3)θ−8arctan((((2tg((θ/2)))/(√3))+(1/(√3)))) ∫(((x^2 +4x)dx)/((2+x)(√(3−3x^2 ))))=−(√3) (√(1−x^2 )) +2(√3)arcsin(x)−8arctan(((2tg(((arcsin(x))/2))+1)/(√3))). −{−(√3).((√3)/2)−2(√3)(π/6)−8.(π/(12)).)}+(1/2){−2(√3).(π/2)+8.(π/6)}+(1/2){2(√3).(π/2)−8.(π/3)} =(3/2)+(π/(√3))](Q73698.png)
Commented by ajfour last updated on 15/Nov/19

Commented by mind is power last updated on 15/Nov/19

Answered by MJS last updated on 15/Nov/19
![∫(2+x)arcsin ((√(3−3x^2 ))/(2+x)) dx= [t=arccos ((2x+1)/(x+2)) → dx=−(((x+2)(√(3−3x^2 )))/3)dt] (1) for 0≤t≤(π/2) =−9∫((t sin t)/((2−cos t)^3 ))dt= [((∫u′v=uv−∫uv′)),((u′=((sin t)/((2−cos t)^3 )) ⇒ u=−(1/(2(2−cos t)^2 )))),((v=t ⇒ v′=1)) ] =((9t)/(2(2−cos t)^2 ))−(9/2)∫(dt/((2−cos t)^2 ))= ∫(dt/((2−cos t)^2 ))= [w=tan (t/2) → dt=((2dw)/(w^2 +1))] =2∫((w^2 +1)/((3w^2 +1)^2 ))dw= =((2w)/(3(3w^2 +1)))+((4(√3))/9)arctan (√3)w = =((sin t)/(3(2−cos t)))+((4(√3))/9)arctan ((√3)tan (t/2)) =((9t)/(2(2−cos t)^2 ))−((3sin t)/(2(2−cos t)))−2(√3)arctan ((√3)tan (t/2)) (2) for (π/2)≤t≤π =−9π∫((sin t)/((2−cos t)^3 ))dt+9∫((t sin t)/((2−cos t)^3 ))dt= =((9π)/(2(2−cos t)^2 ))−(the above) ⇒ ∫_(−1) ^1 (2+x)arcsin ((√(3−3x^2 ))/(2+x)) dx= =((√3)/3)π+(3/2)](Q73714.png)
Commented by ajfour last updated on 15/Nov/19

Commented by ajfour last updated on 15/Nov/19
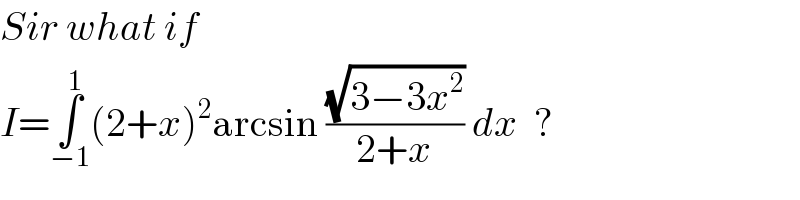
Commented by MJS last updated on 15/Nov/19
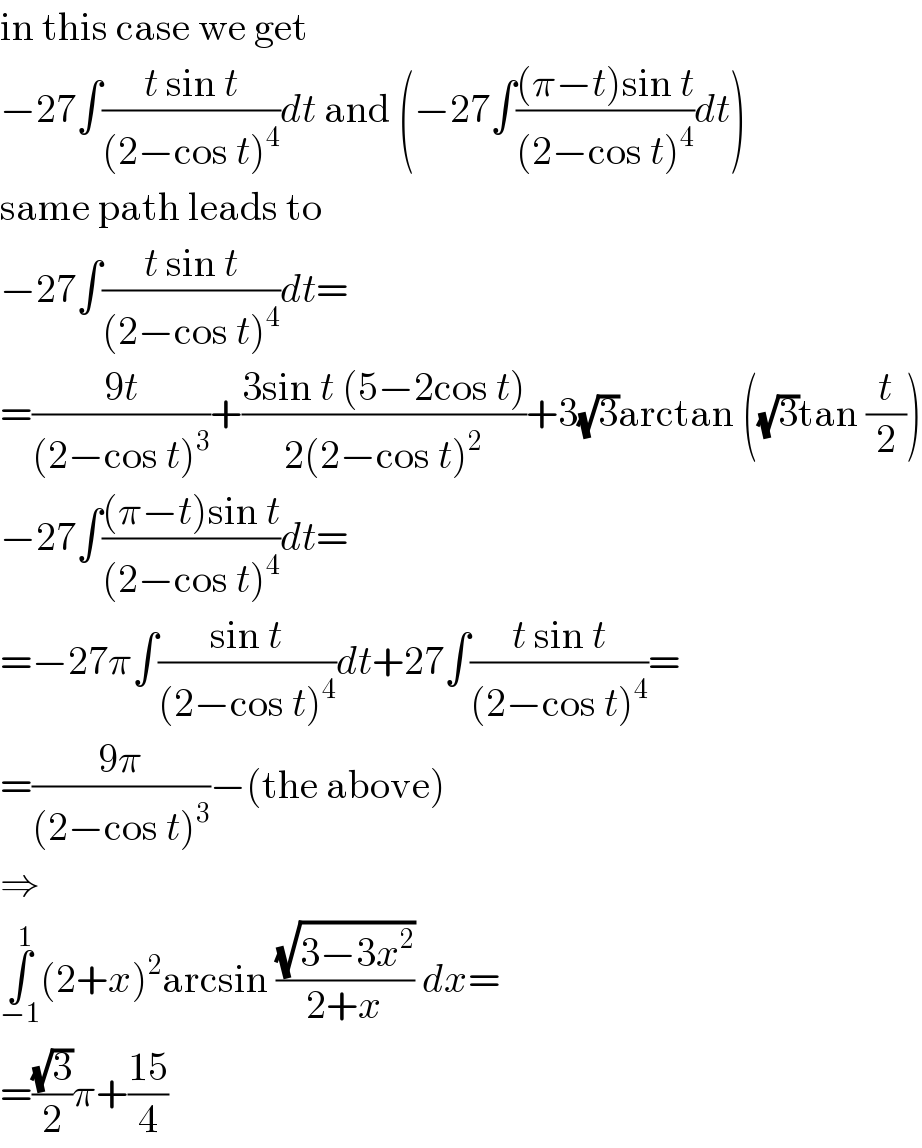
Commented by ajfour last updated on 15/Nov/19

Commented by MJS last updated on 15/Nov/19

