Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 73697 by mathmax by abdo last updated on 14/Nov/19

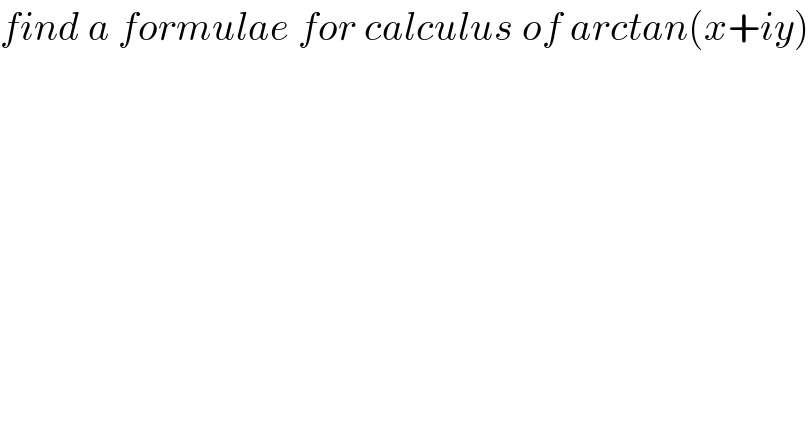
find a formulae for calculus of arctan(x+iy)

$${find}\:{a}\:{formulae}\:{for}\:{calculus}\:{of}\:{arctan}\left({x}+{iy}\right) \\ $$

Commented by mathmax by abdo last updated on 15/Nov/19

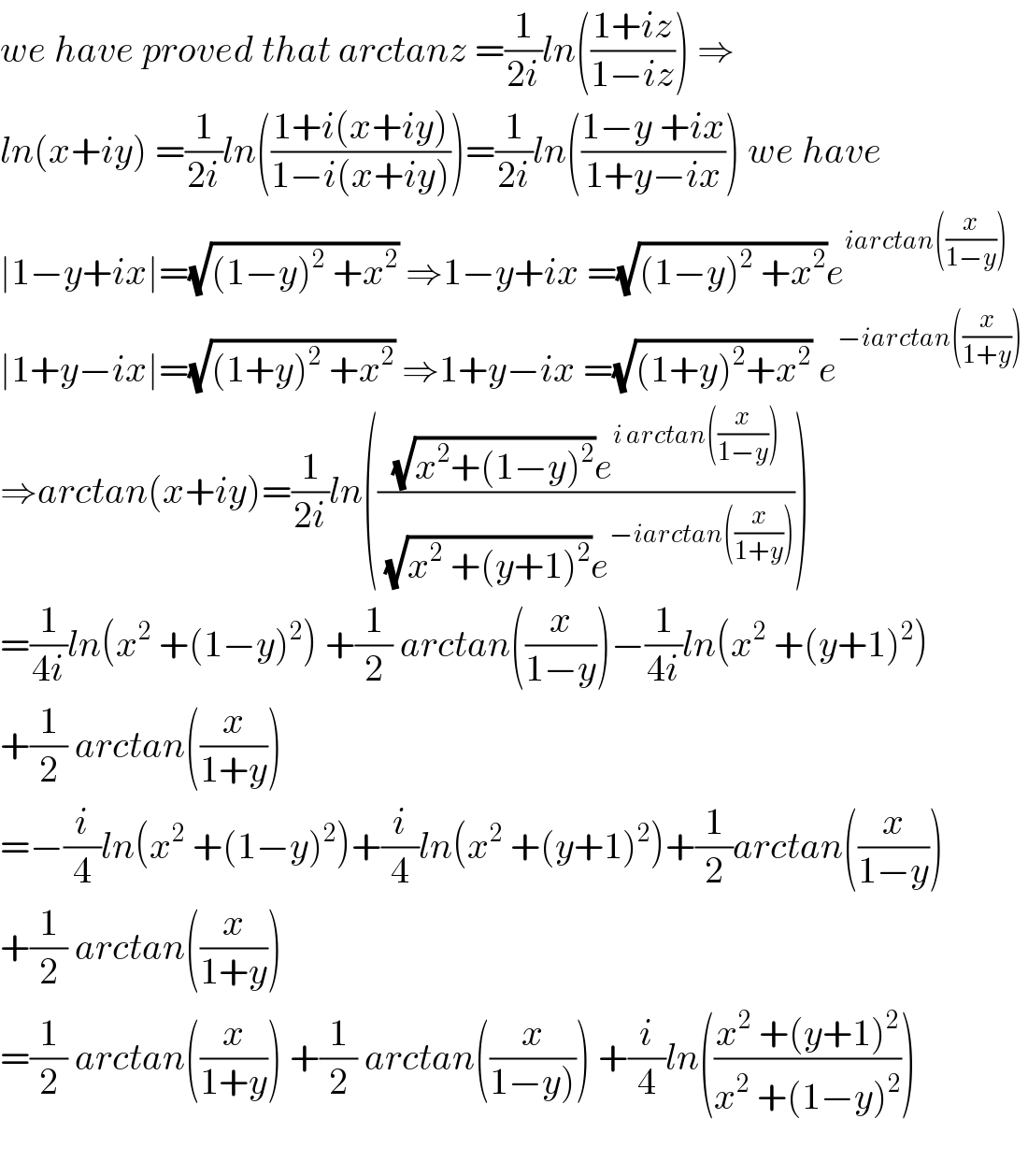
we have proved that arctanz =(1/(2i))ln(((1+iz)/(1−iz))) ⇒ ln(x+iy) =(1/(2i))ln(((1+i(x+iy))/(1−i(x+iy))))=(1/(2i))ln(((1−y +ix)/(1+y−ix))) we have ∣1−y+ix∣=(√((1−y)^2 +x^2 )) ⇒1−y+ix =(√((1−y)^2 +x^2 ))e^(iarctan((x/(1−y)))) ∣1+y−ix∣=(√((1+y)^2 +x^2 )) ⇒1+y−ix =(√((1+y)^2 +x^2 )) e^(−iarctan((x/(1+y))) ) ⇒arctan(x+iy)=(1/(2i))ln((((√(x^2 +(1−y)^2 ))e^(i arctan((x/(1−y)))) )/((√(x^2 +(y+1)^2 ))e^(−iarctan((x/(1+y)))) ))) =(1/(4i))ln(x^2 +(1−y)^2 ) +(1/2) arctan((x/(1−y)))−(1/(4i))ln(x^2 +(y+1)^2 ) +(1/2) arctan((x/(1+y))) =−(i/4)ln(x^2 +(1−y)^2 )+(i/4)ln(x^2 +(y+1)^2 )+(1/2)arctan((x/(1−y))) +(1/2) arctan((x/(1+y))) =(1/2) arctan((x/(1+y))) +(1/2) arctan((x/(1−y)))) +(i/4)ln(((x^2 +(y+1)^2 )/(x^2 +(1−y)^2 )))

$${we}\:{have}\:{proved}\:{that}\:{arctanz}\:=\frac{\mathrm{1}}{\mathrm{2}{i}}{ln}\left(\frac{\mathrm{1}+{iz}}{\mathrm{1}−{iz}}\right)\:\Rightarrow \\ $$$${ln}\left({x}+{iy}\right)\:=\frac{\mathrm{1}}{\mathrm{2}{i}}{ln}\left(\frac{\mathrm{1}+{i}\left({x}+{iy}\right)}{\mathrm{1}−{i}\left({x}+{iy}\right)}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}{ln}\left(\frac{\mathrm{1}−{y}\:+{ix}}{\mathrm{1}+{y}−{ix}}\right)\:{we}\:{have} \\ $$$$\mid\mathrm{1}−{y}+{ix}\mid=\sqrt{\left(\mathrm{1}−{y}\right)^{\mathrm{2}} \:+{x}^{\mathrm{2}} }\:\Rightarrow\mathrm{1}−{y}+{ix}\:=\sqrt{\left(\mathrm{1}−{y}\right)^{\mathrm{2}} \:+{x}^{\mathrm{2}} }{e}^{{iarctan}\left(\frac{{x}}{\mathrm{1}−{y}}\right)} \\ $$$$\mid\mathrm{1}+{y}−{ix}\mid=\sqrt{\left(\mathrm{1}+{y}\right)^{\mathrm{2}} \:+{x}^{\mathrm{2}} }\:\Rightarrow\mathrm{1}+{y}−{ix}\:=\sqrt{\left(\mathrm{1}+{y}\right)^{\mathrm{2}} +{x}^{\mathrm{2}} }\:{e}^{−{iarctan}\left(\frac{{x}}{\mathrm{1}+{y}}\right)\:} \\ $$$$\Rightarrow{arctan}\left({x}+{iy}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}{ln}\left(\frac{\sqrt{{x}^{\mathrm{2}} +\left(\mathrm{1}−{y}\right)^{\mathrm{2}} }{e}^{{i}\:{arctan}\left(\frac{{x}}{\mathrm{1}−{y}}\right)} }{\sqrt{{x}^{\mathrm{2}} \:+\left({y}+\mathrm{1}\right)^{\mathrm{2}} }{e}^{−{iarctan}\left(\frac{{x}}{\mathrm{1}+{y}}\right)} }\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left({x}^{\mathrm{2}} \:+\left(\mathrm{1}−{y}\right)^{\mathrm{2}} \right)\:+\frac{\mathrm{1}}{\mathrm{2}}\:{arctan}\left(\frac{{x}}{\mathrm{1}−{y}}\right)−\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left({x}^{\mathrm{2}} \:+\left({y}+\mathrm{1}\right)^{\mathrm{2}} \right) \\ $$$$+\frac{\mathrm{1}}{\mathrm{2}}\:{arctan}\left(\frac{{x}}{\mathrm{1}+{y}}\right) \\ $$$$=−\frac{{i}}{\mathrm{4}}{ln}\left({x}^{\mathrm{2}} \:+\left(\mathrm{1}−{y}\right)^{\mathrm{2}} \right)+\frac{{i}}{\mathrm{4}}{ln}\left({x}^{\mathrm{2}} \:+\left({y}+\mathrm{1}\right)^{\mathrm{2}} \right)+\frac{\mathrm{1}}{\mathrm{2}}{arctan}\left(\frac{{x}}{\mathrm{1}−{y}}\right) \\ $$$$+\frac{\mathrm{1}}{\mathrm{2}}\:{arctan}\left(\frac{{x}}{\mathrm{1}+{y}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:{arctan}\left(\frac{{x}}{\mathrm{1}+{y}}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\:{arctan}\left(\frac{{x}}{\left.\mathrm{1}−{y}\right)}\right)\:+\frac{{i}}{\mathrm{4}}{ln}\left(\frac{{x}^{\mathrm{2}} \:+\left({y}+\mathrm{1}\right)^{\mathrm{2}} }{{x}^{\mathrm{2}} \:+\left(\mathrm{1}−{y}\right)^{\mathrm{2}} }\right) \\ $$$$ \\ $$

Answered by Smail last updated on 15/Nov/19

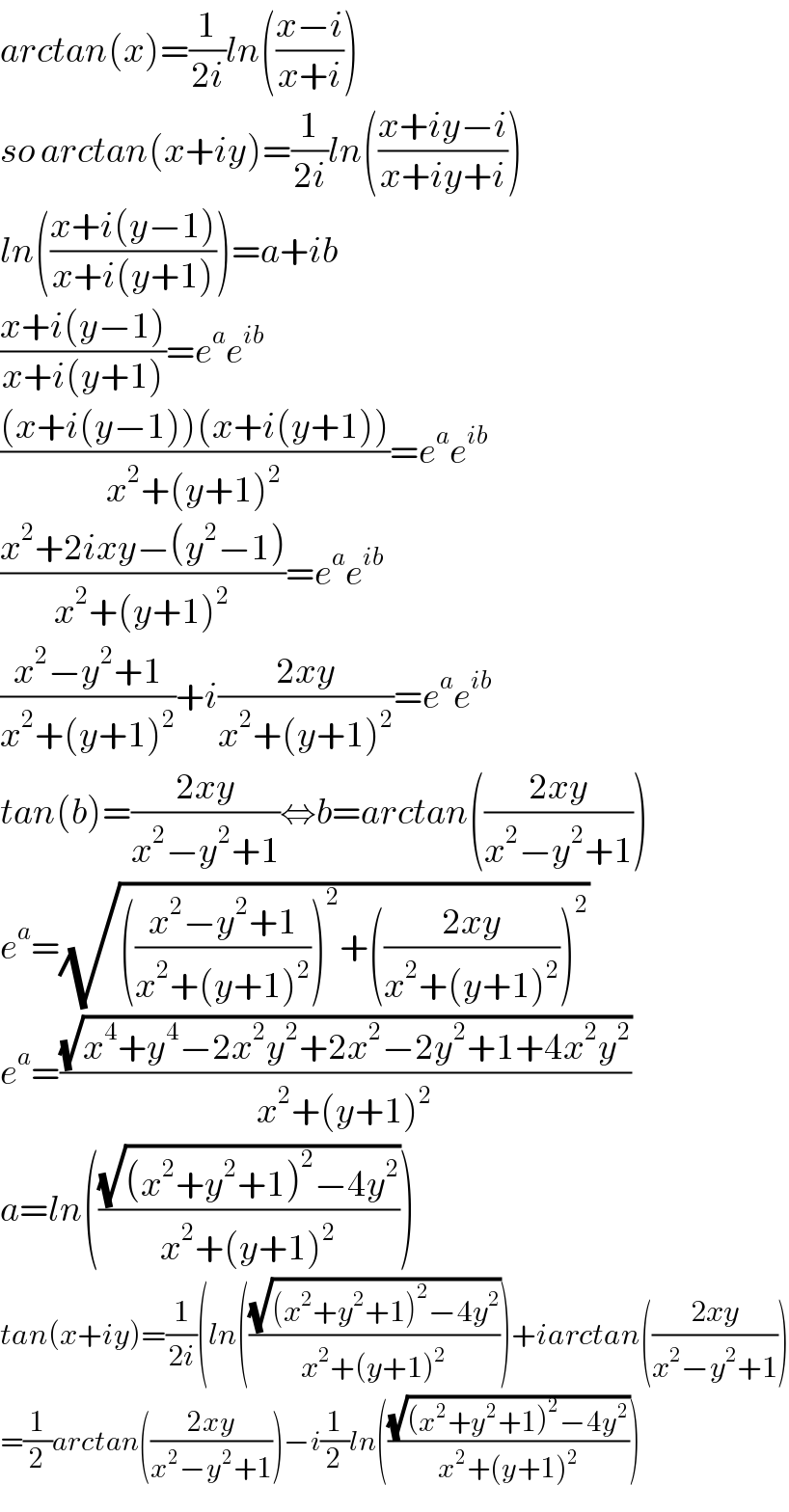
arctan(x)=(1/(2i))ln(((x−i)/(x+i))) so arctan(x+iy)=(1/(2i))ln(((x+iy−i)/(x+iy+i))) ln(((x+i(y−1))/(x+i(y+1))))=a+ib ((x+i(y−1))/(x+i(y+1)))=e^a e^(ib) (((x+i(y−1))(x+i(y+1)))/(x^2 +(y+1)^2 ))=e^a e^(ib) ((x^2 +2ixy−(y^2 −1))/(x^2 +(y+1)^2 ))=e^a e^(ib) ((x^2 −y^2 +1)/(x^2 +(y+1)^2 ))+i((2xy)/(x^2 +(y+1)^2 ))=e^a e^(ib) tan(b)=((2xy)/(x^2 −y^2 +1))⇔b=arctan(((2xy)/(x^2 −y^2 +1))) e^a =(√((((x^2 −y^2 +1)/(x^2 +(y+1)^2 )))^2 +(((2xy)/(x^2 +(y+1)^2 )))^2 )) e^a =((√(x^4 +y^4 −2x^2 y^2 +2x^2 −2y^2 +1+4x^2 y^2 ))/(x^2 +(y+1)^2 )) a=ln(((√((x^2 +y^2 +1)^2 −4y^2 ))/(x^2 +(y+1)^2 ))) tan(x+iy)=(1/(2i))(ln(((√((x^2 +y^2 +1)^2 −4y^2 ))/(x^2 +(y+1)^2 )))+iarctan(((2xy)/(x^2 −y^2 +1))) =(1/2)arctan(((2xy)/(x^2 −y^2 +1)))−i(1/2)ln(((√((x^2 +y^2 +1)^2 −4y^2 ))/(x^2 +(y+1)^2 )))

$${arctan}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}{ln}\left(\frac{{x}−{i}}{{x}+{i}}\right) \\ $$$${so}\:{arctan}\left({x}+{iy}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}{ln}\left(\frac{{x}+{iy}−{i}}{{x}+{iy}+{i}}\right) \\ $$$${ln}\left(\frac{{x}+{i}\left({y}−\mathrm{1}\right)}{{x}+{i}\left({y}+\mathrm{1}\right)}\right)={a}+{ib} \\ $$$$\frac{{x}+{i}\left({y}−\mathrm{1}\right)}{{x}+{i}\left({y}+\mathrm{1}\right)}={e}^{{a}} {e}^{{ib}} \\ $$$$\frac{\left({x}+{i}\left({y}−\mathrm{1}\right)\right)\left({x}+{i}\left({y}+\mathrm{1}\right)\right)}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }={e}^{{a}} {e}^{{ib}} \\ $$$$\frac{{x}^{\mathrm{2}} +\mathrm{2}{ixy}−\left({y}^{\mathrm{2}} −\mathrm{1}\right)}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }={e}^{{a}} {e}^{{ib}} \\ $$$$\frac{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{1}}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }+{i}\frac{\mathrm{2}{xy}}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }={e}^{{a}} {e}^{{ib}} \\ $$$${tan}\left({b}\right)=\frac{\mathrm{2}{xy}}{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{1}}\Leftrightarrow{b}={arctan}\left(\frac{\mathrm{2}{xy}}{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$${e}^{{a}} =\sqrt{\left(\frac{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{1}}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }\right)^{\mathrm{2}} +\left(\frac{\mathrm{2}{xy}}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }\right)^{\mathrm{2}} } \\ $$$${e}^{{a}} =\frac{\sqrt{{x}^{\mathrm{4}} +{y}^{\mathrm{4}} −\mathrm{2}{x}^{\mathrm{2}} {y}^{\mathrm{2}} +\mathrm{2}{x}^{\mathrm{2}} −\mathrm{2}{y}^{\mathrm{2}} +\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} {y}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${a}={ln}\left(\frac{\sqrt{\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{4}{y}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }\right) \\ $$$${tan}\left({x}+{iy}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}}\left({ln}\left(\frac{\sqrt{\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{4}{y}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }\right)+{iarctan}\left(\frac{\mathrm{2}{xy}}{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{1}}\right)\right. \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{arctan}\left(\frac{\mathrm{2}{xy}}{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{1}}\right)−{i}\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\sqrt{\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{4}{y}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} }\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com