Question Number 73918 by arkanmath7@gmail.com last updated on 16/Nov/19
$${find}\:{the}\:{Re}\left({w}\right)\:{and}\:{Im}\left({w}\right) \\ $$$$ \\ $$$${where}\:{w}\:=\:\left({sin}\:{a}\:+\:{icos}\:{a}\right)^{\left({cos}\:{a}\:+\:{isin}\:{a}\right)} \\ $$
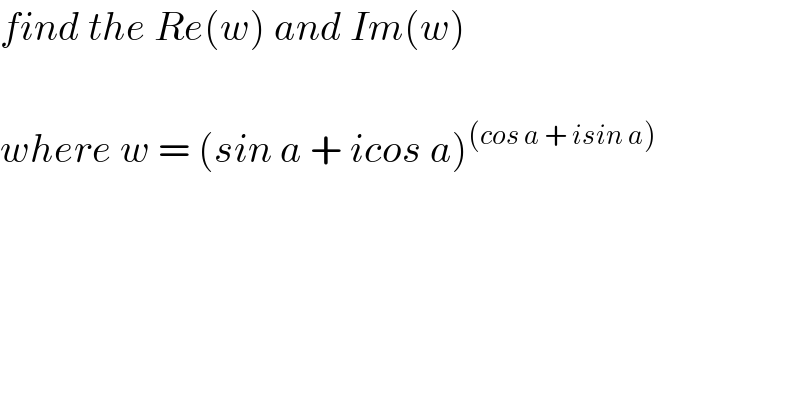
Commented by abdomathmax last updated on 16/Nov/19
$${W}=\left({e}^{{i}\left(\frac{\pi}{\mathrm{2}}−{a}\right)} \right)^{{cosa}\:+{isina}} ={e}^{{i}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\left({cosa}\:+{isina}\right)} \\ $$$$={e}^{{i}\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}−\left(\frac{\pi}{\mathrm{2}}−{a}\right){sina}} \\ $$$$={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} \:×{e}^{{i}\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}} \\ $$$$={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} ×\:\left\{{cos}\left\{\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}\right\}+{isin}\left\{\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}\right\}\right. \\ $$$$\Rightarrow{Re}\left({W}\right)={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} .\:{cos}\left\{\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}\right\} \\ $$$${Im}\left({W}\right)={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} .{sin}\left\{\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}\right\} \\ $$
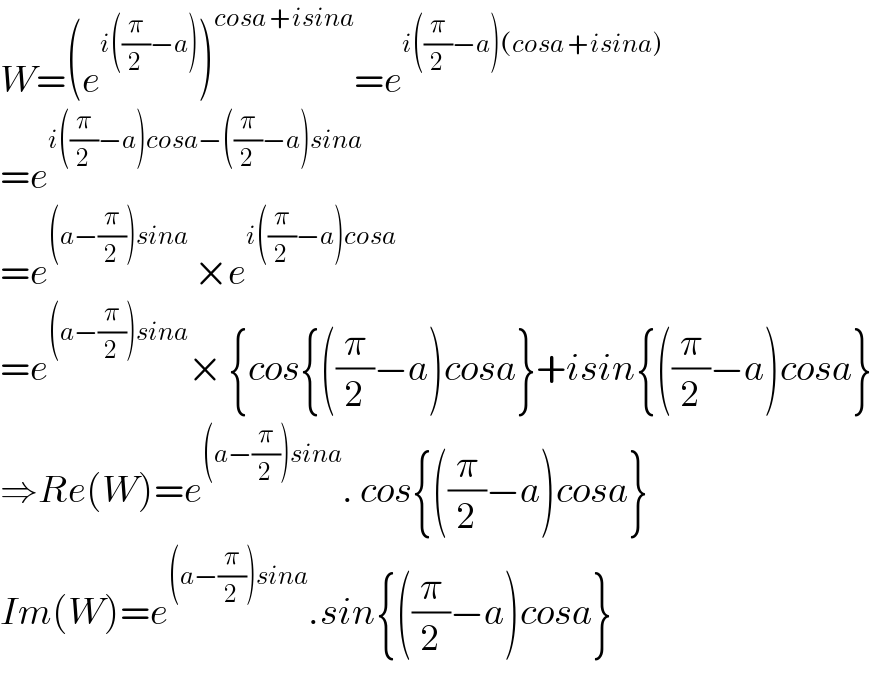
Commented by arkanmath7@gmail.com last updated on 18/Nov/19
$${thnx}\:{sir} \\ $$
Answered by Tanmay chaudhury last updated on 16/Nov/19
![(A+iB)^(P+iQ) =e^((P+iQ)Log(A+iB)) let A=rcosθ B=rsinθ so A+iB=re^(iθ) =e^(i(2nπ+θ)) r=(√(A^2 +B^2 )) tanθ=(B/A)⇛ θ=tan^− ((B/A)) e^((P+iQ)Log(re^(i(2nπ+θ)) )) =e^((P+iQ)[log(√(A^2 +B^2 )) +i(2nπ+tan^(−1) ((B/A))]) e^([P.log(√(A^2 +B^2 )) +i(2nπP+Ptan^(−1) ((B/A))+log(√(A^2 +B^2 )) )−Q(2nπ+tan^(−1) ((B/A)))) =e^([P.log(√(A^2 +B^2 )) −Q(2nπ+tan^(−1) ((B/A)))) ×e^(i(2nπP+Ptan^(−1) ((B/A))+log(√(A^2 +B^2 )) )) now back to problem A=sina B=cosa P=cosa Q=sina log(√(A^2 +B^2 )) =(1/2)log(sin^2 a+cos^2 a)=0 tan^(−1) ((B/A))=tan^(−1) (((cosa)/(sina)))=tan^(−1) (tan((π/2)−a))=(π/2)−a so putting the value =e^([P.0−sina(2nπ+(π/2)−)]) ×e^(i(2nπ.cosa+cosa.((π/2)−a)) =e^([−(2nπ+(π/2))sina) ×[cos(2nπ.cosa+((π/2)−a)cosa)+ sin(2nπ.cosa+((π/2)−a)cosa] pls check upto this...](Q73922.png)
$$\left({A}+{iB}\right)^{{P}+{iQ}} ={e}^{\left({P}+{iQ}\right){Log}\left({A}+{iB}\right)} \\ $$$${let}\:{A}={rcos}\theta\:\:\:{B}={rsin}\theta\:\:\:{so}\:{A}+{iB}={re}^{{i}\theta} =\boldsymbol{{e}}^{\boldsymbol{{i}}\left(\mathrm{2}\boldsymbol{{n}}\pi+\theta\right)} \\ $$$${r}=\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:\:\:{tan}\theta=\frac{{B}}{{A}}\Rrightarrow\:\:\:\theta={tan}^{−} \left(\frac{{B}}{{A}}\right) \\ $$$${e}^{\left({P}+{iQ}\right){Log}\left({re}^{{i}\left(\mathrm{2}{n}\pi+\theta\right)} \right)} \\ $$$$={e}^{\left({P}+{iQ}\right)\left[{log}\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:+{i}\left(\mathrm{2}{n}\pi+{tan}^{−\mathrm{1}} \left(\frac{{B}}{{A}}\right)\right]\right.} \\ $$$${e}^{\left[{P}.{log}\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:+{i}\left(\mathrm{2}{n}\pi{P}+{Ptan}^{−\mathrm{1}} \left(\frac{{B}}{{A}}\right)+{log}\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:\right)−{Q}\left(\mathrm{2}{n}\pi+{tan}^{−\mathrm{1}} \left(\frac{{B}}{{A}}\right)\right)\right.} \\ $$$$={e}^{\left[{P}.{log}\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:−{Q}\left(\mathrm{2}{n}\pi+{tan}^{−\mathrm{1}} \left(\frac{{B}}{{A}}\right)\right)\right.} ×{e}^{{i}\left(\mathrm{2}{n}\pi{P}+{Ptan}^{−\mathrm{1}} \left(\frac{{B}}{{A}}\right)+{log}\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:\right)} \\ $$$${now}\:{back}\:{to}\:{problem}\:\: \\ $$$${A}={sina}\:\:\:{B}={cosa}\:\:\:{P}={cosa}\:\:\:{Q}={sina} \\ $$$${log}\sqrt{{A}^{\mathrm{2}} +{B}^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{2}}{log}\left({sin}^{\mathrm{2}} {a}+{cos}^{\mathrm{2}} {a}\right)=\mathrm{0} \\ $$$${tan}^{−\mathrm{1}} \left(\frac{{B}}{{A}}\right)={tan}^{−\mathrm{1}} \left(\frac{{cosa}}{{sina}}\right)={tan}^{−\mathrm{1}} \left({tan}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right)=\frac{\pi}{\mathrm{2}}−{a} \\ $$$${so}\:\:{putting}\:{the}\:{value} \\ $$$$={e}^{\left[{P}.\mathrm{0}−{sina}\left(\mathrm{2}{n}\pi+\frac{\pi}{\mathrm{2}}−\right)\right]} ×{e}^{{i}\left(\mathrm{2}{n}\pi.{cosa}+{cosa}.\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right.} \\ $$$$={e}^{\left[−\left(\mathrm{2}{n}\pi+\frac{\pi}{\mathrm{2}}\right){sina}\right.} ×\left[{cos}\left(\mathrm{2}{n}\pi.{cosa}+\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}\right)+\right. \\ $$$${sin}\left(\mathrm{2}{n}\pi.{cosa}+\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}\right] \\ $$$$\boldsymbol{{pls}}\:\boldsymbol{{check}}\:\boldsymbol{{upto}}\:\boldsymbol{{this}}... \\ $$$$ \\ $$
Commented by arkanmath7@gmail.com last updated on 16/Nov/19
$${thnx}\:{alot}.\:{it}'{s}\:{a}\:{long}\:{sol},\:{u}\:{mst}\:{be}\:{tired}\:{now} \\ $$$${thnx}\:{again} \\ $$
Commented by Tanmay chaudhury last updated on 16/Nov/19
$${no}\:\:{i}\:{have}\:{solved}\:{in}\:{general}\:{way}\:{not}\:{directly} \\ $$$$\left({A}+{iB}\right)^{{P}+{iQ}} \:\:{format}.. \\ $$$${i}\:{can}\:{solve}\:{the}\:{given}\:{problem}\:{direcly}.. \\ $$$${but}\:{solved}\:\left({A}+{iB}\right)^{{P}+{iQ}} \:{format}\:{for}\:{help}\:{in}\:{other} \\ $$$${problem} \\ $$
Answered by Tanmay chaudhury last updated on 16/Nov/19
![short method [cos((π/2)−a)+isin((π/2)−a)]^((cosa+isina)) =e^(i((π/2)−a)(cosa+isina)) =e^((a−(π/2))sina+i((π/2)−a)cosa) =e^((a−(π/2))sina) ×[cos{cosa((π/2)−a)}+isin{cosa((π/2)−a)}] =e^((a−(π/2))sina) cos{cosa((π/2)−a)}+i e^((a−(π/2))sina) sin{cosa((π/2)−a)}](Q73935.png)
$${short}\:{method} \\ $$$$\left[{cos}\left(\frac{\pi}{\mathrm{2}}−{a}\right)+{isin}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right]^{\left({cosa}+{isina}\right)} \\ $$$$={e}^{{i}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\left({cosa}+{isina}\right)} \\ $$$$={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}+{i}\left(\frac{\pi}{\mathrm{2}}−{a}\right){cosa}} \\ $$$$={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} ×\left[{cos}\left\{{cosa}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right\}+{isin}\left\{{cosa}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right\}\right] \\ $$$$={e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} {cos}\left\{{cosa}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right\}+{i}\:{e}^{\left({a}−\frac{\pi}{\mathrm{2}}\right){sina}} {sin}\left\{{cosa}\left(\frac{\pi}{\mathrm{2}}−{a}\right)\right\} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by peter frank last updated on 17/Nov/19
$${thanks} \\ $$