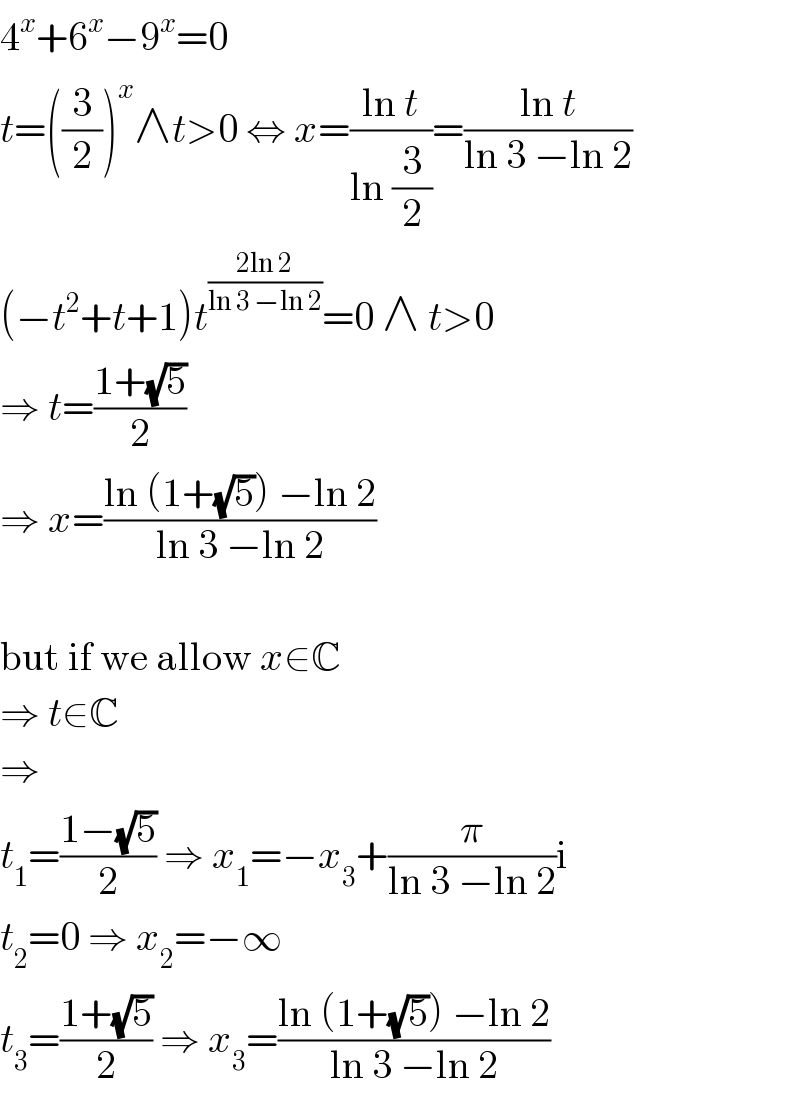Math Question and Answers


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 74168 by mr W last updated on 19/Nov/19

Commented by mr W last updated on 19/Nov/19

$s o l v e f o r x .$
	Answered by Rio Michael last updated on 19/Nov/19

	$d i v i d i n g b y 4^{x},$ $1 + {(\frac{6}{4})}^{x} = {(\frac{9}{4})}^{x}$ $1 + {(\frac{3}{2})}^{x} = {(\frac{3}{2})}^{2 x}$ $l e t {(\frac{3}{2})}^{x} = a$ $\Rightarrow 1 + a = a^{2}$ $a^{2} - a - 1 = 0$ $a = \frac{1 \pm \sqrt{5}}{2}$ $o r {(\frac{3}{2})}^{x} = \frac{1 \pm \sqrt{5}}{2}$ $x (l o g \frac{3}{2}) = l o g [\frac{1 \pm \sqrt{5}}{2}]$ $x = \frac{l o g [\frac{1 \pm \sqrt{5}}{2}]}{l o g \frac{3}{2}}$ $x \approx 1.2$
	Answered by Maclaurin Stickker last updated on 19/Nov/19
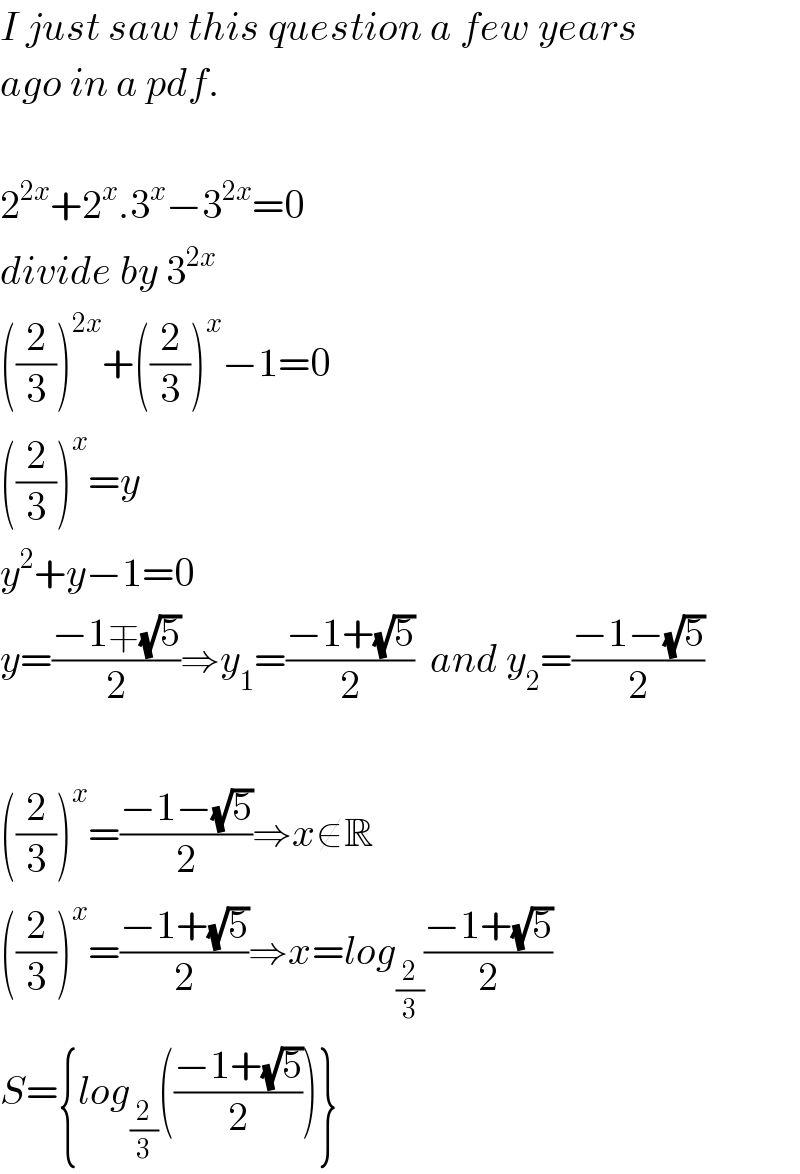
	$I just saw this question a few years ago in a pdf. 2^(2x) +2^x .3^x −3^(2x) =0 divide by 3^(2x) ((2/3))^(2x) +((2/3))^x −1=0 ((2/3))^x =y y^2 +y−1=0 y=((−1∓(√5))/2)⇒y_1 =((−1+(√5))/2) and y_2 =((−1−(√5))/2) ((2/3))^x =((−1−(√5))/2)⇒x∉R ((2/3))^x =((−1+(√5))/2)⇒x=log_(2/3) ((−1+(√5))/2) S={log_(2/3) (((−1+(√5))/2))}$
	$I j u s t s a w t h i s q u e s t i o n a f e w y e a r s$ $a g o i n a p d f .$ $2^{2 x} + 2^{x} . 3^{x} - 3^{2 x} = 0$ $d i v i d e b y 3^{2 x}$ ${(\frac{2}{3})}^{2 x} + {(\frac{2}{3})}^{x} - 1 = 0$ ${(\frac{2}{3})}^{x} = y$ $y^{2} + y - 1 = 0$ $y = \frac{- 1 \mp \sqrt{5}}{2} \Rightarrow y_{1} = \frac{- 1 + \sqrt{5}}{2} a n d y_{2} = \frac{- 1 - \sqrt{5}}{2}$ ${(\frac{2}{3})}^{x} = \frac{- 1 - \sqrt{5}}{2} \Rightarrow x \notin R$ ${(\frac{2}{3})}^{x} = \frac{- 1 + \sqrt{5}}{2} \Rightarrow x = {l o g}_{\frac{2}{3}} \frac{- 1 + \sqrt{5}}{2}$ $S = {{l o g}_{\frac{2}{3}} (\frac{- 1 + \sqrt{5}}{2})}$
	Commented by Maclaurin Stickker last updated on 19/Nov/19

	$I s t h e r e a n o t h e r s o l u t i o n t o t h e p r o b l e m ?$
	Commented by malwaan last updated on 20/Nov/19

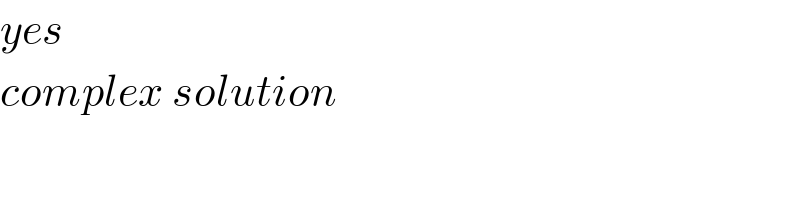
	$y e s$ $c o m p l e x s o l u t i o n$
	Answered by MJS last updated on 20/Nov/19

	$4^{x} + 6^{x} - 9^{x} = 0$ $t = {(\frac{3}{2})}^{x} \land t > 0 \Leftrightarrow x = \frac{\ln t}{\ln \frac{3}{2}} = \frac{\ln t}{\ln 3 - \ln 2}$ $(- t^{2} + t + 1) t^{\frac{2 \ln 2}{\ln 3 - \ln 2}} = 0 \land t > 0$ $\Rightarrow t = \frac{1 + \sqrt{5}}{2}$ $\Rightarrow x = \frac{\ln (1 + \sqrt{5}) - \ln 2}{\ln 3 - \ln 2}$ $but if we allow x \in C$ $\Rightarrow t \in C$ $\Rightarrow$ $t_{1} = \frac{1 - \sqrt{5}}{2} \Rightarrow x_{1} = - x_{3} + \frac{π}{\ln 3 - \ln 2} i$ $t_{2} = 0 \Rightarrow x_{2} = - \infty$ $t_{3} = \frac{1 + \sqrt{5}}{2} \Rightarrow x_{3} = \frac{\ln (1 + \sqrt{5}) - \ln 2}{\ln 3 - \ln 2}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum