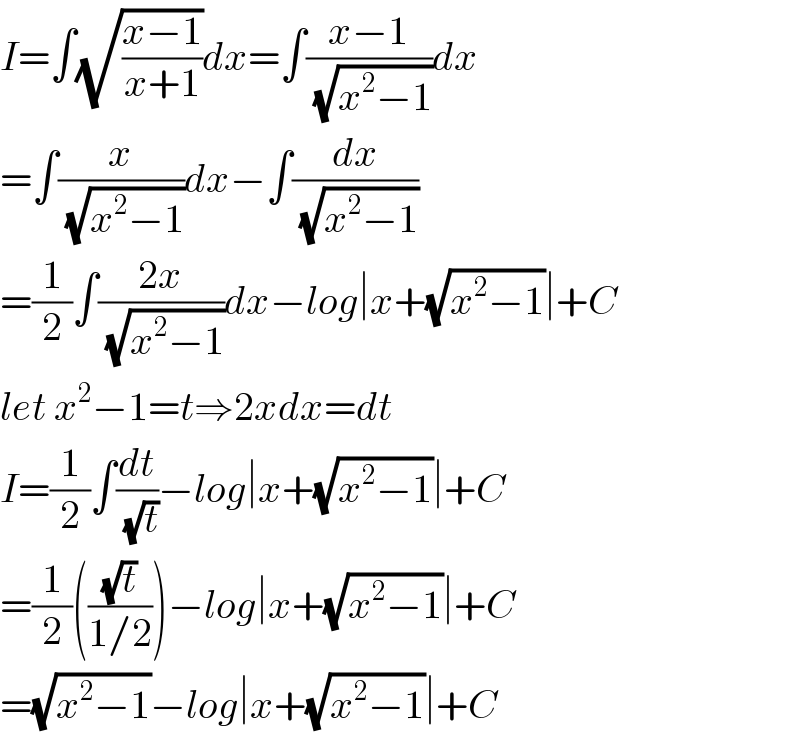Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 74720 by chess1 last updated on 29/Nov/19

Commented by mathmax by abdo last updated on 29/Nov/19

$$changement\sqrt{\frac{x-1}{x+1}}=tgivex-1=t^2(x+1)\Rightarrow (1-t^2)x=1+t^2\Rightarrow$$$$x=\frac{1+t^2}{1-t^2}\Rightarrow \frac{dx}{dt}=\frac{2t(1-t^2)-(1+t^2)(-2t)}{{(1-t^2)}^2}=\frac{2t-2t^3+2t+2t^3}{{(t^2-1)}^2}$$$$=\frac{4t}{{(t^2-1)}^2}\Rightarrow \int \sqrt{\frac{x-1}{x+1}}dx=4\int \frac{t^2}{{(t^2-1)}^2}dtletdecompose$$$$F\left(t\right)=\frac{t^2}{{(t^2-1)}^2}\Rightarrow F\left(t\right)=\frac{t^2}{{(t+1)}^2{(t-1)}^2}$$$$=\frac{a}{t+1}+\frac{b}{{(t+1)}^2}+\frac{c}{t-1}+\frac{d}{{(t-1)}^2}$$$$F(-t)=F\left(t\right)\Rightarrow \frac{-a}{t-1}+\frac{b}{{(t-1)}^2}-\frac{c}{t+1}+\frac{d}{{(t+1)}^2}=\frac{a}{t+1}+\frac{b}{{(t+1)}^2}$$$$+\frac{c}{t-1}+\frac{d}{{(t-1)}^2}\Rightarrow c=-aandb=d\Rightarrow$$$$F\left(t\right)=\frac{a}{t+1}+\frac{b}{{(t+1)}^2}-\frac{a}{t-1}+\frac{b}{{(t-1)}^2}$$$$b={(t+1)}^{2}F\left(t\right){\mid }_{t=-1}=\frac{1}{4}\Rightarrow F\left(t\right)=\frac{a}{t+1}+\frac{1}{4{(t+1)}^2}-\frac{a}{t-1}+\frac{1}{4{(t-1)}^2}$$$$F\left(0\right)=0=a+\frac{1}{4}+a+\frac{1}{4}=2a+\frac{1}{2}\Rightarrow 2a=-\frac{1}{2}\Rightarrow a=-\frac{1}{4}\Rightarrow$$$$F\left(t\right)=\frac{1}{4(t-1)}-\frac{1}{4(t+1)}+\frac{1}{4{(t+1)}^2}+\frac{1}{4{(t-1)}^2}\Rightarrow$$$$\int \sqrt{\frac{x-1}{x+1}}dx=\int \frac{dt}{t-1}-\int \frac{dt}{t+1}+\int \frac{dt}{{(t+1)}^2}+\int \frac{dt}{{(t-1)}^2}$$$$=ln\mid t-1\mid -ln\mid t+1\mid -\frac{1}{t+1}-\frac{1}{t-1}+C$$$$=ln\mid \frac{t-1}{t+1}\mid -(\frac{1}{t+1}+\frac{1}{t-1})+C=ln\mid \frac{t-1}{t+1}\mid -\frac{2t}{t^2-1}+C$$$$=ln\mid \frac{\sqrt{\frac{x-1}{x+1}}-1}{\sqrt{\frac{x-1}{x+1}}+1}\mid -\frac{2\sqrt{\frac{x-1}{x+1}}}{\frac{x-1}{x+1}-1}+C$$$$=ln\mid \frac{\sqrt{\frac{x-1}{x+1}}-1}{\sqrt{\frac{x-1}{x+1}}+1}\mid +(x+1)\sqrt{\frac{x-1}{x+1}}+C.$$$$$$$$$$

Commented by mathmax by abdo last updated on 29/Nov/19

$$wecansimplifyweget$$$$\int \sqrt{\frac{x-1}{x+1}}dx=ln\mid \frac{\sqrt{x-1}-\sqrt{x+1}}{\sqrt{x-1}+\sqrt{x+1}}\mid +\sqrt{x^2-1}+C.$$

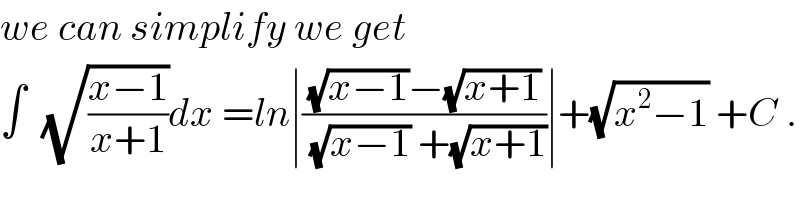
Commented by chess1 last updated on 30/Nov/19

$$\mathrm{thanks}$$

Commented by mathmax by abdo last updated on 30/Nov/19

$$youarewelcome.$$

Commented by mathmax by abdo last updated on 06/Dec/19

$$anotherwayweusethechangementx=ch\left(2t\right)\Rightarrow$$$$I=\int \sqrt{\frac{ch\left(2t\right)-1}{ch\left(2t\right)+1}}2sh\left(2t\right)dt=2\int \sqrt{\frac{2{sh}^2\left(t\right)}{2{ch}^2\left(t\right)}}ch\left(2t\right)dt$$$$=2\int th\left(t\right)2sh\left(t\right)ch\left(t\right)dt=4\int \frac{sh\left(t\right)}{ch\left(t\right)}sh\left(t\right)ch\left(t\right)dt$$$$=4\int {sh}^{2}tdt=4\int \frac{ch\left(2t\right)-1}{2}dt=2\int ch\left(2t\right)dt-2t$$$$=sh\left(2t\right)-2t+c=\sqrt{{ch}^2\left(2t\right)-1}-argch\left(x\right)+c$$$$=\sqrt{x^2-1}-ln(x+\sqrt{x^2-1})+c$$

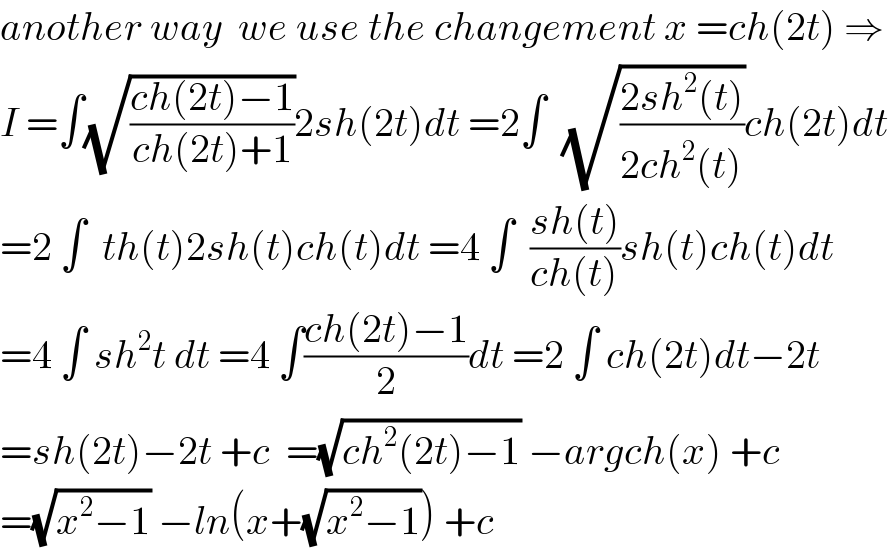
Answered by Tanmay chaudhury last updated on 29/Nov/19

$$\int \frac{x-1}{\sqrt{x^2-1}}dx$$$$\frac{1}{2}\int \frac{d(x^2-1)}{{(x^2-1)}^{\frac{1}{2}}}-\int \frac{dx}{\sqrt{x^2-1}}$$$$I_1=\frac{1}{2}\times \frac{{(x^2-1)}^{\frac{-1}{2}+1}}{\frac{-1}{2}+1}+c_1\Rrightarrow {(x^2-1)}^{\frac{1}{2}}+c_1$$$$I_2=\int \frac{dx}{\sqrt{x^2-1}}letx=sec\alpha dx=sec\alpha tan\alpha d\alpha$$$$=\int \frac{sec\alpha tan\alpha d\alpha }{tan\alpha }=\int sec\alpha d\alpha =ln(sec\alpha +tan\alpha )+c_2$$$$=ln(x+\sqrt{x^2-1})+c_2$$

Commented by chess1 last updated on 30/Nov/19

$$\mathrm{thanks}$$

Answered by Kunal12588 last updated on 30/Nov/19

$$I=\int \sqrt{\frac{x-1}{x+1}}dx=\int \frac{x-1}{\sqrt{x^2-1}}dx$$$$=\int \frac{x}{\sqrt{x^2-1}}dx-\int \frac{dx}{\sqrt{x^2-1}}$$$$=\frac{1}{2}\int \frac{2x}{\sqrt{x^2-1}}dx-log\mid x+\sqrt{x^2-1}\mid +C$$$$let{x}^2-1=t\Rightarrow 2xdx=dt$$$$I=\frac{1}{2}\int \frac{dt}{\sqrt{t}}-log\mid x+\sqrt{x^2-1}\mid +C$$$$=\frac{1}{2}\left(\frac{\sqrt{t}}{1/2}\right)-log\mid x+\sqrt{x^2-1}\mid +C$$$$=\sqrt{x^2-1}-log\mid x+\sqrt{x^2-1}\mid +C$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com