
Question and Answers Forum
Question Number 74748 by mr W last updated on 30/Nov/19

Commented by mr W last updated on 30/Nov/19

Commented by mr W last updated on 30/Nov/19

Commented by ajfour last updated on 30/Nov/19

Answered by ajfour last updated on 30/Nov/19
![O(0,0) , A(1,0) , B(rcos θ, rsin θ) let p=OA=1 q^2 = (−rcos θ+1)^2 +r^2 sin^2 θ qsin φ=rsin θ △_0 = 1+r^2 +1+r^2 −2rcos θ = 2+2r(r−cos θ) O_1 =(−rsin θ, rcos θ) O_2 =(0,−1) A_1 =(1,−1) A_2 =(1+rsin θ, 1−rcos θ) B_1 =(rcos θ+rsin θ, 1+rsin θ−rcos θ) B_2 =(rcos θ−rsin θ, rsin θ+rcos θ) q_1 ^2 =(O_1 O_2 )^2 = (1+rcos θ)^2 +r^2 sin^2 θ r_1 ^2 =(A_1 A_2 )^2 = (2−rcos θ)^2 +r^2 sin^2 θ p_1 ^2 =(B_1 B_2 )^2 =4r^2 sin^2 θ+(2rcos θ−1)^2 △_1 = p_1 ^2 +q_1 ^2 +r_1 ^2 = 6+6r(r−cos θ) ⇒ △_1 = 6+3(△_0 −2) = 3△_0 ________________________ p_1 ^� =−2rsin θ+i(2rcos θ−1) q_1 ^� = rsin θ−i(1+rcos θ) r_1 ^� = rsin θ+i(2−rcos θ) A_(11) = A_1 −ir_1 ^� = 1−i−i[rsin θ+i(2−rcos θ)] = 3−rcos θ−i(1+rsin θ) O_(22) = O_2 −iq_1 ^� = −i−i[rsin θ−i(1+rcos θ)] = −(1+rcos θ)−i(1+rsin θ) u= O_(22) A_(11) = 4 = 4p A_(22) =A_2 −ir_1 ^� = 1+rsin θ+i(1−rcos θ) −i[rsin θ+i(2−rcos θ)] = 3+rsin θ−rcos θ +i(1−rcos θ−rsin θ) B_(11) =B_1 −ip_1 ^� = rcos θ+rsin θ+i(1+rsin θ−rcos θ) −i[−2rsin θ+i(2rcos θ−1)] = 3rcos θ−1+rsin θ+i(1+3rsin θ−rcos θ) v=A_(22) B_(11) =4rcos θ−4+i(4rsin θ) ∣A_(22) B_(11) ∣=4(√(1+r(r−2cos θ))) ((∣A_(22) B_(11) ∣)/q)=((4(√(1+r(r−2cos θ))))/(√(1+r(r−2cos θ)))) = 4 ⇒ v= 4q similary w= B_(22) O_(11) = 4r ⇒ Sum of areas of green squares = △_2 = u^2 +v^2 +w^2 = 16(p^2 +q^2 +r^2 ) = 16△_0 .](Q74781.png)
Commented by mr W last updated on 30/Nov/19

Commented by mr W last updated on 01/Dec/19
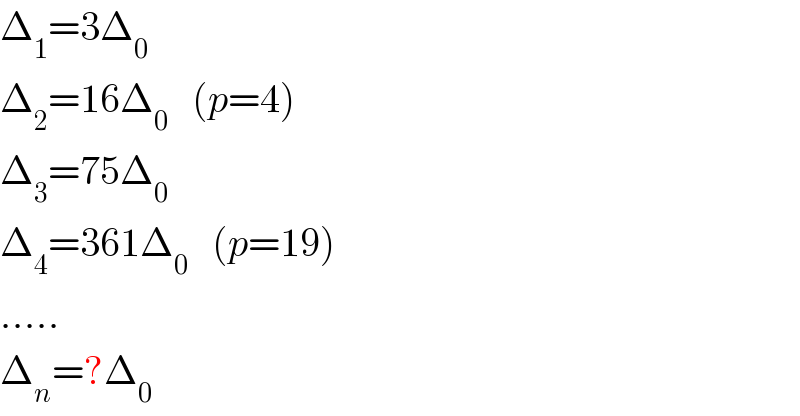
Commented by mr W last updated on 01/Dec/19

Commented by mr W last updated on 01/Dec/19

