
Question and Answers Forum
Question Number 75793 by ~blr237~ last updated on 17/Dec/19
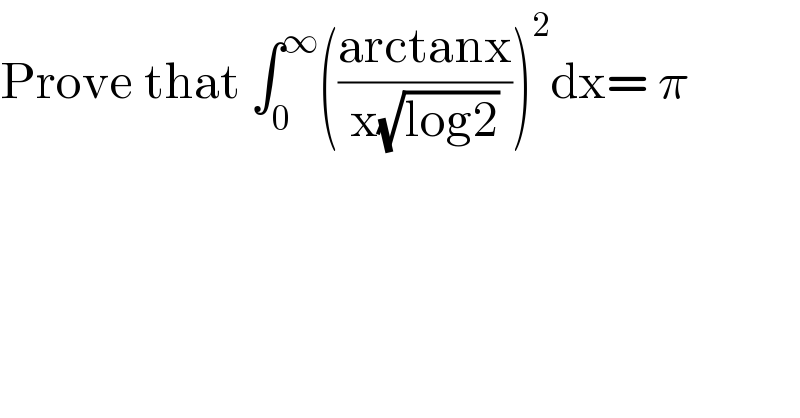
Commented by mathmax by abdo last updated on 18/Dec/19
![let I =∫_0 ^∞ (((arctanx)/(x(√(ln(2))))))^2 dx ⇒ ln(2)I =∫_0 ^∞ ((arctan^2 x)/x^2 )dx by parts u^′ =(1/x^2 ) and v=arctan^2 (x) ⇒ln(2)I =[−(1/x) arctan^2 (x)]_0 ^(+∞) −∫_0 ^∞ (−(1/x))((2arctan(x))/(1+x^2 ))dx=2∫_0 ^∞ ((arcctan(x))/(x(1+x^2 )))dx ⇒ I=(2/(ln(2)))∫_0 ^∞ ((arctan(x))/(x(1+x^2 )))dx =_(arctanx=u) (2/(ln(2))) ∫_0 ^(π/2) (u/(tan(u)(1+tan^2 u)))(1+tan^2 u)du =(2/(ln(2)))∫_0 ^(π/2) (u/(tanu))du =(2/(ln(2)))∫_0 ^(π/2) u ((cosu)/(sinu))du by parts f=u and g^′ =((cosu)/(sinu)) ((ln(2))/2)I =∫_0 ^(π/2) u ((cosu)/(sinu))du=[u ln(sinu)]_0 ^(π/2) −∫_0 ^(π/2) ln(sinu)du=−(−(π/2)ln(2)) ((ln(2))/2)I =((πln(2))/2) ⇒ I =π](Q75819.png)
Answered by mind is power last updated on 17/Dec/19
![=[((−arctan^2 (x))/(xlog(2)))]+(1/(log(2)))∫_0 ^(+∞) ((2arctan(x))/(x(x^2 +1)))dx =(2/(log(2)))∫_0 ^(+∞) ((arcran(x))/(x(x^2 +1)))dx u=arcran(x)⇒dx=(1+tg^2 (u))du ⇔(2/(log(2)))∫_0 ^(π/2) (u/(tg(u)))du=(2/(log(2)))∫_0 ^(π/2) ((ucos(u))/(sin(u)))du by part⇔ (2/(log(2)))[ulog(sin(u))]_0 ^(π/2) −(2/(log(2)))∫_0 ^(π/2) log(sin(u))du =−(2/(log(2)))∫_0 ^(π/2) log(sin(u))du ∫_0 ^(π/2) log(sin(u))du=−log(2)(π/2) done many Times so We get −(2/(log(2))).((−log(2))/2).π=π](Q75805.png)
| ||
Question and Answers Forum | ||
Question Number 75793 by ~blr237~ last updated on 17/Dec/19 | ||
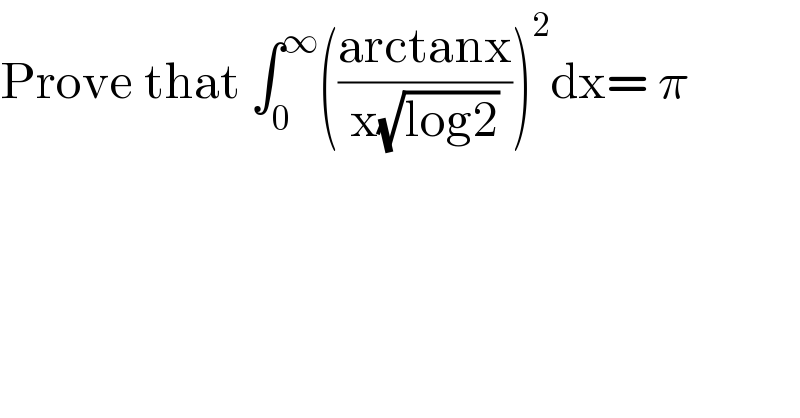 | ||
Commented by mathmax by abdo last updated on 18/Dec/19 | ||
![let I =∫_0 ^∞ (((arctanx)/(x(√(ln(2))))))^2 dx ⇒ ln(2)I =∫_0 ^∞ ((arctan^2 x)/x^2 )dx by parts u^′ =(1/x^2 ) and v=arctan^2 (x) ⇒ln(2)I =[−(1/x) arctan^2 (x)]_0 ^(+∞) −∫_0 ^∞ (−(1/x))((2arctan(x))/(1+x^2 ))dx=2∫_0 ^∞ ((arcctan(x))/(x(1+x^2 )))dx ⇒ I=(2/(ln(2)))∫_0 ^∞ ((arctan(x))/(x(1+x^2 )))dx =_(arctanx=u) (2/(ln(2))) ∫_0 ^(π/2) (u/(tan(u)(1+tan^2 u)))(1+tan^2 u)du =(2/(ln(2)))∫_0 ^(π/2) (u/(tanu))du =(2/(ln(2)))∫_0 ^(π/2) u ((cosu)/(sinu))du by parts f=u and g^′ =((cosu)/(sinu)) ((ln(2))/2)I =∫_0 ^(π/2) u ((cosu)/(sinu))du=[u ln(sinu)]_0 ^(π/2) −∫_0 ^(π/2) ln(sinu)du=−(−(π/2)ln(2)) ((ln(2))/2)I =((πln(2))/2) ⇒ I =π](Q75819.png) | ||
Answered by mind is power last updated on 17/Dec/19 | ||
![=[((−arctan^2 (x))/(xlog(2)))]+(1/(log(2)))∫_0 ^(+∞) ((2arctan(x))/(x(x^2 +1)))dx =(2/(log(2)))∫_0 ^(+∞) ((arcran(x))/(x(x^2 +1)))dx u=arcran(x)⇒dx=(1+tg^2 (u))du ⇔(2/(log(2)))∫_0 ^(π/2) (u/(tg(u)))du=(2/(log(2)))∫_0 ^(π/2) ((ucos(u))/(sin(u)))du by part⇔ (2/(log(2)))[ulog(sin(u))]_0 ^(π/2) −(2/(log(2)))∫_0 ^(π/2) log(sin(u))du =−(2/(log(2)))∫_0 ^(π/2) log(sin(u))du ∫_0 ^(π/2) log(sin(u))du=−log(2)(π/2) done many Times so We get −(2/(log(2))).((−log(2))/2).π=π](Q75805.png) | ||
| ||