
Question and Answers Forum
Question Number 77232 by naka3546 last updated on 04/Jan/20

Answered by MJS last updated on 04/Jan/20
![∫ln ((√x)+(√(1−x))) dx= [t=arcsin (√x) → dx=2(√(x(1−x)))dt] =2∫cos t sin t ln (cos t +sin t) dt= by parts u=ln (cos t +sin t) → u′=((cos t −sin t)/(cos t +sin t)) v′=cos t sin t → v=−(1/2)cos^2 t =−cos^2 t ln (cos t +sin t) +∫((cos t −sin t)/(cos t +sin t))cos^2 t dt= ∫((cos t −sin t)/(cos t +sin t))cos^2 t dt= [u=tan t → dt=cos^2 t du] =−∫((u−1)/((u+1)(u^2 +1)^2 ))du= =−∫(u/((u^2 +1)^2 ))du−(1/2)∫((u−1)/(u^2 +1))du+(1/2)∫(du/(u+1))= =(1/(2(u^2 +1)))−(1/4)ln (u^2 +1) +(1/2)arctan u +(1/2)ln (u+1) = =(1/2)cos^2 t +(1/2)ln cos x +(1/2)t +(1/2)ln (1+tan x) =(1/2)(t+cos^2 t +ln (cos t +sin t)) = =(1/2)(−x+ln ((√x)+(√(1−x)))+arcsin (√x))+C ⇒ answer is (π/4)−(1/2)](Q77254.png)
Commented by naka3546 last updated on 04/Jan/20
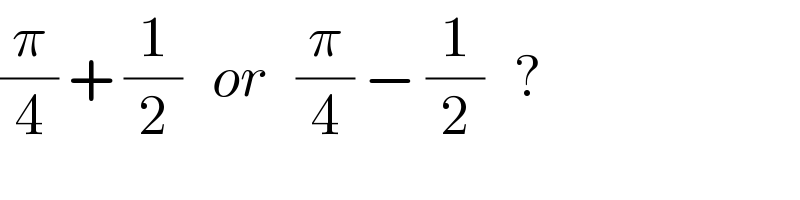
Commented by MJS last updated on 04/Jan/20

| ||
Question and Answers Forum | ||
Question Number 77232 by naka3546 last updated on 04/Jan/20 | ||
 | ||
Answered by MJS last updated on 04/Jan/20 | ||
![∫ln ((√x)+(√(1−x))) dx= [t=arcsin (√x) → dx=2(√(x(1−x)))dt] =2∫cos t sin t ln (cos t +sin t) dt= by parts u=ln (cos t +sin t) → u′=((cos t −sin t)/(cos t +sin t)) v′=cos t sin t → v=−(1/2)cos^2 t =−cos^2 t ln (cos t +sin t) +∫((cos t −sin t)/(cos t +sin t))cos^2 t dt= ∫((cos t −sin t)/(cos t +sin t))cos^2 t dt= [u=tan t → dt=cos^2 t du] =−∫((u−1)/((u+1)(u^2 +1)^2 ))du= =−∫(u/((u^2 +1)^2 ))du−(1/2)∫((u−1)/(u^2 +1))du+(1/2)∫(du/(u+1))= =(1/(2(u^2 +1)))−(1/4)ln (u^2 +1) +(1/2)arctan u +(1/2)ln (u+1) = =(1/2)cos^2 t +(1/2)ln cos x +(1/2)t +(1/2)ln (1+tan x) =(1/2)(t+cos^2 t +ln (cos t +sin t)) = =(1/2)(−x+ln ((√x)+(√(1−x)))+arcsin (√x))+C ⇒ answer is (π/4)−(1/2)](Q77254.png) | ||
| ||
Commented by naka3546 last updated on 04/Jan/20 | ||
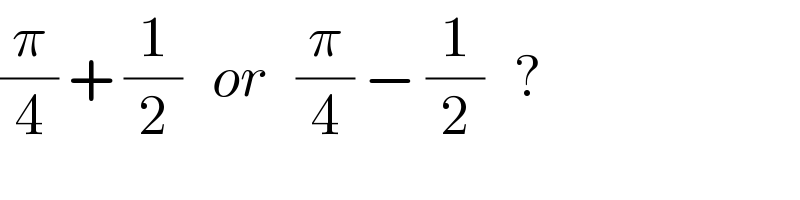 | ||
Commented by MJS last updated on 04/Jan/20 | ||
 | ||