Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 78264 by msup trace by abdo last updated on 15/Jan/20

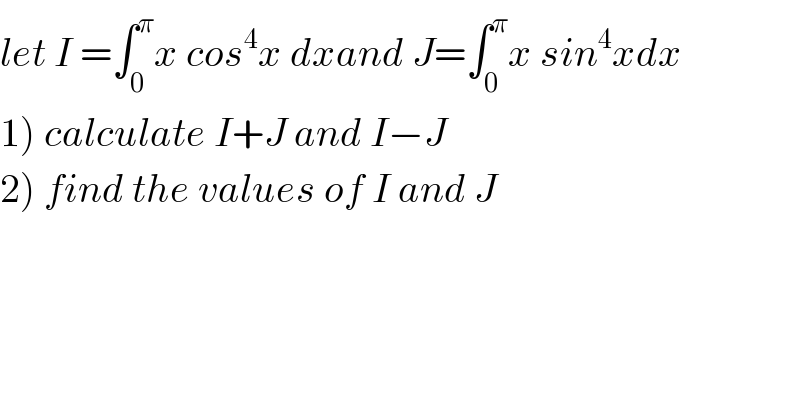
let I =∫_0 ^π x cos^4 x dxand J=∫_0 ^π x sin^4 xdx 1) calculate I+J and I−J 2) find the values of I and J

$${let}\:{I}\:=\int_{\mathrm{0}} ^{\pi} {x}\:{cos}^{\mathrm{4}} {x}\:{dxand}\:{J}=\int_{\mathrm{0}} ^{\pi} {x}\:{sin}^{\mathrm{4}} {xdx} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{I}+{J}\:{and}\:{I}−{J} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{values}\:{of}\:{I}\:{and}\:{J} \\ $$

Commented by jagoll last updated on 15/Jan/20

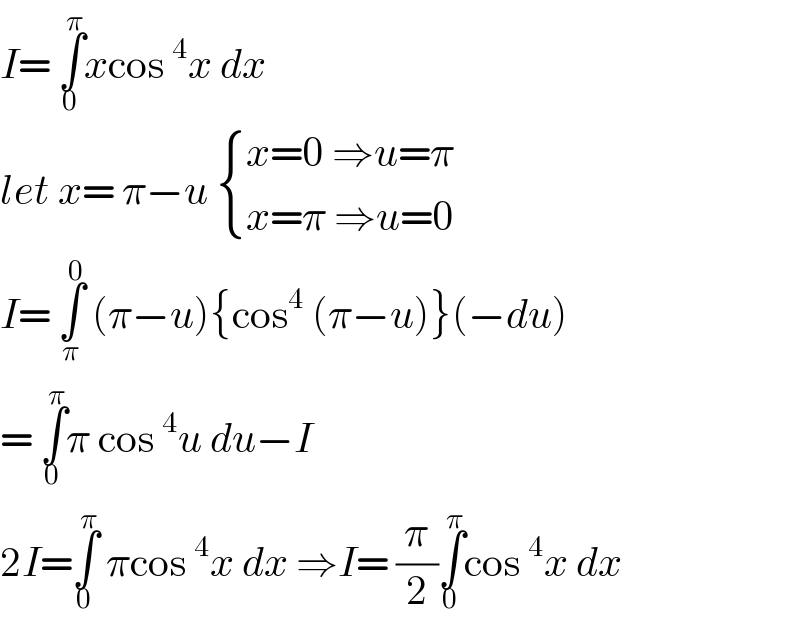
I= ∫_0 ^π xcos^4 x dx let x= π−u { ((x=0 ⇒u=π)),((x=π ⇒u=0)) :} I= ∫_π ^0 (π−u){cos^4 (π−u)}(−du) = ∫_0 ^π π cos^4 u du−I 2I=∫_0 ^π πcos^4 x dx ⇒I= (π/2)∫_0 ^π cos^4 x dx

$${I}=\:\underset{\mathrm{0}} {\overset{\pi} {\int}}{x}\mathrm{cos}\:^{\mathrm{4}} {x}\:{dx} \\ $$$${let}\:{x}=\:\pi−{u}\:\begin{cases}{{x}=\mathrm{0}\:\Rightarrow{u}=\pi}\\{{x}=\pi\:\Rightarrow{u}=\mathrm{0}}\end{cases} \\ $$$${I}=\:\underset{\pi} {\overset{\mathrm{0}} {\int}}\:\left(\pi−{u}\right)\left\{\mathrm{cos}^{\mathrm{4}} \:\left(\pi−{u}\right)\right\}\left(−{du}\right) \\ $$$$=\:\underset{\mathrm{0}} {\overset{\pi} {\int}}\pi\:\mathrm{cos}\:^{\mathrm{4}} {u}\:{du}−{I} \\ $$$$\mathrm{2}{I}=\underset{\mathrm{0}} {\overset{\pi} {\int}}\:\pi\mathrm{cos}\:^{\mathrm{4}} {x}\:{dx}\:\Rightarrow{I}=\:\frac{\pi}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\pi} {\int}}\mathrm{cos}\:^{\mathrm{4}} {x}\:{dx} \\ $$

Commented by jagoll last updated on 15/Jan/20

J = ∫_0 ^π xsin^4 x dx. let x=π−u J=∫_0 ^π πsin^4 x dx − J J= (π/2)∫_0 ^π sin^4 x dx

$${J}\:=\:\underset{\mathrm{0}} {\overset{\pi} {\int}}{x}\mathrm{sin}\:^{\mathrm{4}} {x}\:{dx}.\:{let}\:{x}=\pi−{u} \\ $$$${J}=\underset{\mathrm{0}} {\overset{\pi} {\int}}\pi\mathrm{sin}\:^{\mathrm{4}} {x}\:{dx}\:−\:{J} \\ $$$${J}=\:\frac{\pi}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\pi} {\int}}\:\mathrm{sin}\:^{\mathrm{4}} {x}\:{dx} \\ $$

Commented by jagoll last updated on 15/Jan/20

I+J = (π/2)∫_0 ^π cos^4 x+sin^4 x dx =(π/2)∫_0 ^π (1−(1/2)sin^2 (2x))dx =(π/2)∫_0 ^π ((1/4)+(1/4)cos (4x))dx

$${I}+{J}\:=\:\frac{\pi}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\pi} {\int}}\:\mathrm{cos}\:^{\mathrm{4}} {x}+\mathrm{sin}\:^{\mathrm{4}} {x}\:{dx} \\ $$$$=\frac{\pi}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\pi} {\int}}\:\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\:^{\mathrm{2}} \left(\mathrm{2}{x}\right)\right){dx} \\ $$$$=\frac{\pi}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\pi} {\int}}\left(\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{4}}\mathrm{cos}\:\left(\mathrm{4}{x}\right)\right){dx} \\ $$

Commented by mathmax by abdo last updated on 18/Jan/20

1) we have I +J =∫_0 ^π x(cos^4 x +sin^4 x)dx =∫_0 ^π x{ (cos^2 x +sin^2 x)^2 −2cos^2 x sin^2 x}dx =∫_0 ^π x{ 1−2((1/2)sin(2x))^2 }dx =∫_0 ^π x{1−(1/2)sin^2 (2x)}dx =∫_0 ^π x{1−(1/2)×((1−cos(4x))/2)}dx =∫_0 ^π x{(3/4)+(1/4)cos(4x)}dx =(3/4)∫_0 ^π xdx +(1/4) ∫_0 ^π xcos(4x)dx =(3/4)×(π^2 /2) +(1/4){ [(x/4)sin(4x)]_0 ^π −∫_0 ^π (1/4)sin(4x)dx} =((3π^2 )/8) −(1/(16))[−(1/4)cos(4x)]_0 ^π =((3π^2 )/8) also we have I−J =∫_0 ^π x(cos^4 x −sin^4 x)dx =∫_0 ^π x(cos^2 x −sin^2 x)dx =∫_0 ^π xcos(2x)dx =[(x/2)sin(2x)]_0 ^π −∫_0 ^π (1/2)sin(2x)dx} =−(1/2)[−(1/2)cos(2x)]_0 ^π =0 ⇒I =J ⇒2I =((3π^2 )/8) ⇒I =((3π^2 )/(16)) and J =((3π^2 )/(16))

$$\left.\mathrm{1}\right)\:{we}\:{have}\:{I}\:+{J}\:=\int_{\mathrm{0}} ^{\pi} {x}\left({cos}^{\mathrm{4}} {x}\:+{sin}^{\mathrm{4}} {x}\right){dx} \\ $$$$=\int_{\mathrm{0}} ^{\pi} {x}\left\{\:\left({cos}^{\mathrm{2}} {x}\:+{sin}^{\mathrm{2}} {x}\right)^{\mathrm{2}} −\mathrm{2}{cos}^{\mathrm{2}} {x}\:{sin}^{\mathrm{2}} {x}\right\}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\pi} {x}\left\{\:\mathrm{1}−\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{2}}{sin}\left(\mathrm{2}{x}\right)\right)^{\mathrm{2}} \right\}{dx}\:=\int_{\mathrm{0}} ^{\pi} {x}\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}{sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)\right\}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\pi} {x}\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}−{cos}\left(\mathrm{4}{x}\right)}{\mathrm{2}}\right\}{dx}\:=\int_{\mathrm{0}} ^{\pi} {x}\left\{\frac{\mathrm{3}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{4}}{cos}\left(\mathrm{4}{x}\right)\right\}{dx} \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\pi} {xdx}\:+\frac{\mathrm{1}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\pi} \:{xcos}\left(\mathrm{4}{x}\right){dx} \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}×\frac{\pi^{\mathrm{2}} }{\mathrm{2}}\:+\frac{\mathrm{1}}{\mathrm{4}}\left\{\:\left[\frac{{x}}{\mathrm{4}}{sin}\left(\mathrm{4}{x}\right)\right]_{\mathrm{0}} ^{\pi} −\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{1}}{\mathrm{4}}{sin}\left(\mathrm{4}{x}\right){dx}\right\} \\ $$$$=\frac{\mathrm{3}\pi^{\mathrm{2}} }{\mathrm{8}}\:−\frac{\mathrm{1}}{\mathrm{16}}\left[−\frac{\mathrm{1}}{\mathrm{4}}{cos}\left(\mathrm{4}{x}\right)\right]_{\mathrm{0}} ^{\pi} \:=\frac{\mathrm{3}\pi^{\mathrm{2}} }{\mathrm{8}}\:\:{also}\:{we}\:{have} \\ $$$${I}−{J}\:=\int_{\mathrm{0}} ^{\pi} {x}\left({cos}^{\mathrm{4}} {x}\:−{sin}^{\mathrm{4}} {x}\right){dx}\:=\int_{\mathrm{0}} ^{\pi} {x}\left({cos}^{\mathrm{2}} {x}\:−{sin}^{\mathrm{2}} {x}\right){dx} \\ $$$$\left.=\int_{\mathrm{0}} ^{\pi} {xcos}\left(\mathrm{2}{x}\right){dx}\:=\left[\frac{{x}}{\mathrm{2}}{sin}\left(\mathrm{2}{x}\right)\right]_{\mathrm{0}} ^{\pi} \:−\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{1}}{\mathrm{2}}{sin}\left(\mathrm{2}{x}\right){dx}\right\} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\left[−\frac{\mathrm{1}}{\mathrm{2}}{cos}\left(\mathrm{2}{x}\right)\right]_{\mathrm{0}} ^{\pi} =\mathrm{0}\:\Rightarrow{I}\:={J}\:\Rightarrow\mathrm{2}{I}\:=\frac{\mathrm{3}\pi^{\mathrm{2}} }{\mathrm{8}}\:\Rightarrow{I}\:=\frac{\mathrm{3}\pi^{\mathrm{2}} }{\mathrm{16}}\:{and} \\ $$$${J}\:=\frac{\mathrm{3}\pi^{\mathrm{2}} }{\mathrm{16}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com