Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 7831 by Chantria last updated on 18/Sep/16

Commented by prakash jain last updated on 18/Sep/16

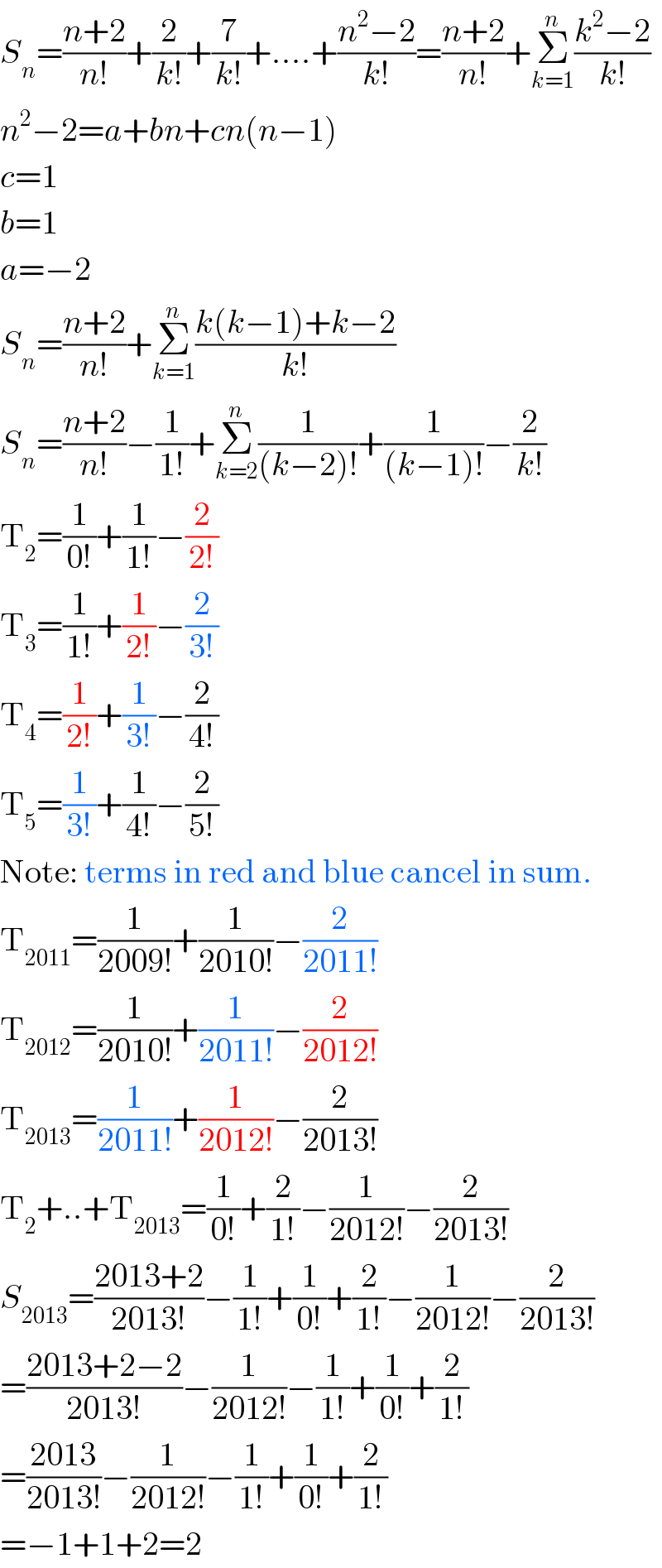
S_n =((n+2)/(n!))+(2/(k!))+(7/(k!))+....+((n^2 −2)/(k!))=((n+2)/(n!))+Σ_(k=1) ^n ((k^2 −2)/(k!)) n^2 −2=a+bn+cn(n−1) c=1 b=1 a=−2 S_n =((n+2)/(n!))+Σ_(k=1) ^n ((k(k−1)+k−2)/(k!)) S_n =((n+2)/(n!))−(1/(1!))+Σ_(k=2) ^n (1/((k−2)!))+(1/((k−1)!))−(2/(k!)) T_2 =(1/(0!))+(1/(1!))−(2/(2!)) T_3 =(1/(1!))+(1/(2!))−(2/(3!)) T_4 =(1/(2!))+(1/(3!))−(2/(4!)) T_5 =(1/(3!))+(1/(4!))−(2/(5!)) Note: terms in red and blue cancel in sum. T_(2011) =(1/(2009!))+(1/(2010!))−(2/(2011!)) T_(2012) =(1/(2010!))+(1/(2011!))−(2/(2012!)) T_(2013) =(1/(2011!))+(1/(2012!))−(2/(2013!)) T_2 +..+T_(2013) =(1/(0!))+(2/(1!))−(1/(2012!))−(2/(2013!)) S_(2013) =((2013+2)/(2013!))−(1/(1!))+(1/(0!))+(2/(1!))−(1/(2012!))−(2/(2013!)) =((2013+2−2)/(2013!))−(1/(2012!))−(1/(1!))+(1/(0!))+(2/(1!)) =((2013)/(2013!))−(1/(2012!))−(1/(1!))+(1/(0!))+(2/(1!)) =−1+1+2=2

$${S}_{{n}} =\frac{{n}+\mathrm{2}}{{n}!}+\frac{\mathrm{2}}{{k}!}+\frac{\mathrm{7}}{{k}!}+....+\frac{{n}^{\mathrm{2}} −\mathrm{2}}{{k}!}=\frac{{n}+\mathrm{2}}{{n}!}+\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{{k}^{\mathrm{2}} −\mathrm{2}}{{k}!} \\ $$$${n}^{\mathrm{2}} −\mathrm{2}={a}+{bn}+{cn}\left({n}−\mathrm{1}\right) \\ $$$${c}=\mathrm{1} \\ $$$${b}=\mathrm{1} \\ $$$${a}=−\mathrm{2} \\ $$$${S}_{{n}} =\frac{{n}+\mathrm{2}}{{n}!}+\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{{k}\left({k}−\mathrm{1}\right)+{k}−\mathrm{2}}{{k}!} \\ $$$${S}_{{n}} =\frac{{n}+\mathrm{2}}{{n}!}−\frac{\mathrm{1}}{\mathrm{1}!}+\underset{{k}=\mathrm{2}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{\left({k}−\mathrm{2}\right)!}+\frac{\mathrm{1}}{\left({k}−\mathrm{1}\right)!}−\frac{\mathrm{2}}{{k}!} \\ $$$$\mathrm{T}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{0}!}+\frac{\mathrm{1}}{\mathrm{1}!}−\frac{\mathrm{2}}{\mathrm{2}!} \\ $$$$\mathrm{T}_{\mathrm{3}} =\frac{\mathrm{1}}{\mathrm{1}!}+\frac{\mathrm{1}}{\mathrm{2}!}−\frac{\mathrm{2}}{\mathrm{3}!} \\ $$$$\mathrm{T}_{\mathrm{4}} =\frac{\mathrm{1}}{\mathrm{2}!}+\frac{\mathrm{1}}{\mathrm{3}!}−\frac{\mathrm{2}}{\mathrm{4}!} \\ $$$$\mathrm{T}_{\mathrm{5}} =\frac{\mathrm{1}}{\mathrm{3}!}+\frac{\mathrm{1}}{\mathrm{4}!}−\frac{\mathrm{2}}{\mathrm{5}!} \\ $$$$\mathrm{Note}:\:\mathrm{terms}\:\mathrm{in}\:\mathrm{red}\:\mathrm{and}\:\mathrm{blue}\:\mathrm{cancel}\:\mathrm{in}\:\mathrm{sum}. \\ $$$$\mathrm{T}_{\mathrm{2011}} =\frac{\mathrm{1}}{\mathrm{2009}!}+\frac{\mathrm{1}}{\mathrm{2010}!}−\frac{\mathrm{2}}{\mathrm{2011}!} \\ $$$$\mathrm{T}_{\mathrm{2012}} =\frac{\mathrm{1}}{\mathrm{2010}!}+\frac{\mathrm{1}}{\mathrm{2011}!}−\frac{\mathrm{2}}{\mathrm{2012}!} \\ $$$$\mathrm{T}_{\mathrm{2013}} =\frac{\mathrm{1}}{\mathrm{2011}!}+\frac{\mathrm{1}}{\mathrm{2012}!}−\frac{\mathrm{2}}{\mathrm{2013}!} \\ $$$$\mathrm{T}_{\mathrm{2}} +..+\mathrm{T}_{\mathrm{2013}} =\frac{\mathrm{1}}{\mathrm{0}!}+\frac{\mathrm{2}}{\mathrm{1}!}−\frac{\mathrm{1}}{\mathrm{2012}!}−\frac{\mathrm{2}}{\mathrm{2013}!} \\ $$$${S}_{\mathrm{2013}} =\frac{\mathrm{2013}+\mathrm{2}}{\mathrm{2013}!}−\frac{\mathrm{1}}{\mathrm{1}!}+\frac{\mathrm{1}}{\mathrm{0}!}+\frac{\mathrm{2}}{\mathrm{1}!}−\frac{\mathrm{1}}{\mathrm{2012}!}−\frac{\mathrm{2}}{\mathrm{2013}!} \\ $$$$=\frac{\mathrm{2013}+\mathrm{2}−\mathrm{2}}{\mathrm{2013}!}−\frac{\mathrm{1}}{\mathrm{2012}!}−\frac{\mathrm{1}}{\mathrm{1}!}+\frac{\mathrm{1}}{\mathrm{0}!}+\frac{\mathrm{2}}{\mathrm{1}!} \\ $$$$=\frac{\mathrm{2013}}{\mathrm{2013}!}−\frac{\mathrm{1}}{\mathrm{2012}!}−\frac{\mathrm{1}}{\mathrm{1}!}+\frac{\mathrm{1}}{\mathrm{0}!}+\frac{\mathrm{2}}{\mathrm{1}!} \\ $$$$=−\mathrm{1}+\mathrm{1}+\mathrm{2}=\mathrm{2} \\ $$

Commented by prakash jain last updated on 18/Sep/16

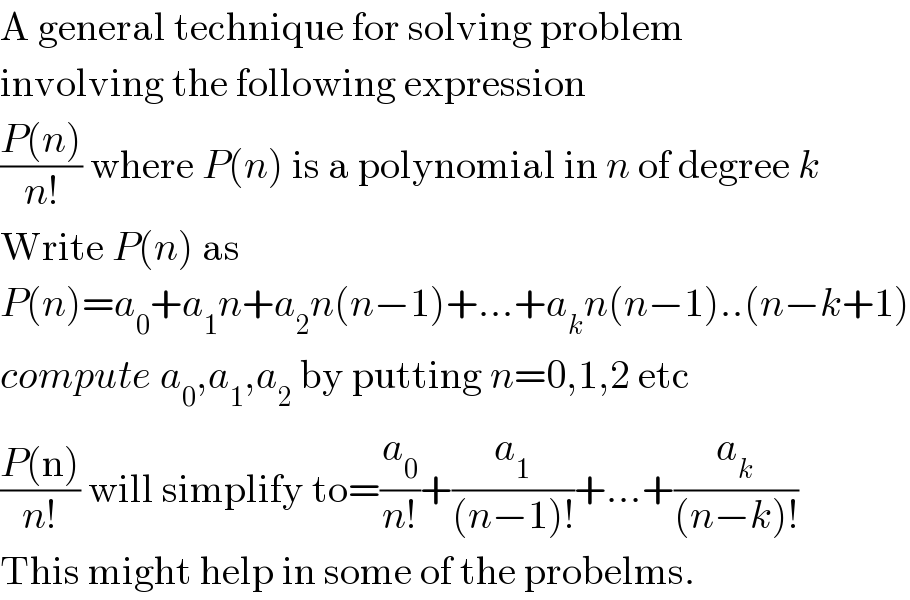
A general technique for solving problem involving the following expression ((P(n))/(n!)) where P(n) is a polynomial in n of degree k Write P(n) as P(n)=a_0 +a_1 n+a_2 n(n−1)+...+a_k n(n−1)..(n−k+1) compute a_0 ,a_1 ,a_2 by putting n=0,1,2 etc ((P(n))/(n!)) will simplify to=(a_0 /(n!))+(a_1 /((n−1)!))+...+(a_k /((n−k)!)) This might help in some of the probelms.

$$\mathrm{A}\:\mathrm{general}\:\mathrm{technique}\:\mathrm{for}\:\mathrm{solving}\:\mathrm{problem} \\ $$$$\mathrm{involving}\:\mathrm{the}\:\mathrm{following}\:\mathrm{expression} \\ $$$$\frac{{P}\left({n}\right)}{{n}!}\:\mathrm{where}\:{P}\left({n}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{in}\:{n}\:\mathrm{of}\:\mathrm{degree}\:{k} \\ $$$$\mathrm{Write}\:{P}\left({n}\right)\:\mathrm{as} \\ $$$${P}\left({n}\right)={a}_{\mathrm{0}} +{a}_{\mathrm{1}} {n}+{a}_{\mathrm{2}} {n}\left({n}−\mathrm{1}\right)+...+{a}_{{k}} {n}\left({n}−\mathrm{1}\right)..\left({n}−{k}+\mathrm{1}\right) \\ $$$${compute}\:{a}_{\mathrm{0}} ,{a}_{\mathrm{1}} ,{a}_{\mathrm{2}} \:\mathrm{by}\:\mathrm{putting}\:{n}=\mathrm{0},\mathrm{1},\mathrm{2}\:\mathrm{etc} \\ $$$$\frac{{P}\left(\mathrm{n}\right)}{{n}!}\:\mathrm{will}\:\mathrm{simplify}\:\mathrm{to}=\frac{{a}_{\mathrm{0}} }{{n}!}+\frac{{a}_{\mathrm{1}} }{\left({n}−\mathrm{1}\right)!}+...+\frac{{a}_{{k}} }{\left({n}−{k}\right)!} \\ $$$$\mathrm{This}\:\mathrm{might}\:\mathrm{help}\:\mathrm{in}\:\mathrm{some}\:\mathrm{of}\:\mathrm{the}\:\mathrm{probelms}. \\ $$

Answered by prakash jain last updated on 02/Oct/16

see comments

$$\mathrm{see}\:\mathrm{comments} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com