Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 7841 by ankursharma532 last updated on 19/Sep/16

tanΠ/16=(√(4+2(√2) )) − ((√2) +1)

$${tan}\Pi/\mathrm{16}=\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}\:}\:−\:\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right) \\ $$

Commented by Yozzia last updated on 19/Sep/16

tan4x=((2tan2x)/(1−tan^2 2x)) Let x=π/16⇒tan4x=tan(π/4)=1 ∴2tan2x=1−tan^2 2x tan^2 2x+2tan2x=1 tan^2 2x+2tan2x+1=2 (tan2x+1)^2 =2 tan2x+1=(√2) ∵ 0<x<π/2 tan2x=(√2)−1 ((2tanx)/(1−tan^2 x))=(√2)−1 2tanx=(√2)−1−((√2)−1)tan^2 x ((√2)−1)tan^2 x+2tanx−((√2)−1)=0 tanx=((−2±(√(2^2 +4((√2)−1)((√2)−1))))/(2((√2)−1))) tanx=((−2±2(√(1+((√2)−1)^2 )))/(2((√2)−1))) tanx=((−1±(√(1+((√2)−1)^2 )))/((√2)−1)) ∵ (√(1+((√2)−1)^2 ))>1⇒−(√(1+((√2)−1)^2 ))<−1 ⇒−1−(√(1+((√2)−1)^2 ))<−2<0 while tanx>0 if 0<x<π/2 ∴ tanx=((−1+(√(1+2+1−2(√2))))/((√2)−1)) tanx=((−1+(√(4−2(√2))))/((√2)−1)) tanx=(((−1+(√(4−2(√2))))((√2)+1))/(2−1)) tanx=(√(4−2(√2)))((√2)+1)−((√2)+1) tanx=(√((4−2(√2))((√2)+1)^2 ))−((√2)+1) tanx=(√((4−2(√2))(3+2(√2))))−((√2)+1) tanx=(√(12−8+8(√2)−6(√2)))−((√2)+1) tanx=(√(4+2(√2)))−((√2)+1) tan(π/(16))=(√(4+2(√2)))−((√2)+1)

$${tan}\mathrm{4}{x}=\frac{\mathrm{2}{tan}\mathrm{2}{x}}{\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{2}{x}} \\ $$$${Let}\:{x}=\pi/\mathrm{16}\Rightarrow{tan}\mathrm{4}{x}={tan}\frac{\pi}{\mathrm{4}}=\mathrm{1} \\ $$$$\therefore\mathrm{2}{tan}\mathrm{2}{x}=\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{2}{x} \\ $$$${tan}^{\mathrm{2}} \mathrm{2}{x}+\mathrm{2}{tan}\mathrm{2}{x}=\mathrm{1} \\ $$$${tan}^{\mathrm{2}} \mathrm{2}{x}+\mathrm{2}{tan}\mathrm{2}{x}+\mathrm{1}=\mathrm{2} \\ $$$$\left({tan}\mathrm{2}{x}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{2} \\ $$$${tan}\mathrm{2}{x}+\mathrm{1}=\sqrt{\mathrm{2}}\:\:\:\because\:\mathrm{0}<{x}<\pi/\mathrm{2} \\ $$$${tan}\mathrm{2}{x}=\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$$\frac{\mathrm{2}{tanx}}{\mathrm{1}−{tan}^{\mathrm{2}} {x}}=\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$$\mathrm{2}{tanx}=\sqrt{\mathrm{2}}−\mathrm{1}−\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){tan}^{\mathrm{2}} {x} \\ $$$$\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){tan}^{\mathrm{2}} {x}+\mathrm{2}{tanx}−\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)=\mathrm{0} \\ $$$${tanx}=\frac{−\mathrm{2}\pm\sqrt{\mathrm{2}^{\mathrm{2}} +\mathrm{4}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)}}{\mathrm{2}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)} \\ $$$${tanx}=\frac{−\mathrm{2}\pm\mathrm{2}\sqrt{\mathrm{1}+\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} }}{\mathrm{2}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)} \\ $$$${tanx}=\frac{−\mathrm{1}\pm\sqrt{\mathrm{1}+\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} }}{\sqrt{\mathrm{2}}−\mathrm{1}} \\ $$$$\because\:\sqrt{\mathrm{1}+\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} }>\mathrm{1}\Rightarrow−\sqrt{\mathrm{1}+\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} }<−\mathrm{1} \\ $$$$\Rightarrow−\mathrm{1}−\sqrt{\mathrm{1}+\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} }<−\mathrm{2}<\mathrm{0}\:{while}\:{tanx}>\mathrm{0}\:{if}\:\mathrm{0}<{x}<\pi/\mathrm{2} \\ $$$$\therefore\:{tanx}=\frac{−\mathrm{1}+\sqrt{\mathrm{1}+\mathrm{2}+\mathrm{1}−\mathrm{2}\sqrt{\mathrm{2}}}}{\sqrt{\mathrm{2}}−\mathrm{1}} \\ $$$${tanx}=\frac{−\mathrm{1}+\sqrt{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{2}}}}{\sqrt{\mathrm{2}}−\mathrm{1}} \\ $$$${tanx}=\frac{\left(−\mathrm{1}+\sqrt{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{2}}}\right)\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)}{\mathrm{2}−\mathrm{1}} \\ $$$${tanx}=\sqrt{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{2}}}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${tanx}=\sqrt{\left(\mathrm{4}−\mathrm{2}\sqrt{\mathrm{2}}\right)\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)^{\mathrm{2}} }−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${tanx}=\sqrt{\left(\mathrm{4}−\mathrm{2}\sqrt{\mathrm{2}}\right)\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)}−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${tanx}=\sqrt{\mathrm{12}−\mathrm{8}+\mathrm{8}\sqrt{\mathrm{2}}−\mathrm{6}\sqrt{\mathrm{2}}}−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${tanx}=\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}}−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${tan}\frac{\pi}{\mathrm{16}}=\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}}−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Commented by Yozzia last updated on 19/Sep/16

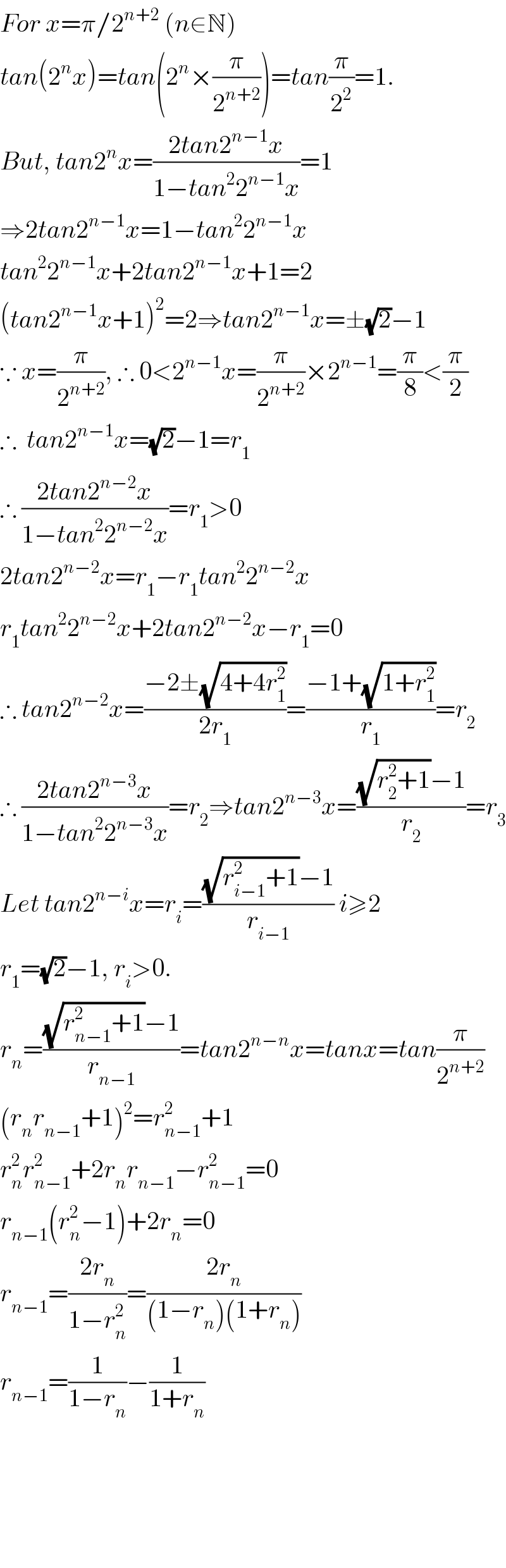
For x=π/2^(n+2) (n∈N) tan(2^n x)=tan(2^n ×(π/2^(n+2) ))=tan(π/2^2 )=1. But, tan2^n x=((2tan2^(n−1) x)/(1−tan^2 2^(n−1) x))=1 ⇒2tan2^(n−1) x=1−tan^2 2^(n−1) x tan^2 2^(n−1) x+2tan2^(n−1) x+1=2 (tan2^(n−1) x+1)^2 =2⇒tan2^(n−1) x=±(√2)−1 ∵ x=(π/2^(n+2) ), ∴ 0<2^(n−1) x=(π/2^(n+2) )×2^(n−1) =(π/8)<(π/2) ∴ tan2^(n−1) x=(√2)−1=r_1 ∴ ((2tan2^(n−2) x)/(1−tan^2 2^(n−2) x))=r_1 >0 2tan2^(n−2) x=r_1 −r_1 tan^2 2^(n−2) x r_1 tan^2 2^(n−2) x+2tan2^(n−2) x−r_1 =0 ∴ tan2^(n−2) x=((−2±(√(4+4r_1 ^2 )))/(2r_1 ))=((−1+(√(1+r_1 ^2 )))/r_1 )=r_2 ∴ ((2tan2^(n−3) x)/(1−tan^2 2^(n−3) x))=r_2 ⇒tan2^(n−3) x=(((√(r_2 ^2 +1))−1)/r_2 )=r_3 Let tan2^(n−i) x=r_i =(((√(r_(i−1) ^2 +1))−1)/r_(i−1) ) i≥2 r_1 =(√2)−1, r_i >0. r_n =(((√(r_(n−1) ^2 +1))−1)/r_(n−1) )=tan2^(n−n) x=tanx=tan(π/2^(n+2) ) (r_n r_(n−1) +1)^2 =r_(n−1) ^2 +1 r_n ^2 r_(n−1) ^2 +2r_n r_(n−1) −r_(n−1) ^2 =0 r_(n−1) (r_n ^2 −1)+2r_n =0 r_(n−1) =((2r_n )/(1−r_n ^2 ))=((2r_n )/((1−r_n )(1+r_n ))) r_(n−1) =(1/(1−r_n ))−(1/(1+r_n ))

$${For}\:{x}=\pi/\mathrm{2}^{{n}+\mathrm{2}} \:\left({n}\in\mathbb{N}\right)\: \\ $$$${tan}\left(\mathrm{2}^{{n}} {x}\right)={tan}\left(\mathrm{2}^{{n}} ×\frac{\pi}{\mathrm{2}^{{n}+\mathrm{2}} }\right)={tan}\frac{\pi}{\mathrm{2}^{\mathrm{2}} }=\mathrm{1}. \\ $$$${But},\:{tan}\mathrm{2}^{{n}} {x}=\frac{\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{1}} {x}}{\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{1}} {x}}=\mathrm{1} \\ $$$$\Rightarrow\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{1}} {x}=\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{1}} {x} \\ $$$${tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{1}} {x}+\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{1}} {x}+\mathrm{1}=\mathrm{2} \\ $$$$\left({tan}\mathrm{2}^{{n}−\mathrm{1}} {x}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{2}\Rightarrow{tan}\mathrm{2}^{{n}−\mathrm{1}} {x}=\pm\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$$\because\:{x}=\frac{\pi}{\mathrm{2}^{{n}+\mathrm{2}} },\:\therefore\:\mathrm{0}<\mathrm{2}^{{n}−\mathrm{1}} {x}=\frac{\pi}{\mathrm{2}^{{n}+\mathrm{2}} }×\mathrm{2}^{{n}−\mathrm{1}} =\frac{\pi}{\mathrm{8}}<\frac{\pi}{\mathrm{2}} \\ $$$$\therefore\:\:{tan}\mathrm{2}^{{n}−\mathrm{1}} {x}=\sqrt{\mathrm{2}}−\mathrm{1}={r}_{\mathrm{1}} \\ $$$$\therefore\:\frac{\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{2}} {x}}{\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{2}} {x}}={r}_{\mathrm{1}} >\mathrm{0} \\ $$$$\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{2}} {x}={r}_{\mathrm{1}} −{r}_{\mathrm{1}} {tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{2}} {x} \\ $$$${r}_{\mathrm{1}} {tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{2}} {x}+\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{2}} {x}−{r}_{\mathrm{1}} =\mathrm{0} \\ $$$$\therefore\:{tan}\mathrm{2}^{{n}−\mathrm{2}} {x}=\frac{−\mathrm{2}\pm\sqrt{\mathrm{4}+\mathrm{4}{r}_{\mathrm{1}} ^{\mathrm{2}} }}{\mathrm{2}{r}_{\mathrm{1}} }=\frac{−\mathrm{1}+\sqrt{\mathrm{1}+{r}_{\mathrm{1}} ^{\mathrm{2}} }}{{r}_{\mathrm{1}} }={r}_{\mathrm{2}} \\ $$$$\therefore\:\frac{\mathrm{2}{tan}\mathrm{2}^{{n}−\mathrm{3}} {x}}{\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{2}^{{n}−\mathrm{3}} {x}}={r}_{\mathrm{2}} \Rightarrow{tan}\mathrm{2}^{{n}−\mathrm{3}} {x}=\frac{\sqrt{{r}_{\mathrm{2}} ^{\mathrm{2}} +\mathrm{1}}−\mathrm{1}}{{r}_{\mathrm{2}} }={r}_{\mathrm{3}} \\ $$$${Let}\:{tan}\mathrm{2}^{{n}−{i}} {x}={r}_{{i}} =\frac{\sqrt{{r}_{{i}−\mathrm{1}} ^{\mathrm{2}} +\mathrm{1}}−\mathrm{1}}{{r}_{{i}−\mathrm{1}} }\:{i}\geqslant\mathrm{2} \\ $$$${r}_{\mathrm{1}} =\sqrt{\mathrm{2}}−\mathrm{1},\:{r}_{{i}} >\mathrm{0}. \\ $$$${r}_{{n}} =\frac{\sqrt{{r}_{{n}−\mathrm{1}} ^{\mathrm{2}} +\mathrm{1}}−\mathrm{1}}{{r}_{{n}−\mathrm{1}} }={tan}\mathrm{2}^{{n}−{n}} {x}={tanx}={tan}\frac{\pi}{\mathrm{2}^{{n}+\mathrm{2}} } \\ $$$$\left({r}_{{n}} {r}_{{n}−\mathrm{1}} +\mathrm{1}\right)^{\mathrm{2}} ={r}_{{n}−\mathrm{1}} ^{\mathrm{2}} +\mathrm{1} \\ $$$${r}_{{n}} ^{\mathrm{2}} {r}_{{n}−\mathrm{1}} ^{\mathrm{2}} +\mathrm{2}{r}_{{n}} {r}_{{n}−\mathrm{1}} −{r}_{{n}−\mathrm{1}} ^{\mathrm{2}} =\mathrm{0} \\ $$$${r}_{{n}−\mathrm{1}} \left({r}_{{n}} ^{\mathrm{2}} −\mathrm{1}\right)+\mathrm{2}{r}_{{n}} =\mathrm{0} \\ $$$${r}_{{n}−\mathrm{1}} =\frac{\mathrm{2}{r}_{{n}} }{\mathrm{1}−{r}_{{n}} ^{\mathrm{2}} }=\frac{\mathrm{2}{r}_{{n}} }{\left(\mathrm{1}−{r}_{{n}} \right)\left(\mathrm{1}+{r}_{{n}} \right)} \\ $$$${r}_{{n}−\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{1}−{r}_{{n}} }−\frac{\mathrm{1}}{\mathrm{1}+{r}_{{n}} } \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Answered by prakash jain last updated on 02/Oct/16

in comments

$$\mathrm{in}\:\mathrm{comments} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com