Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 79538 by Pratah last updated on 26/Jan/20

Commented by john santu last updated on 26/Jan/20

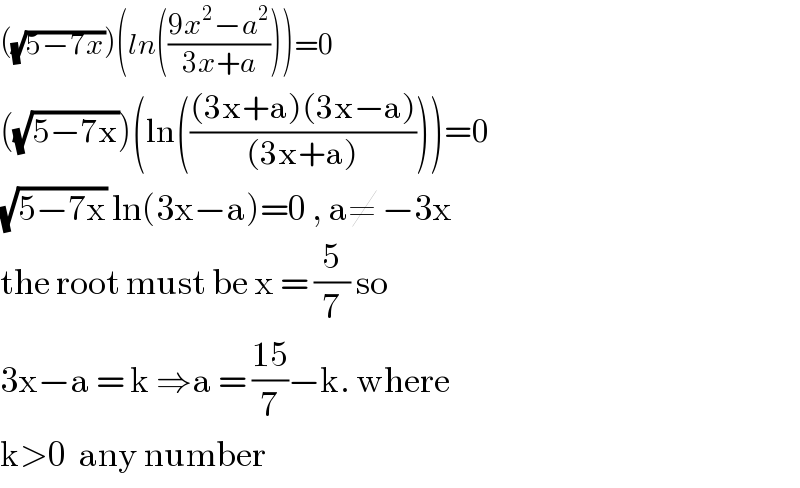
((√(5−7x)))(ln(((9x^2 −a^2 )/(3x+a))))=0 ((√(5−7x)))(ln((((3x+a)(3x−a))/((3x+a)))))=0 (√(5−7x)) ln(3x−a)=0 , a≠ −3x the root must be x = (5/7) so 3x−a = k ⇒a = ((15)/7)−k. where k>0 any number

$$\left(\sqrt{\mathrm{5}−\mathrm{7}{x}}\right)\left({ln}\left(\frac{\mathrm{9}{x}^{\mathrm{2}} −{a}^{\mathrm{2}} }{\mathrm{3}{x}+{a}}\right)\right)=\mathrm{0} \\ $$$$\left(\sqrt{\mathrm{5}−\mathrm{7x}}\right)\left(\mathrm{ln}\left(\frac{\left(\mathrm{3x}+\mathrm{a}\right)\left(\mathrm{3x}−\mathrm{a}\right)}{\left(\mathrm{3x}+\mathrm{a}\right)}\right)\right)=\mathrm{0} \\ $$$$\sqrt{\mathrm{5}−\mathrm{7x}}\:\mathrm{ln}\left(\mathrm{3x}−\mathrm{a}\right)=\mathrm{0}\:,\:\mathrm{a}\neq\:−\mathrm{3x} \\ $$$$\mathrm{the}\:\mathrm{root}\:\mathrm{must}\:\mathrm{be}\:\mathrm{x}\:=\:\frac{\mathrm{5}}{\mathrm{7}}\:\mathrm{so} \\ $$$$\mathrm{3x}−\mathrm{a}\:=\:\mathrm{k}\:\Rightarrow\mathrm{a}\:=\:\frac{\mathrm{15}}{\mathrm{7}}−\mathrm{k}.\:\mathrm{where}\: \\ $$$$\mathrm{k}>\mathrm{0}\:\:\mathrm{any}\:\mathrm{number} \\ $$

Commented by Pratah last updated on 26/Jan/20

thanks

$$\mathrm{thanks} \\ $$

Commented by john santu last updated on 26/Jan/20

this answer right?

$${this}\:{answer}\:{right}? \\ $$

Commented by john santu last updated on 26/Jan/20

oo yes . the equation has one roots. i correct my answer

$$\mathrm{oo}\:\mathrm{yes}\:.\:\mathrm{the}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{one}\:\mathrm{roots}.\: \\ $$$$\mathrm{i}\:\mathrm{correct}\:\mathrm{my}\:\mathrm{answer} \\ $$

Commented by john santu last updated on 26/Jan/20

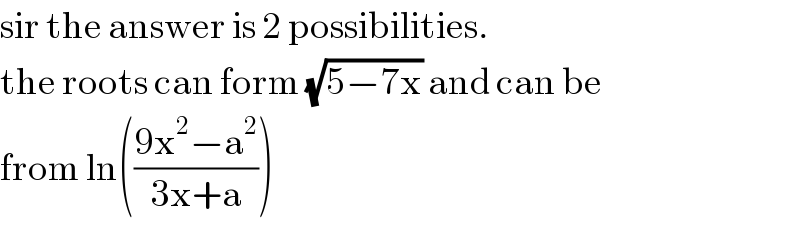
sir the answer is 2 possibilities. the roots can form (√(5−7x)) and can be from ln(((9x^2 −a^2 )/(3x+a)))

$$\mathrm{sir}\:\mathrm{the}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{2}\:\mathrm{possibilities}.\: \\ $$$$\mathrm{the}\:\mathrm{roots}\:\mathrm{can}\:\mathrm{form}\:\sqrt{\mathrm{5}−\mathrm{7x}}\:\mathrm{and}\:\mathrm{can}\:\mathrm{be}\: \\ $$$$\mathrm{from}\:\mathrm{ln}\left(\frac{\mathrm{9x}^{\mathrm{2}} −\mathrm{a}^{\mathrm{2}} }{\mathrm{3x}+\mathrm{a}}\right) \\ $$

Commented by john santu last updated on 26/Jan/20

when we take the root out of shape (√(5−7x)) , so must be a≠ (8/7) ∧a≠−((15)/7)

$$\mathrm{when}\:\mathrm{we}\:\mathrm{take}\:\mathrm{the}\:\mathrm{root}\:\mathrm{out}\: \\ $$$$\mathrm{of}\:\mathrm{shape}\:\sqrt{\mathrm{5}−\mathrm{7x}}\:,\:\mathrm{so}\:\mathrm{must}\:\mathrm{be}\: \\ $$$$\mathrm{a}\neq\:\frac{\mathrm{8}}{\mathrm{7}}\:\wedge\mathrm{a}\neq−\frac{\mathrm{15}}{\mathrm{7}} \\ $$

Commented by jagoll last updated on 26/Jan/20

please post your solution sir

$$\mathrm{please}\:\mathrm{post}\:\mathrm{your}\:\mathrm{solution}\:\mathrm{sir} \\ $$

Commented by mr W last updated on 26/Jan/20

to jagoll sir: are you talking to Pratah sir? then you can directly talk to a wall. a wall may give yoy an echo back, but he not.

$${to}\:{jagoll}\:{sir}: \\ $$$${are}\:{you}\:{talking}\:{to}\:{Pratah}\:{sir}?\:{then} \\ $$$${you}\:{can}\:{directly}\:{talk}\:{to}\:{a}\:{wall}.\:{a}\:{wall} \\ $$$${may}\:{give}\:{yoy}\:{an}\:{echo}\:{back},\:{but}\:{he}\:{not}. \\ $$

Commented by jagoll last updated on 26/Jan/20

to Mr W sir. i need your explain

$$\mathrm{to}\:\mathrm{Mr}\:\mathrm{W}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{need}\:\mathrm{your}\:\mathrm{explain} \\ $$

Commented by jagoll last updated on 26/Jan/20

i′m interested the quation

$$\mathrm{i}'\mathrm{m}\:\mathrm{interested}\:\mathrm{the}\:\mathrm{quation} \\ $$

Commented by john santu last updated on 26/Jan/20

yes sir, i wonder if this is an ambiguous?

$$\mathrm{yes}\:\mathrm{sir},\:\mathrm{i}\:\mathrm{wonder}\:\mathrm{if}\:\mathrm{this}\:\mathrm{is}\:\mathrm{an}\:\mathrm{ambiguous}? \\ $$

Commented by john santu last updated on 26/Jan/20

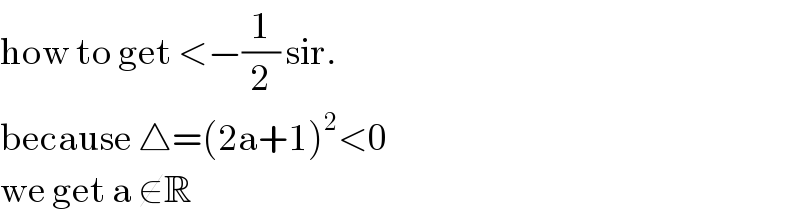
how to get <−(1/2) sir. because △=(2a+1)^2 <0 we get a ∉R

$$\mathrm{how}\:\mathrm{to}\:\mathrm{get}\:<−\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{sir}.\:\: \\ $$$$\mathrm{because}\:\bigtriangleup=\left(\mathrm{2a}+\mathrm{1}\right)^{\mathrm{2}} <\mathrm{0}\: \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{a}\:\notin\mathbb{R} \\ $$

Commented by john santu last updated on 26/Jan/20

thank you sir

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$

Answered by mr W last updated on 26/Jan/20

the original equation can be formed to (√(5−7x)){ln (9x^2 −a^2 )−ln (3x+a)}=0 without “loss”. such that the equation makes sense, 5−7x≥0 ⇒x≤(5/7) 3x+a>0 9x^2 −a^2 >0 ⇒(3x−a)(3x+a)>0 ⇒3x−a>0 we see the original eqn. has the root x=(5/7), when (3×(5/7))^2 >a^2 ⇒−((15)/7)<a<((15)/7) and 3×(5/7)>−a ⇒a>−((15)/7) that means if −((15)/7)<a<((15)/7) the eqn. has always the root x=(5/7). such that the eqn. has only this root, the equation ln (9x^2 −a^2 )−ln (3x+a)=0 should have no root or only the root (5/7). ln (9x^2 −a^2 )−ln (3x+a)=0 ⇒ln (3x−a)=0 ⇒3x−a=1 it has always a root x=((a+1)/3) so this root must be (5/7). ((a+1)/3)=(5/7) ⇒a=(8/7) which lies in (−((15)/7),((15)/7)) therefore the answer is when a=(8/7), the original equation has “one” root.

$${the}\:{original}\:{equation}\:{can}\:{be}\:{formed}\:{to} \\ $$$$\sqrt{\mathrm{5}−\mathrm{7}{x}}\left\{\mathrm{ln}\:\left(\mathrm{9}{x}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)−\mathrm{ln}\:\left(\mathrm{3}{x}+{a}\right)\right\}=\mathrm{0} \\ $$$${without}\:``{loss}''. \\ $$$${such}\:{that}\:{the}\:{equation}\:{makes}\:{sense}, \\ $$$$\mathrm{5}−\mathrm{7}{x}\geqslant\mathrm{0}\:\Rightarrow{x}\leqslant\frac{\mathrm{5}}{\mathrm{7}} \\ $$$$\mathrm{3}{x}+{a}>\mathrm{0}\: \\ $$$$\mathrm{9}{x}^{\mathrm{2}} −{a}^{\mathrm{2}} >\mathrm{0}\:\Rightarrow\left(\mathrm{3}{x}−{a}\right)\left(\mathrm{3}{x}+{a}\right)>\mathrm{0}\:\Rightarrow\mathrm{3}{x}−{a}>\mathrm{0} \\ $$$$ \\ $$$${we}\:{see}\:{the}\:{original}\:{eqn}.\:{has}\:{the}\:{root} \\ $$$${x}=\frac{\mathrm{5}}{\mathrm{7}},\:{when}\:\left(\mathrm{3}×\frac{\mathrm{5}}{\mathrm{7}}\right)^{\mathrm{2}} >{a}^{\mathrm{2}} \:\Rightarrow−\frac{\mathrm{15}}{\mathrm{7}}<{a}<\frac{\mathrm{15}}{\mathrm{7}} \\ $$$${and}\:\mathrm{3}×\frac{\mathrm{5}}{\mathrm{7}}>−{a}\:\Rightarrow{a}>−\frac{\mathrm{15}}{\mathrm{7}} \\ $$$${that}\:{means}\:{if}\:−\frac{\mathrm{15}}{\mathrm{7}}<{a}<\frac{\mathrm{15}}{\mathrm{7}}\:{the}\:{eqn}. \\ $$$${has}\:{always}\:{the}\:{root}\:{x}=\frac{\mathrm{5}}{\mathrm{7}}. \\ $$$${such}\:{that}\:{the}\:{eqn}.\:{has}\:{only}\:{this}\:{root}, \\ $$$${the}\:{equation} \\ $$$$\mathrm{ln}\:\left(\mathrm{9}{x}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)−\mathrm{ln}\:\left(\mathrm{3}{x}+{a}\right)=\mathrm{0} \\ $$$${should}\:{have}\:{no}\:{root}\:{or}\:{only}\:{the}\:{root}\:\frac{\mathrm{5}}{\mathrm{7}}. \\ $$$$\mathrm{ln}\:\left(\mathrm{9}{x}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)−\mathrm{ln}\:\left(\mathrm{3}{x}+{a}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{ln}\:\left(\mathrm{3}{x}−{a}\right)=\mathrm{0}\:\Rightarrow\mathrm{3}{x}−{a}=\mathrm{1} \\ $$$${it}\:{has}\:{always}\:{a}\:{root}\:{x}=\frac{{a}+\mathrm{1}}{\mathrm{3}} \\ $$$${so}\:{this}\:{root}\:{must}\:{be}\:\frac{\mathrm{5}}{\mathrm{7}}. \\ $$$$\frac{{a}+\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{5}}{\mathrm{7}} \\ $$$$\Rightarrow{a}=\frac{\mathrm{8}}{\mathrm{7}}\:{which}\:{lies}\:{in}\:\left(−\frac{\mathrm{15}}{\mathrm{7}},\frac{\mathrm{15}}{\mathrm{7}}\right) \\ $$$$ \\ $$$${therefore}\:{the}\:{answer}\:{is} \\ $$$${when}\:{a}=\frac{\mathrm{8}}{\mathrm{7}},\:{the}\:{original}\:{equation} \\ $$$${has}\:``{one}''\:{root}. \\ $$

Commented by mr W last updated on 26/Jan/20

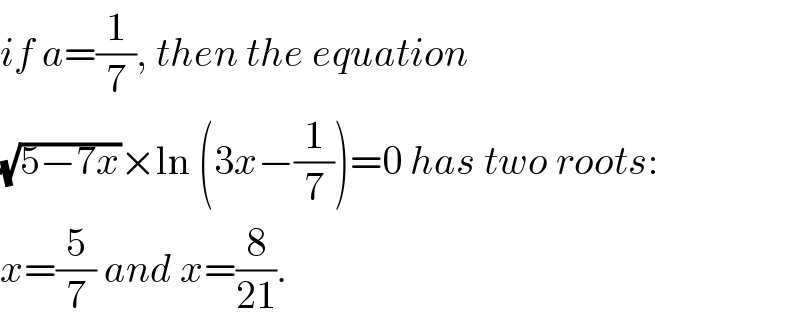
if a=(1/7), then the equation (√(5−7x))×ln (3x−(1/7))=0 has two roots: x=(5/7) and x=(8/(21)).

$${if}\:{a}=\frac{\mathrm{1}}{\mathrm{7}},\:{then}\:{the}\:{equation} \\ $$$$\sqrt{\mathrm{5}−\mathrm{7}{x}}×\mathrm{ln}\:\left(\mathrm{3}{x}−\frac{\mathrm{1}}{\mathrm{7}}\right)=\mathrm{0}\:{has}\:{two}\:{roots}: \\ $$$${x}=\frac{\mathrm{5}}{\mathrm{7}}\:{and}\:{x}=\frac{\mathrm{8}}{\mathrm{21}}. \\ $$

Commented by john santu last updated on 26/Jan/20

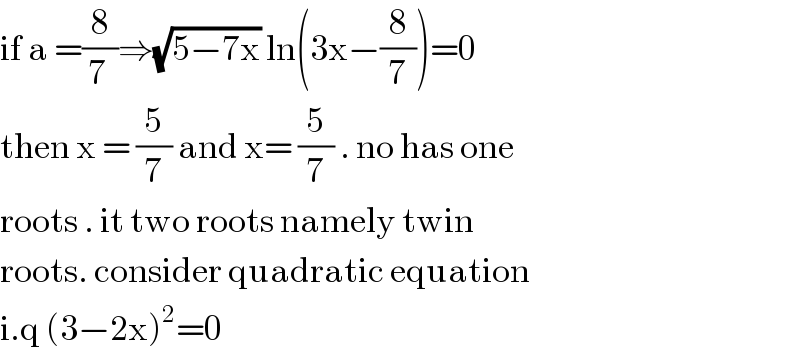
if a =(8/(7 ))⇒(√(5−7x)) ln(3x−(8/7))=0 then x = (5/7) and x= (5/7) . no has one roots . it two roots namely twin roots. consider quadratic equation i.q (3−2x)^2 =0

$$\mathrm{if}\:\mathrm{a}\:=\frac{\mathrm{8}}{\mathrm{7}\:}\Rightarrow\sqrt{\mathrm{5}−\mathrm{7x}}\:\mathrm{ln}\left(\mathrm{3x}−\frac{\mathrm{8}}{\mathrm{7}}\right)=\mathrm{0} \\ $$$$\mathrm{then}\:\mathrm{x}\:=\:\frac{\mathrm{5}}{\mathrm{7}}\:\mathrm{and}\:\mathrm{x}=\:\frac{\mathrm{5}}{\mathrm{7}}\:.\:\mathrm{no}\:\mathrm{has}\:\mathrm{one}\: \\ $$$$\mathrm{roots}\:.\:\mathrm{it}\:\mathrm{two}\:\mathrm{roots}\:\mathrm{namely}\:\mathrm{twin} \\ $$$$\mathrm{roots}.\:\mathrm{consider}\:\mathrm{quadratic}\:\mathrm{equation} \\ $$$$\mathrm{i}.\mathrm{q}\:\left(\mathrm{3}−\mathrm{2x}\right)^{\mathrm{2}} =\mathrm{0} \\ $$

Commented by john santu last updated on 26/Jan/20

i think the value of ln(3x−a) not must be = 0 or 3x−a=1 . let if 3x−a=2 a=((15)/7)−2= (1/7)

$$\mathrm{i}\:\mathrm{think}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{ln}\left(\mathrm{3x}−\mathrm{a}\right)\: \\ $$$$\mathrm{not}\:\mathrm{must}\:\mathrm{be}\:=\:\mathrm{0}\:\mathrm{or}\:\mathrm{3x}−\mathrm{a}=\mathrm{1}\:.\:\mathrm{let}\:\mathrm{if}\:\mathrm{3x}−\mathrm{a}=\mathrm{2} \\ $$$$\mathrm{a}=\frac{\mathrm{15}}{\mathrm{7}}−\mathrm{2}=\:\frac{\mathrm{1}}{\mathrm{7}} \\ $$

Commented by mr W last updated on 26/Jan/20

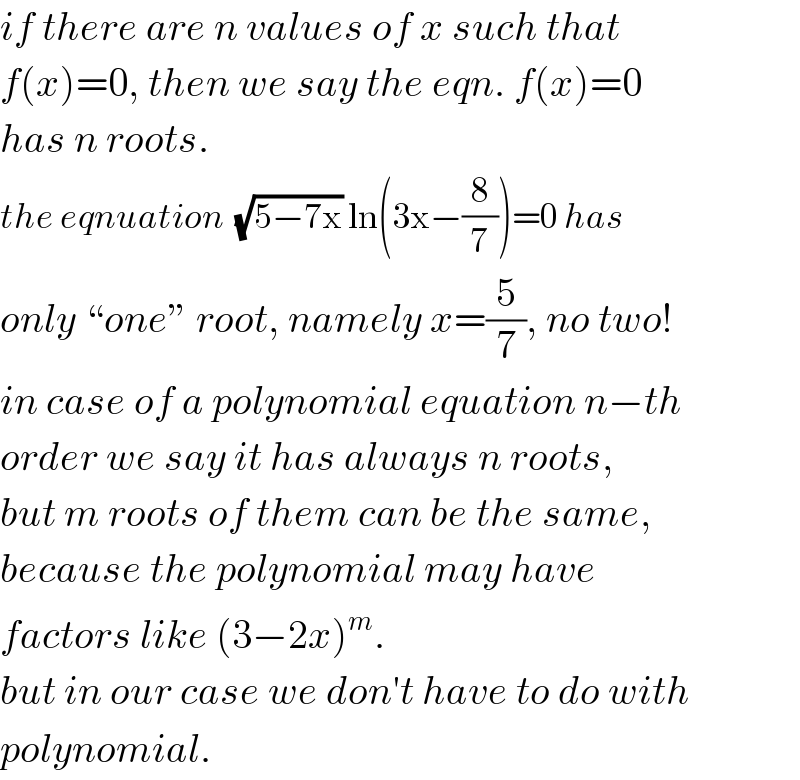
if there are n values of x such that f(x)=0, then we say the eqn. f(x)=0 has n roots. the eqnuation (√(5−7x)) ln(3x−(8/7))=0 has only “one” root, namely x=(5/7), no two! in case of a polynomial equation n−th order we say it has always n roots, but m roots of them can be the same, because the polynomial may have factors like (3−2x)^m . but in our case we don′t have to do with polynomial.

$${if}\:{there}\:{are}\:{n}\:{values}\:{of}\:{x}\:{such}\:{that} \\ $$$${f}\left({x}\right)=\mathrm{0},\:{then}\:{we}\:{say}\:{the}\:{eqn}.\:{f}\left({x}\right)=\mathrm{0} \\ $$$${has}\:{n}\:{roots}. \\ $$$${the}\:{eqnuation}\:\:\sqrt{\mathrm{5}−\mathrm{7x}}\:\mathrm{ln}\left(\mathrm{3x}−\frac{\mathrm{8}}{\mathrm{7}}\right)=\mathrm{0}\:{has} \\ $$$${only}\:``{one}''\:{root},\:{namely}\:{x}=\frac{\mathrm{5}}{\mathrm{7}},\:{no}\:{two}! \\ $$$${in}\:{case}\:{of}\:{a}\:{polynomial}\:{equation}\:{n}−{th} \\ $$$${order}\:{we}\:{say}\:{it}\:{has}\:{always}\:{n}\:{roots}, \\ $$$${but}\:{m}\:{roots}\:{of}\:{them}\:{can}\:{be}\:{the}\:{same}, \\ $$$${because}\:{the}\:{polynomial}\:{may}\:{have} \\ $$$${factors}\:{like}\:\left(\mathrm{3}−\mathrm{2}{x}\right)^{{m}} . \\ $$$${but}\:{in}\:{our}\:{case}\:{we}\:{don}'{t}\:{have}\:{to}\:{do}\:{with} \\ $$$${polynomial}. \\ $$

Commented by mr W last updated on 26/Jan/20

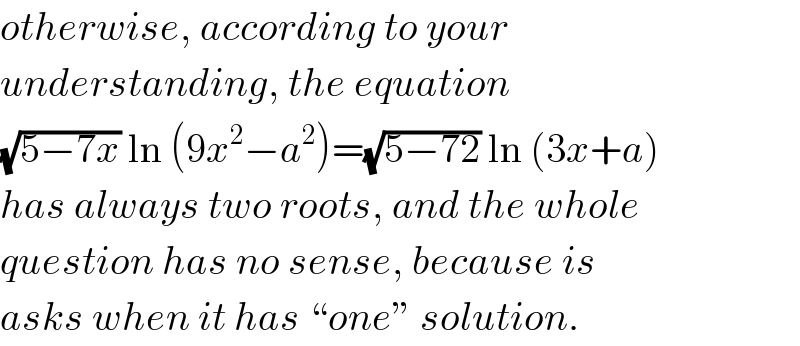
otherwise, according to your understanding, the equation (√(5−7x)) ln (9x^2 −a^2 )=(√(5−72)) ln (3x+a) has always two roots, and the whole question has no sense, because is asks when it has “one” solution.

$${otherwise},\:{according}\:{to}\:{your} \\ $$$${understanding},\:{the}\:{equation} \\ $$$$\sqrt{\mathrm{5}−\mathrm{7}{x}}\:\mathrm{ln}\:\left(\mathrm{9}{x}^{\mathrm{2}} −{a}^{\mathrm{2}} \right)=\sqrt{\mathrm{5}−\mathrm{72}}\:\mathrm{ln}\:\left(\mathrm{3}{x}+{a}\right) \\ $$$${has}\:{always}\:{two}\:{roots},\:{and}\:{the}\:{whole} \\ $$$${question}\:{has}\:{no}\:{sense},\:{because}\:{is} \\ $$$${asks}\:{when}\:{it}\:{has}\:``{one}''\:{solution}. \\ $$

Commented by john santu last updated on 26/Jan/20

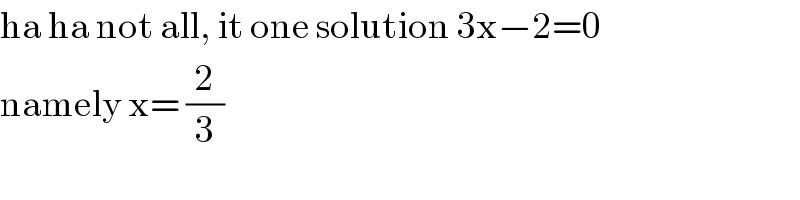
ha ha not all, it one solution 3x−2=0 namely x= (2/3)

$$\mathrm{ha}\:\mathrm{ha}\:\mathrm{not}\:\mathrm{all},\:\mathrm{it}\:\mathrm{one}\:\mathrm{solution}\:\mathrm{3x}−\mathrm{2}=\mathrm{0} \\ $$$$\mathrm{namely}\:\mathrm{x}=\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$

Commented by john santu last updated on 26/Jan/20

ok deal a = (8/7)

$$\mathrm{ok}\:\mathrm{deal}\:\mathrm{a}\:=\:\frac{\mathrm{8}}{\mathrm{7}} \\ $$

Commented by john santu last updated on 26/Jan/20

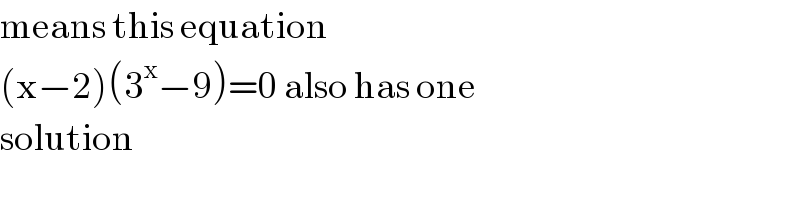
means this equation (x−2)(3^x −9)=0 also has one solution

$$\mathrm{means}\:\mathrm{this}\:\mathrm{equation}\: \\ $$$$\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{3}^{\mathrm{x}} −\mathrm{9}\right)=\mathrm{0}\:\mathrm{also}\:\mathrm{has}\:\mathrm{one}\: \\ $$$$\mathrm{solution} \\ $$

Commented by mr W last updated on 26/Jan/20

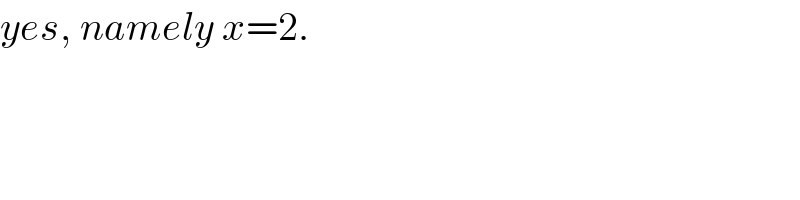
yes, namely x=2.

$${yes},\:{namely}\:{x}=\mathrm{2}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com