Question and Answers Forum

All Questions      Topic List

Mensuration Questions

Previous in All Question      Next in All Question      

Previous in Mensuration      Next in Mensuration      

Question Number 8016 by Nayon last updated on 28/Sep/16

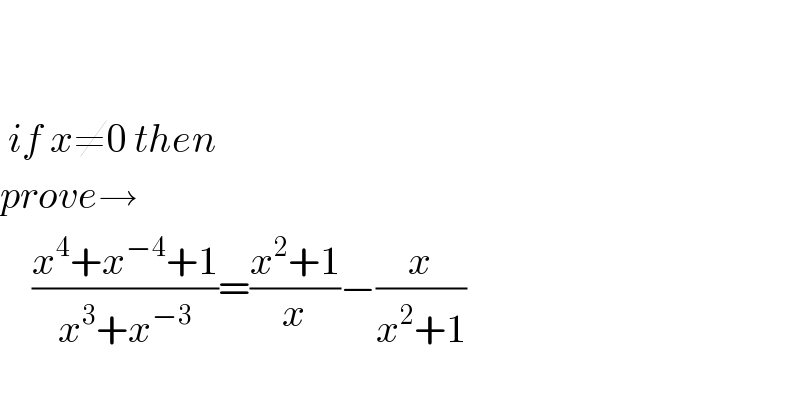
if x≠0 then prove→ ((x^4 +x^(−4) +1)/(x^3 +x^(−3) ))=((x^2 +1)/x)−(x/(x^2 +1))

$$ \\ $$$$ \\ $$$$\:{if}\:{x}\neq\mathrm{0}\:{then} \\ $$$${prove}\rightarrow \\ $$$$\:\:\:\:\frac{{x}^{\mathrm{4}} +{x}^{−\mathrm{4}} +\mathrm{1}}{{x}^{\mathrm{3}} +{x}^{−\mathrm{3}} }=\frac{{x}^{\mathrm{2}} +\mathrm{1}}{{x}}−\frac{{x}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$

Commented by FilupSmith last updated on 28/Sep/16

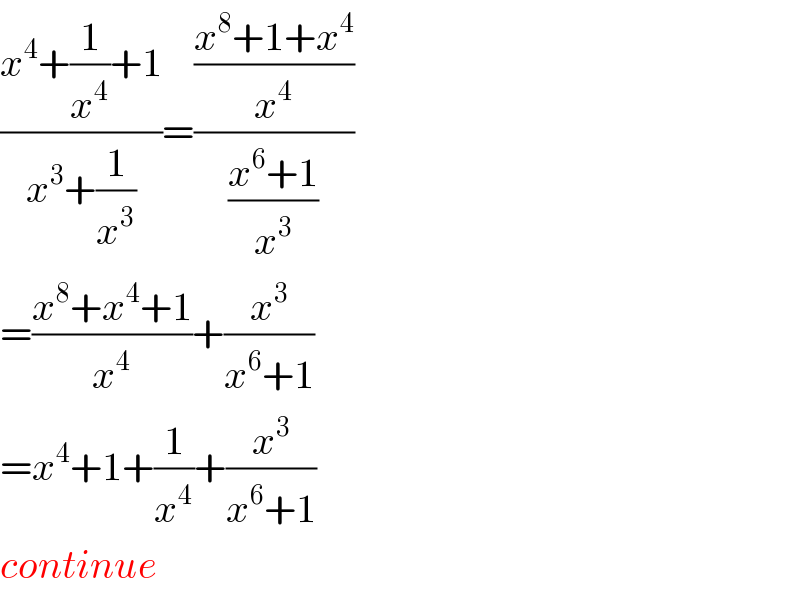
((x^4 +(1/x^4 )+1)/(x^3 +(1/x^3 )))=(((x^8 +1+x^4 )/x^4 )/((x^6 +1)/x^3 )) =((x^8 +x^4 +1)/x^4 )+(x^3 /(x^6 +1)) =x^4 +1+(1/x^4 )+(x^3 /(x^6 +1)) continue

$$\frac{{x}^{\mathrm{4}} +\frac{\mathrm{1}}{{x}^{\mathrm{4}} }+\mathrm{1}}{{x}^{\mathrm{3}} +\frac{\mathrm{1}}{{x}^{\mathrm{3}} }}=\frac{\frac{{x}^{\mathrm{8}} +\mathrm{1}+{x}^{\mathrm{4}} }{{x}^{\mathrm{4}} }}{\frac{{x}^{\mathrm{6}} +\mathrm{1}}{{x}^{\mathrm{3}} }} \\ $$$$=\frac{{x}^{\mathrm{8}} +{x}^{\mathrm{4}} +\mathrm{1}}{{x}^{\mathrm{4}} }+\frac{{x}^{\mathrm{3}} }{{x}^{\mathrm{6}} +\mathrm{1}} \\ $$$$={x}^{\mathrm{4}} +\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{4}} }+\frac{{x}^{\mathrm{3}} }{{x}^{\mathrm{6}} +\mathrm{1}} \\ $$$${continue} \\ $$

Commented by Nayon last updated on 28/Sep/16

wrong way filup

$${wrong}\:{way}\:{filup}\: \\ $$

Answered by Rasheed Soomro last updated on 28/Sep/16

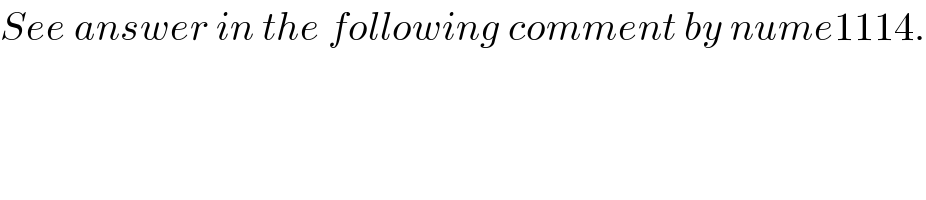
See answer in the following comment by nume1114.

$${See}\:{answer}\:{in}\:{the}\:{following}\:{comment}\:{by}\:{nume}\mathrm{1114}. \\ $$

Commented by Nayon last updated on 28/Sep/16

the question is that to prove it. it′s a identity, not only a equetion.

$${the}\:{question}\:{is}\:{that}\:{to}\:{prove}\:{it}.\:{it}'{s}\:{a}\:{identity}, \\ $$$${not}\:{only}\:{a}\:{equetion}. \\ $$

Commented by Rasheed Soomro last updated on 28/Sep/16

Sorry I misread.

$${Sorry}\:{I}\:{misread}. \\ $$

Commented by nume1114 last updated on 28/Sep/16

Let x+x^(−1) =y x^2 +x^(−2) =y^2 −2 x^3 +x^(−3) =y^3 −3y x^4 +x^(−4) =y^4 −4y^2 +2 LHS=((y^4 −4y^2 +3)/(y^3 −3y))=(((y^2 −3)(y^2 −1))/(y(y^2 −3))) [((if x>0)),((y=x+x^(−1) ≥2(√(xx^(−1) ))=2)),((if x<0)),((−y=−x+(−x)^(−1) ≥2(√((−x)∙(−x^(−1) )))=2)),((y≤−2)),((so,y^2 −3≠0)) ] (((y^2 −3)(y^2 −1))/(y(y^2 −3)))=((y^2 −1)/y)=y−(1/y) =x+(1/x)−(1/(x+(1/x)))=RHS Proved

$${Let}\:{x}+{x}^{−\mathrm{1}} ={y} \\ $$$${x}^{\mathrm{2}} +{x}^{−\mathrm{2}} ={y}^{\mathrm{2}} −\mathrm{2} \\ $$$${x}^{\mathrm{3}} +{x}^{−\mathrm{3}} ={y}^{\mathrm{3}} −\mathrm{3}{y} \\ $$$${x}^{\mathrm{4}} +{x}^{−\mathrm{4}} ={y}^{\mathrm{4}} −\mathrm{4}{y}^{\mathrm{2}} +\mathrm{2} \\ $$$${LHS}=\frac{{y}^{\mathrm{4}} −\mathrm{4}{y}^{\mathrm{2}} +\mathrm{3}}{{y}^{\mathrm{3}} −\mathrm{3}{y}}=\frac{\left({y}^{\mathrm{2}} −\mathrm{3}\right)\left({y}^{\mathrm{2}} −\mathrm{1}\right)}{{y}\left({y}^{\mathrm{2}} −\mathrm{3}\right)} \\ $$$$\:\:\:\begin{bmatrix}{{if}\:{x}>\mathrm{0}}\\{{y}={x}+{x}^{−\mathrm{1}} \geqslant\mathrm{2}\sqrt{{xx}^{−\mathrm{1}} }=\mathrm{2}}\\{{if}\:{x}<\mathrm{0}}\\{−{y}=−{x}+\left(−{x}\right)^{−\mathrm{1}} \geqslant\mathrm{2}\sqrt{\left(−{x}\right)\centerdot\left(−{x}^{−\mathrm{1}} \right)}=\mathrm{2}}\\{{y}\leqslant−\mathrm{2}}\\{{so},{y}^{\mathrm{2}} −\mathrm{3}\neq\mathrm{0}}\end{bmatrix} \\ $$$$\:\:\:\:\frac{\left({y}^{\mathrm{2}} −\mathrm{3}\right)\left({y}^{\mathrm{2}} −\mathrm{1}\right)}{{y}\left({y}^{\mathrm{2}} −\mathrm{3}\right)}=\frac{{y}^{\mathrm{2}} −\mathrm{1}}{{y}}={y}−\frac{\mathrm{1}}{{y}} \\ $$$$={x}+\frac{\mathrm{1}}{{x}}−\frac{\mathrm{1}}{{x}+\frac{\mathrm{1}}{{x}}}={RHS} \\ $$$${Proved} \\ $$

Answered by sandy_suhendra last updated on 28/Sep/16

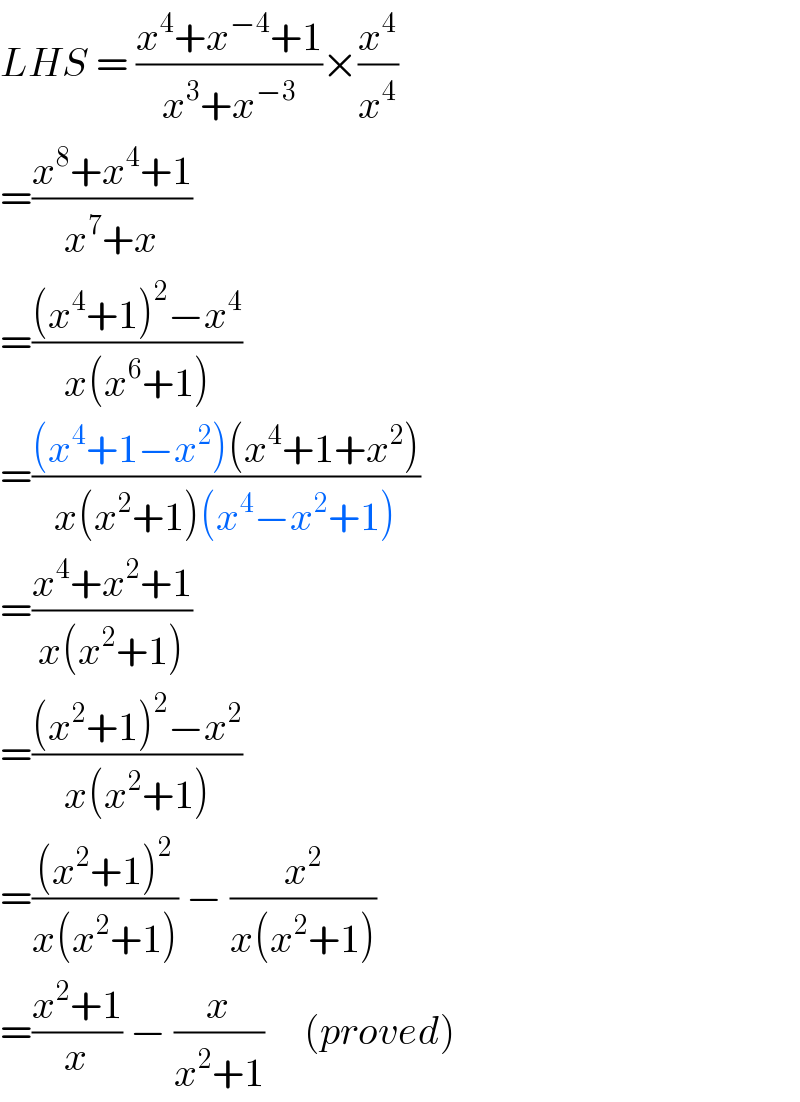
LHS = ((x^4 +x^(−4) +1)/(x^3 +x^(−3) ))×(x^4 /x^4 ) =((x^8 +x^4 +1)/(x^7 +x)) =(((x^4 +1)^2 −x^4 )/(x(x^6 +1))) =(((x^4 +1−x^2 )(x^4 +1+x^2 ))/(x(x^2 +1)(x^4 −x^2 +1))) =((x^4 +x^2 +1)/(x(x^2 +1))) =(((x^2 +1)^2 −x^2 )/(x(x^2 +1))) =(((x^2 +1)^2 )/(x(x^2 +1))) − (x^2 /(x(x^2 +1))) =((x^2 +1)/x) − (x/(x^2 +1)) (proved)

$${LHS}\:=\:\frac{{x}^{\mathrm{4}} +{x}^{−\mathrm{4}} +\mathrm{1}}{{x}^{\mathrm{3}} +{x}^{−\mathrm{3}} }×\frac{{x}^{\mathrm{4}} }{{x}^{\mathrm{4}} } \\ $$$$=\frac{{x}^{\mathrm{8}} +{x}^{\mathrm{4}} +\mathrm{1}}{{x}^{\mathrm{7}} +{x}} \\ $$$$=\frac{\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} −{x}^{\mathrm{4}} }{{x}\left({x}^{\mathrm{6}} +\mathrm{1}\right)} \\ $$$$=\frac{\left({x}^{\mathrm{4}} +\mathrm{1}−{x}^{\mathrm{2}} \right)\left({x}^{\mathrm{4}} +\mathrm{1}+{x}^{\mathrm{2}} \right)}{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{4}} −{x}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$=\frac{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$=\frac{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −{x}^{\mathrm{2}} }{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$=\frac{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)}\:−\:\frac{{x}^{\mathrm{2}} }{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$=\frac{{x}^{\mathrm{2}} +\mathrm{1}}{{x}}\:−\:\frac{{x}}{{x}^{\mathrm{2}} +\mathrm{1}}\:\:\:\:\:\left({proved}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com