Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 80180 by mathocean1 last updated on 31/Jan/20

h(x)=((x−x^2 )/(x+1)) we defined this function on R−{−1}→R 1) Study the variations of h then draw up its table of variation. please sirs i need your kind help

$$\mathrm{h}\left({x}\right)=\frac{{x}−{x}^{\mathrm{2}} }{{x}+\mathrm{1}} \\ $$$${we}\:{defined}\:{this}\:{function}\:{on} \\ $$$$\mathbb{R}−\left\{−\mathrm{1}\right\}\rightarrow\mathbb{R} \\ $$$$ \\ $$$$\left.\mathrm{1}\right)\:\mathrm{Study}\:\mathrm{the}\:\mathrm{variations}\:\mathrm{of}\:\mathrm{h}\:\mathrm{then} \\ $$$$\mathrm{draw}\:\mathrm{up}\:\mathrm{its}\:\mathrm{table}\:\mathrm{of}\:\mathrm{variation}. \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{sirs}\:\mathrm{i}\:\mathrm{need}\:\mathrm{your}\:\mathrm{kind}\:\mathrm{help} \\ $$

Answered by Rio Michael last updated on 31/Jan/20

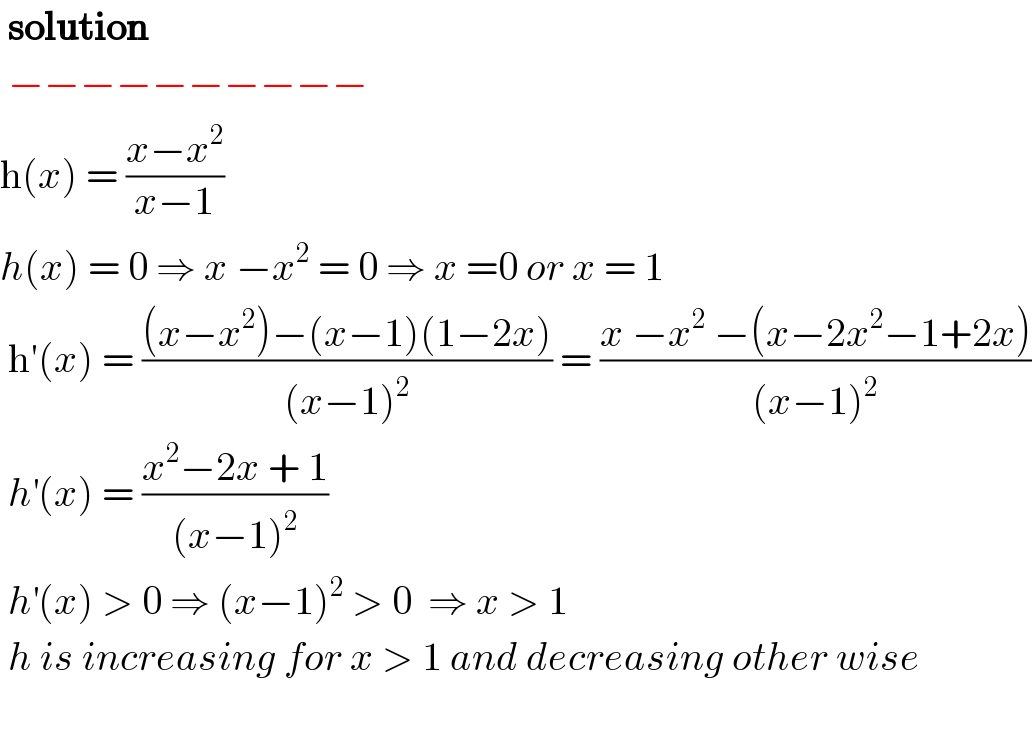
solution −−−−−−−−−− h(x) = ((x−x^2 )/(x−1)) h(x) = 0 ⇒ x −x^2 = 0 ⇒ x =0 or x = 1 h′(x) = (((x−x^2 )−(x−1)(1−2x))/((x−1)^2 )) = ((x −x^2 −(x−2x^2 −1+2x))/((x−1)^2 )) h^′ (x) = ((x^2 −2x + 1)/((x−1)^2 )) h^′ (x) > 0 ⇒ (x−1)^2 > 0 ⇒ x > 1 h is increasing for x > 1 and decreasing other wise

$$\:\boldsymbol{\mathrm{solution}} \\ $$$$\:−−−−−−−−−− \\ $$$$\mathrm{h}\left({x}\right)\:=\:\frac{{x}−{x}^{\mathrm{2}} }{{x}−\mathrm{1}} \\ $$$${h}\left({x}\right)\:=\:\mathrm{0}\:\Rightarrow\:{x}\:−{x}^{\mathrm{2}} \:=\:\mathrm{0}\:\Rightarrow\:{x}\:=\mathrm{0}\:{or}\:{x}\:=\:\mathrm{1} \\ $$$$\:\mathrm{h}'\left({x}\right)\:=\:\frac{\left({x}−{x}^{\mathrm{2}} \right)−\left({x}−\mathrm{1}\right)\left(\mathrm{1}−\mathrm{2}{x}\right)}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} }\:=\:\frac{{x}\:−{x}^{\mathrm{2}} \:−\left({x}−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}+\mathrm{2}{x}\right)}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\:{h}^{'} \left({x}\right)\:=\:\frac{{x}^{\mathrm{2}} −\mathrm{2}{x}\:+\:\mathrm{1}}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} }\: \\ $$$$\:{h}^{'} \left({x}\right)\:>\:\mathrm{0}\:\Rightarrow\:\left({x}−\mathrm{1}\right)^{\mathrm{2}} \:>\:\mathrm{0}\:\:\Rightarrow\:{x}\:>\:\mathrm{1} \\ $$$$\:{h}\:{is}\:{increasing}\:{for}\:{x}\:>\:\mathrm{1}\:{and}\:{decreasing}\:{other}\:{wise} \\ $$$$ \\ $$

Commented by mathocean1 last updated on 31/Jan/20

Please sir h′ is not like this: h′=(((1−2x)(x+1)−(x−x^2 ))/((x+1)^2 )) ?

$$\mathrm{Please}\:\mathrm{sir}\:\mathrm{h}'\:\mathrm{is}\:\mathrm{not}\:\mathrm{like}\:\mathrm{this}: \\ $$$$\mathrm{h}'=\frac{\left(\mathrm{1}−\mathrm{2}{x}\right)\left({x}+\mathrm{1}\right)−\left({x}−{x}^{\mathrm{2}} \right)}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }\:\:?\:\: \\ $$$$\: \\ $$

Commented by mathocean1 last updated on 31/Jan/20

h(x)=((x−x^2 )/(x+1))

$$\mathrm{h}\left({x}\right)=\frac{{x}−{x}^{\mathrm{2}} }{{x}+\mathrm{1}} \\ $$

Commented by Rio Michael last updated on 31/Jan/20

sorry i copied the question wrongly

$${sorry}\:{i}\:{copied}\:{the}\:{question}\:{wrongly} \\ $$

Commented by mathocean1 last updated on 31/Jan/20

please sir how can i draw up its table of variation when h′=((−x^2 −2x+1)/((x+1)^2 ))=−1 ?

$$\mathrm{please}\:\mathrm{sir}\:\mathrm{how}\:\mathrm{can}\:\mathrm{i}\:\mathrm{draw}\:\mathrm{up}\:\mathrm{its}\: \\ $$$$\mathrm{table}\:\mathrm{of}\:\mathrm{variation}\:\mathrm{when}\: \\ $$$$\mathrm{h}'=\frac{−{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }=−\mathrm{1}\:? \\ $$

Commented by JDamian last updated on 01/Feb/20

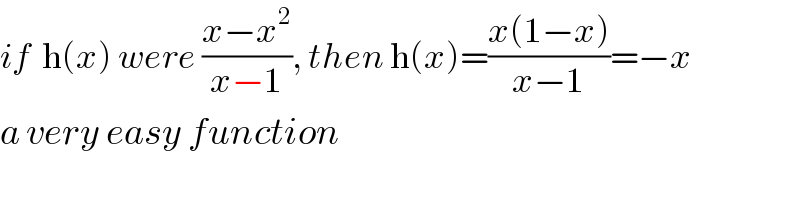
if h(x) were ((x−x^2 )/(x−1)), then h(x)=((x(1−x))/(x−1))=−x a very easy function

$${if}\:\:\mathrm{h}\left({x}\right)\:{were}\:\frac{{x}−{x}^{\mathrm{2}} }{{x}−\mathrm{1}},\:{then}\:\mathrm{h}\left({x}\right)=\frac{{x}\left(\mathrm{1}−{x}\right)}{{x}−\mathrm{1}}=−{x} \\ $$$${a}\:{very}\:{easy}\:{function} \\ $$

Commented by mathocean1 last updated on 01/Feb/20

so... we can study h(x)=((x−x^2 )/(x+1))???

$$\mathrm{so}...\:\mathrm{we}\:\mathrm{can}\:\mathrm{study}\:\mathrm{h}\left({x}\right)=\frac{{x}−{x}^{\mathrm{2}} }{{x}+\mathrm{1}}??? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com