
Question and Answers Forum
Question Number 80334 by ~blr237~ last updated on 02/Feb/20
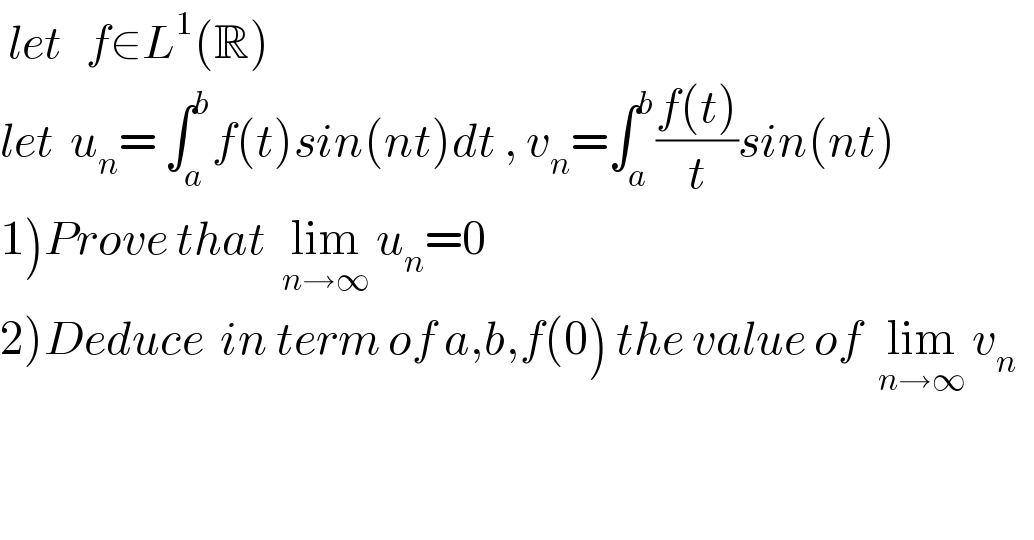
Commented by abdomathmax last updated on 02/Feb/20
![1) by parts u=f and v^′ =sin(nt) ⇒ u_n =[−(1/n)cos(nt)f(t)]_a ^b +(1/n)∫_a ^b f^′ (t)cos(nt)dt =(1/n){f(a)cos(na)−f(b)cos(nb)} +(1/n) ∫_a ^b f^′ (t)cos(nt)dt ⇒ ∣u_n ∣≤(1/n){f(a)+f(b)} +((m(b−a))/n)→0(n→+∞) with m=sup∣f^′ (t)∣_(t∈[a,b])](Q80350.png)
| ||
Question and Answers Forum | ||
Question Number 80334 by ~blr237~ last updated on 02/Feb/20 | ||
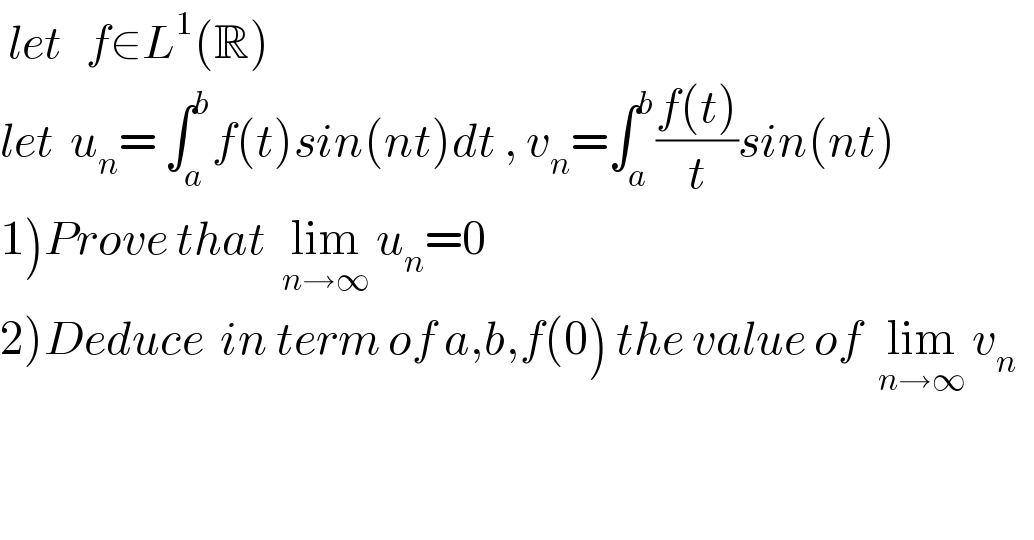 | ||
Commented by abdomathmax last updated on 02/Feb/20 | ||
![1) by parts u=f and v^′ =sin(nt) ⇒ u_n =[−(1/n)cos(nt)f(t)]_a ^b +(1/n)∫_a ^b f^′ (t)cos(nt)dt =(1/n){f(a)cos(na)−f(b)cos(nb)} +(1/n) ∫_a ^b f^′ (t)cos(nt)dt ⇒ ∣u_n ∣≤(1/n){f(a)+f(b)} +((m(b−a))/n)→0(n→+∞) with m=sup∣f^′ (t)∣_(t∈[a,b])](Q80350.png) | ||