
Question and Answers Forum
Question Number 80764 by M±th+et£s last updated on 06/Feb/20
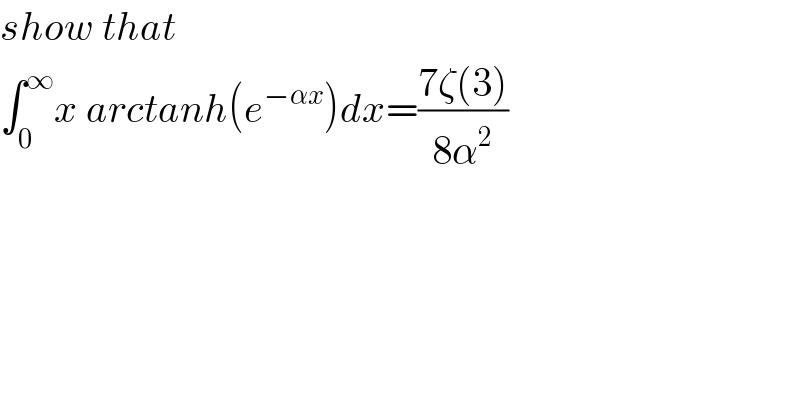
Answered by ~blr237~ last updated on 06/Feb/20

Commented by M±th+et£s last updated on 06/Feb/20

| ||
Question and Answers Forum | ||
Question Number 80764 by M±th+et£s last updated on 06/Feb/20 | ||
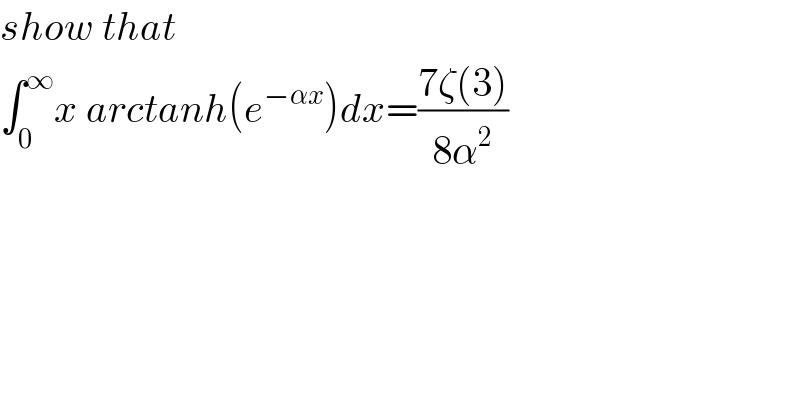 | ||
Answered by ~blr237~ last updated on 06/Feb/20 | ||
 | ||
| ||
Commented by M±th+et£s last updated on 06/Feb/20 | ||
 | ||