Math Question and Answers


Question and Answers Forum

All Questions Topic List
Mechanics Questions
Previous in All Question Next in All Question
Previous in Mechanics Next in Mechanics
Question Number 82057 by ajfour last updated on 17/Feb/20

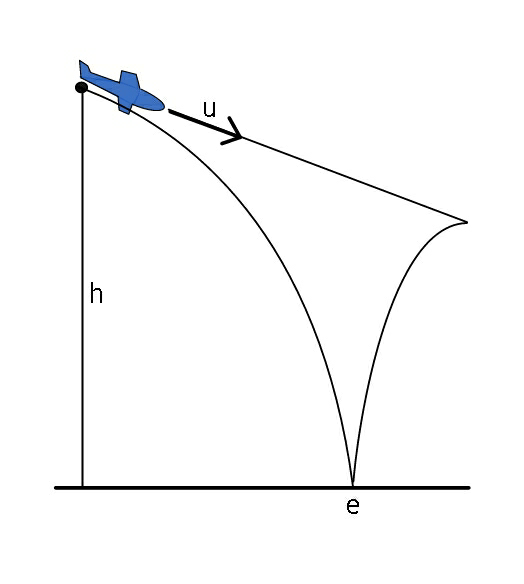
Commented by ajfour last updated on 17/Feb/20

$T h e p l a n e d i v i n g a t s p e e d u d r o p s$ $a b a l l w h i c h u p o n o n e b o u n c e$ $c o l l i d e s w i t h t h e p l a n e w h e n$ $i t r e a c h e s i t s m a x i m u m h e i g h t .$ $F i n d t h e d e c l i n a t i o n o f \bar{u} f r o m$ $h o r i z o n t a l .$
	Answered by mr W last updated on 18/Feb/20

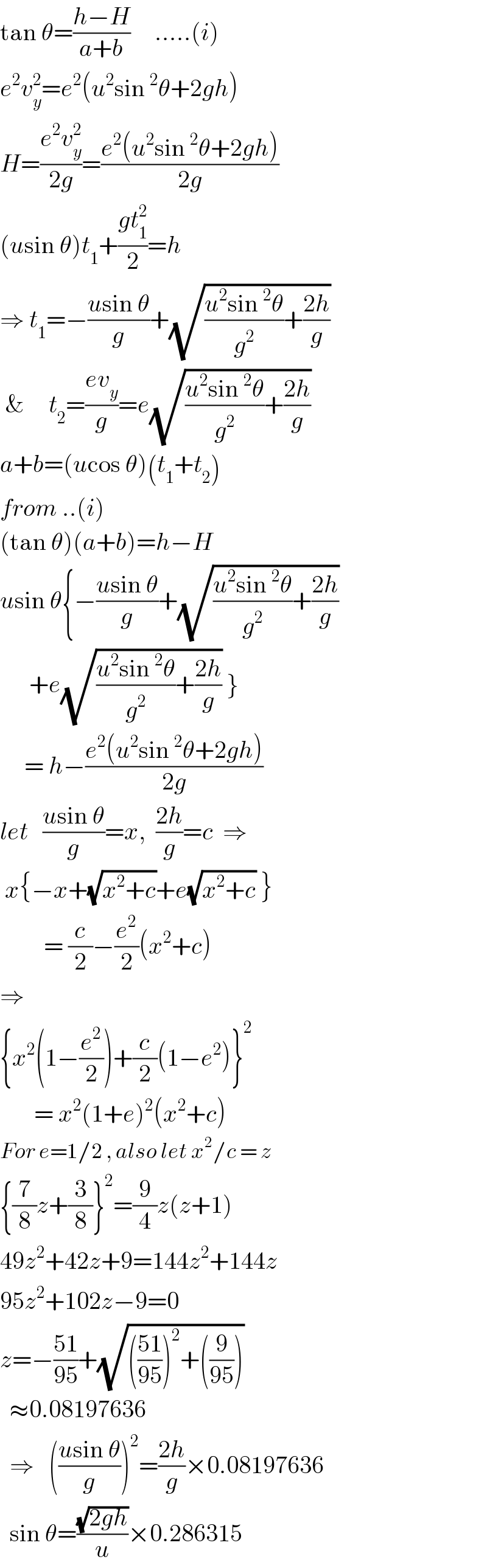
	Commented by mr W last updated on 18/Feb/20

	$e \sqrt{2 g h + {(u \sin θ)}^{2}} = \sqrt{2 {g h}_{2}}$ $\Rightarrow h_{2} = e^{2} (1 + \frac{u^{2} \sin^{2} θ}{2 g h}) h$ $t = \frac{\sqrt{u^{2} \sin^{2} θ + 2 g h} - u \sin θ + \sqrt{2 {g h}_{2}}}{g}$ $t = \frac{L}{u \cos θ} = \frac{h_{1}}{\tan θ u \cos θ} = \frac{h - h_{2}}{u \sin θ}$ $\Rightarrow L = \frac{1}{\tan θ} (1 - e^{2} - \frac{e^{2} u^{2} \sin^{2} θ}{2 g h}) h$ $\frac{\sqrt{u^{2} \sin^{2} θ + 2 g h} - u \sin θ + \sqrt{2 {g h}_{2}}}{g} = \frac{h}{u \sin θ} - \frac{h_{2}}{u \sin θ}$ $\sqrt{u^{2} \sin^{2} θ + 2 g h} - u \sin θ + \sqrt{2 {g h e}^{2} (1 + \frac{u^{2} \sin^{2} θ}{2 g h})} = \frac{g h}{u \sin θ} - \frac{g h}{u \sin θ} e^{2} (1 + \frac{u^{2} \sin^{2} θ}{2 g h})$ $(1 + e) \sqrt{u^{2} \sin^{2} θ + 2 g h} = \frac{(1 - e^{2}) g h}{u \sin θ} + \frac{(2 - e^{2}) u \sin θ}{2}$ $2 (1 + e) \sqrt{1 + \frac{2 g h}{u^{2} \sin^{2} θ}} = \frac{(1 - e^{2}) 2 g h}{u^{2} \sin^{2} θ} + 2 - e^{2}$ $l e t λ = \frac{2 g h}{u^{2} \sin^{2} θ}$ $2 (1 + e) \sqrt{1 + λ} = (1 - e^{2}) λ + 2 - e^{2}$ ${(1 - e^{2})}^{2} λ^{2} - 2 e (1 + e) (4 + e - e^{2}) λ - e (8 + 7 e - e^{3}) = 0$ $λ = \frac{e (4 + e - e^{2}) + \sqrt{e (8 + 7 e + 2 e^{2} - e^{3})}}{(1 + e) {(1 - e)}^{2}}$ $\Rightarrow \frac{2 g h}{u^{2} \sin^{2} θ} = \frac{e (4 + e - e^{2}) + \sqrt{e (8 + 7 e + 2 e^{2} - e^{3})}}{(1 + e) {(1 - e)}^{2}}$ $\Rightarrow θ = \sin^{- 1} [\frac{(1 - e) \sqrt{1 + e}}{\sqrt{e (4 + e - e^{2}) + \sqrt{e (8 + 7 e + 2 e^{2} - e^{3})}}} \sqrt{\frac{2 g h}{u^{2}}}]$ $e x a m p l e : e = 0.5$ $θ \approx \sin^{- 1} (0.286716 \sqrt{\frac{2 g h}{u^{2}}})$
	Commented by ajfour last updated on 18/Feb/20

	$V e r y N i c e, S i r . A w f u l l y G o o d!$
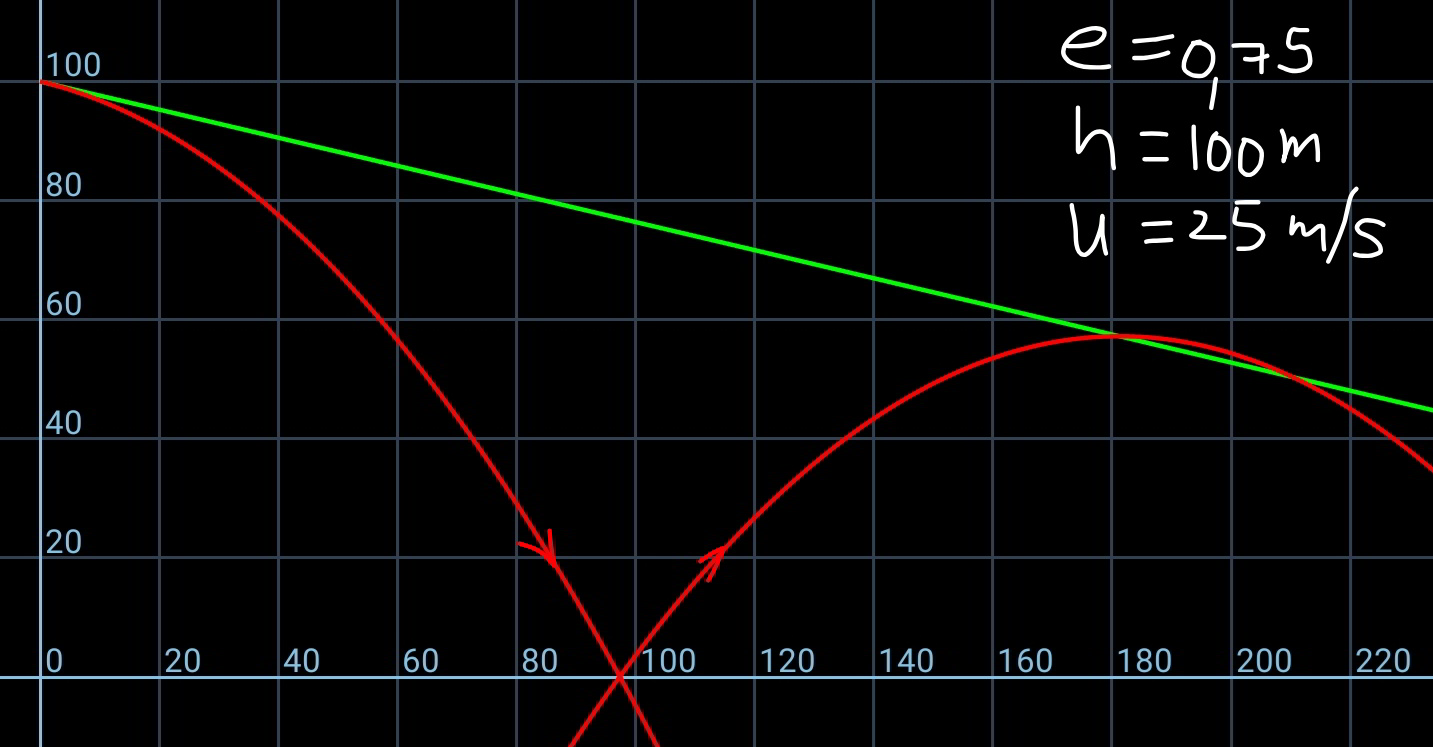
	Commented by mr W last updated on 18/Feb/20

	Commented by mr W last updated on 18/Feb/20

	Commented by mr W last updated on 18/Feb/20

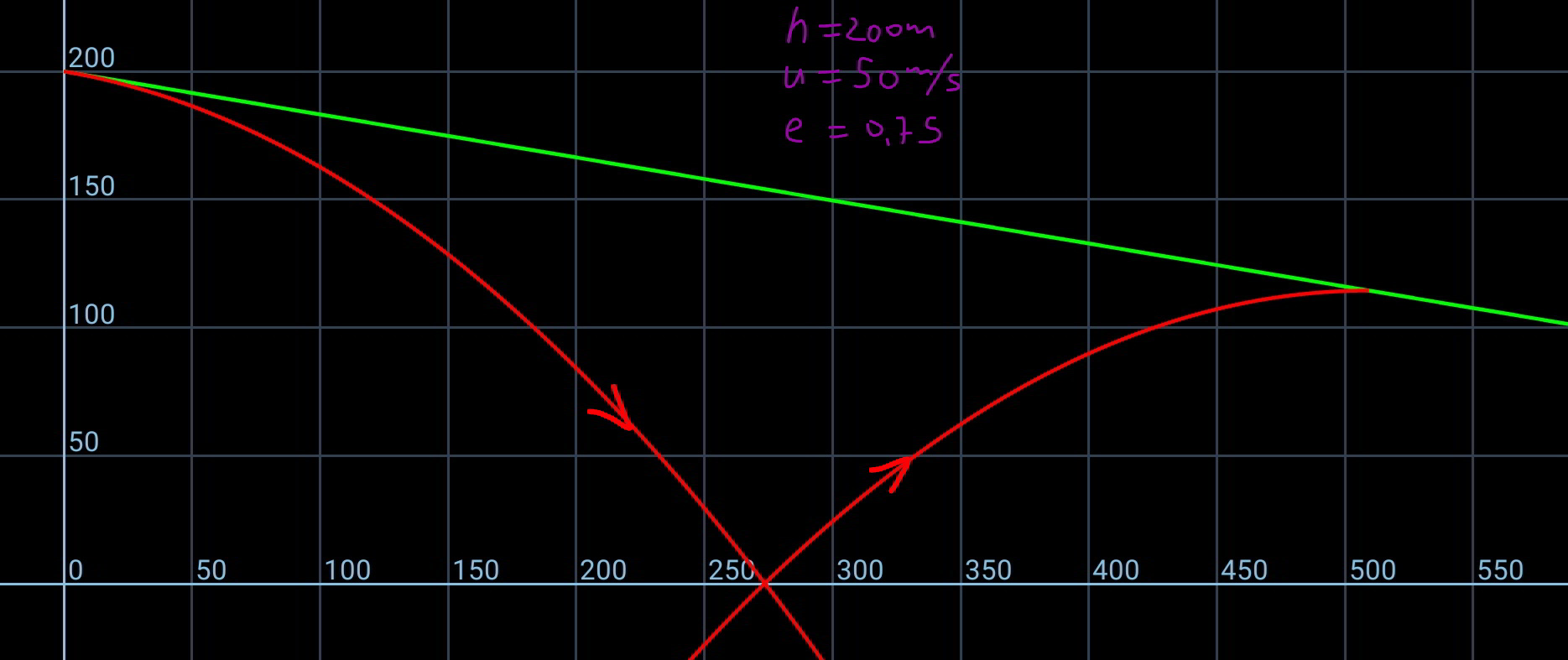
	Answered by ajfour last updated on 18/Feb/20
	$tan θ=((h−H)/(a+b)) .....(i) e^2 v_y ^2 =e^2 (u^2 sin^2 θ+2gh) H=((e^2 v_y ^2 )/(2g))=((e^2 (u^2 sin^2 θ+2gh))/(2g)) (usin θ)t_1 +((gt_1 ^2 )/2)=h ⇒ t_1 =−((usin θ)/g)+(√(((u^2 sin^2 θ)/g^2 )+((2h)/g))) & t_2 =((ev_y )/g)=e(√(((u^2 sin^2 θ)/g^2 )+((2h)/g))) a+b=(ucos θ)(t_1 +t_2 ) from ..(i) (tan θ)(a+b)=h−H usin θ{−((usin θ)/g)+(√(((u^2 sin^2 θ)/g^2 )+((2h)/g))) +e(√(((u^2 sin^2 θ)/g^2 )+((2h)/g))) } = h−((e^2 (u^2 sin^2 θ+2gh))/(2g)) let ((usin θ)/g)=x, ((2h)/g)=c ⇒ x{−x+(√(x^2 +c))+e(√(x^2 +c)) } = (c/2)−(e^2 /2)(x^2 +c) ⇒ {x^2 (1−(e^2 /2))+(c/2)(1−e^2 )}^2 = x^2 (1+e)^2 (x^2 +c) For e=1/2 , also let x^2 /c = z {(7/8)z+(3/8)}^2 =(9/4)z(z+1) 49z^2 +42z+9=144z^2 +144z 95z^2 +102z−9=0 z=−((51)/(95))+(√((((51)/(95)))^2 +((9/(95))))) ≈0.08197636 ⇒ (((usin θ)/g))^2 =((2h)/g)×0.08197636 sin θ=((√(2gh))/u)×0.286315$
	$\tan θ = \frac{h - H}{a + b} . . . . . (i)$ $e^{2} v_{y}^{2} = e^{2} (u^{2} \sin^{2} θ + 2 g h)$ $H = \frac{e^{2} v_{y}^{2}}{2 g} = \frac{e^{2} (u^{2} \sin^{2} θ + 2 g h)}{2 g}$ $(u \sin θ) t_{1} + \frac{{g t}_{1}^{2}}{2} = h$ $\Rightarrow t_{1} = - \frac{u \sin θ}{g} + \sqrt{\frac{u^{2} \sin^{2} θ}{g^{2}} + \frac{2 h}{g}}$ $& t_{2} = \frac{{e v}_{y}}{g} = e \sqrt{\frac{u^{2} \sin^{2} θ}{g^{2}} + \frac{2 h}{g}}$ $a + b = (u \cos θ) (t_{1} + t_{2})$ $f r o m . . (i)$ $(\tan θ) (a + b) = h - H$ $u \sin θ {- \frac{u \sin θ}{g} + \sqrt{\frac{u^{2} \sin^{2} θ}{g^{2}} + \frac{2 h}{g}}$ $+ e \sqrt{\frac{u^{2} \sin^{2} θ}{g^{2}} + \frac{2 h}{g}}}$ $= h - \frac{e^{2} (u^{2} \sin^{2} θ + 2 g h)}{2 g}$ $l e t \frac{u \sin θ}{g} = x, \frac{2 h}{g} = c \Rightarrow$ $x {- x + \sqrt{x^{2} + c} + e \sqrt{x^{2} + c}}$ $= \frac{c}{2} - \frac{e^{2}}{2} (x^{2} + c)$ $\Rightarrow$ ${x^{2} (1 - \frac{e^{2}}{2}) + \frac{c}{2} (1 - e^{2})}^{2}$ $= x^{2} {(1 + e)}^{2} (x^{2} + c)$ $F o r e = 1 / 2, a l s o l e t x^{2} / c = z$ ${\frac{7}{8} z + \frac{3}{8}}^{2} = \frac{9}{4} z (z + 1)$ $49 z^{2} + 42 z + 9 = 144 z^{2} + 144 z$ $95 z^{2} + 102 z - 9 = 0$ $z = - \frac{51}{95} + \sqrt{{(\frac{51}{95})}^{2} + (\frac{9}{95})}$ $\approx 0.08197636$ $\Rightarrow {(\frac{u \sin θ}{g})}^{2} = \frac{2 h}{g} \times 0.08197636$ $\sin θ = \frac{\sqrt{2 g h}}{u} \times 0.286315$
	Commented by ajfour last updated on 18/Feb/20

	$Yes sir, i agree, i shall reexamine$ $this one later, i intend to try to$ $obtain s_{\max}, s_{\min} of our eql . △ Q$ $presently . .$
	Commented by mr W last updated on 18/Feb/20

	$o u r r e s u l t s d i f f e r f r o m e a c h o t h e r .$ $i {c a n}^{'} t s a y w h e r e m y o r y o u r e r r o r i s .$ $i g r a p h e d m y r e s u l t f o r v a r i o u s c a s e s,$ $b o t h c u r v e s m e e t e x a c t l y o n a p o i n t$ $o n t h e x - a x i s, s o m y r e s u l t s e e m s t o$ $b e o k .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum