
Question and Answers Forum
Question Number 82131 by mr W last updated on 18/Feb/20

Commented by ajfour last updated on 18/Feb/20
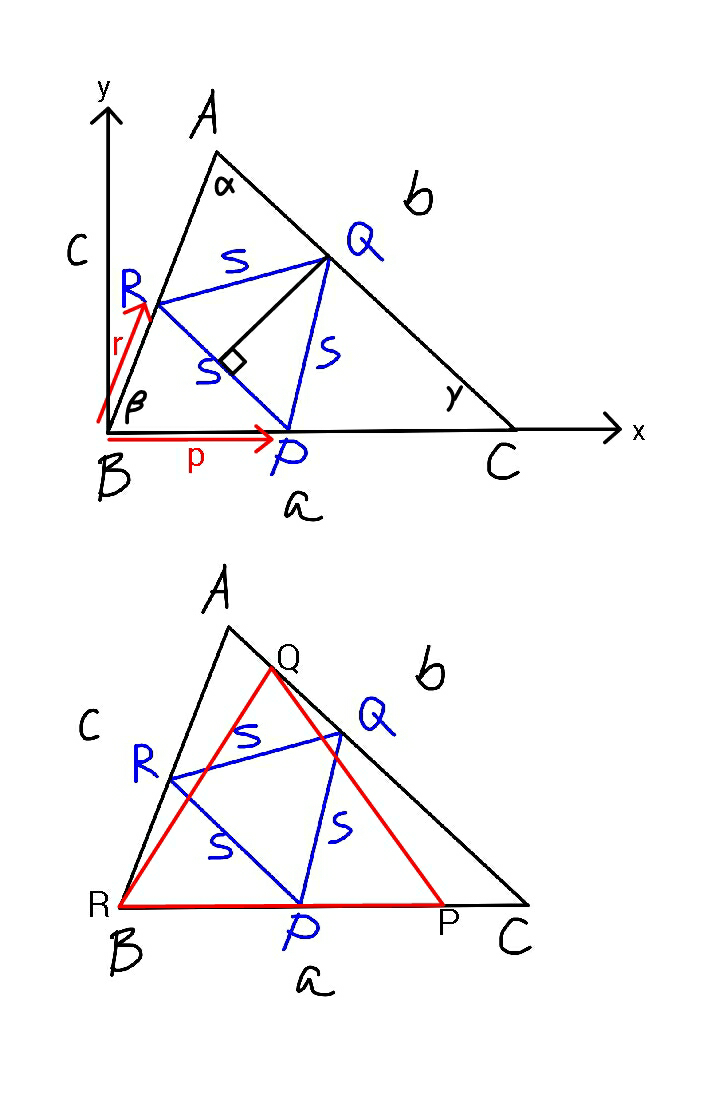
Commented by mr W last updated on 18/Feb/20
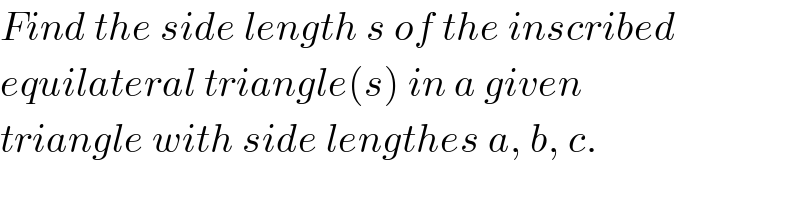
Commented by ajfour last updated on 18/Feb/20

Commented by mr W last updated on 18/Feb/20
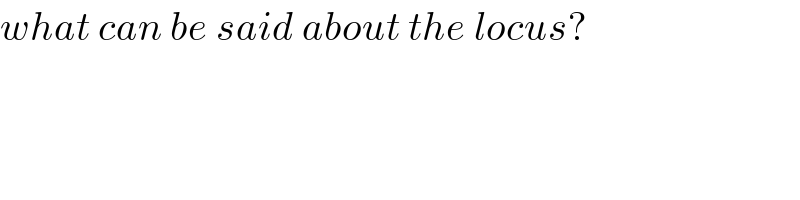
Answered by mr W last updated on 19/Feb/20

Commented by mr W last updated on 19/Feb/20

Commented by mr W last updated on 19/Feb/20
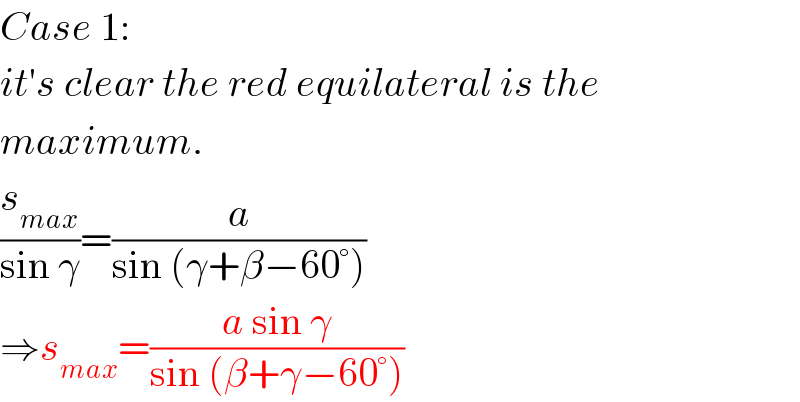
Commented by mr W last updated on 19/Feb/20

Commented by mr W last updated on 20/Feb/20
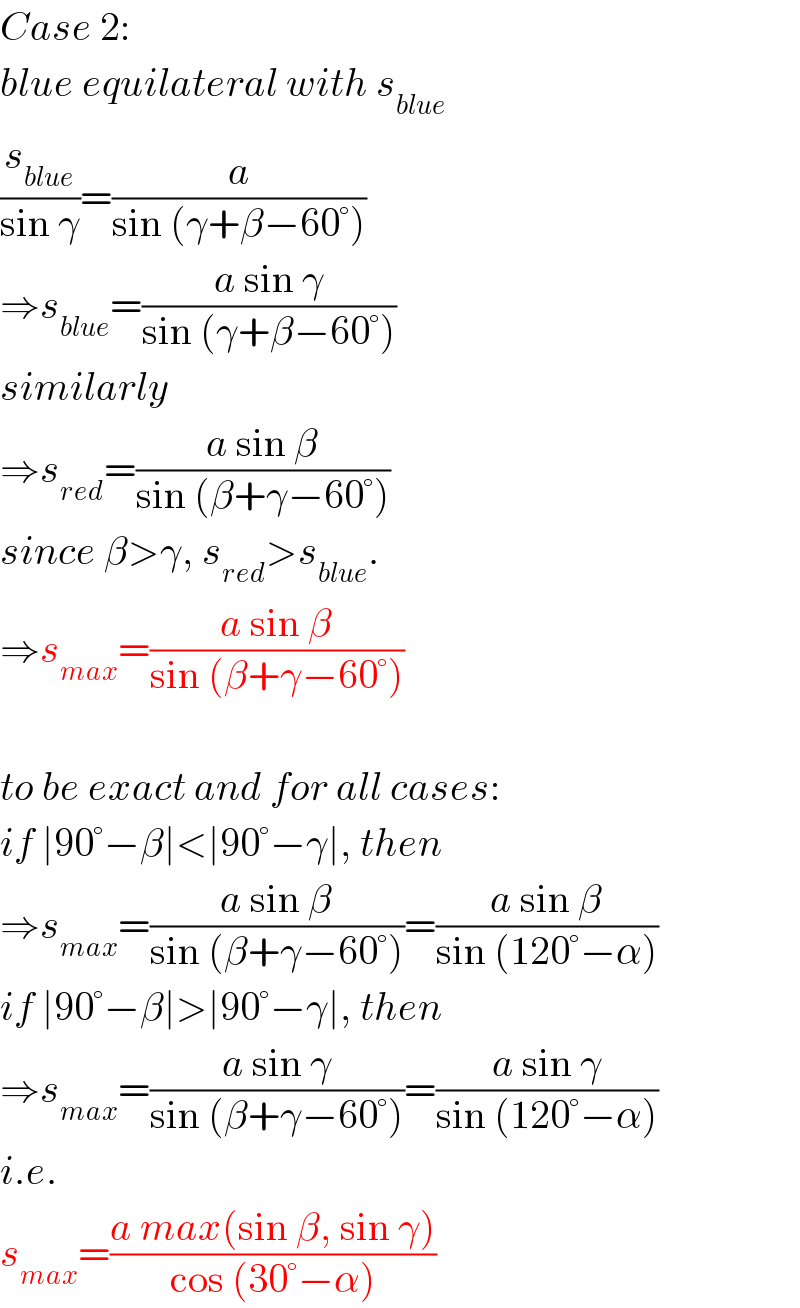
Answered by mr W last updated on 19/Feb/20

Commented by mr W last updated on 19/Feb/20

Commented by mr W last updated on 19/Feb/20

Commented by mr W last updated on 19/Feb/20
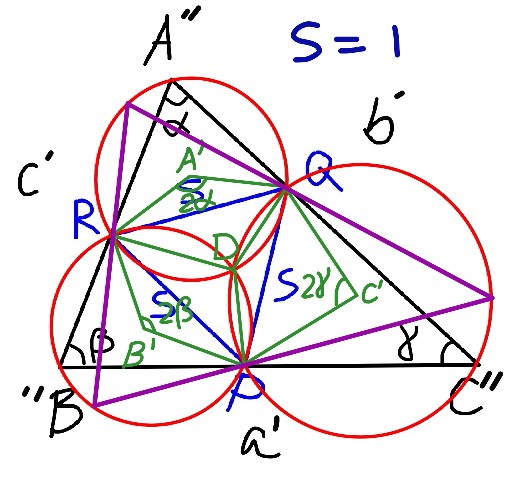
Commented by mr W last updated on 19/Feb/20
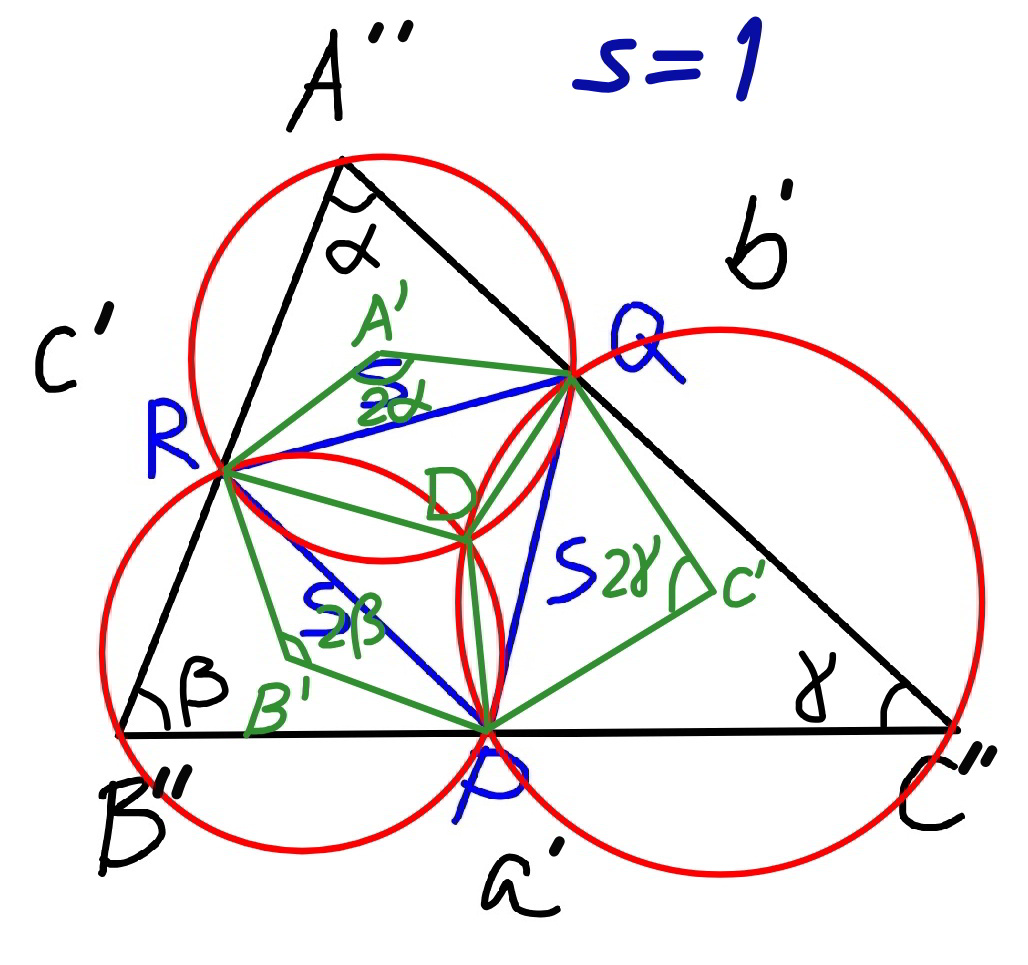
Commented by mr W last updated on 20/Feb/20
![r_A =(s/(2 sin α))=(1/(2 sin α)) r_B =(s/(2 sin β))=(1/(2 sin β)) r_C =(s/(2 sin γ))=(1/(2 sin γ)) when its vertexes lie on the three circles with radii r_A ,r_B ,r_C and centers at A′,B′,C′, then the circumtriangle always has the inner angles α,β,γ. the maximum circumtriangle is A′′B′′C′′. we know this is the case when A′′D, B′′D and C′′D are the diameters of the circles. B′C′^2 =r_B ^2 +r_C ^2 −2r_B r_C cos (60°+90°−β+90°−γ) B′C′^2 =r_B ^2 +r_C ^2 −2r_B r_C cos (60°+α°) B′C′^2 =(1/4)[(1/(sin^2 β))+(1/(sin^2 γ))−((2 cos (60°+α))/(sin β sin γ))] B′C′=(1/2)(√((1/(sin^2 β))+(1/(sin^2 γ))−((2 cos (60°+α))/(sin β sin γ)))) a′=B′′C′′=2B′C′ ⇒a′=(√((1/(sin^2 β))+(1/(sin^2 γ))−((2 cos (60°+α))/(sin β sin γ)))) similarly ⇒b′=(√((1/(sin^2 γ))+(1/(sin^2 α))−((2 cos (60°+β))/(sin γ sin α)))) ⇒c′=(√((1/(sin^2 α))+(1/(sin^2 β))−((2 cos (60°+γ))/(sin α sin β)))) as described above, s_(min) =(a/(a′)) s_(min) =(a/(√((1/(sin^2 β))+(1/(sin^2 γ))−((2 cos (60°+α))/(sin β sin γ))))) or s_(min) =(b/(√((1/(sin^2 γ))+(1/(sin^2 α))−((2 cos (60°+β))/(sin γ sin a))))) or s_(min) =(c/(√((1/(sin^2 α))+(1/(sin^2 β))−((2 cos (60°+γ))/(sin α sin β))))) sin α=(a/(2R)), sin β=(b/(2R)), sin γ=(c/(2R)) cos (60°+γ)=(1/2)(cos γ−(√3) sin γ) cos (60°+γ)=(1/2)(((a^2 +b^2 −c^2 )/(2ab))−(((√3)c)/(2R))) s_(min) =(c/(√(((4R^2 )/a^2 )+((4R^2 )/b^2 )−((4R^2 )/(ab))(((a^2 +b^2 −c^2 )/(2ab))−(((√3)c)/(2R)))))) s_(min) =(c/(√(4R^2 (((a^2 +b^2 +c^2 )/(2a^2 b^2 )))+((2R(√3)c)/(ab))))) s_(min) =(1/(√(((2R^2 )/(a^2 b^2 c^2 ))(a^2 +b^2 +c^2 )+((2R(√3))/(abc))))) with R=((abc)/(4Δ)) s_(min) =(1/(√((1/(8Δ^2 ))(a^2 +b^2 +c^2 )+((√3)/(2Δ))))) ⇒s_(min) =((2(√2)Δ)/(√(a^2 +b^2 +c^2 +4(√3)Δ))) with Δ=area of ΔABC.](Q82263.png)
Commented by mr W last updated on 19/Feb/20
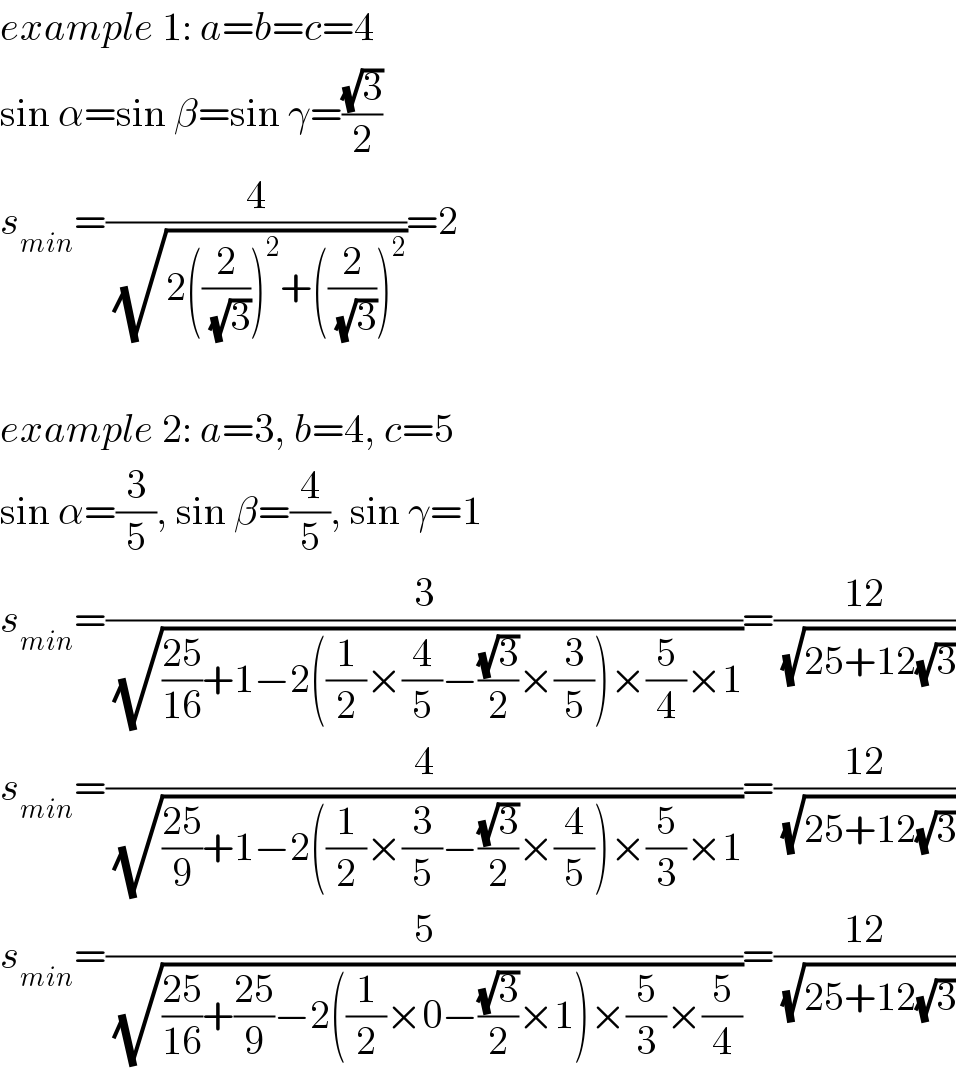
Commented by mr W last updated on 19/Feb/20
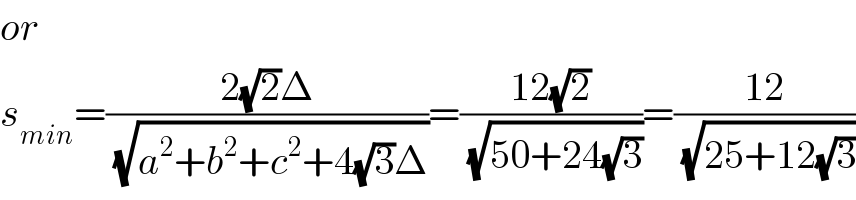
Commented by ajfour last updated on 22/Feb/20

Commented by mr W last updated on 23/Feb/20

